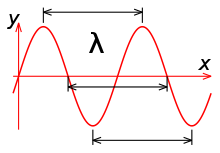
The wavelength of a sine wave, λ, can be measured between any two points with the same phase, such as between crests (on top), or troughs (on bottom), or corresponding zero crossings as shown.
In physics, the wavelength is the spatial period of a periodic wave—the distance over which the wave’s shape repeats.[1][2] It is the distance between consecutive corresponding points of the same phase on the wave, such as two adjacent crests, troughs, or zero crossings, and is a characteristic of both traveling waves and standing waves, as well as other spatial wave patterns.[3][4] The inverse of the wavelength is called the spatial frequency. Wavelength is commonly designated by the Greek letter lambda (λ).
The term wavelength is also sometimes applied to modulated waves, and to the sinusoidal envelopes of modulated waves or waves formed by interference of several sinusoids.[5]
Assuming a sinusoidal wave moving at a fixed wave speed, wavelength is inversely proportional to frequency of the wave: waves with higher frequencies have shorter wavelengths, and lower frequencies have longer wavelengths.[6]
Wavelength depends on the medium (for example, vacuum, air, or water) that a wave travels through. Examples of waves are sound waves, light, water waves and periodic electrical signals in a conductor. A sound wave is a variation in air pressure, while in light and other electromagnetic radiation the strength of the electric and the magnetic field vary. Water waves are variations in the height of a body of water. In a crystal lattice vibration, atomic positions vary.
The range of wavelengths or frequencies for wave phenomena is called a spectrum. The name originated with the visible light spectrum but now can be applied to the entire electromagnetic spectrum as well as to a sound spectrum or vibration spectrum.
Sinusoidal waves[edit]
In linear media, any wave pattern can be described in terms of the independent propagation of sinusoidal components. The wavelength λ of a sinusoidal waveform traveling at constant speed 
where 

In the case of electromagnetic radiation—such as light—in free space, the phase speed is the speed of light, about 3×108 m/s. Thus the wavelength of a 100 MHz electromagnetic (radio) wave is about: 3×108 m/s divided by 108 Hz = 3 metres. The wavelength of visible light ranges from deep red, roughly 700 nm, to violet, roughly 400 nm (for other examples, see electromagnetic spectrum).
For sound waves in air, the speed of sound is 343 m/s (at room temperature and atmospheric pressure). The wavelengths of sound frequencies audible to the human ear (20 Hz–20 kHz) are thus between approximately 17 m and 17 mm, respectively. Somewhat higher frequencies are used by bats so they can resolve targets smaller than 17 mm. Wavelengths in audible sound are much longer than those in visible light.
Sinusoidal standing waves in a box that constrains the end points to be nodes will have an integer number of half wavelengths fitting in the box.
A standing wave (black) depicted as the sum of two propagating waves traveling in opposite directions (red and blue)
Standing waves[edit]
A standing wave is an undulatory motion that stays in one place. A sinusoidal standing wave includes stationary points of no motion, called nodes, and the wavelength is twice the distance between nodes.
The upper figure shows three standing waves in a box. The walls of the box are considered to require the wave to have nodes at the walls of the box (an example of boundary conditions) determining which wavelengths are allowed. For example, for an electromagnetic wave, if the box has ideal metal walls, the condition for nodes at the walls results because the metal walls cannot support a tangential electric field, forcing the wave to have zero amplitude at the wall.
The stationary wave can be viewed as the sum of two traveling sinusoidal waves of oppositely directed velocities.[8] Consequently, wavelength, period, and wave velocity are related just as for a traveling wave. For example, the speed of light can be determined from observation of standing waves in a metal box containing an ideal vacuum.
Mathematical representation[edit]
Traveling sinusoidal waves are often represented mathematically in terms of their velocity v (in the x direction), frequency f and wavelength λ as:
where y is the value of the wave at any position x and time t, and A is the amplitude of the wave. They are also commonly expressed in terms of wavenumber k (2π times the reciprocal of wavelength) and angular frequency ω (2π times the frequency) as:
in which wavelength and wavenumber are related to velocity and frequency as:
or
In the second form given above, the phase (kx − ωt) is often generalized to (k•r − ωt), by replacing the wavenumber k with a wave vector that specifies the direction and wavenumber of a plane wave in 3-space, parameterized by position vector r. In that case, the wavenumber k, the magnitude of k, is still in the same relationship with wavelength as shown above, with v being interpreted as scalar speed in the direction of the wave vector. The first form, using reciprocal wavelength in the phase, does not generalize as easily to a wave in an arbitrary direction.
Generalizations to sinusoids of other phases, and to complex exponentials, are also common; see plane wave. The typical convention of using the cosine phase instead of the sine phase when describing a wave is based on the fact that the cosine is the real part of the complex exponential in the wave
General media[edit]
Wavelength is decreased in a medium with slower propagation.
Refraction: upon entering a medium where its speed is lower, the wave changes direction.
Separation of colors by a prism (click for animation)
The speed of a wave depends upon the medium in which it propagates. In particular, the speed of light in a medium is less than in vacuum, which means that the same frequency will correspond to a shorter wavelength in the medium than in vacuum, as shown in the figure at right.
This change in speed upon entering a medium causes refraction, or a change in direction of waves that encounter the interface between media at an angle.[9] For electromagnetic waves, this change in the angle of propagation is governed by Snell’s law.
The wave velocity in one medium not only may differ from that in another, but the velocity typically varies with wavelength. As a result, the change in direction upon entering a different medium changes with the wavelength of the wave.
For electromagnetic waves the speed in a medium is governed by its refractive index according to
where c is the speed of light in vacuum and n(λ0) is the refractive index of the medium at wavelength λ0, where the latter is measured in vacuum rather than in the medium. The corresponding wavelength in the medium is
When wavelengths of electromagnetic radiation are quoted, the wavelength in vacuum usually is intended unless the wavelength is specifically identified as the wavelength in some other medium. In acoustics, where a medium is essential for the waves to exist, the wavelength value is given for a specified medium.
The variation in speed of light with wavelength is known as dispersion, and is also responsible for the familiar phenomenon in which light is separated into component colors by a prism. Separation occurs when the refractive index inside the prism varies with wavelength, so different wavelengths propagate at different speeds inside the prism, causing them to refract at different angles. The mathematical relationship that describes how the speed of light within a medium varies with wavelength is known as a dispersion relation.
Nonuniform media[edit]
Various local wavelengths on a crest-to-crest basis in an ocean wave approaching shore[10]
Wavelength can be a useful concept even if the wave is not periodic in space. For example, in an ocean wave approaching shore, shown in the figure, the incoming wave undulates with a varying local wavelength that depends in part on the depth of the sea floor compared to the wave height. The analysis of the wave can be based upon comparison of the local wavelength with the local water depth.[10]
A sinusoidal wave travelling in a nonuniform medium, with loss
Waves that are sinusoidal in time but propagate through a medium whose properties vary with position (an inhomogeneous medium) may propagate at a velocity that varies with position, and as a result may not be sinusoidal in space. The figure at right shows an example. As the wave slows down, the wavelength gets shorter and the amplitude increases; after a place of maximum response, the short wavelength is associated with a high loss and the wave dies out.
The analysis of differential equations of such systems is often done approximately, using the WKB method (also known as the Liouville–Green method). The method integrates phase through space using a local wavenumber, which can be interpreted as indicating a «local wavelength» of the solution as a function of time and space.[11][12]
This method treats the system locally as if it were uniform with the local properties; in particular, the local wave velocity associated with a frequency is the only thing needed to estimate the corresponding local wavenumber or wavelength. In addition, the method computes a slowly changing amplitude to satisfy other constraints of the equations or of the physical system, such as for conservation of energy in the wave.
Crystals[edit]
A wave on a line of atoms can be interpreted according to a variety of wavelengths.
Waves in crystalline solids are not continuous, because they are composed of vibrations of discrete particles arranged in a regular lattice. This produces aliasing because the same vibration can be considered to have a variety of different wavelengths, as shown in the figure.[13] Descriptions using more than one of these wavelengths are redundant; it is conventional to choose the longest wavelength that fits the phenomenon. The range of wavelengths sufficient to provide a description of all possible waves in a crystalline medium corresponds to the wave vectors confined to the Brillouin zone.[14]
This indeterminacy in wavelength in solids is important in the analysis of wave phenomena such as energy bands and lattice vibrations. It is mathematically equivalent to the aliasing of a signal that is sampled at discrete intervals.
More general waveforms[edit]
Near-periodic waves over shallow water
The concept of wavelength is most often applied to sinusoidal, or nearly sinusoidal, waves, because in a linear system the sinusoid is the unique shape that propagates with no shape change – just a phase change and potentially an amplitude change.[15] The wavelength (or alternatively wavenumber or wave vector) is a characterization of the wave in space, that is functionally related to its frequency, as constrained by the physics of the system. Sinusoids are the simplest traveling wave solutions, and more complex solutions can be built up by superposition.
In the special case of dispersion-free and uniform media, waves other than sinusoids propagate with unchanging shape and constant velocity. In certain circumstances, waves of unchanging shape also can occur in nonlinear media; for example, the figure shows ocean waves in shallow water that have sharper crests and flatter troughs than those of a sinusoid, typical of a cnoidal wave,[16] a traveling wave so named because it is described by the Jacobi elliptic function of m-th order, usually denoted as cn(x; m).[17] Large-amplitude ocean waves with certain shapes can propagate unchanged, because of properties of the nonlinear surface-wave medium.[18]
Wavelength of a periodic but non-sinusoidal waveform.
If a traveling wave has a fixed shape that repeats in space or in time, it is a periodic wave.[19] Such waves are sometimes regarded as having a wavelength even though they are not sinusoidal.[20] As shown in the figure, wavelength is measured between consecutive corresponding points on the waveform.
Wave packets[edit]
A propagating wave packet
Localized wave packets, «bursts» of wave action where each wave packet travels as a unit, find application in many fields of physics. A wave packet has an envelope that describes the overall amplitude of the wave; within the envelope, the distance between adjacent peaks or troughs is sometimes called a local wavelength.[21][22] An example is shown in the figure. In general, the envelope of the wave packet moves at a speed different from the constituent waves.[23]
Using Fourier analysis, wave packets can be analyzed into infinite sums (or integrals) of sinusoidal waves of different wavenumbers or wavelengths.[24]
Louis de Broglie postulated that all particles with a specific value of momentum p have a wavelength λ = h/p, where h is Planck’s constant. This hypothesis was at the basis of quantum mechanics. Nowadays, this wavelength is called the de Broglie wavelength. For example, the electrons in a CRT display have a De Broglie wavelength of about 10−13 m. To prevent the wave function for such a particle being spread over all space, de Broglie proposed using wave packets to represent particles that are localized in space.[25] The spatial spread of the wave packet, and the spread of the wavenumbers of sinusoids that make up the packet, correspond to the uncertainties in the particle’s position and momentum, the product of which is bounded by Heisenberg uncertainty principle.[24]
Interference and diffraction[edit]
Double-slit interference[edit]
Pattern of light intensity on a screen for light passing through two slits. The labels on the right refer to the difference of the path lengths from the two slits, which are idealized here as point sources.
When sinusoidal waveforms add, they may reinforce each other (constructive interference) or cancel each other (destructive interference) depending upon their relative phase. This phenomenon is used in the interferometer. A simple example is an experiment due to Young where light is passed through two slits.[26]
As shown in the figure, light is passed through two slits and shines on a screen. The path of the light to a position on the screen is different for the two slits, and depends upon the angle θ the path makes with the screen. If we suppose the screen is far enough from the slits (that is, s is large compared to the slit separation d) then the paths are nearly parallel, and the path difference is simply d sin θ. Accordingly, the condition for constructive interference is:[27]
where m is an integer, and for destructive interference is:
Thus, if the wavelength of the light is known, the slit separation can be determined from the interference pattern or fringes, and vice versa.
For multiple slits, the pattern is[28]
where q is the number of slits, and g is the grating constant. The first factor, I1, is the single-slit result, which modulates the more rapidly varying second factor that depends upon the number of slits and their spacing. In the figure I1 has been set to unity, a very rough approximation.
The effect of interference is to redistribute the light, so the energy contained in the light is not altered, just where it shows up.[29]
Single-slit diffraction[edit]
Diffraction pattern of a double slit has a single-slit envelope.
The notion of path difference and constructive or destructive interference used above for the double-slit experiment applies as well to the display of a single slit of light intercepted on a screen. The main result of this interference is to spread out the light from the narrow slit into a broader image on the screen. This distribution of wave energy is called diffraction.
Two types of diffraction are distinguished, depending upon the separation between the source and the screen: Fraunhofer diffraction or far-field diffraction at large separations and Fresnel diffraction or near-field diffraction at close separations.
In the analysis of the single slit, the non-zero width of the slit is taken into account, and each point in the aperture is taken as the source of one contribution to the beam of light (Huygens’ wavelets). On the screen, the light arriving from each position within the slit has a different path length, albeit possibly a very small difference. Consequently, interference occurs.
In the Fraunhofer diffraction pattern sufficiently far from a single slit, within a small-angle approximation, the intensity spread S is related to position x via a squared sinc function:[30]
with
where L is the slit width, R is the distance of the pattern (on the screen) from the slit, and λ is the wavelength of light used. The function S has zeros where u is a non-zero integer, where are at x values at a separation proportion to wavelength.
Diffraction-limited resolution[edit]
Diffraction is the fundamental limitation on the resolving power of optical instruments, such as telescopes (including radiotelescopes) and microscopes.[31]
For a circular aperture, the diffraction-limited image spot is known as an Airy disk; the distance x in the single-slit diffraction formula is replaced by radial distance r and the sine is replaced by 2J1, where J1 is a first order Bessel function.[32]
The resolvable spatial size of objects viewed through a microscope is limited according to the Rayleigh criterion, the radius to the first null of the Airy disk, to a size proportional to the wavelength of the light used, and depending on the numerical aperture:[33]
where the numerical aperture is defined as 
The angular size of the central bright portion (radius to first null of the Airy disk) of the image diffracted by a circular aperture, a measure most commonly used for telescopes and cameras, is:[34]
where λ is the wavelength of the waves that are focused for imaging, D the entrance pupil diameter of the imaging system, in the same units, and the angular resolution δ is in radians.
As with other diffraction patterns, the pattern scales in proportion to wavelength, so shorter wavelengths can lead to higher resolution.
Subwavelength[edit]
The term subwavelength is used to describe an object having one or more dimensions smaller than the length of the wave with which the object interacts. For example, the term subwavelength-diameter optical fibre means an optical fibre whose diameter is less than the wavelength of light propagating through it.
A subwavelength particle is a particle smaller than the wavelength of light with which it interacts (see Rayleigh scattering). Subwavelength apertures are holes smaller than the wavelength of light propagating through them. Such structures have applications in extraordinary optical transmission, and zero-mode waveguides, among other areas of photonics.
Subwavelength may also refer to a phenomenon involving subwavelength objects; for example, subwavelength imaging.
Angular wavelength[edit]
Relationship between wavelength, angular wavelength, and other wave properties.
A quantity related to the wavelength is the angular wavelength (also known as reduced wavelength), usually symbolized by ƛ (lambda-bar). It is equal to the «regular» wavelength «reduced» by a factor of 2π (ƛ = λ/2π). It is usually encountered in quantum mechanics, where it is used in combination with the reduced Planck constant (symbol ħ, h-bar) and the angular frequency (symbol ω) or angular wavenumber (symbol k). It is also used to measure the amplitude of mechanical waves, where the amplitude is equal to displacement divided by reduced wavelength.
See also[edit]
- Emission spectrum
- Envelope (waves)
- Fraunhofer lines – dark lines in the solar spectrum, traditionally used as standard optical wavelength references
- Index of wave articles
- Length measurement
- Spectral line
- Spectroscopy
- Spectrum
References[edit]
- ^
Hecht, Eugene (1987). Optics (2nd ed.). Addison Wesley. pp. 15–16. ISBN 0-201-11609-X. - ^
Brian Hilton Flowers (2000). «§21.2 Periodic functions». An introduction to numerical methods in C++ (2nd ed.). Cambridge University Press. p. 473. ISBN 0-19-850693-7. - ^
Raymond A. Serway; John W. Jewett (2006). Principles of physics (4th ed.). Cengage Learning. pp. 404, 440. ISBN 0-534-49143-X. - ^
A. A. Sonin (1995). The surface physics of liquid crystals. Taylor & Francis. p. 17. ISBN 2-88124-995-7. - ^
Keqian Zhang & Dejie Li (2007). Electromagnetic Theory for Microwaves and Optoelectronics. Springer. p. 533. ISBN 978-3-540-74295-1. - ^
Theo Koupelis & Karl F. Kuhn (2007). In Quest of the Universe. Jones & Bartlett Publishers. p. 102. ISBN 978-0-7637-4387-1.wavelength lambda light sound frequency wave speed.
- ^
David C. Cassidy; Gerald James Holton; Floyd James Rutherford (2002). Understanding physics. Birkhäuser. pp. 339 ff. ISBN 0-387-98756-8. - ^
John Avison (1999). The World of Physics. Nelson Thornes. p. 460. ISBN 978-0-17-438733-6. - ^
To aid imagination, this bending of the wave often is compared to the analogy of a column of marching soldiers crossing from solid ground into mud. See, for example, Raymond T. Pierrehumbert (2010). Principles of Planetary Climate. Cambridge University Press. p. 327. ISBN 978-0-521-86556-2. - ^ a b
Paul R Pinet (2009). op. cit. p. 242. ISBN 978-0-7637-5993-3. - ^
Bishwanath Chakraborty (2007). Principles of Plasma Mechanics. New Age International. p. 454. ISBN 978-81-224-1446-2. - ^
Jeffrey A. Hogan & Joseph D. Lakey (2005). Time-frequency and time-scale methods: adaptive decompositions, uncertainty principles, and sampling. Birkhäuser. p. 348. ISBN 978-0-8176-4276-1. - ^ See Figure 4.20 in A. Putnis (1992). Introduction to mineral sciences. Cambridge University Press. p. 97. ISBN 0-521-42947-1. and Figure 2.3 in Martin T. Dove (1993). Introduction to lattice dynamics (4th ed.). Cambridge University Press. p. 22. ISBN 0-521-39293-4.
- ^ Manijeh Razeghi (2006). Fundamentals of solid state engineering (2nd ed.). Birkhäuser. pp. 165 ff. ISBN 0-387-28152-5.
- ^
See Lord Rayleigh (1890). «Wave theory». Encyclopædia Britannica (9th ed.). The Henry G Allen Company. p. 422. - ^
Valery N. Pilipchuk (2010). «Figure 4.4: Transition from quasi-harmonic to cnoidal wave». Nonlinear Dynamics: Between Linear and Impact Limits. Springer. p. 127. ISBN 978-3642127984. - ^
Andrei Ludu (2012). «§18.3 Special functions». Nonlinear Waves and Solitons on Contours and Closed Surfaces (2nd ed.). Springer. pp. 469 ff. ISBN 978-3642228940. - ^ Alfred Osborne (2010). «Chapter 1: Brief history and overview of nonlinear water waves». Nonlinear Ocean Waves and the Inverse Scattering Transform. Academic Press. pp. 3 ff. ISBN 978-0-12-528629-9.
- ^
Alexander McPherson (2009). «Waves and their properties». Introduction to Macromolecular Crystallography (2 ed.). Wiley. p. 77. ISBN 978-0-470-18590-2. - ^
Eric Stade (2011). Fourier Analysis. John Wiley & Sons. p. 1. ISBN 978-1-118-16551-5. - ^
Peter R. Holland (1995). The Quantum Theory of Motion: An Account of the de Broglie–Bohm Causal Interpretation of Quantum Mechanics. Cambridge University Press. p. 160. ISBN 978-0-521-48543-2. - ^
Jeffery Cooper (1998). Introduction to partial differential equations with MATLAB. Springer. p. 272. ISBN 0-8176-3967-5.The local wavelength λ of a dispersing wave is twice the distance between two successive zeros. … the local wavelength and the local wave number k are related by k = 2π / λ.
- ^ A. T. Fromhold (1991). «Wave packet solutions». Quantum Mechanics for Applied Physics and Engineering (Reprint of Academic Press 1981 ed.). Courier Dover Publications. pp. 59 ff. ISBN 0-486-66741-3.
(p. 61) … the individual waves move more slowly than the packet and therefore pass back through the packet as it advances
- ^ a b See, for example, Figs. 2.8–2.10 in
Joy Manners (2000). «Heisenberg’s uncertainty principle». Quantum Physics: An Introduction. CRC Press. pp. 53–56. ISBN 978-0-7503-0720-8. - ^
Ming Chiang Li (1980). «Electron Interference». In L. Marton; Claire Marton (eds.). Advances in Electronics and Electron Physics. Vol. 53. Academic Press. p. 271. ISBN 0-12-014653-3. - ^
Greenfield Sluder & David E. Wolf (2007). «IV. Young’s Experiment: Two-Slit Interference». Digital microscopy (3rd ed.). Academic Press. p. 15. ISBN 978-0-12-374025-0. - ^
Halliday, Resnick, Walker (2008). «§35-4 Young’s interference experiment». Fundamentals of Physics (Extended 8th ed.). Wiley-India. p. 965. ISBN 978-81-265-1442-7.{{cite book}}: CS1 maint: multiple names: authors list (link) - ^
Kordt Griepenkerl (2002). «§9.8.2 Diffraction by a grating». In John W Harris; Walter Benenson; Horst Stöcker; Holger Lutz (eds.). Handbook of physics. Springer. pp. 307 ff. ISBN 0-387-95269-1. - ^
Douglas B. Murphy (2002). Fundamentals of light microscopy and electronic imaging. Wiley/IEEE. p. 64. ISBN 0-471-23429-X. - ^ John C. Stover (1995). Optical scattering: measurement and analysis (2nd ed.). SPIE Press. p. 64. ISBN 978-0-8194-1934-7.
- ^
Graham Saxby (2002). «Diffraction limitation». The science of imaging. CRC Press. p. 57. ISBN 0-7503-0734-X. - ^
Grant R. Fowles (1989). Introduction to Modern Optics. Courier Dover Publications. pp. 117–120. ISBN 978-0-486-65957-2. - ^
James B. Pawley (1995). Handbook of biological confocal microscopy (2nd ed.). Springer. p. 112. ISBN 978-0-306-44826-3. - ^
Ray N. Wilson (2004). Reflecting Telescope Optics I: Basic Design Theory and Its Historical Development. Springer. p. 302. ISBN 978-3-540-40106-3.
External links[edit]
Wikimedia Commons has media related to Wavelength.
- Conversion: Wavelength to Frequency and vice versa – Sound waves and radio waves
- Teaching resource for 14–16 years on sound including wavelength
- The visible electromagnetic spectrum displayed in web colors with according wavelengths
The wavelength of a sine wave, λ, can be measured between any two points with the same phase, such as between crests (on top), or troughs (on bottom), or corresponding zero crossings as shown.
In physics, the wavelength is the spatial period of a periodic wave—the distance over which the wave’s shape repeats.[1][2] It is the distance between consecutive corresponding points of the same phase on the wave, such as two adjacent crests, troughs, or zero crossings, and is a characteristic of both traveling waves and standing waves, as well as other spatial wave patterns.[3][4] The inverse of the wavelength is called the spatial frequency. Wavelength is commonly designated by the Greek letter lambda (λ).
The term wavelength is also sometimes applied to modulated waves, and to the sinusoidal envelopes of modulated waves or waves formed by interference of several sinusoids.[5]
Assuming a sinusoidal wave moving at a fixed wave speed, wavelength is inversely proportional to frequency of the wave: waves with higher frequencies have shorter wavelengths, and lower frequencies have longer wavelengths.[6]
Wavelength depends on the medium (for example, vacuum, air, or water) that a wave travels through. Examples of waves are sound waves, light, water waves and periodic electrical signals in a conductor. A sound wave is a variation in air pressure, while in light and other electromagnetic radiation the strength of the electric and the magnetic field vary. Water waves are variations in the height of a body of water. In a crystal lattice vibration, atomic positions vary.
The range of wavelengths or frequencies for wave phenomena is called a spectrum. The name originated with the visible light spectrum but now can be applied to the entire electromagnetic spectrum as well as to a sound spectrum or vibration spectrum.
Sinusoidal waves[edit]
In linear media, any wave pattern can be described in terms of the independent propagation of sinusoidal components. The wavelength λ of a sinusoidal waveform traveling at constant speed 
where 

In the case of electromagnetic radiation—such as light—in free space, the phase speed is the speed of light, about 3×108 m/s. Thus the wavelength of a 100 MHz electromagnetic (radio) wave is about: 3×108 m/s divided by 108 Hz = 3 metres. The wavelength of visible light ranges from deep red, roughly 700 nm, to violet, roughly 400 nm (for other examples, see electromagnetic spectrum).
For sound waves in air, the speed of sound is 343 m/s (at room temperature and atmospheric pressure). The wavelengths of sound frequencies audible to the human ear (20 Hz–20 kHz) are thus between approximately 17 m and 17 mm, respectively. Somewhat higher frequencies are used by bats so they can resolve targets smaller than 17 mm. Wavelengths in audible sound are much longer than those in visible light.
Sinusoidal standing waves in a box that constrains the end points to be nodes will have an integer number of half wavelengths fitting in the box.
A standing wave (black) depicted as the sum of two propagating waves traveling in opposite directions (red and blue)
Standing waves[edit]
A standing wave is an undulatory motion that stays in one place. A sinusoidal standing wave includes stationary points of no motion, called nodes, and the wavelength is twice the distance between nodes.
The upper figure shows three standing waves in a box. The walls of the box are considered to require the wave to have nodes at the walls of the box (an example of boundary conditions) determining which wavelengths are allowed. For example, for an electromagnetic wave, if the box has ideal metal walls, the condition for nodes at the walls results because the metal walls cannot support a tangential electric field, forcing the wave to have zero amplitude at the wall.
The stationary wave can be viewed as the sum of two traveling sinusoidal waves of oppositely directed velocities.[8] Consequently, wavelength, period, and wave velocity are related just as for a traveling wave. For example, the speed of light can be determined from observation of standing waves in a metal box containing an ideal vacuum.
Mathematical representation[edit]
Traveling sinusoidal waves are often represented mathematically in terms of their velocity v (in the x direction), frequency f and wavelength λ as:
where y is the value of the wave at any position x and time t, and A is the amplitude of the wave. They are also commonly expressed in terms of wavenumber k (2π times the reciprocal of wavelength) and angular frequency ω (2π times the frequency) as:
in which wavelength and wavenumber are related to velocity and frequency as:
or
In the second form given above, the phase (kx − ωt) is often generalized to (k•r − ωt), by replacing the wavenumber k with a wave vector that specifies the direction and wavenumber of a plane wave in 3-space, parameterized by position vector r. In that case, the wavenumber k, the magnitude of k, is still in the same relationship with wavelength as shown above, with v being interpreted as scalar speed in the direction of the wave vector. The first form, using reciprocal wavelength in the phase, does not generalize as easily to a wave in an arbitrary direction.
Generalizations to sinusoids of other phases, and to complex exponentials, are also common; see plane wave. The typical convention of using the cosine phase instead of the sine phase when describing a wave is based on the fact that the cosine is the real part of the complex exponential in the wave
General media[edit]
Wavelength is decreased in a medium with slower propagation.
Refraction: upon entering a medium where its speed is lower, the wave changes direction.
Separation of colors by a prism (click for animation)
The speed of a wave depends upon the medium in which it propagates. In particular, the speed of light in a medium is less than in vacuum, which means that the same frequency will correspond to a shorter wavelength in the medium than in vacuum, as shown in the figure at right.
This change in speed upon entering a medium causes refraction, or a change in direction of waves that encounter the interface between media at an angle.[9] For electromagnetic waves, this change in the angle of propagation is governed by Snell’s law.
The wave velocity in one medium not only may differ from that in another, but the velocity typically varies with wavelength. As a result, the change in direction upon entering a different medium changes with the wavelength of the wave.
For electromagnetic waves the speed in a medium is governed by its refractive index according to
where c is the speed of light in vacuum and n(λ0) is the refractive index of the medium at wavelength λ0, where the latter is measured in vacuum rather than in the medium. The corresponding wavelength in the medium is
When wavelengths of electromagnetic radiation are quoted, the wavelength in vacuum usually is intended unless the wavelength is specifically identified as the wavelength in some other medium. In acoustics, where a medium is essential for the waves to exist, the wavelength value is given for a specified medium.
The variation in speed of light with wavelength is known as dispersion, and is also responsible for the familiar phenomenon in which light is separated into component colors by a prism. Separation occurs when the refractive index inside the prism varies with wavelength, so different wavelengths propagate at different speeds inside the prism, causing them to refract at different angles. The mathematical relationship that describes how the speed of light within a medium varies with wavelength is known as a dispersion relation.
Nonuniform media[edit]
Various local wavelengths on a crest-to-crest basis in an ocean wave approaching shore[10]
Wavelength can be a useful concept even if the wave is not periodic in space. For example, in an ocean wave approaching shore, shown in the figure, the incoming wave undulates with a varying local wavelength that depends in part on the depth of the sea floor compared to the wave height. The analysis of the wave can be based upon comparison of the local wavelength with the local water depth.[10]
A sinusoidal wave travelling in a nonuniform medium, with loss
Waves that are sinusoidal in time but propagate through a medium whose properties vary with position (an inhomogeneous medium) may propagate at a velocity that varies with position, and as a result may not be sinusoidal in space. The figure at right shows an example. As the wave slows down, the wavelength gets shorter and the amplitude increases; after a place of maximum response, the short wavelength is associated with a high loss and the wave dies out.
The analysis of differential equations of such systems is often done approximately, using the WKB method (also known as the Liouville–Green method). The method integrates phase through space using a local wavenumber, which can be interpreted as indicating a «local wavelength» of the solution as a function of time and space.[11][12]
This method treats the system locally as if it were uniform with the local properties; in particular, the local wave velocity associated with a frequency is the only thing needed to estimate the corresponding local wavenumber or wavelength. In addition, the method computes a slowly changing amplitude to satisfy other constraints of the equations or of the physical system, such as for conservation of energy in the wave.
Crystals[edit]
A wave on a line of atoms can be interpreted according to a variety of wavelengths.
Waves in crystalline solids are not continuous, because they are composed of vibrations of discrete particles arranged in a regular lattice. This produces aliasing because the same vibration can be considered to have a variety of different wavelengths, as shown in the figure.[13] Descriptions using more than one of these wavelengths are redundant; it is conventional to choose the longest wavelength that fits the phenomenon. The range of wavelengths sufficient to provide a description of all possible waves in a crystalline medium corresponds to the wave vectors confined to the Brillouin zone.[14]
This indeterminacy in wavelength in solids is important in the analysis of wave phenomena such as energy bands and lattice vibrations. It is mathematically equivalent to the aliasing of a signal that is sampled at discrete intervals.
More general waveforms[edit]
Near-periodic waves over shallow water
The concept of wavelength is most often applied to sinusoidal, or nearly sinusoidal, waves, because in a linear system the sinusoid is the unique shape that propagates with no shape change – just a phase change and potentially an amplitude change.[15] The wavelength (or alternatively wavenumber or wave vector) is a characterization of the wave in space, that is functionally related to its frequency, as constrained by the physics of the system. Sinusoids are the simplest traveling wave solutions, and more complex solutions can be built up by superposition.
In the special case of dispersion-free and uniform media, waves other than sinusoids propagate with unchanging shape and constant velocity. In certain circumstances, waves of unchanging shape also can occur in nonlinear media; for example, the figure shows ocean waves in shallow water that have sharper crests and flatter troughs than those of a sinusoid, typical of a cnoidal wave,[16] a traveling wave so named because it is described by the Jacobi elliptic function of m-th order, usually denoted as cn(x; m).[17] Large-amplitude ocean waves with certain shapes can propagate unchanged, because of properties of the nonlinear surface-wave medium.[18]
Wavelength of a periodic but non-sinusoidal waveform.
If a traveling wave has a fixed shape that repeats in space or in time, it is a periodic wave.[19] Such waves are sometimes regarded as having a wavelength even though they are not sinusoidal.[20] As shown in the figure, wavelength is measured between consecutive corresponding points on the waveform.
Wave packets[edit]
A propagating wave packet
Localized wave packets, «bursts» of wave action where each wave packet travels as a unit, find application in many fields of physics. A wave packet has an envelope that describes the overall amplitude of the wave; within the envelope, the distance between adjacent peaks or troughs is sometimes called a local wavelength.[21][22] An example is shown in the figure. In general, the envelope of the wave packet moves at a speed different from the constituent waves.[23]
Using Fourier analysis, wave packets can be analyzed into infinite sums (or integrals) of sinusoidal waves of different wavenumbers or wavelengths.[24]
Louis de Broglie postulated that all particles with a specific value of momentum p have a wavelength λ = h/p, where h is Planck’s constant. This hypothesis was at the basis of quantum mechanics. Nowadays, this wavelength is called the de Broglie wavelength. For example, the electrons in a CRT display have a De Broglie wavelength of about 10−13 m. To prevent the wave function for such a particle being spread over all space, de Broglie proposed using wave packets to represent particles that are localized in space.[25] The spatial spread of the wave packet, and the spread of the wavenumbers of sinusoids that make up the packet, correspond to the uncertainties in the particle’s position and momentum, the product of which is bounded by Heisenberg uncertainty principle.[24]
Interference and diffraction[edit]
Double-slit interference[edit]
Pattern of light intensity on a screen for light passing through two slits. The labels on the right refer to the difference of the path lengths from the two slits, which are idealized here as point sources.
When sinusoidal waveforms add, they may reinforce each other (constructive interference) or cancel each other (destructive interference) depending upon their relative phase. This phenomenon is used in the interferometer. A simple example is an experiment due to Young where light is passed through two slits.[26]
As shown in the figure, light is passed through two slits and shines on a screen. The path of the light to a position on the screen is different for the two slits, and depends upon the angle θ the path makes with the screen. If we suppose the screen is far enough from the slits (that is, s is large compared to the slit separation d) then the paths are nearly parallel, and the path difference is simply d sin θ. Accordingly, the condition for constructive interference is:[27]
where m is an integer, and for destructive interference is:
Thus, if the wavelength of the light is known, the slit separation can be determined from the interference pattern or fringes, and vice versa.
For multiple slits, the pattern is[28]
where q is the number of slits, and g is the grating constant. The first factor, I1, is the single-slit result, which modulates the more rapidly varying second factor that depends upon the number of slits and their spacing. In the figure I1 has been set to unity, a very rough approximation.
The effect of interference is to redistribute the light, so the energy contained in the light is not altered, just where it shows up.[29]
Single-slit diffraction[edit]
Diffraction pattern of a double slit has a single-slit envelope.
The notion of path difference and constructive or destructive interference used above for the double-slit experiment applies as well to the display of a single slit of light intercepted on a screen. The main result of this interference is to spread out the light from the narrow slit into a broader image on the screen. This distribution of wave energy is called diffraction.
Two types of diffraction are distinguished, depending upon the separation between the source and the screen: Fraunhofer diffraction or far-field diffraction at large separations and Fresnel diffraction or near-field diffraction at close separations.
In the analysis of the single slit, the non-zero width of the slit is taken into account, and each point in the aperture is taken as the source of one contribution to the beam of light (Huygens’ wavelets). On the screen, the light arriving from each position within the slit has a different path length, albeit possibly a very small difference. Consequently, interference occurs.
In the Fraunhofer diffraction pattern sufficiently far from a single slit, within a small-angle approximation, the intensity spread S is related to position x via a squared sinc function:[30]
with
where L is the slit width, R is the distance of the pattern (on the screen) from the slit, and λ is the wavelength of light used. The function S has zeros where u is a non-zero integer, where are at x values at a separation proportion to wavelength.
Diffraction-limited resolution[edit]
Diffraction is the fundamental limitation on the resolving power of optical instruments, such as telescopes (including radiotelescopes) and microscopes.[31]
For a circular aperture, the diffraction-limited image spot is known as an Airy disk; the distance x in the single-slit diffraction formula is replaced by radial distance r and the sine is replaced by 2J1, where J1 is a first order Bessel function.[32]
The resolvable spatial size of objects viewed through a microscope is limited according to the Rayleigh criterion, the radius to the first null of the Airy disk, to a size proportional to the wavelength of the light used, and depending on the numerical aperture:[33]
where the numerical aperture is defined as 
The angular size of the central bright portion (radius to first null of the Airy disk) of the image diffracted by a circular aperture, a measure most commonly used for telescopes and cameras, is:[34]
where λ is the wavelength of the waves that are focused for imaging, D the entrance pupil diameter of the imaging system, in the same units, and the angular resolution δ is in radians.
As with other diffraction patterns, the pattern scales in proportion to wavelength, so shorter wavelengths can lead to higher resolution.
Subwavelength[edit]
The term subwavelength is used to describe an object having one or more dimensions smaller than the length of the wave with which the object interacts. For example, the term subwavelength-diameter optical fibre means an optical fibre whose diameter is less than the wavelength of light propagating through it.
A subwavelength particle is a particle smaller than the wavelength of light with which it interacts (see Rayleigh scattering). Subwavelength apertures are holes smaller than the wavelength of light propagating through them. Such structures have applications in extraordinary optical transmission, and zero-mode waveguides, among other areas of photonics.
Subwavelength may also refer to a phenomenon involving subwavelength objects; for example, subwavelength imaging.
Angular wavelength[edit]
Relationship between wavelength, angular wavelength, and other wave properties.
A quantity related to the wavelength is the angular wavelength (also known as reduced wavelength), usually symbolized by ƛ (lambda-bar). It is equal to the «regular» wavelength «reduced» by a factor of 2π (ƛ = λ/2π). It is usually encountered in quantum mechanics, where it is used in combination with the reduced Planck constant (symbol ħ, h-bar) and the angular frequency (symbol ω) or angular wavenumber (symbol k). It is also used to measure the amplitude of mechanical waves, where the amplitude is equal to displacement divided by reduced wavelength.
See also[edit]
- Emission spectrum
- Envelope (waves)
- Fraunhofer lines – dark lines in the solar spectrum, traditionally used as standard optical wavelength references
- Index of wave articles
- Length measurement
- Spectral line
- Spectroscopy
- Spectrum
References[edit]
- ^
Hecht, Eugene (1987). Optics (2nd ed.). Addison Wesley. pp. 15–16. ISBN 0-201-11609-X. - ^
Brian Hilton Flowers (2000). «§21.2 Periodic functions». An introduction to numerical methods in C++ (2nd ed.). Cambridge University Press. p. 473. ISBN 0-19-850693-7. - ^
Raymond A. Serway; John W. Jewett (2006). Principles of physics (4th ed.). Cengage Learning. pp. 404, 440. ISBN 0-534-49143-X. - ^
A. A. Sonin (1995). The surface physics of liquid crystals. Taylor & Francis. p. 17. ISBN 2-88124-995-7. - ^
Keqian Zhang & Dejie Li (2007). Electromagnetic Theory for Microwaves and Optoelectronics. Springer. p. 533. ISBN 978-3-540-74295-1. - ^
Theo Koupelis & Karl F. Kuhn (2007). In Quest of the Universe. Jones & Bartlett Publishers. p. 102. ISBN 978-0-7637-4387-1.wavelength lambda light sound frequency wave speed.
- ^
David C. Cassidy; Gerald James Holton; Floyd James Rutherford (2002). Understanding physics. Birkhäuser. pp. 339 ff. ISBN 0-387-98756-8. - ^
John Avison (1999). The World of Physics. Nelson Thornes. p. 460. ISBN 978-0-17-438733-6. - ^
To aid imagination, this bending of the wave often is compared to the analogy of a column of marching soldiers crossing from solid ground into mud. See, for example, Raymond T. Pierrehumbert (2010). Principles of Planetary Climate. Cambridge University Press. p. 327. ISBN 978-0-521-86556-2. - ^ a b
Paul R Pinet (2009). op. cit. p. 242. ISBN 978-0-7637-5993-3. - ^
Bishwanath Chakraborty (2007). Principles of Plasma Mechanics. New Age International. p. 454. ISBN 978-81-224-1446-2. - ^
Jeffrey A. Hogan & Joseph D. Lakey (2005). Time-frequency and time-scale methods: adaptive decompositions, uncertainty principles, and sampling. Birkhäuser. p. 348. ISBN 978-0-8176-4276-1. - ^ See Figure 4.20 in A. Putnis (1992). Introduction to mineral sciences. Cambridge University Press. p. 97. ISBN 0-521-42947-1. and Figure 2.3 in Martin T. Dove (1993). Introduction to lattice dynamics (4th ed.). Cambridge University Press. p. 22. ISBN 0-521-39293-4.
- ^ Manijeh Razeghi (2006). Fundamentals of solid state engineering (2nd ed.). Birkhäuser. pp. 165 ff. ISBN 0-387-28152-5.
- ^
See Lord Rayleigh (1890). «Wave theory». Encyclopædia Britannica (9th ed.). The Henry G Allen Company. p. 422. - ^
Valery N. Pilipchuk (2010). «Figure 4.4: Transition from quasi-harmonic to cnoidal wave». Nonlinear Dynamics: Between Linear and Impact Limits. Springer. p. 127. ISBN 978-3642127984. - ^
Andrei Ludu (2012). «§18.3 Special functions». Nonlinear Waves and Solitons on Contours and Closed Surfaces (2nd ed.). Springer. pp. 469 ff. ISBN 978-3642228940. - ^ Alfred Osborne (2010). «Chapter 1: Brief history and overview of nonlinear water waves». Nonlinear Ocean Waves and the Inverse Scattering Transform. Academic Press. pp. 3 ff. ISBN 978-0-12-528629-9.
- ^
Alexander McPherson (2009). «Waves and their properties». Introduction to Macromolecular Crystallography (2 ed.). Wiley. p. 77. ISBN 978-0-470-18590-2. - ^
Eric Stade (2011). Fourier Analysis. John Wiley & Sons. p. 1. ISBN 978-1-118-16551-5. - ^
Peter R. Holland (1995). The Quantum Theory of Motion: An Account of the de Broglie–Bohm Causal Interpretation of Quantum Mechanics. Cambridge University Press. p. 160. ISBN 978-0-521-48543-2. - ^
Jeffery Cooper (1998). Introduction to partial differential equations with MATLAB. Springer. p. 272. ISBN 0-8176-3967-5.The local wavelength λ of a dispersing wave is twice the distance between two successive zeros. … the local wavelength and the local wave number k are related by k = 2π / λ.
- ^ A. T. Fromhold (1991). «Wave packet solutions». Quantum Mechanics for Applied Physics and Engineering (Reprint of Academic Press 1981 ed.). Courier Dover Publications. pp. 59 ff. ISBN 0-486-66741-3.
(p. 61) … the individual waves move more slowly than the packet and therefore pass back through the packet as it advances
- ^ a b See, for example, Figs. 2.8–2.10 in
Joy Manners (2000). «Heisenberg’s uncertainty principle». Quantum Physics: An Introduction. CRC Press. pp. 53–56. ISBN 978-0-7503-0720-8. - ^
Ming Chiang Li (1980). «Electron Interference». In L. Marton; Claire Marton (eds.). Advances in Electronics and Electron Physics. Vol. 53. Academic Press. p. 271. ISBN 0-12-014653-3. - ^
Greenfield Sluder & David E. Wolf (2007). «IV. Young’s Experiment: Two-Slit Interference». Digital microscopy (3rd ed.). Academic Press. p. 15. ISBN 978-0-12-374025-0. - ^
Halliday, Resnick, Walker (2008). «§35-4 Young’s interference experiment». Fundamentals of Physics (Extended 8th ed.). Wiley-India. p. 965. ISBN 978-81-265-1442-7.{{cite book}}: CS1 maint: multiple names: authors list (link) - ^
Kordt Griepenkerl (2002). «§9.8.2 Diffraction by a grating». In John W Harris; Walter Benenson; Horst Stöcker; Holger Lutz (eds.). Handbook of physics. Springer. pp. 307 ff. ISBN 0-387-95269-1. - ^
Douglas B. Murphy (2002). Fundamentals of light microscopy and electronic imaging. Wiley/IEEE. p. 64. ISBN 0-471-23429-X. - ^ John C. Stover (1995). Optical scattering: measurement and analysis (2nd ed.). SPIE Press. p. 64. ISBN 978-0-8194-1934-7.
- ^
Graham Saxby (2002). «Diffraction limitation». The science of imaging. CRC Press. p. 57. ISBN 0-7503-0734-X. - ^
Grant R. Fowles (1989). Introduction to Modern Optics. Courier Dover Publications. pp. 117–120. ISBN 978-0-486-65957-2. - ^
James B. Pawley (1995). Handbook of biological confocal microscopy (2nd ed.). Springer. p. 112. ISBN 978-0-306-44826-3. - ^
Ray N. Wilson (2004). Reflecting Telescope Optics I: Basic Design Theory and Its Historical Development. Springer. p. 302. ISBN 978-3-540-40106-3.
External links[edit]
Wikimedia Commons has media related to Wavelength.
- Conversion: Wavelength to Frequency and vice versa – Sound waves and radio waves
- Teaching resource for 14–16 years on sound including wavelength
- The visible electromagnetic spectrum displayed in web colors with according wavelengths
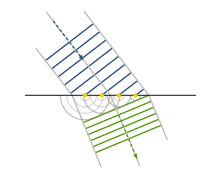
Длина волны — это расстояние между двумя последовательными пиками (гребнями) или впадинами. Самое высокое положение волны называется пиком. Самое нижнее положение волны называется впадиной.
Цикл — это полное колебание, например, кривая между двумя гребнями или двумя впадинами. Максимальное расстояние волны от равновесного положения называется амплитудой.
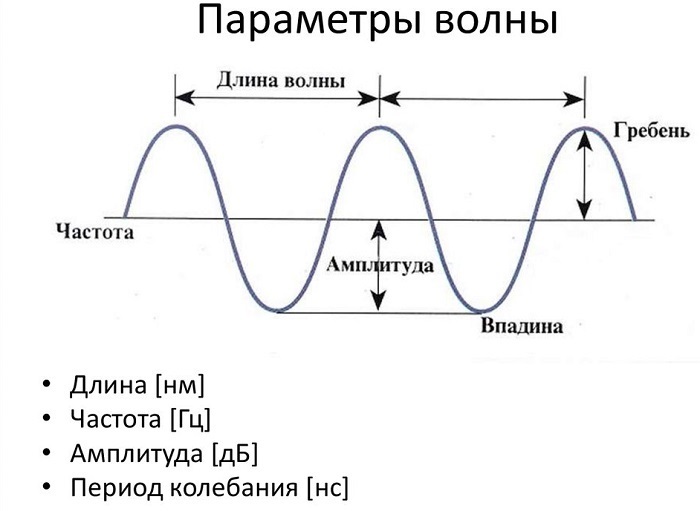
На рисунке показаны основные параметры волны, используемые в физике:
Определение и формула длины волн
Волна — это возмущение, распространяющееся от точки, в которой она возникла, в окружающую среду. Такое возмущение переносит энергию без чистого переноса вещества.
Длина представляет собой фактическое расстояние, пройденное волной, которое не всегда совпадает с расстоянием среды, или частиц, в которых распространяется волна. Ее также определяют как пространственный период волнового процесса.
Греческая буква «λ» (лямбда) в физике используется для обозначения длины в уравнениях. Она обратно пропорциональна частоте волны.
Период Т — время завершения полного колебания, единица измерения секунды (с).
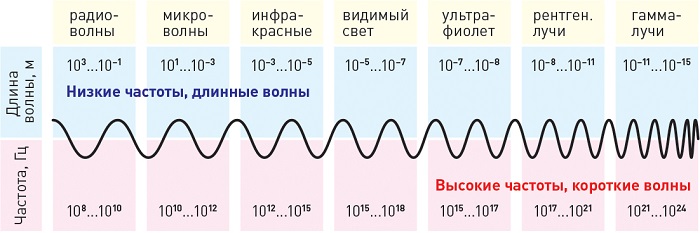
Длинная волна соответствует низкой частоте, а короткая — высокой. Длина измеряется в метрах. Количество волн, излучаемых в каждую секунду, называется частотой и обратно пропорционально периоду.
У различных длин разная скорость распространения. Например, скорость света в воде равна 3/4 от скорости в вакууме.
Пространственный период волны — это расстояние, которое точка с постоянной фазой «пролетает» за интервал времени, соответствующий периоду колебаний.
Частота f — количество полных колебаний в единицу времени. Измеряется в Герцах (Гц).
При одном полном колебании в секунду f = 1 Гц; при 1000 колебаний в секунду f = 1 килогерц (кГц); 1 млн. колебаний в секунду f = 1 мегагерц (1 МГц).
Зная, что скорость света в вакууме с — 300 000 км/с, или 300 000 000 м/с, то для перевода длины волны в частоту нужно 3 х 108 м/с поделить на длину в метрах.
Единицы измерения длины волны λ — нанометры и ангстремы, где нанометр является миллиардной частью метра (1 м = 109 нм) и ангстрем является десятимиллиардной частью метра (1 м = 1010 А), то есть нанометр эквивалентен 10 ангстрем (1 нм = 10 А).
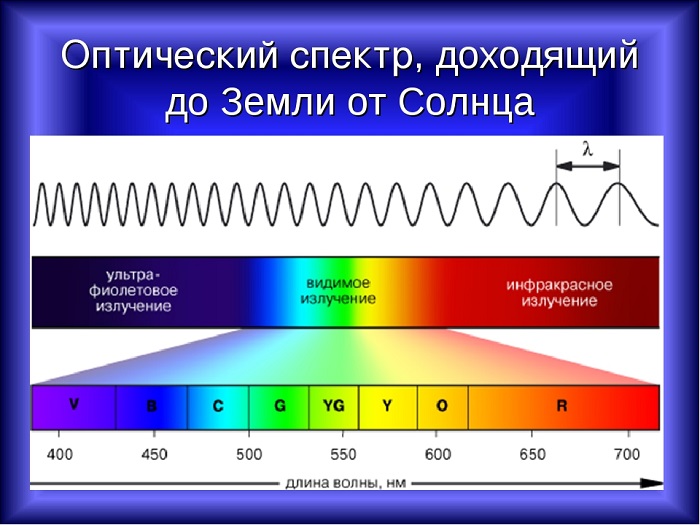
Свет, который исходит от Солнца, является электромагнитным излучением, которое движется со скоростью 300 000 км/с, но длина не одинакова для любого фотона, а колеблется между 400 нм и 700 нм. Длина световой волны влияет на цвет.
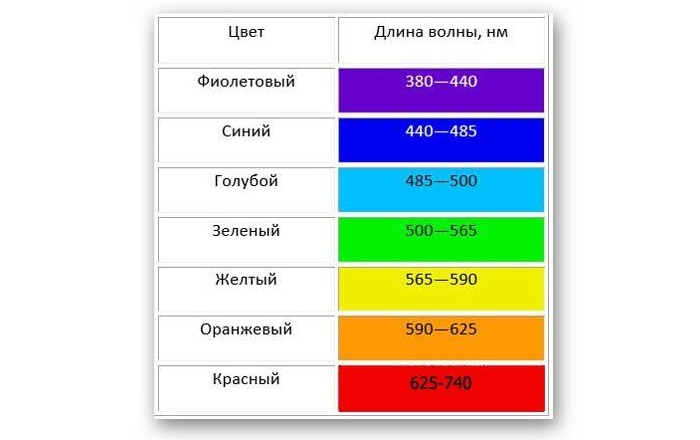
Белый свет разлагается на спектр различных цветных полос, каждая из которых определяется своей длиной волны. Таким образом, светом с наименьшей длиной является фиолетовый, который составляет около 400 нм, а светом с наибольшей длиной — красный, который составляет около 700 нм.
Таблица показывает длину волны в зависимости от цвета:
Излучения с длиной меньше фиолетового называются ультрафиолетовым излучением, рентгеновским и гамма-лучами в порядке уменьшения. Излучения больше красного называются инфракрасными, микроволнами и радиоволнами, в порядке возрастания.
Предельная дальность связи зависит от длины. Размеры антенны часто превышают рабочую длину радиоэлектронного средства.
Рисунок показывает длину волн и частоту (нм), исходящих от различных источников:
Примеры расчета длины волны для звуковых, электромагнитных и радиоволн
Задача №1
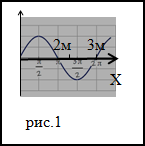
Скорость звука в воде 1450 м/с. На каком расстоянии находятся ближайшие точки, совершающие колебания в противоположных фазах, если частота колебаний равна 725 Гц?
Задача №2
Мимо неподвижного наблюдателя, стоящего на берегу озера, за 6 с. прошло 4 гребня волны. Расстояние между первым и третьим гребнями равно 12 м. Определить период колебания частиц волны, скорость распространения и длину волны.
Задача №3
Голосовые связки певца, поющего тенором (высоким мужским голосом), колеблются с частотой от 130 до 520 Гц. Определите максимальную и минимальную длину излучаемой звуковой волны в воздухе. Скорость звука в воздухе 330 м/с.
Каждая волна имеет свои параметры движения.
Скорость волны — скорость распространения возмущения.
Пример:
воздействуя на стальной стержень с одного конца, можно вызвать волны сжатия и разрежения со скоростью (5000 frac{м}{с}).
Скорость волны зависит от строения вещества и взаимодействия между её молекулами (атомами). Поэтому в различных средах скорость одной и той же волны будет отличаться.
Помимо скорости, важной характеристикой волны является длина волны.
Длина волны — расстояние, на которое распространяется волна за время, равное периоду колебаний в ней.
Рассмотрим процесс передачи колебаний от точки к точке при распространении поперечной волны.
Используется модель, в которой частицы среды заменяют шариками. Для удобства их можно пронумеровать (рис. (1)).
Частицы среды связаны между собой межмолекулярными силами взаимодействия, поэтому волна передаётся от одной частицы к другой.
Рис. (1). Модель упругой среды для демонстрации колебаний
Отклоним первый шарик от положения равновесия. Силы притяжения передадут движение второму, третьему шарику. Каждый элемент вещества (молекула, атом) повторит движение первой частицы с запаздыванием, которые называют сдвигом фазы. Это запаздывание зависит от расстояния, на котором находится рассматриваемый шарик по отношению к первому шарику.
Предположим, что первый шарик достиг максимального смещения от положения равновесия (рис. (2)). В этот момент четвёртый шарик только начнет движение, следовательно, он отстаёт от первого на (1/4) колебания.
Рис. (2). Изображение максимального смещения от положения равновесия первого шарика
В момент времени, когда смещение четвертого шарика будет наибольшим (рис. (3)), седьмой шарик будет отставать от него на (1/4) колебания. А если рассмотреть отставание седьмого шарика от первого, то оно составляет (1/2) колебания.
Рис. (3). Изображение максимального смещения от положения равновесия четвёртого шарика
Между седьмым и четвёртым шариком, а также седьмым и десятым (1/4) часть колебания (рис. (4)).
Рис. (4). Изображение максимального смещения от положения равновесия седьмого шарика
Первый и тринадцатый шарик совершают одно колебание, то есть двигаются в одной фазе (рис. (5)). Это значит, что между ними все шарики с первого по двенадцатый проходят полный колебательный процесс или составляют одну волну.
Рис. (5). Изображение максимального смещения от положения равновесия десятого шарика
Начиная с тринадцатого шарика, мы можем отсчитывать новую волну (рис. (6)).
Рис. (6). Изображение модели новой волны
Длину волны измеряют расстоянием, на которое перемещается волновая поверхность за один период колебания источника волн;
Длиной волны является расстояние между двумя ближайшими точками бегущей волны на одном луче, который колеблется в одинаковой фазе:
, где (λ) («лямбда») — длина волны, (upsilon) — скорость волны, (T) — период колебания.
Период колебаний можно выразить как величину, обратную частоте колебаний:
T=1ν
.
Тогда выразим длину волны как отношение скорости и частоты:
λ=υν
.
Длина волны прямо пропорциональна скорости волны и обратно пропорциональна частоте колебаний (прямо пропорциональна периоду колебаний).
Поперечные и продольные волны описываются одними и теми же законами.
Выразим скорость волны:
как отношение длины волны к периоду колебаний:
υ=λT
;
как произведение длины волны на частоту колебаний:
υ=λν
.
За длину волны (λ) примем расстояние между шариками, колеблющимися в одинаковых фазах. Например (см. рис. (6)), между четвёртым и шестнадцатым, третьим и пятнадцатым.
Колебания проходят шарики, начиная с первого и заканчивая двенадцатым, проходят все фазы колебания. Новая волна начинается с тринадцатого шарика. Каждый шарик совершает одно полное колебание за время, которое называют периодом колебаний (T). За это время колебательный процесс проходит расстояние, называемое длиной волны (λ.)
Модель распространения продольных волн представлена на рисунке (7).
Длиной волны будет расстояние между соседними центрами сжатия пружины.
Рис. (7). Распространение продольных волн в упругой пружине
Источником колебаний генерируется волна той же частоты, поэтому вынужденные колебания совпадают по частоте с осциллятором и не зависит от плотности среды, в которой движется волна.
Если в ходе движения волна переходит в среду другой плотности, то скорость движения волны изменяется, а частота колебаний остаётся прежней.
Источники:
Рис. 1. Модель упругой среды для демонстрации колебаний. © ЯКласс.
Рис. 2. Изображение максимального смещения от положения равновесия первого шарика. © ЯКласс.
Рис. 3. Изображение максимального смещения от положения равновесия четвёртого шарика. © ЯКласс.
Рис. 4. Изображение максимального смещения от положения равновесия седьмого шарика. © ЯКласс.
Рис. 5. Изображение максимального смещения от положения равновесия десятого шарика. © ЯКласс.
Рис. 6. Изображение модели новой волны. © ЯКласс.
Рис. 7. Распространение продольных волн в упругой пружине. © ЯКласс.
Морфемный разбор слова:
Однокоренные слова к слову:
Длина волны
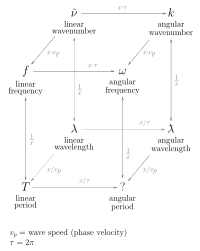
Длина́ волны́ — расстояние между двумя ближайшими друг к другу точками, колеблющимися в одинаковых фазах, обычно длина волны обозначается греческой буквой 

Получить соотношение, связывающее длину волны с фазовой скоростью (


Волнам де Бройля также соответствует определенная длина волны. Частице с энергией Е и импульсом p, соответствуют:
Примеры
Приближённо, с ошибкой около 0,07%, рассчитать длину радиоволны можно так: 300 делим на частоту в мегагерцах, получаем длину волны в метрах, например для 80 Гц, длина волны 3750 километра, для 89 МГц — 3,37 метра, для 2,4 ГГц — 12,5 см.
Точная формула для расчёта длины волны электромагнитного излучения в вакууме выглядит так:
где 
Для определения длины волны электромагнитного излучения в какой-либо среде следует использовать формулу:
где 
Примечания
Литература
Полезное
Смотреть что такое «Длина волны» в других словарях:
длина волны — (λ) Расстояние, на которое смещается поверхность равной фазы волны за один период колебаний. [ГОСТ 7601 78] длина волны Расстояние, проходимое упругой волной за время, равное одному полному периоду колебаний. [BS EN 1330 4:2000. Non… … Справочник технического переводчика
ДЛИНА ВОЛНЫ — (обозначение l), расстояние между следующими друг за другом точками волны, находящимися в одинаковой ФАЗЕ. Например, длину морской волны можно измерить как расстояние от гребня до гребня. Длина волны видимого света (колеблющаяся в пределах от 390 … Научно-технический энциклопедический словарь
ДЛИНА ВОЛНЫ — пространственный период волны, т. е. расстояние между двумя ближайшими точками гармонич. бегущей волны, находящимися в одинаковой фазе колебаний, или удвоенное расстояние между двумя ближайшими узлами или пучностями стоячей волны. Д. в. l связана … Физическая энциклопедия
ДЛИНА ВОЛНЫ — расстояние между двумя ближайшими точками гармонической волны, находящимися в одинаковой фазе. Длина волны l = uT, где T период колебаний, u фазовая скорость волны … Большой Энциклопедический словарь
длина волны — Расстояние по горизонтали между двумя последовательными гребнями или подошвами волны … Словарь по географии
ДЛИНА ВОЛНЫ — (Length of wave) расстояние между гребнями (вершинами) двух смежных волн. Самойлов К. И. Морской словарь. М. Л.: Государственное Военно морское Издательство НКВМФ Союза ССР, 1941 … Морской словарь
длина волны — 3.42 длина волны (wavelength): Расстояние между двумя точками одинаковых фаз двух последовательных волновых циклов, измеряемое в направлении распространения волны. Длина волны λ зависит от фазовой скорости vp и частоты f и рассчитывается по… … Словарь-справочник терминов нормативно-технической документации
ДЛИНА ВОЛНЫ — хар ка гармонич. волны, равная расстоянию между двумя ближайшими точками, разность фаз волны в к рых равна 2ПИ. Д. в. X связана с частотой колебаний v и фазовой скоростью волны u соотношением Лямбда = u/v. См. рис. К ст. Длина волны … Большой энциклопедический политехнический словарь
Источник
Длина волны — формулы, свойства и расчеты
Длина волны — это расстояние между двумя последовательными пиками (гребнями) или впадинами. Самое высокое положение волны называется пиком. Самое нижнее положение волны называется впадиной.
Цикл — это полное колебание, например, кривая между двумя гребнями или двумя впадинами. Максимальное расстояние волны от равновесного положения называется амплитудой.
На рисунке показаны основные параметры волны, используемые в физике:
Определение и формула длины волн
Волна — это возмущение, распространяющееся от точки, в которой она возникла, в окружающую среду. Такое возмущение переносит энергию без чистого переноса вещества.
Длина представляет собой фактическое расстояние, пройденное волной, которое не всегда совпадает с расстоянием среды, или частиц, в которых распространяется волна. Ее также определяют как пространственный период волнового процесса.
Греческая буква «λ» (лямбда) в физике используется для обозначения длины в уравнениях. Она обратно пропорциональна частоте волны.
Период Т — время завершения полного колебания, единица измерения секунды (с).
Длинная волна соответствует низкой частоте, а короткая — высокой. Длина измеряется в метрах. Количество волн, излучаемых в каждую секунду, называется частотой и обратно пропорционально периоду.
У различных длин разная скорость распространения. Например, скорость света в воде равна 3/4 от скорости в вакууме.
Пространственный период волны — это расстояние, которое точка с постоянной фазой «пролетает» за интервал времени, соответствующий периоду колебаний.
Частота f — количество полных колебаний в единицу времени. Измеряется в Герцах (Гц).
При одном полном колебании в секунду f = 1 Гц; при 1000 колебаний в секунду f = 1 килогерц (кГц); 1 млн. колебаний в секунду f = 1 мегагерц (1 МГц).
Зная, что скорость света в вакууме с — 300 000 км/с, или 300 000 000 м/с, то для перевода длины волны в частоту нужно 3 х 10 8 м/с поделить на длину в метрах.
Единицы измерения длины волны λ — нанометры и ангстремы, где нанометр является миллиардной частью метра (1 м = 109 нм) и ангстрем является десятимиллиардной частью метра (1 м = 1010 А), то есть нанометр эквивалентен 10 ангстрем (1 нм = 10 А).
Свет, который исходит от Солнца, является электромагнитным излучением, которое движется со скоростью 300 000 км/с, но длина не одинакова для любого фотона, а колеблется между 400 нм и 700 нм. Длина световой волны влияет на цвет.
Белый свет разлагается на спектр различных цветных полос, каждая из которых определяется своей длиной волны. Таким образом, светом с наименьшей длиной является фиолетовый, который составляет около 400 нм, а светом с наибольшей длиной — красный, который составляет около 700 нм.
Таблица показывает длину волны в зависимости от цвета:
Излучения с длиной меньше фиолетового называются ультрафиолетовым излучением, рентгеновским и гамма-лучами в порядке уменьшения. Излучения больше красного называются инфракрасными, микроволнами и радиоволнами, в порядке возрастания.
Предельная дальность связи зависит от длины. Размеры антенны часто превышают рабочую длину радиоэлектронного средства.
Рисунок показывает длину волн и частоту (нм), исходящих от различных источников:
Примеры расчета длины волны для звуковых, электромагнитных и радиоволн
Задача №1
Скорость звука в воде 1450 м/с. На каком расстоянии находятся ближайшие точки, совершающие колебания в противоположных фазах, если частота колебаний равна 725 Гц?
Задача №2
Мимо неподвижного наблюдателя, стоящего на берегу озера, за 6 с. прошло 4 гребня волны. Расстояние между первым и третьим гребнями равно 12 м. Определить период колебания частиц волны, скорость распространения и длину волны.
Задача №3
Голосовые связки певца, поющего тенором (высоким мужским голосом), колеблются с частотой от 130 до 520 Гц. Определите максимальную и минимальную длину излучаемой звуковой волны в воздухе. Скорость звука в воздухе 330 м/с.
Источник
На рисунке показаны основные параметры волны, используемые в физике:
Определение и формула длины волн
Длина представляет собой фактическое расстояние, пройденное волной, которое не всегда совпадает с расстоянием среды, или частиц, в которых распространяется волна. Ее также определяют как пространственный период волнового процесса.
Греческая буква «λ» (лямбда) в физике используется для обозначения длины в уравнениях. Она обратно пропорциональна частоте волны.
Период Т — время завершения полного колебания, единица измерения секунды (с).
У различных длин разная скорость распространения. Например, скорость света в воде равна 3/4 от скорости в вакууме.
Частота f — количество полных колебаний в единицу времени. Измеряется в Герцах (Гц).
При одном полном колебании в секунду f = 1 Гц; при 1000 колебаний в секунду f = 1 килогерц (кГц); 1 млн. колебаний в секунду f = 1 мегагерц (1 МГц).
Зная, что скорость света в вакууме с — 300 000 км/с, или 300 000 000 м/с, то для перевода длины волны в частоту нужно 3 х 10 8 м/с поделить на длину в метрах.
Свет, который исходит от Солнца, является электромагнитным излучением, которое движется со скоростью 300 000 км/с, но длина не одинакова для любого фотона, а колеблется между 400 нм и 700 нм. Длина световой волны влияет на цвет.
Таблица показывает длину волны в зависимости от цвета:
Излучения с длиной меньше фиолетового называются ультрафиолетовым излучением, рентгеновским и гамма-лучами в порядке уменьшения. Излучения больше красного называются инфракрасными, микроволнами и радиоволнами, в порядке возрастания.
Предельная дальность связи зависит от длины. Размеры антенны часто превышают рабочую длину радиоэлектронного средства.
Рисунок показывает длину волн и частоту (нм), исходящих от различных источников:
Примеры расчета длины волны для звуковых, электромагнитных и радиоволн
Задача №1
Скорость звука в воде 1450 м/с. На каком расстоянии находятся ближайшие точки, совершающие колебания в противоположных фазах, если частота колебаний равна 725 Гц?
Задача №2
Мимо неподвижного наблюдателя, стоящего на берегу озера, за 6 с. прошло 4 гребня волны. Расстояние между первым и третьим гребнями равно 12 м. Определить период колебания частиц волны, скорость распространения и длину волны.
Задача №3
Голосовые связки певца, поющего тенором (высоким мужским голосом), колеблются с частотой от 130 до 520 Гц. Определите максимальную и минимальную длину излучаемой звуковой волны в воздухе. Скорость звука в воздухе 330 м/с.
Источник
Длина волны
Статья находится на проверке у методистов Skysmart.
Если вы заметили ошибку, сообщите об этом в онлайн-чат
(в правом нижнем углу экрана).
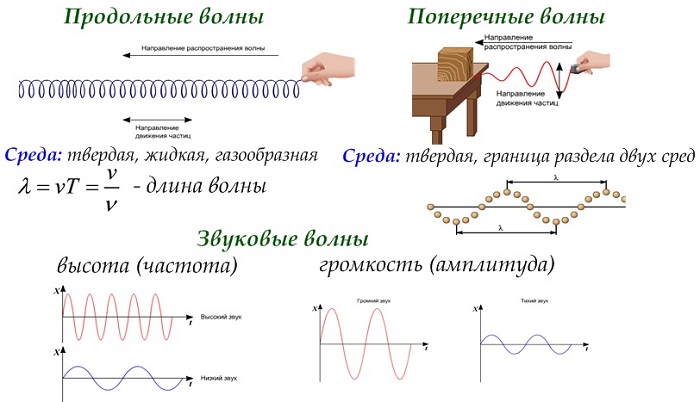
Волна: продольная и поперечная
Начнем с того, что волна — это распространение колебания в пространстве.
Волны бывают механическими и электромагнитными.
Механические волны — это те волны, колебания которых можно почувствовать физически, потому что они распространяются в упругой среде.
Представьте, что вы стоите на железнодорожных путях. Нет, вы не Анна Каренина, вы — экспериментатор.
Если к вам приближается поезд, вы рано или поздно его услышите. Вернее, услышите, как только звуковая волна со скоростью ? = 330 м/с достигнет ваших ушей.
Если приложить ухо к рельсу, то это произойдет значительно быстрее, потому что скорость звука в твердом теле больше, чем в воздухе. Кстати, под водой скорость звука больше, чем в воздухе, но меньше, чем в твердых телах.
Если вы когда-нибудь трогали музыкальную колонку, то знаете, что звук чувствуется и на ощупь.
Электромагнитные волны — это те волны, которые мы потрогать не можем.
Для них работают все те же самые законы, просто их скорость значительно больше и равна скорости света ? = 3*10^8 м/с. И источники у них разные.
Волны также принято делить на продольные и поперечные:
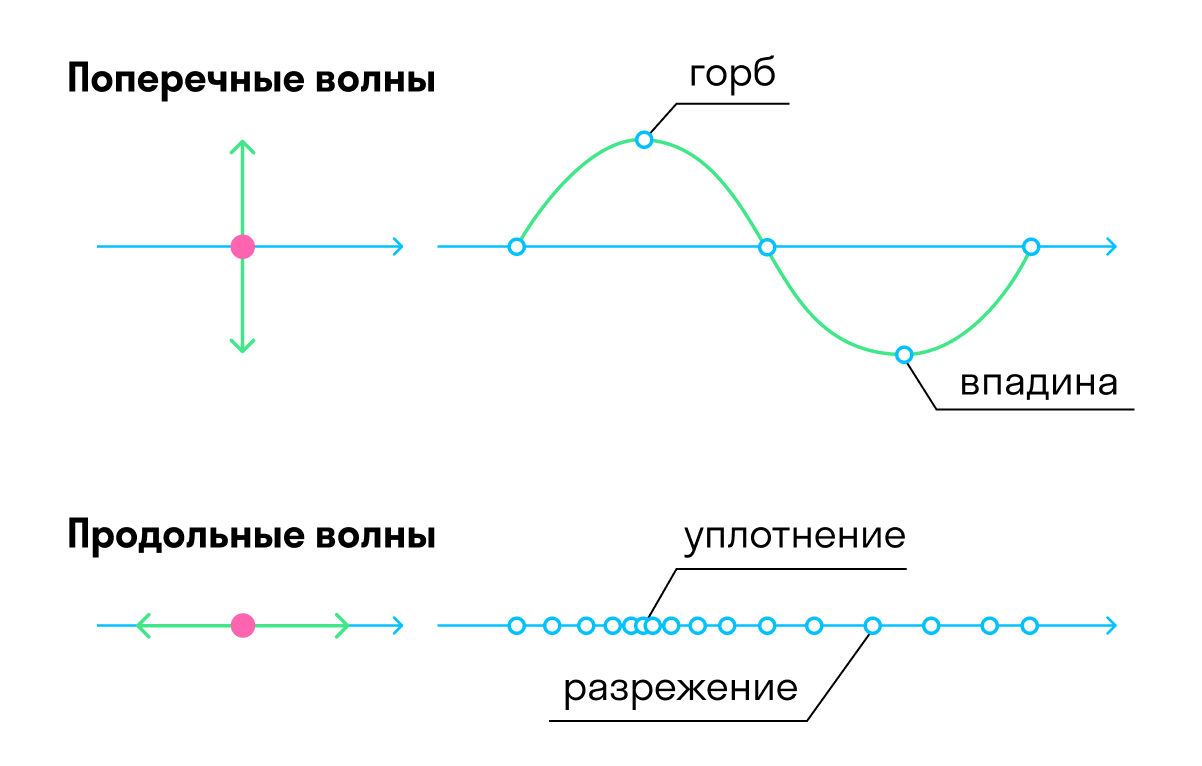
Продольные — это те волны, у которых колебание происходит вдоль направления распространения волны.
Поперечные — волны, у которых колебание происходит поперек направления распространения волны.
Длина волны: определение и расчет
Конечно, у любой волны есть характеристики. Одна из таких характеристик — это длина волны.
Длиной волны называется расстояние между двумя точками этой волны, колеблющихся в одной фазе. Если проще, то это расстояние между двумя «гребнями».
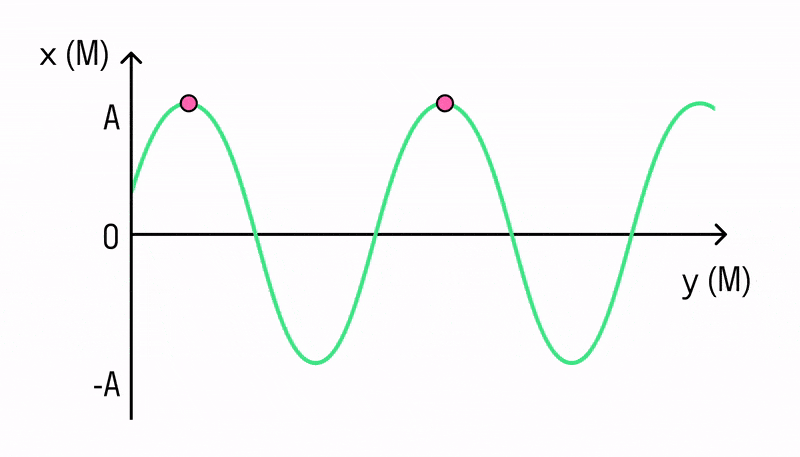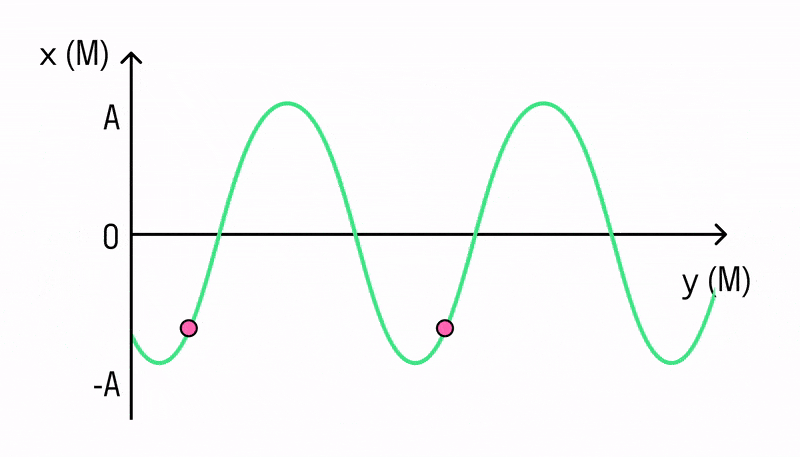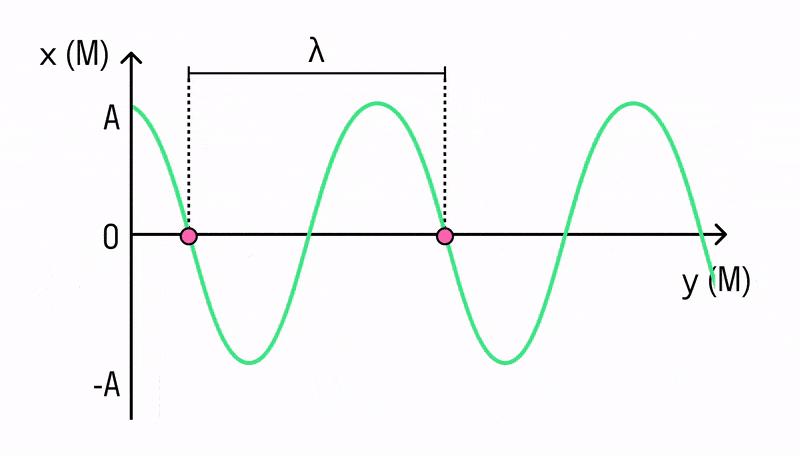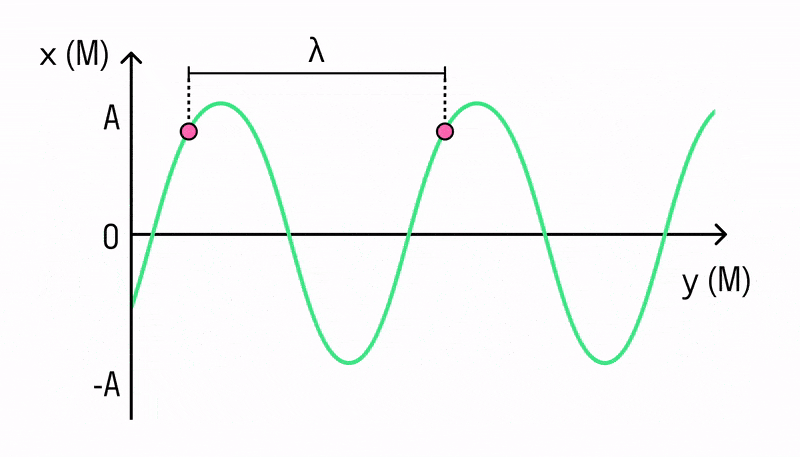
Еще длиной волны можно назвать расстояние, пройденное волной, за один период колебания.
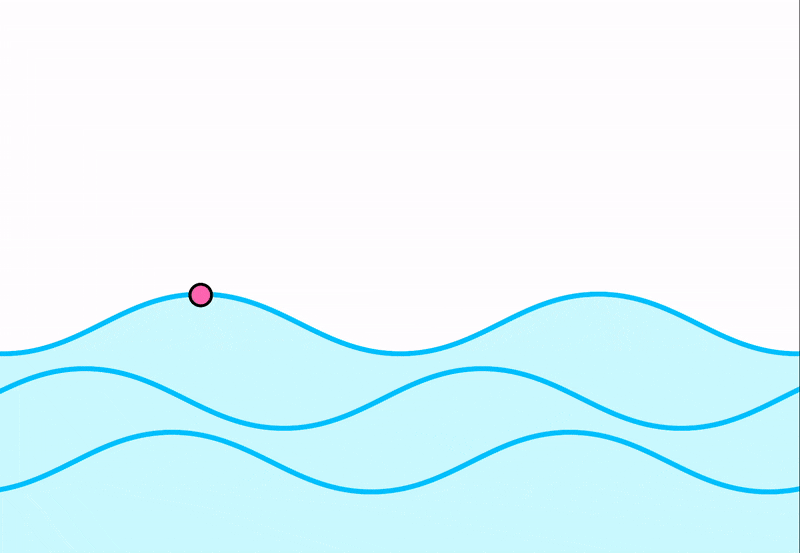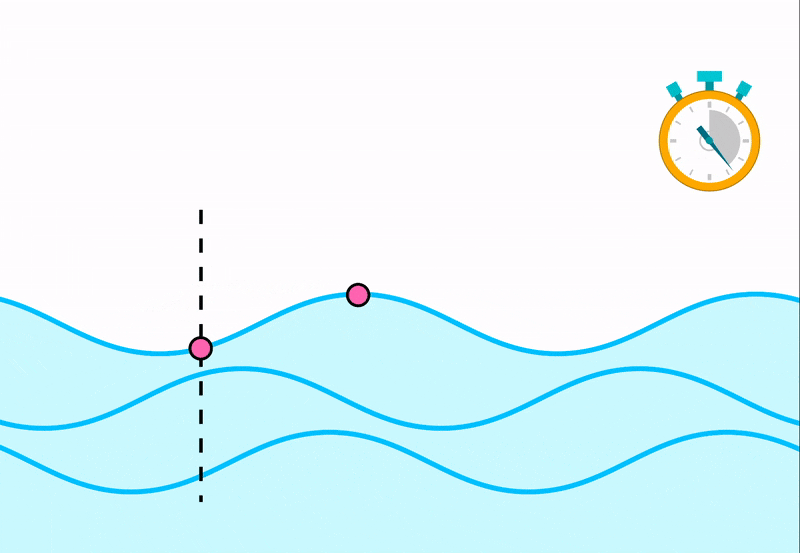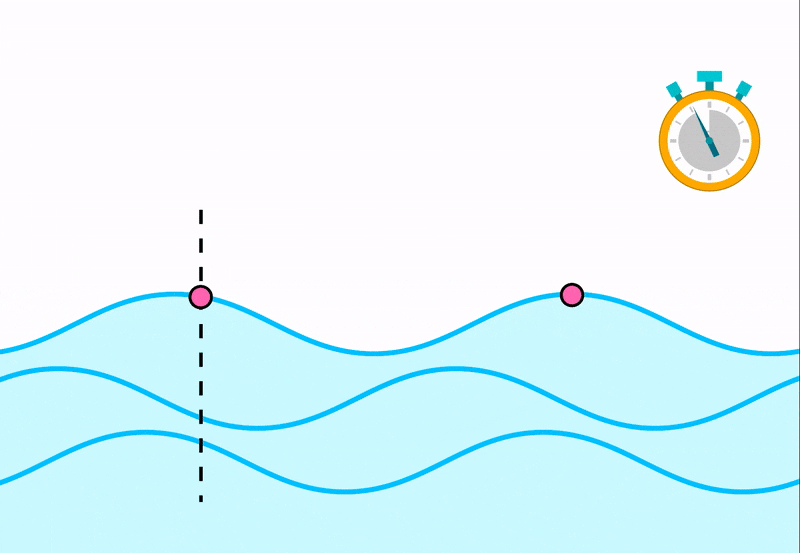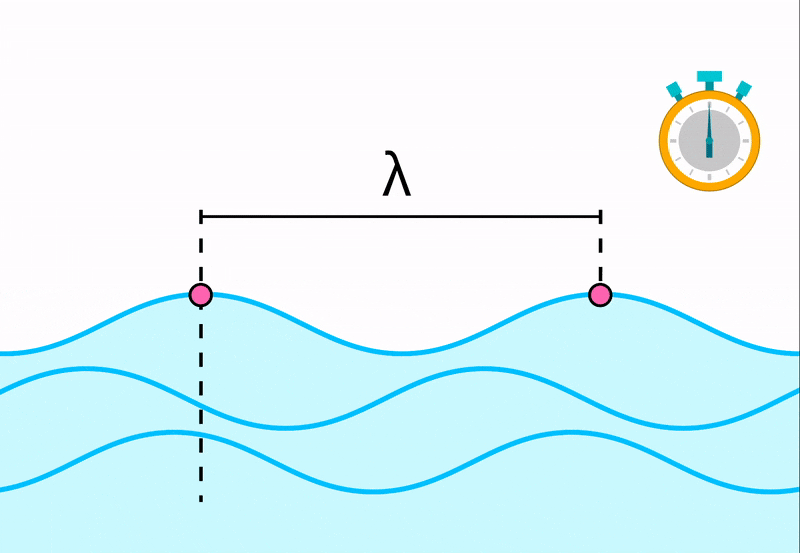
Период — это время, за которое происходит одно колебание. То есть, если дано время распространения волны и количество колебаний, можно рассчитать период.
Формула периода колебания волны
T = t/N
N — количество колебаний [-]
Курсы подготовки к ОГЭ по физике помогут снять стресс перед экзаменом и получить высокий балл.
Связь со скоростью
Чтобы вывести формулу скорости через длину волны, нужно вспомнить формулу скорости из кинематики — это раздел физики, в котором изучается движение тел без учета внешнего воздействия).
Формула скорости
? = S/t
Переходя к волнам, можно провести следующие аналогии:
А для скорости даже аналогия не нужна — скорость и Африке скорость.
Формула скорости волны
? = λ/T
Задачка
Лодка совершает колебания на волнах. За 40 с она совершила 10 колебаний. Какова скорость распространения волны, если расстояние между соседними гребнями волны равно 1 м?
Решение:
Резонанс
Если громко говорить в одном помещении с гитарой — можно услышать, как на ней начал играть призрак. На самом деле частота струны совпала с частотой голоса и возник резонанс.
На графике ниже можно увидеть, что на некоторой частоте резко увеличивается амплитуда. Эта частота называется частотой резонанса.

Частота — это величина, обратная периоду. Она показывает, за какое время происходит одно колебание.
Формула частоты
ν = N/t
N — количество колебаний [-]
В мире существует очень много историй про то, как солдаты шли в ногу по мосту, он впал в резонанс и все провалились. А вот еще одна история про гидрологов — как говорится, из первых уст?
Команда гидрологов — специалистов по внутренним водам — работала на Алтае и изучала местную реку. Через реку был протянут веревочный мост, а по центру моста стояла лебедка, которая помогает поднять пробу воды из речки, не спускаясь до нее.
В один из дней экспедиции начался сильный, почти штормовой, ветер. Исследователи работали на мосту, а когда поняли, что находиться на веревочной конструкции в такой сильный ветер небезопасно, начали с него уходить. Как только последний человек из команды сделал шаг с моста на землю, мост вместе с лебедкой разнесло в щепки. Это произошло из-за того, что частота ветра совпала с собственной частотой раскачивающегося моста. Хорошо, что история закончилась именно так.
Источник
Что нужно знать о длине волны в физике — суть явления, способы нахождения
Длина волны в физике: определение
Волной принято называть распространение колебательного движения в пространстве.
Любая волна обладает рядом характеристик: период, амплитуда, частота, скорость и длина волны.
График с основными параметрами:
Длина механической волны — это расстояние между двумя ближайшими друг к другу точками в пространстве, которые колеблются в одной фазе.
Длина электромагнитной волны — это расстояние, на котором фаза электромагнитной волны вдоль направления распространения меняется на 2π.
Обозначение: λ. Единица измерения — метр (м).
Как определить длину волны
Формулы нахождения длины механической волны:
2. λ=V/v, где v — частота (Гц).
Формулы нахождения длины электромагнитной волны:
Любую формулу можно изменить в зависимости от конкретной задачи.
На что влияет длина волны
В зависимости от того, какую длину имеют волны, излучаемые источником, данный источник будет применяться в определенных областях:
Примеры решения задач
В качестве помощи для понимания темы разберем примеры:
Рассчитать длину электромагнитной волны, если v=1 МГц, ε=2, μ=1
Сначала узнаем n=√εμ=√2*1≈1,4, тогда длина волны равна
Определить длину механической волны, если V=0,25 м/с, t=40 с, N=10.
Источник
Теперь вы знаете какие однокоренные слова подходят к слову Как пишется длина волны в физике, а так же какой у него корень, приставка, суффикс и окончание. Вы можете дополнить список однокоренных слов к слову «Как пишется длина волны в физике», предложив свой вариант в комментариях ниже, а также выразить свое несогласие проведенным с морфемным разбором.
Содержание:
- Определение и формула длины волны
- Длина стоячей волны
- Длина бегущей волны
- Длина бегущей волны
- Длина электромагнитной волны
- Единицы измерения длины волны
- Примеры решения задач
Определение и формула длины волны
Определение
Длиной волны называют кратчайшее пространственное расстояние между ее точками, совершающими колебания в одной фазе.
Обозначают длину волны, чаще всего буквой $lambda$ .
Для синусоидальных волн $lambda$ – это расстояние, на которое волна распространяется за один период
(T). Длину волны в этом случае еще называют пространственным периодом. Тогда формулой длины волны можно считать выражение:
$$lambda=v T=frac{v}{nu}=frac{2 pi}{k}$$
где v – скорость распространения волны, $nu=frac{1}{T}$ – частота колебаний,
$k=frac{omega}{v}$ – волновое число,
$T=frac{2 pi}{omega}$ – период волны,
$omega$ – циклическая частота волны.
Длина стоячей волны
Длиной стоячей волны($lambda_{st}$) называют расстояние в пространстве между
двумя пучностями (или узлами):
$$lambda_{s t}=frac{pi}{k}=frac{lambda}{2}(2)$$
где $lambda$ – длина бегущей волны. Надо заметить, что расстояние между соседними пучностью и
узлом связывает равенство:
$$frac{lambda_{s t}}{2}=frac{lambda}{4}(3)$$
Длина бегущей волны
В бегущей волне длина волны связана с фазовой скоростью (vph) формулой:
$$lambda=frac{v_{p h}}{nu}(4)$$
Длина бегущей волны
Разность фаз и длина волны
Две точки волны находящиеся на расстоянии
$Delta x$ имеют при колебании разность
фаз ($Delta varphi$), которая равна:
$$Delta varphi=frac{2 pi Delta x}{lambda}(5)$$
Длина электромагнитной волны
Скорость распространения электромагнитных волн в вакууме равна скорости света в вакууме
($c approx 3 cdot 10^{8}$ м/с), следовательно, длина электромагнитной волны в
вакууме, может быть рассчитана при помощи формулы:
$$lambda=c T=frac{c}{nu}(6)$$
Длина электромагнитной волны в веществе равна:
$$lambda=frac{c}{n nu}(7)$$
где $n=sqrt{varepsilon mu}$ – показатель преломления вещества,
$varepsilon$ – диэлектрическая проницаемость вещества,
$mu$ – магнитная проницаемость вещества.
Отметим, что все рассматриваемые формулы относят к случаю T=const.
Единицы измерения длины волны
Основной единицей измерения длины волны в системе СИ является: [$lambda$]=м
В СГС: [$lambda$]=см
Примеры решения задач
Пример
Задание. Каково приращение длины электромагнитной волны, имеющей частоту v=1 МГц при ее переходе в немагнитную среду,
которая имеет диэлектрическую проницаемость $varepsilon$=2?
Решение. Так как речь в условии задачи идет о немагнитной среде, в которую переходит волна, то считаем магнитную
проницаемость вещества равной единице ($mu$=1).
Длина рассматриваемой нами волны в вакууме равна:
$$lambda_{1}=frac{c}{nu}(1.1)$$
Длина волны в веществе:
$$lambda_{2}=frac{c}{n nu}=frac{c}{sqrt{varepsilon mu} cdot nu}(1.2)$$
Используя выражения (1.1) и (1.2) найдем изменение длины волны:
$$Delta lambda=lambda_{2}-lambda_{1}=frac{c}{sqrt{varepsilon mu} cdot nu}-frac{c}{nu}=frac{c}{nu}left(frac{1}{sqrt{varepsilon mu}}-1right)$$
Проведем вычисления, если нам известно помимо данных приведенных в условии задачи, что
$c approx 3 cdot 10^{8}$ м/с- скорость света в вакууме, и v=1 МГц=106 Гц:
$$Delta lambda=frac{3 cdot 10^{8}}{10^{6}}left(frac{1}{sqrt{4 cdot 1}}-1right)=-1,5 cdot 10^{2}(mathrm{~m})$$
Ответ. Длина волны уменьшится на 150 м

236
проверенных автора готовы помочь в написании работы любой сложности
Мы помогли уже 4 396 ученикам и студентам сдать работы от решения задач до дипломных на отлично! Узнай стоимость своей работы за 15 минут!
Пример
Задание. Какова длина плоской синусоидальной волны, которая распространяется по оси X. Две точки, которые
находятся на оси X расположенные на расстояниях 2 м и 3 м от источника совершают колебания с разностью фаз равной
$Delta varphi=frac{3 pi}{5}$ . Каким будет период колебаний в волне, если ее скорость в данной среде равна v=2м/с?
Решение. Сделаем рисунок.
Основой для решения задачи будет формула:
$$Delta varphi=frac{2 pi Delta x}{lambda}=frac{2 pileft(x_{2}-x_{1}right)}{lambda}(2.1)$$
Выразим из (2.1) искомую длину волны, получим:
$$lambda=frac{2 pileft(x_{2}-x_{1}right)}{Delta varphi}(2.2)$$
Период колебаний связан с длиной волны формулой:
$$T=frac{lambda}{v}(2.3)$$
C учетом (2.2), имеем:
$$T=frac{2 pileft(x_{2}-x_{1}right)}{Delta varphi v}$$
Проведем вычисления:
$$
begin{array}{c}
lambda=frac{2 pi(3-2)}{3 pi} cdot 5=frac{10}{3}(m) \
T=frac{10}{3 cdot 2}=1,67(c)
end{array}
$$
Ответ. $lambda approx 3,3 mathrm{~m} ; T approx 1,67 mathrm{c}$
Читать дальше: Формула количества теплоты.