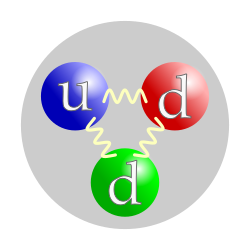
The quark content of the neutron. The color assignment of individual quarks is arbitrary, but all three colors must be present. Forces between quarks are mediated by gluons. |
|
| Classification | Baryon |
|---|---|
| Composition | 1 up quark, 2 down quarks |
| Statistics | Fermionic |
| Family | Hadron |
| Interactions | Gravity, weak, strong, electromagnetic |
| Symbol | n , n0 , N0 |
| Antiparticle | Antineutron |
| Theorized | Ernest Rutherford[1] (1920) |
| Discovered | James Chadwick[2] (1932) |
| Mass | 1.67492749804(95)×10−27 kg[3] 939.56542052(54) MeV/c2[3] 1.00866491588(49) Da[4] |
| Mean lifetime | 879.4(6) s (free)[5] |
| Electric charge | 0 e (−2±  ×10−22 e (experimental limits)[6] ×10−22 e (experimental limits)[6] |
| Electric dipole moment | < 2.9×10−26 e⋅cm (experimental upper limit) |
| Electric polarizability | 1.16(15)×10−3 fm3 |
| Magnetic moment | −0.96623650(23)×10−26 J·T−1[4] −1.04187563(25)×10−3 μB[4] −1.91304273(45) μN[4] |
| Magnetic polarizability | 3.7(20)×10−4 fm3 |
| Spin | 1/2 |
| Isospin | −1/2 |
| Parity | +1 |
| Condensed | I(JP) = 1/2(1/2+) |
The neutron is a subatomic particle, symbol
n
or
n0
, which has a neutral (not positive or negative) charge, and a mass slightly greater than that of a proton. Protons and neutrons constitute the nuclei of atoms. Since protons and neutrons behave similarly within the nucleus, and each has a mass of approximately one atomic mass unit, they are both referred to as nucleons.[7] Their properties and interactions are described by nuclear physics. Protons and neutrons are not elementary particles; each is composed of three quarks.
The chemical properties of an atom are mostly determined by the configuration of electrons that orbit the atom’s heavy nucleus. The electron configuration is determined by the charge of the nucleus, which is determined by the number of protons, or atomic number. The number of neutrons is the neutron number. Neutrons do not affect the electron configuration, but the sum of atomic and neutron numbers is the mass of the nucleus.
Atoms of a chemical element that differ only in neutron number are called isotopes. For example, carbon, with atomic number 6, has an abundant isotope carbon-12 with 6 neutrons and a rare isotope carbon-13 with 7 neutrons. Some elements occur in nature with only one stable isotope, such as fluorine; Other elements occur with many stable isotopes, such as tin with ten stable isotopes, and some elements such as technetium have no stable isotope.
The properties of an atomic nucleus depend on both atomic and neutron numbers. With their positive charge, the protons within the nucleus are repelled by the long-range electromagnetic force, but the much stronger, but short-range, nuclear force binds the nucleons closely together. Neutrons are required for the stability of nuclei, with the exception of the single-proton hydrogen nucleus. Neutrons are produced copiously in nuclear fission and fusion. They are a primary contributor to the nucleosynthesis of chemical elements within stars through fission, fusion, and neutron capture processes.
The neutron is essential to the production of nuclear power. In the decade after the neutron was discovered by James Chadwick in 1932,[8] neutrons were used to induce many different types of nuclear transmutations. With the discovery of nuclear fission in 1938,[9] it was quickly realized that, if a fission event produced neutrons, each of these neutrons might cause further fission events, in a cascade known as a nuclear chain reaction.[10] These events and findings led to the first self-sustaining nuclear reactor (Chicago Pile-1, 1942) and the first nuclear weapon (Trinity, 1945).
Dedicated neutron sources like neutron generators, research reactors and spallation sources produce free neutrons for use in irradiation and in neutron scattering experiments. A free neutron spontaneously decays to a proton, an electron, and an antineutrino, with a mean lifetime of about 15 minutes.[11] Free neutrons do not directly ionize atoms, but they do indirectly cause ionizing radiation, so they can be a biological hazard, depending on dose.[10] A small natural «neutron background» flux of free neutrons exists on Earth, caused by cosmic ray showers, and by the natural radioactivity of spontaneously fissionable elements in the Earth’s crust.[12]
Description[edit]
An atomic nucleus is formed by a number of protons, Z (the atomic number), and a number of neutrons, N (the neutron number), bound together by the nuclear force. The atomic number determines the chemical properties of the atom, and the neutron number determines the isotope or nuclide.[10] The terms isotope and nuclide are often used synonymously, but they refer to chemical and nuclear properties, respectively. Isotopes are nuclides with the same atomic number, but different neutron number. Nuclides with the same neutron number, but different atomic number, are called isotones. The atomic mass number, A, is equal to the sum of atomic and neutron numbers. Nuclides with the same atomic mass number, but different atomic and neutron numbers, are called isobars.
The nucleus of the most common isotope of the hydrogen atom (with the chemical symbol 1H) is a lone proton. The nuclei of the heavy hydrogen isotopes deuterium (D or 2H) and tritium (T or 3H) contain one proton bound to one and two neutrons, respectively. All other types of atomic nuclei are composed of two or more protons and various numbers of neutrons. The most common nuclide of the common chemical element lead, 208Pb, has 82 protons and 126 neutrons, for example. The table of nuclides comprises all the known nuclides. Even though it is not a chemical element, the neutron is included in this table.[13]
The free neutron has a mass of 939565413.3 eV/c2, or 1.674927471×10−27 kg, or 1.00866491588 Da.[4] The neutron has a mean square radius of about 0.8×10−15 m, or 0.8 fm,[14] and it is a spin-½ fermion.[15] The neutron has no measurable electric charge. With its positive electric charge, the proton is directly influenced by electric fields, whereas the neutron is unaffected by electric fields. But the neutron has a magnetic moment, so the neutron is influenced by magnetic fields. The neutron’s magnetic moment has a negative value, because its orientation is opposite to the neutron’s spin.[16]
A free neutron is unstable, decaying to a proton, electron and antineutrino with a mean lifetime of just under 15 minutes (879.6±0.8 s).[5] This radioactive decay, known as beta decay, is possible because the mass of the neutron is slightly greater than that of the proton. The free proton is stable. However, neutrons or protons bound in a nucleus can be stable or unstable, depending on the nuclide. Beta decay, in which neutrons decay to protons, or vice versa, is governed by the weak force, and it requires the emission or absorption of electrons and neutrinos, or their antiparticles.
Nuclear fission caused by absorption of a neutron by uranium-235. The heavy nuclide fragments into lighter components and additional neutrons.
Protons and neutrons behave almost identically under the influence of the nuclear force within the nucleus. The concept of isospin, in which the proton and neutron are viewed as two quantum states of the same particle, is used to model the interactions of nucleons by the nuclear or weak forces. Because of the strength of the nuclear force at short distances, the binding energy of nucleons is more than seven orders of magnitude larger than the electromagnetic energy binding electrons in atoms. Nuclear reactions (such as nuclear fission) therefore have an energy density that is more than ten million times that of chemical reactions. Because of the mass–energy equivalence, nuclear binding energies reduce the mass of nuclei. Ultimately, the ability of the nuclear force to store energy arising from the electromagnetic repulsion of nuclear components is the basis for most of the energy that makes nuclear reactors or bombs possible. In nuclear fission, the absorption of a neutron by a heavy nuclide (e.g., uranium-235) causes the nuclide to become unstable and break into light nuclides and additional neutrons. The positively charged light nuclides then repel, releasing electromagnetic potential energy.
The neutron is classified as a hadron, because it is a composite particle made of quarks. The neutron is also classified as a baryon, because it is composed of three valence quarks.[17] The finite size of the neutron and its magnetic moment both indicate that the neutron is a composite, rather than elementary, particle. A neutron contains two down quarks with charge −1/3e and one up quark with charge +2/3e.
Like protons, the quarks of the neutron are held together by the strong force, mediated by gluons.[18] The nuclear force results from secondary effects of the more fundamental strong force.
Discovery[edit]
The story of the discovery of the neutron and its properties is central to the extraordinary developments in atomic physics that occurred in the first half of the 20th century, leading ultimately to the atomic bomb in 1945. In the 1911 Rutherford model, the atom consisted of a small positively charged massive nucleus surrounded by a much larger cloud of negatively charged electrons. In 1920, Ernest Rutherford suggested that the nucleus consisted of positive protons and neutrally charged particles, suggested to be a proton and an electron bound in some way.[19] Electrons were assumed to reside within the nucleus because it was known that beta radiation consisted of electrons emitted from the nucleus.[19]
About the time Rutherford suggested the neutral proton-electron composite, several other publications appeared making similar suggestions, and in 1921 the American chemist W.D. Harkins first named the hypothetical particle a «neutron».[20][21] The name derives from the Latin root for neutralis (neuter) and the Greek suffix -on (a suffix used in the names of subatomic particles, i.e. electron and proton).[22][23] References to the word neutron in connection with the atom can be found in the literature as early as 1899, however.[21]
Throughout the 1920s, physicists assumed that the atomic nucleus was composed of protons and «nuclear electrons»[24][25] but there were obvious problems. It was difficult to reconcile the proton–electron model for nuclei with the Heisenberg uncertainty relation of quantum mechanics.[26][27] The Klein paradox,[28] discovered by Oskar Klein in 1928, presented further quantum mechanical objections to the notion of an electron confined within a nucleus.[26] Observed properties of atoms and molecules were inconsistent with the nuclear spin expected from the proton–electron hypothesis. Both protons and electrons carry an intrinsic spin of 1/2ħ. Isotopes of the same species (i.e. having the same number of protons) can have both integer or fractional spin, i.e. the neutron spin must be also fractional (1/2ħ). But there is no way to arrange the spins of an electron and a proton (supposed to bond to form a neutron) to get the fractional spin of a neutron.
In 1931, Walther Bothe and Herbert Becker found that if alpha particle radiation from polonium fell on beryllium, boron, or lithium, an unusually penetrating radiation was produced. The radiation was not influenced by an electric field, so Bothe and Becker assumed it was gamma radiation.[29][30] The following year Irène Joliot-Curie and Frédéric Joliot-Curie in Paris showed that if this «gamma» radiation fell on paraffin, or any other hydrogen-containing compound, it ejected protons of very high energy.[31] Neither Rutherford nor James Chadwick at the Cavendish Laboratory in Cambridge were convinced by the gamma ray interpretation.[32] Chadwick quickly performed a series of experiments that showed that the new radiation consisted of uncharged particles with about the same mass as the proton.[8][33][34] These particles were neutrons. Chadwick won the 1935 Nobel Prize in Physics for this discovery.[2]
Models depicting the nucleus and electron energy levels in hydrogen, helium, lithium, and neon atoms. In reality, the diameter of the nucleus is about 100,000 times smaller than the diameter of the atom.
Models for an atomic nucleus consisting of protons and neutrons were quickly developed by Werner Heisenberg[35][36][37] and others.[38][39] The proton–neutron model explained the puzzle of nuclear spins. The origins of beta radiation were explained by Enrico Fermi in 1934 by the process of beta decay, in which the neutron decays to a proton by creating an electron and a (at the time undiscovered) neutrino.[40] In 1935, Chadwick and his doctoral student Maurice Goldhaber reported the first accurate measurement of the mass of the neutron.[41][42]
By 1934, Fermi had bombarded heavier elements with neutrons to induce radioactivity in elements of high atomic number. In 1938, Fermi received the Nobel Prize in Physics «for his demonstrations of the existence of new radioactive elements produced by neutron irradiation, and for his related discovery of nuclear reactions brought about by slow neutrons».[43] In 1938 Otto Hahn, Lise Meitner, and Fritz Strassmann discovered nuclear fission, or the fractionation of uranium nuclei into light elements, induced by neutron bombardment.[44][45][46] In 1945 Hahn received the 1944 Nobel Prize in Chemistry «for his discovery of the fission of heavy atomic nuclei».[47][48][49] The discovery of nuclear fission would lead to the development of nuclear power and the atomic bomb by the end of World War II.
Beta decay and the stability of the nucleus[edit]
Neutrons are a necessary constituent of any atomic nucleus that contains more than one proton. Interacting protons have a mutual electromagnetic repulsion that is stronger than their attractive nuclear interaction, so proton-only nuclei are unstable (see diproton and neutron–proton ratio).[50] Neutrons bind with protons and one another in the nucleus via the nuclear force, effectively moderating the repulsive forces between the protons and stabilizing the nucleus.
Within the nucleus, neutrons can decay to protons, or vice versa. This process is called beta decay, and, for the neutron, it requires the emission of an electron and an anti-neutrino:
n0
→
p+
+
e−
+
ν
e
where
p+
,
e−
, and
ν
e denote the proton, electron and electron antineutrino products, respectively.[51] The decay of the proton to a neutron is similar:
p+
→
n0
+
e+
+
ν
e
where
n0
,
e+
, and
ν
e denote the neutron, positron and electron neutrino products, respectively. In these reactions, the original particle is not composed of the product particles; rather, the product particles are created at the instant of the reaction.
The neutrons and protons bound in a nucleus form a quantum mechanical system wherein each nucleon is bound in a particular, hierarchical quantum state. The emitted particles, that is, the decay products, carry away the energy excess as a nucleon falls from one quantum state to a lower energy state, while the proton (or neutron) changes to a neutron (or proton). Such decay processes can occur only if allowed by basic energy conservation and quantum mechanical constraints. The stability of nuclei is a consequence of these constraints.
«Free» neutrons or protons are nucleons that exist independently, free of any nucleus. Since the neutron is slightly more massive than a proton, the decay of a free neutron to a proton is allowed, while the decay of a free proton is energetically disallowed. A high-energy collision of a proton and an electron or neutrino can result in a neutron, however.
Free neutron decay[edit]
Outside the nucleus, free neutrons are unstable and have a mean lifetime of 879.6±0.8 s (about 14 minutes, 40 seconds); therefore the half-life for this process (which differs from the mean lifetime by a factor of ln(2) = 0.693) is 610.1±0.7 s (about 10 minutes, 10 seconds).[11][52] This decay is only possible because the mass of the proton is less than that of the neutron. By the mass-energy equivalence, when a neutron decays to a proton this way, a lower energy state is attained.
For the free neutron the decay energy for this process (based on the masses of the neutron, proton, and electron) is 0.782343 MeV. The maximal energy of the beta decay electron (in the process wherein the neutrino receives a vanishingly small amount of kinetic energy) has been measured at 0.782±0.013 MeV.[53] The latter number is not well-enough measured to determine the comparatively tiny rest mass of the neutrino (which must in theory be subtracted from the maximal electron kinetic energy) as well as neutrino mass is constrained by many other methods.
A small fraction (about one in 1000) of free neutrons decay with the same products, but add an extra particle in the form of an emitted gamma ray:
n0
→
p+
+
e−
+
ν
e +
γ
This gamma ray may be thought of as an «internal bremsstrahlung» that arises from the electromagnetic interaction of the emitted beta particle with the proton. Internal bremsstrahlung gamma ray production is also a minor feature of beta decays of bound neutrons (as discussed below).
A schematic of the nucleus of an atom indicating
β−
radiation, the emission of a fast electron from the nucleus (the accompanying antineutrino is omitted). In the Rutherford model for the nucleus, red spheres were protons with positive charge and blue spheres were protons tightly bound to an electron with no net charge.
The inset shows beta decay of a free neutron as it is understood today; an electron and antineutrino are created in this process.
A very small minority of neutron decays (about four per million) are so-called «two-body (neutron) decays», in which a proton, electron and antineutrino are produced as usual, but the electron fails to gain the 13.6 eV necessary energy to escape the proton (the ionization energy of hydrogen), and therefore simply remains bound to it, as a neutral hydrogen atom (one of the «two bodies»). In this type of free neutron decay, almost all of the neutron decay energy is carried off by the antineutrino (the other «body»). (The hydrogen atom recoils with a speed of only about (decay energy)/(hydrogen rest energy) times the speed of light, or 250 km/s.)
Bound neutron decay[edit]
While a free neutron has a half life of about 10.2 min, most neutrons within nuclei are stable. According to the nuclear shell model, the protons and neutrons of a nuclide are a quantum mechanical system organized into discrete energy levels with unique quantum numbers. For a neutron to decay, the resulting proton requires an available state at lower energy than the initial neutron state. In stable nuclei the possible lower energy states are all filled, meaning each state is occupied by a pair of protons, one with spin up, another with spin down. When all available proton states are filled, the Pauli exclusion principle disallows the decay of a neutron to a proton within stable nuclei. The situation is similar to electrons of an atom, where electrons that occupy distinct atomic orbitals are prevented by the exclusion principle from decaying to lower, already-filled, energy states, with the emission of a photon.
Neutrons in unstable nuclei can decay by beta decay as described above. In this case, an energetically allowed quantum state is available for the proton resulting from the decay. One example of this decay is carbon-14 (6 protons, 8 neutrons) that decays to nitrogen-14 (7 protons, 7 neutrons) with a half-life of about 5,730 years.
Similarly, a proton inside a nucleus can decay into a neutron, if an energetically allowed quantum state is available for the neutron.
The transformation of a proton to a neutron inside of a nucleus is also possible through electron capture:
p+
+
e−
→
n0
+
ν
e
Positron capture by neutrons in nuclei that contain an excess of neutrons is also possible, but is hindered because positrons are repelled by the positive nucleus, and quickly annihilate when they encounter electrons. Similar, but far more rare, reactions involve the capture of a neutrino by a nucleon in inverse beta decay.
Competition of beta decay types[edit]
Three types of beta decay in competition are illustrated by the single isotope copper-64 (29 protons, 35 neutrons), which has a half-life of about 12.7 hours. This isotope has one unpaired proton and one unpaired neutron, so either the proton or the neutron can decay. This particular nuclide is almost equally likely to undergo proton decay (by positron emission, 18% or by electron capture, 43%) or neutron decay (by electron emission, 39%).
Decay of the neutron by elementary particle physics[edit]
Within the theoretical framework of Standard Model for particle physics, the neutron is composed of two down quarks and an up quark. The only possible decay mode for the neutron that conserves baryon number is for one of the neutron’s quarks to change flavour via the weak interaction. The decay of one of the neutron’s down quarks into a lighter up quark can be achieved by the emission of a W boson. By this process, the Standard Model description of beta decay, the neutron decays into a proton (which contains one down and two up quarks), an electron, and an electron antineutrino.
The decay of the proton to a neutron occurs similarly through the electroweak force. The decay of one of the proton’s up quarks into a down quark can be achieved by the emission of a W boson. The proton decays into a neutron, a positron, and an electron neutrino. This reaction can only occur within an atomic nucleus which has a quantum state at lower energy available for the created neutron.
Intrinsic properties[edit]
Mass[edit]
The mass of a neutron cannot be directly determined by mass spectrometry since it has no electric charge. But since the masses of a proton and of a deuteron can be measured with a mass spectrometer, the mass of a neutron can be deduced by subtracting proton mass from deuteron mass, with the difference being the mass of the neutron plus the binding energy of deuterium (expressed as a positive emitted energy). The latter can be directly measured by measuring the energy (

The energy of the gamma ray can be measured to high precision by X-ray diffraction techniques, as was first done by Bell and Elliot in 1948. The best modern (1986) values for neutron mass by this technique are provided by Greene, et al.[54] These give a neutron mass of:
- mneutron = 1.008644904(14) Da
The value for the neutron mass in MeV is less accurately known, due to less accuracy in the known conversion of Da to MeV/c2:[55]
- mneutron = 939.56563(28) MeV/c2.
Another method to determine the mass of a neutron starts from the beta decay of the neutron, when the momenta of the resulting proton and electron are measured.
Electric charge[edit]
The total electric charge of the neutron is 0 e. This zero value has been tested experimentally, and the present experimental limit for the charge of the neutron is −2(8)×10−22 e,[6] or −3(13)×10−41 C. This value is consistent with zero, given the experimental uncertainties (indicated in parentheses). By comparison, the charge of the proton is +1 e.
Magnetic moment[edit]
Even though the neutron is a neutral particle, the magnetic moment of a neutron is not zero. The neutron is not affected by electric fields, but it is affected by magnetic fields. The value for the neutron’s magnetic moment was first directly measured by Luis Alvarez and Felix Bloch at Berkeley, California, in 1940.[56] Alvarez and Bloch determined the magnetic moment of the neutron to be μn= −1.93(2) μN, where μN is the nuclear magneton.
The magnetic moment of the neutron is an indication of its quark substructure and internal charge distribution.[57] In the quark model for hadrons, the neutron is composed of one up quark (charge +2/3 e) and two down quarks (charge −1/3 e).[57] The magnetic moment of the neutron can be modeled as a sum of the magnetic moments of the constituent quarks.[58] The calculation assumes that the quarks behave like pointlike Dirac particles, each having their own magnetic moment. Simplistically, the magnetic moment of the neutron can be viewed as resulting from the vector sum of the three quark magnetic moments, plus the orbital magnetic moments caused by the movement of the three charged quarks within the neutron.
In one of the early successes of the Standard Model in 1964 Mirza A.B. Beg, Benjamin W. Lee, and Abraham Pais theoretically calculated the ratio of proton to neutron magnetic moments to be −3/2, which agrees with the experimental value to within 3%.[59][60][61] The measured value for this ratio is −1.45989805(34).[4]
The above treatment compares neutrons with protons, allowing the complex behavior of quarks to be subtracted out between models, and merely exploring what the effects would be of differing quark charges (or quark type). Such calculations are enough to show that the interior of neutrons is very much like that of protons, save for the difference in quark composition with a down quark in the neutron replacing an up quark in the proton.
The neutron magnetic moment can be roughly computed by assuming a simple nonrelativistic, quantum mechanical wavefunction for baryons composed of three quarks. A straightforward calculation gives fairly accurate estimates for the magnetic moments of neutrons, protons, and other baryons.[58] For a neutron, the result of this calculation is that the magnetic moment of the neutron is given by μn= 4/3 μd − 1/3 μu, where μd and μu are the magnetic moments for the down and up quarks, respectively. This result combines the intrinsic magnetic moments of the quarks with their orbital magnetic moments, and assumes the three quarks are in a particular, dominant quantum state.
| Baryon | Magnetic moment of quark model |
Computed (  ) )
|
Observed (  ) )
|
|---|---|---|---|
| p | 4/3 μu − 1/3 μd | 2.79 | 2.793 |
| n | 4/3 μd − 1/3 μu | −1.86 | −1.913 |
The results of this calculation are encouraging, but the masses of the up or down quarks were assumed to be 1/3 the mass of a nucleon.[58] The masses of the quarks are actually only about 1% that of a nucleon.[62] The discrepancy stems from the complexity of the Standard Model for nucleons, where most of their mass originates in the gluon fields, virtual particles, and their associated energy that are essential aspects of the strong force.[62][63] Furthermore, the complex system of quarks and gluons that constitute a neutron requires a relativistic treatment.[64] But the nucleon magnetic moment has been successfully computed numerically from first principles, including all of the effects mentioned and using more realistic values for the quark masses. The calculation gave results that were in fair agreement with measurement, but it required significant computing resources.[65][66]
Spin[edit]
The neutron is a spin 1/2 particle, that is, it is a fermion with intrinsic angular momentum equal to 1/2 ħ, where ħ is the reduced Planck constant. For many years after the discovery of the neutron, its exact spin was ambiguous. Although it was assumed to be a spin 1/2 Dirac particle, the possibility that the neutron was a spin 3/2 particle lingered. The interactions of the neutron’s magnetic moment with an external magnetic field were exploited to finally determine the spin of the neutron.[67] In 1949, Hughes and Burgy measured neutrons reflected from a ferromagnetic mirror and found that the angular distribution of the reflections was consistent with spin 1/2.[68] In 1954, Sherwood, Stephenson, and Bernstein employed neutrons in a Stern–Gerlach experiment that used a magnetic field to separate the neutron spin states. They recorded two such spin states, consistent with a spin 1/2 particle.[67][69]
As a fermion, the neutron is subject to the Pauli exclusion principle; two neutrons cannot have the same quantum numbers. This is the source of the degeneracy pressure which makes neutron stars possible.
Structure and geometry of charge distribution[edit]
An article published in 2007 featuring a model-independent analysis concluded that the neutron has a negatively charged exterior, a positively charged middle, and a negative core.[70] In a simplified classical view, the negative «skin» of the neutron assists it to be attracted to the protons with which it interacts in the nucleus; but the main attraction between neutrons and protons is via the nuclear force, which does not involve electric charge.
The simplified classical view of the neutron’s charge distribution also «explains» the fact that the neutron magnetic dipole points in the opposite direction from its spin angular momentum vector (as compared to the proton). This gives the neutron, in effect, a magnetic moment which resembles a negatively charged particle. This can be reconciled classically with a neutral neutron composed of a charge distribution in which the negative sub-parts of the neutron have a larger average radius of distribution, and therefore contribute more to the particle’s magnetic dipole moment, than do the positive parts that are, on average, nearer the core.
Electric dipole moment[edit]
The Standard Model of particle physics predicts a tiny separation of positive and negative charge within the neutron leading to a permanent electric dipole moment.[71] But the predicted value is well below the current sensitivity of experiments. From several unsolved puzzles in particle physics, it is clear that the Standard Model is not the final and full description of all particles and their interactions. New theories going beyond the Standard Model generally lead to much larger predictions for the electric dipole moment of the neutron. Currently, there are at least four experiments trying to measure for the first time a finite neutron electric dipole moment, including:
- Cryogenic neutron EDM experiment being set up at the Institut Laue–Langevin[72]
- nEDM experiment under construction at the new UCN source at the Paul Scherrer Institute[73]
- nEDM experiment being envisaged at the Spallation Neutron Source[74][75]
- nEDM experiment being built at the Institut Laue–Langevin[76]
Antineutron[edit]
The antineutron is the antiparticle of the neutron. It was discovered by Bruce Cork in 1956, a year after the antiproton was discovered. CPT-symmetry puts strong constraints on the relative properties of particles and antiparticles, so studying antineutrons provides stringent tests on CPT-symmetry. The fractional difference in the masses of the neutron and antineutron is (9±6)×10−5. Since the difference is only about two standard deviations away from zero, this does not give any convincing evidence of CPT-violation.[11]
Neutron compounds[edit]
Dineutrons and tetraneutrons[edit]
The existence of stable clusters of 4 neutrons, or tetraneutrons, has been hypothesised by a team led by Francisco-Miguel Marqués at the CNRS Laboratory for Nuclear Physics based on observations of the disintegration of beryllium-14 nuclei. This is particularly interesting because current theory suggests that these clusters should not be stable.
In February 2016, Japanese physicist Susumu Shimoura of the University of Tokyo and co-workers reported they had observed the purported tetraneutrons for the first time experimentally.[77] Nuclear physicists around the world say this discovery, if confirmed, would be a milestone in the field of nuclear physics and certainly would deepen our understanding of the nuclear forces.[78][79]
The dineutron is another hypothetical particle. In 2012, Artemis Spyrou from Michigan State University and coworkers reported that they observed, for the first time, the dineutron emission in the decay of 16Be. The dineutron character is evidenced by a small emission angle between the two neutrons. The authors measured the two-neutron separation energy to be 1.35(10) MeV, in good agreement with shell model calculations, using standard interactions for this mass region.[80]
Neutronium and neutron stars[edit]
At extremely high pressures and temperatures, nucleons and electrons are believed to collapse into bulk neutronic matter, called neutronium. This is presumed to happen in neutron stars.
The extreme pressure inside a neutron star may deform the neutrons into a cubic symmetry, allowing tighter packing of neutrons.[81]
Detection[edit]
The common means of detecting a charged particle by looking for a track of ionization (such as in a cloud chamber) does not work for neutrons directly. Neutrons that elastically scatter off atoms can create an ionization track that is detectable, but the experiments are not as simple to carry out; other means for detecting neutrons, consisting of allowing them to interact with atomic nuclei, are more commonly used. The commonly used methods to detect neutrons can therefore be categorized according to the nuclear processes relied upon, mainly neutron capture or elastic scattering.[82]
Neutron detection by neutron capture[edit]
A common method for detecting neutrons involves converting the energy released from neutron capture reactions into electrical signals. Certain nuclides have a high neutron capture cross section, which is the probability of absorbing a neutron. Upon neutron capture, the compound nucleus emits more easily detectable radiation, for example an alpha particle, which is then detected. The nuclides 3
He
, 6
Li
, 10
B
, 233
U
, 235
U
, 237
Np
, and 239
Pu
are useful for this purpose.
Neutron detection by elastic scattering[edit]
Neutrons can elastically scatter off nuclei, causing the struck nucleus to recoil. Kinematically, a neutron can transfer more energy to a light nucleus such as hydrogen or helium than to a heavier nucleus. Detectors relying on elastic scattering are called fast neutron detectors. Recoiling nuclei can ionize and excite further atoms through collisions. Charge and/or scintillation light produced in this way can be collected to produce a detected signal. A major challenge in fast neutron detection is discerning such signals from erroneous signals produced by gamma radiation in the same detector. Methods such as pulse shape discrimination can be used in distinguishing neutron signals from gamma-ray signals, although certain inorganic scintillator-based detectors have been developed [83][84] to selectively detect neutrons in mixed radiation fields inherently without any additional techniques.
Fast neutron detectors have the advantage of not requiring a moderator, and are therefore capable of measuring the neutron’s energy, time of arrival, and in certain cases direction of incidence.
Sources and production[edit]
Free neutrons are unstable, although they have the longest half-life of any unstable subatomic particle by several orders of magnitude. Their half-life is still only about 10 minutes, so they can be obtained only from sources that produce them continuously.
Natural neutron background. A small natural background flux of free neutrons exists everywhere on Earth. In the atmosphere and deep into the ocean, the «neutron background» is caused by muons produced by cosmic ray interaction with the atmosphere. These high-energy muons are capable of penetration to considerable depths in water and soil. There, in striking atomic nuclei, among other reactions they induce spallation reactions in which a neutron is liberated from the nucleus. Within the Earth’s crust a second source is neutrons produced primarily by spontaneous fission of uranium and thorium present in crustal minerals. The neutron background is not strong enough to be a biological hazard, but it is of importance to very high resolution particle detectors that are looking for very rare events, such as (hypothesized) interactions that might be caused by particles of dark matter.[12] Recent research has shown that even thunderstorms can produce neutrons with energies of up to several tens of MeV.[85] Recent research has shown that the fluence of these neutrons lies between 10−9 and 10−13 per ms and per m2 depending on the detection altitude. The energy of most of these neutrons, even with initial energies of 20 MeV, decreases down to the keV range within 1 ms.[86]
Even stronger neutron background radiation is produced at the surface of Mars, where the atmosphere is thick enough to generate neutrons from cosmic ray muon production and neutron-spallation, but not thick enough to provide significant protection from the neutrons produced. These neutrons not only produce a Martian surface neutron radiation hazard from direct downward-going neutron radiation but may also produce a significant hazard from reflection of neutrons from the Martian surface, which will produce reflected neutron radiation penetrating upward into a Martian craft or habitat from the floor.[87]
Sources of neutrons for research. These include certain types of radioactive decay (spontaneous fission and neutron emission), and from certain nuclear reactions. Convenient nuclear reactions include tabletop reactions such as natural alpha and gamma bombardment of certain nuclides, often beryllium or deuterium, and induced nuclear fission, such as occurs in nuclear reactors. In addition, high-energy nuclear reactions (such as occur in cosmic radiation showers or accelerator collisions) also produce neutrons from disintegration of target nuclei. Small (tabletop) particle accelerators optimized to produce free neutrons in this way, are called neutron generators.
In practice, the most commonly used small laboratory sources of neutrons use radioactive decay to power neutron production. One noted neutron-producing radioisotope, californium-252 decays (half-life 2.65 years) by spontaneous fission 3% of the time with production of 3.7 neutrons per fission, and is used alone as a neutron source from this process. Nuclear reaction sources (that involve two materials) powered by radioisotopes use an alpha decay source plus a beryllium target, or else a source of high-energy gamma radiation from a source that undergoes beta decay followed by gamma decay, which produces photoneutrons on interaction of the high-energy gamma ray with ordinary stable beryllium, or else with the deuterium in heavy water. A popular source of the latter type is radioactive antimony-124 plus beryllium, a system with a half-life of 60.9 days, which can be constructed from natural antimony (which is 42.8% stable antimony-123) by activating it with neutrons in a nuclear reactor, then transported to where the neutron source is needed.[88]
Nuclear fission reactors naturally produce free neutrons; their role is to sustain the energy-producing chain reaction. The intense neutron radiation can also be used to produce various radioisotopes through the process of neutron activation, which is a type of neutron capture.
Experimental nuclear fusion reactors produce free neutrons as a waste product. But it is these neutrons that possess most of the energy, and converting that energy to a useful form has proved a difficult engineering challenge. Fusion reactors that generate neutrons are likely to create radioactive waste, but the waste is composed of neutron-activated lighter isotopes, which have relatively short (50–100 years) decay periods as compared to typical half-lives of 10,000 years[89] for fission waste, which is long due primarily to the long half-life of alpha-emitting transuranic actinides.[90] Some nuclear fusion-fission hybrids are proposed to make use of those neutrons to either maintain a subcritical reactor or to aid in nuclear transmutation of harmful long lived nuclear waste to shorter lived or stable nuclides.
Neutron beams and modification of beams after production[edit]
Free neutron beams are obtained from neutron sources by neutron transport. For access to intense neutron sources, researchers must go to a specialized neutron facility that operates a research reactor or a spallation source.
The neutron’s lack of total electric charge makes it difficult to steer or accelerate them. Charged particles can be accelerated, decelerated, or deflected by electric or magnetic fields. These methods have little effect on neutrons. But some effects may be attained by use of inhomogeneous magnetic fields because of the neutron’s magnetic moment. Neutrons can be controlled by methods that include moderation, reflection, and velocity selection. Thermal neutrons can be polarized by transmission through magnetic materials in a method analogous to the Faraday effect for photons. Cold neutrons of wavelengths of 6–7 angstroms can be produced in beams of a high degree of polarization, by use of magnetic mirrors and magnetized interference filters.[91]
Applications[edit]
The neutron plays an important role in many nuclear reactions. For example, neutron capture often results in neutron activation, inducing radioactivity. In particular, knowledge of neutrons and their behavior has been important in the development of nuclear reactors and nuclear weapons. The fissioning of elements like uranium-235 and plutonium-239 is caused by their absorption of neutrons.
Cold, thermal, and hot neutron radiation is commonly employed in neutron scattering facilities, where the radiation is used in a similar way one uses X-rays for the analysis of condensed matter. Neutrons are complementary to the latter in terms of atomic contrasts by different scattering cross sections; sensitivity to magnetism; energy range for inelastic neutron spectroscopy; and deep penetration into matter.
The development of «neutron lenses» based on total internal reflection within hollow glass capillary tubes or by reflection from dimpled aluminum plates has driven ongoing research into neutron microscopy and neutron/gamma ray tomography.[92][93][94][95]
A major use of neutrons is to excite delayed and prompt gamma rays from elements in materials. This forms the basis of neutron activation analysis (NAA) and prompt gamma neutron activation analysis (PGNAA). NAA is most often used to analyze small samples of materials in a nuclear reactor whilst PGNAA is most often used to analyze subterranean rocks around bore holes and industrial bulk materials on conveyor belts.
Another use of neutron emitters is the detection of light nuclei, in particular the hydrogen found in water molecules. When a fast neutron collides with a light nucleus, it loses a large fraction of its energy. By measuring the rate at which slow neutrons return to the probe after reflecting off of hydrogen nuclei, a neutron probe may determine the water content in soil.
Medical therapies[edit]
Because neutron radiation is both penetrating and ionizing, it can be exploited for medical treatments. However, neutron radiation can have the unfortunate side-effect of leaving the affected area radioactive. Neutron tomography is therefore not a viable medical application.
Fast neutron therapy uses high-energy neutrons typically greater than 20 MeV to treat cancer. Radiation therapy of cancers is based upon the biological response of cells to ionizing radiation. If radiation is delivered in small sessions to damage cancerous areas, normal tissue will have time to repair itself, while tumor cells often cannot.[96] Neutron radiation can deliver energy to a cancerous region at a rate an order of magnitude larger than gamma radiation.[97]
Beams of low-energy neutrons are used in boron neutron capture therapy to treat cancer. In boron neutron capture therapy, the patient is given a drug that contains boron and that preferentially accumulates in the tumor to be targeted. The tumor is then bombarded with very low-energy neutrons (although often higher than thermal energy) which are captured by the boron-10 isotope in the boron, which produces an excited state of boron-11 that then decays to produce lithium-7 and an alpha particle that have sufficient energy to kill the malignant cell, but insufficient range to damage nearby cells. For such a therapy to be applied to the treatment of cancer, a neutron source having an intensity of the order of a thousand million (109) neutrons per second per cm2 is preferred. Such fluxes require a research nuclear reactor.
Protection[edit]
Exposure to free neutrons can be hazardous, since the interaction of neutrons with molecules in the body can cause disruption to molecules and atoms, and can also cause reactions that give rise to other forms of radiation (such as protons). The normal precautions of radiation protection apply: Avoid exposure, stay as far from the source as possible, and keep exposure time to a minimum. But particular thought must be given to how to protect from neutron exposure. For other types of radiation, e.g., alpha particles, beta particles, or gamma rays, material of a high atomic number and with high density makes for good shielding; frequently, lead is used. However, this approach will not work with neutrons, since the absorption of neutrons does not increase straightforwardly with atomic number, as it does with alpha, beta, and gamma radiation. Instead one needs to look at the particular interactions neutrons have with matter (see the section on detection above). For example, hydrogen-rich materials are often used to shield against neutrons, since ordinary hydrogen both scatters and slows neutrons. This often means that simple concrete blocks or even paraffin-loaded plastic blocks afford better protection from neutrons than do far more dense materials. After slowing, neutrons may then be absorbed with an isotope that has high affinity for slow neutrons without causing secondary capture radiation, such as lithium-6.
Hydrogen-rich ordinary water affects neutron absorption in nuclear fission reactors: Usually, neutrons are so strongly absorbed by normal water that fuel enrichment with fissionable isotope is required.[clarification needed] The deuterium in heavy water has a very much lower absorption affinity for neutrons than does protium (normal light hydrogen). Deuterium is, therefore, used in CANDU-type reactors, in order to slow (moderate) neutron velocity, to increase the probability of nuclear fission compared to neutron capture.
Neutron temperature[edit]
Thermal neutrons[edit]
Thermal neutrons are free neutrons whose energies have a Maxwell–Boltzmann distribution with kT = 0.0253 eV (4.0×10−21 J) at room temperature. This gives characteristic (not average, or median) speed of 2.2 km/s. The name ‘thermal’ comes from their energy being that of the room temperature gas or material they are permeating. (see kinetic theory for energies and speeds of molecules). After a number of collisions (often in the range of 10–20) with nuclei, neutrons arrive at this energy level, provided that they are not absorbed.
In many substances, thermal neutron reactions show a much larger effective cross-section than reactions involving faster neutrons, and thermal neutrons can therefore be absorbed more readily (i.e., with higher probability) by any atomic nuclei that they collide with, creating a heavier – and often unstable – isotope of the chemical element as a result.
Most fission reactors use a neutron moderator to slow down, or thermalize the neutrons that are emitted by nuclear fission so that they are more easily captured, causing further fission. Others, called fast breeder reactors, use fission energy neutrons directly.
Cold neutrons[edit]
Cold neutrons are thermal neutrons that have been equilibrated in a very cold substance such as liquid deuterium. Such a cold source is placed in the moderator of a research reactor or spallation source. Cold neutrons are particularly valuable for neutron scattering experiments.[98]
The use of cold and very cold neutrons (VCN) have been a bit limited compared to the use of thermal neutrons due to the relatively lower flux and lack in optical components. However, Innovative solutions have been proposed to offer more options to the scientific community to promote the use of VCN.[99][100]
Cold neutron source providing neutrons at about the temperature of liquid hydrogen
Ultracold neutrons[edit]
Ultracold neutrons are produced by inelastic scattering of cold neutrons in substances with a low neutron absorption cross section at a temperature of a few kelvins, such as solid deuterium[101] or superfluid helium.[102] An alternative production method is the mechanical deceleration of cold neutrons exploiting the Doppler shift.[103][104]
Fission energy neutrons[edit]
A fast neutron is a free neutron with a kinetic energy level close to 1 MeV (1.6×10−13 J), hence a speed of ~14000 km/s (~ 5% of the speed of light). They are named fission energy or fast neutrons to distinguish them from lower-energy thermal neutrons, and high-energy neutrons produced in cosmic showers or accelerators. Fast neutrons are produced by nuclear processes such as nuclear fission. Neutrons produced in fission, as noted above, have a Maxwell–Boltzmann distribution of kinetic energies from 0 to ~14 MeV, a mean energy of 2 MeV (for 235U fission neutrons), and a mode of only 0.75 MeV, which means that more than half of them do not qualify as fast (and thus have almost no chance of initiating fission in fertile materials, such as 238U and 232Th).
Fast neutrons can be made into thermal neutrons via a process called moderation. This is done with a neutron moderator. In reactors, typically heavy water, light water, or graphite are used to moderate neutrons.
Fusion neutrons[edit]
The fusion reaction rate increases rapidly with temperature until it maximizes and then gradually drops off. The D–T rate peaks at a lower temperature (about 70 keV, or 800 million kelvins) and at a higher value than other reactions commonly considered for fusion energy.
D–T (deuterium–tritium) fusion is the fusion reaction that produces the most energetic neutrons, with 14.1 MeV of kinetic energy and traveling at 17% of the speed of light. D–T fusion is also the easiest fusion reaction to ignite, reaching near-peak rates even when the deuterium and tritium nuclei have only a thousandth as much kinetic energy as the 14.1 MeV that will be produced.
14.1 MeV neutrons have about 10 times as much energy as fission neutrons, and are very effective at fissioning even non-fissile heavy nuclei, and these high-energy fissions produce more neutrons on average than fissions by lower-energy neutrons. This makes D–T fusion neutron sources such as proposed tokamak power reactors useful for transmutation of transuranic waste. 14.1 MeV neutrons can also produce neutrons by knocking them loose from nuclei.
On the other hand, these very high-energy neutrons are less likely to simply be captured without causing fission or spallation. For these reasons, nuclear weapon design extensively uses D–T fusion 14.1 MeV neutrons to cause more fission. Fusion neutrons are able to cause fission in ordinarily non-fissile materials, such as depleted uranium (uranium-238), and these materials have been used in the jackets of thermonuclear weapons. Fusion neutrons also can cause fission in substances that are unsuitable or difficult to make into primary fission bombs, such as reactor grade plutonium. This physical fact thus causes ordinary non-weapons grade materials to become of concern in certain nuclear proliferation discussions and treaties.
Other fusion reactions produce much less energetic neutrons. D–D fusion produces a 2.45 MeV neutron and helium-3 half of the time, and produces tritium and a proton but no neutron the rest of the time. D–3He fusion produces no neutron.
Intermediate-energy neutrons[edit]
A fission energy neutron that has slowed down but not yet reached thermal energies is called an epithermal neutron.
Cross sections for both capture and fission reactions often have multiple resonance peaks at specific energies in the epithermal energy range. These are of less significance in a fast-neutron reactor, where most neutrons are absorbed before slowing down to this range, or in a well-moderated thermal reactor, where epithermal neutrons interact mostly with moderator nuclei, not with either fissile or fertile actinide nuclides. But in a partially moderated reactor with more interactions of epithermal neutrons with heavy metal nuclei, there are greater possibilities for transient changes in reactivity that might make reactor control more difficult.
Ratios of capture reactions to fission reactions are also worse (more captures without fission) in most nuclear fuels such as plutonium-239, making epithermal-spectrum reactors using these fuels less desirable, as captures not only waste the one neutron captured but also usually result in a nuclide that is not fissile with thermal or epithermal neutrons, though still fissionable with fast neutrons. The exception is uranium-233 of the thorium cycle, which has good capture-fission ratios at all neutron energies.
High-energy neutrons[edit]
High-energy neutrons have much more energy than fission energy neutrons and are generated as secondary particles by particle accelerators or in the atmosphere from cosmic rays. These high-energy neutrons are extremely efficient at ionization and far more likely to cause cell death than X-rays or protons.[105][106]
See also[edit]
Wikimedia Commons has media related to Neutrons.
- Ionizing radiation
- Isotope
- List of particles
- Neutron radiation and the Sievert radiation scale
- Neutronium
- Nuclear reaction
- Nucleosynthesis
- Neutron capture nucleosynthesis
- R-process
- S-process
- Thermal reactor
Neutron sources[edit]
- Neutron generator
- Neutron source
Processes involving neutrons[edit]
- Neutron bomb
- Neutron diffraction
- Neutron flux
- Neutron transport
- Cosmogenic radionuclide dating
References[edit]
- ^ Ernest Rutherford Archived 2011-08-03 at the Wayback Machine. Chemed.chem.purdue.edu. Retrieved on 2012-08-16.
- ^ a b 1935 Nobel Prize in Physics Archived 2017-10-03 at the Wayback Machine. Nobelprize.org. Retrieved on 2012-08-16.
- ^ a b «2018 CODATA recommended values» https://physics.nist.gov/cuu/Constants/index.html Archived 2018-01-22 at the Wayback Machine
- ^ a b c d e f Mohr, P.J.; Taylor, B.N. and Newell, D.B. (2014), «The 2014 CODATA Recommended Values of the Fundamental Physical Constants» Archived 2013-10-09 at the Wayback Machine (Web Version 7.0). The database was developed by J. Baker, M. Douma, and S. Kotochigova. (2014). National Institute of Standards and Technology, Gaithersburg, Maryland 20899.
- ^ a b Zyla, P. A. (2020). «n MEAN LIFE». PDG Live: 2020 Review of Particle Physics. Particle Data Group. Archived from the original on 17 January 2021. Retrieved 25 February 2021.
- ^ a b Olive, K.A.; (Particle Data Group); et al. (2014). «Review of Particle Physics» (PDF). Chinese Physics C. 38 (9): 1–708. arXiv:1412.1408. Bibcode:2014ChPhC..38i0001O. doi:10.1088/1674-1137/38/9/090001. PMID 10020536. S2CID 118395784. Archived (PDF) from the original on 2020-06-01. Retrieved 2017-10-26.
- ^ Thomas, A.W.; Weise, W. (2001), The Structure of the Nucleon, Wiley-WCH, Berlin, ISBN 978-3-527-40297-7
- ^ a b
Chadwick, James (1932). «Possible Existence of a Neutron». Nature. 129 (3252): 312. Bibcode:1932Natur.129Q.312C. doi:10.1038/129312a0. S2CID 4076465. - ^ Hahn, O. & Strassmann, F. (1939). «Über den Nachweis und das Verhalten der bei der Bestrahlung des Urans mittels Neutronen entstehenden Erdalkalimetalle» [On the detection and characteristics of the alkaline earth metals formed by irradiation of uranium with neutrons]. Die Naturwissenschaften. 27 (1): 11–15. Bibcode:1939NW…..27…11H. doi:10.1007/BF01488241. S2CID 5920336.
- ^ a b c Glasstone, Samuel; Dolan, Philip J., eds. (1977), The Effects of Nuclear Weapons (3rd ed.), U.S. Dept. of Defense and Energy Research and Development Administration, U.S. Government Printing Office, ISBN 978-1-60322-016-3
- ^ a b c Nakamura, K (2010). «Review of Particle Physics». Journal of Physics G. 37 (7A): 1–708. Bibcode:2010JPhG…37g5021N. doi:10.1088/0954-3899/37/7A/075021. PMID 10020536. PDF with 2011 partial update for the 2012 edition Archived 2012-09-20 at the Wayback Machine.
The exact value of the mean lifetime is still uncertain, due to conflicting results from experiments.
The Particle Data Group reports values up to six seconds apart (more than four standard deviations), commenting that «our 2006, 2008, and 2010 Reviews stayed with 885.7±0.8 s; but we noted that in light of SEREBROV 05 our value should be regarded as suspect until further experiments clarified matters. Since our 2010 Review, PICHLMAIER 10 has obtained a mean life of 880.7±1.8 s, closer to the value of SEREBROV 05 than to our average. And SEREBROV 10B[…] claims their values should be lowered by about 6 s, which would bring them into line with the two lower values. But those re-evaluations have not received an enthusiastic response from the experimenters in question; and in any case the Particle Data Group would have to await published changes (by those experimenters) of published values.At this point, we can think of nothing better to do than to average the seven best but discordant measurements, getting 881.5±1.5 s. Note that the error includes a scale factor of 2.7. This is a jump of 4.2 old (and 2.8 new) standard deviations. This state of affairs is a particularly unhappy one, because the value is so important. We again call upon the experimenters to clear this up.»
- ^ a b
Carson, M.J.; et al. (2004). «Neutron background in large-scale xenon detectors for dark matter searches». Astroparticle Physics. 21 (6): 667–687. arXiv:hep-ex/0404042. Bibcode:2004APh….21..667C. doi:10.1016/j.astropartphys.2004.05.001. S2CID 17887096. - ^ Nudat 2 Archived 2009-08-17 at the Wayback Machine. Nndc.bnl.gov. Retrieved on 2010-12-04.
- ^ Povh, B.; Rith, K.; Scholz, C.; Zetsche, F. (2002). Particles and Nuclei: An Introduction to the Physical Concepts. Berlin: Springer-Verlag. p. 73. ISBN 978-3-540-43823-6.
- ^
Basdevant, J.-L.; Rich, J.; Spiro, M. (2005). Fundamentals in Nuclear Physics. Springer. p. 155. ISBN 978-0-387-01672-6. - ^ Tipler, Paul Allen; Llewellyn, Ralph A. (2002). Modern Physics (4 ed.). Macmillan. p. 310. ISBN 978-0-7167-4345-3. Archived from the original on 2022-04-07. Retrieved 2020-08-27.
- ^
Adair, R.K. (1989). The Great Design: Particles, Fields, and Creation. Oxford University Press. p. 214. Bibcode:1988gdpf.book…..A. - ^
Cottingham, W.N.; Greenwood, D.A. (1986). An Introduction to Nuclear Physics. Cambridge University Press. ISBN 9780521657334. - ^ a b
Rutherford, E. (1920). «Nuclear Constitution of Atoms». Proceedings of the Royal Society A. 97 (686): 374–400. Bibcode:1920RSPSA..97..374R. doi:10.1098/rspa.1920.0040. - ^ Harkins, William (1921). «The constitution and stability of atomic nuclei. (A contribution to the subject of inorganic evolution.)». Philos. Mag. 42 (249): 305. doi:10.1080/14786442108633770.
- ^ a b Feather, N. (1960). «A history of neutrons and nuclei. Part 1». Contemporary Physics. 1 (3): 191–203. Bibcode:1960ConPh…1..191F. doi:10.1080/00107516008202611.
- ^ Pauli, Wolfgang; Hermann, A.; Meyenn, K.v; Weisskopff, V.F (1985). «Das Jahr 1932 Die Entdeckung des Neutrons». Wolfgang Pauli. Sources in the History of Mathematics and Physical Sciences. Vol. 6. pp. 105–144. doi:10.1007/978-3-540-78801-0_3. ISBN 978-3-540-13609-5.
- ^ Hendry, John, ed. (1984). Cambridge Physics in the Thirties. Bristol: Adam Hilger. ISBN 978-0852747612.
- ^ Brown, Laurie M. (1978). «The idea of the neutrino». Physics Today. 31 (9): 23–28. Bibcode:1978PhT….31i..23B. doi:10.1063/1.2995181.
- ^ Friedlander G., Kennedy J.W. and Miller J.M. (1964) Nuclear and Radiochemistry (2nd edition), Wiley, pp. 22–23 and 38–39
- ^ a b Stuewer, Roger H. (1985). «Niels Bohr and Nuclear Physics». In French, A.P.; Kennedy, P.J. (eds.). Niels Bohr: A Centenary Volume. Harvard University Press. pp. 197–220. ISBN 978-0674624160.
- ^ Pais, Abraham (1986). Inward Bound. Oxford: Oxford University Press. p. 299. ISBN 978-0198519973.
- ^ Klein, O. (1929). «Die Reflexion von Elektronen an einem Potentialsprung nach der relativistischen Dynamik von Dirac». Zeitschrift für Physik. 53 (3–4): 157–165. Bibcode:1929ZPhy…53..157K. doi:10.1007/BF01339716. S2CID 121771000.
- ^ Bothe, W.; Becker, H. (1930). «Künstliche Erregung von Kern-γ-Strahlen» [Artificial excitation of nuclear γ-radiation]. Zeitschrift für Physik. 66 (5–6): 289–306. Bibcode:1930ZPhy…66..289B. doi:10.1007/BF01390908. S2CID 122888356.
- ^ Becker, H.; Bothe, W. (1932). «Die in Bor und Beryllium erregten γ-Strahlen» [Γ-rays excited in boron and beryllium]. Zeitschrift für Physik. 76 (7–8): 421–438. Bibcode:1932ZPhy…76..421B. doi:10.1007/BF01336726. S2CID 121188471.
- ^ Joliot-Curie, Irène & Joliot, Frédéric (1932). «Émission de protons de grande vitesse par les substances hydrogénées sous l’influence des rayons γ très pénétrants» [Emission of high-speed protons by hydrogenated substances under the influence of very penetrating γ-rays]. Comptes Rendus. 194: 273. Archived from the original on 2022-03-04. Retrieved 2012-06-16.
- ^ Brown, Andrew (1997). The Neutron and the Bomb: A Biography of Sir James Chadwick. Oxford University Press. ISBN 978-0-19-853992-6.
- ^ «Atop the Physics Wave: Rutherford Back in Cambridge, 1919–1937». Rutherford’s Nuclear World. American Institute of Physics. 2011–2014. Archived from the original on 21 October 2014. Retrieved 19 August 2014.
- ^ Chadwick, J. (1933). «Bakerian Lecture. The Neutron». Proceedings of the Royal Society A. 142 (846): 1–25. Bibcode:1933RSPSA.142….1C. doi:10.1098/rspa.1933.0152.
- ^ Heisenberg, W. (1932). «Über den Bau der Atomkerne. I». Zeitschrift für Physik. 77 (1–2): 1–11. Bibcode:1932ZPhy…77….1H. doi:10.1007/BF01342433. S2CID 186218053.
- ^ Heisenberg, W. (1932). «Über den Bau der Atomkerne. II». Zeitschrift für Physik. 78 (3–4): 156–164. Bibcode:1932ZPhy…78..156H. doi:10.1007/BF01337585. S2CID 186221789.
- ^ Heisenberg, W. (1933). «Über den Bau der Atomkerne. III». Zeitschrift für Physik. 80 (9–10): 587–596. Bibcode:1933ZPhy…80..587H. doi:10.1007/BF01335696. S2CID 126422047.
- ^ Iwanenko, D. (1932). «The Neutron Hypothesis». Nature. 129 (3265): 798. Bibcode:1932Natur.129..798I. doi:10.1038/129798d0. S2CID 4096734.
- ^ Miller A.I. (1995) Early Quantum Electrodynamics: A Sourcebook, Cambridge University Press, Cambridge, ISBN 0521568919, pp. 84–88.
- ^ Wilson, Fred L. (1968). «Fermi’s Theory of Beta Decay». American Journal of Physics. 36 (12): 1150–1160. Bibcode:1968AmJPh..36.1150W. doi:10.1119/1.1974382.
- ^ Chadwick, J.; Goldhaber, M. (1934). «A nuclear photo-effect: disintegration of the diplon by gamma rays». Nature. 134 (3381): 237–238. Bibcode:1934Natur.134..237C. doi:10.1038/134237a0. S2CID 4137231.
- ^ Chadwick, J.; Goldhaber, M. (1935). «A nuclear photoelectric effect». Proceedings of the Royal Society of London A. 151 (873): 479–493. Bibcode:1935RSPSA.151..479C. doi:10.1098/rspa.1935.0162.
- ^ Cooper, Dan (1999). Enrico Fermi: And the Revolutions in Modern physics. New York: Oxford University Press. ISBN 978-0-19-511762-2. OCLC 39508200.
- ^ Hahn, O. (1958). «The Discovery of Fission». Scientific American. 198 (2): 76–84. Bibcode:1958SciAm.198b..76H. doi:10.1038/scientificamerican0258-76.
- ^ Rife, Patricia (1999). Lise Meitner and the dawn of the nuclear age. Basel, Switzerland: Birkhäuser. ISBN 978-0-8176-3732-3.
- ^ Hahn, O.; Strassmann, F. (10 February 1939). «Proof of the Formation of Active Isotopes of Barium from Uranium and Thorium Irradiated with Neutrons; Proof of the Existence of More Active Fragments Produced by Uranium Fission». Die Naturwissenschaften. 27 (6): 89–95. Bibcode:1939NW…..27…89H. doi:10.1007/BF01488988. S2CID 33512939.
- ^ «The Nobel Prize in Chemistry 1944». Nobel Foundation. Archived from the original on 2018-12-26. Retrieved 2007-12-17.
- ^ Bernstein, Jeremy (2001). Hitler’s uranium club: the secret recordings at Farm Hall. New York: Copernicus. p. 281. ISBN 978-0-387-95089-1.
- ^ «The Nobel Prize in Chemistry 1944: Presentation Speech». Nobel Foundation. Archived from the original on 2007-10-25. Retrieved 2008-01-03.
- ^ Sir James Chadwick’s Discovery of Neutrons Archived 2011-10-26 at the Wayback Machine. ANS Nuclear Cafe. Retrieved on 2012-08-16.
- ^ Particle Data Group Summary Data Table on Baryons Archived 2011-09-10 at the Wayback Machine. lbl.gov (2007). Retrieved on 2012-08-16.
- ^ Tanabashi, M. (2018). «The Review of Particle Physics». Physical Review D. 54 (1): 1653. doi:10.1103/physrevd.54.1. PMID 10020536. Archived from the original on 2020-10-19. Retrieved 2020-10-14.
- ^ Basic Ideas and Concepts in Nuclear Physics: An Introductory Approach, Third Edition; K. Heyde Taylor & Francis 2004. Print ISBN 978-0-7503-0980-6, 978-1-4200-5494-1. doi:10.1201/9781420054941. full text[permanent dead link]
- ^ Greene, GL; et al. (1986). «New determination of the deuteron binding energy and the neutron mass». Physical Review Letters. 56 (8): 819–822. Bibcode:1986PhRvL..56..819G. doi:10.1103/PhysRevLett.56.819. PMID 10033294.
- ^ Byrne, J. Neutrons, Nuclei, and Matter, Dover Publications, Mineola, New York, 2011, ISBN 0486482383, pp. 18–19
- ^ Alvarez, L.W; Bloch, F. (1940). «A quantitative determination of the neutron magnetic moment in absolute nuclear magnetons». Physical Review. 57 (2): 111–122. Bibcode:1940PhRv…57..111A. doi:10.1103/physrev.57.111.
- ^ a b Gell, Y.; Lichtenberg, D.B. (1969). «Quark model and the magnetic moments of proton and neutron». Il Nuovo Cimento A. Series 10. 61 (1): 27–40. Bibcode:1969NCimA..61…27G. doi:10.1007/BF02760010. S2CID 123822660.
- ^ a b c Perkins, Donald H. (1982). Introduction to High Energy Physics. Addison Wesley, Reading, Massachusetts. pp. 201–202. ISBN 978-0-201-05757-7.
- ^
Greenberg, O.W. (2009), «Color charge degree of freedom in particle physics», Compendium of Quantum Physics, Springer Berlin Heidelberg, pp. 109–111, arXiv:0805.0289, doi:10.1007/978-3-540-70626-7_32, ISBN 978-3-540-70622-9, S2CID 17512393 - ^ Beg, M.A.B.; Lee, B.W.; Pais, A. (1964). «SU(6) and electromagnetic interactions». Physical Review Letters. 13 (16): 514–517, erratum 650. Bibcode:1964PhRvL..13..514B. doi:10.1103/physrevlett.13.514.
- ^ Sakita, B. (1964). «Electromagnetic properties of baryons in the supermultiplet scheme of elementary particles». Physical Review Letters. 13 (21): 643–646. Bibcode:1964PhRvL..13..643S. doi:10.1103/physrevlett.13.643.
- ^ a b Cho, Adrian (2 April 2010). «Mass of the Common Quark Finally Nailed Down». Science. American Association for the Advancement of Science. Archived from the original on 27 August 2015. Retrieved 27 September 2014.
- ^ Wilczek, F. (2003). «The Origin of Mass» (PDF). MIT Physics Annual: 24–35. Archived (PDF) from the original on June 20, 2015. Retrieved May 8, 2015.
- ^
Ji, Xiangdong (1995). «A QCD Analysis of the Mass Structure of the Nucleon». Physical Review Letters. 74 (7): 1071–1074. arXiv:hep-ph/9410274. Bibcode:1995PhRvL..74.1071J. doi:10.1103/PhysRevLett.74.1071. PMID 10058927. S2CID 15148740. - ^ Martinelli, G.; Parisi, G.; Petronzio, R.; Rapuano, F. (1982). «The proton and neutron magnetic moments in lattice QCD» (PDF). Physics Letters B. 116 (6): 434–436. Bibcode:1982PhLB..116..434M. doi:10.1016/0370-2693(82)90162-9. Archived (PDF) from the original on 2020-04-20. Retrieved 2019-08-25.
- ^ Kincade, Kathy (2 February 2015). «Pinpointing the magnetic moments of nuclear matter». Phys.org. Archived from the original on 2 May 2015. Retrieved May 8, 2015.
- ^ a b J. Byrne (2011). Neutrons, Nuclei and Matter: An exploration of the physics of slow neutrons. Mineola, NY: Dover Publications. pp. 28–31. ISBN 978-0486482385.
- ^ Hughes, D.J.; Burgy, M.T. (1949). «Reflection and polarization of neutrons by magnetized mirrors» (PDF). Physical Review. 76 (9): 1413–1414. Bibcode:1949PhRv…76.1413H. doi:10.1103/PhysRev.76.1413. Archived from the original (PDF) on 2016-08-13. Retrieved 2016-06-26.
- ^ Sherwood, J.E.; Stephenson, T.E.; Bernstein, S. (1954). «Stern-Gerlach experiment on polarized neutrons». Physical Review. 96 (6): 1546–1548. Bibcode:1954PhRv…96.1546S. doi:10.1103/PhysRev.96.1546.
- ^
Miller, G.A. (2007). «Charge Densities of the Neutron and Proton». Physical Review Letters. 99 (11): 112001. arXiv:0705.2409. Bibcode:2007PhRvL..99k2001M. doi:10.1103/PhysRevLett.99.112001. PMID 17930428. S2CID 119120565. - ^ «Pear-shaped particles probe big-bang mystery» (Press release). University of Sussex. 20 February 2006. Archived from the original on 2011-06-07. Retrieved 2009-12-14.
- ^ A cryogenic experiment to search for the EDM of the neutron Archived 2012-02-16 at the Wayback Machine. Hepwww.rl.ac.uk. Retrieved on 2012-08-16.
- ^ Search for the neutron electric dipole moment: nEDM Archived 2015-09-25 at the Wayback Machine. Nedm.web.psi.ch (2001-09-12). Retrieved on 2012-08-16.
- ^ US nEDM ORNL experiment public page Archived 2017-04-30 at the Wayback Machine. Retrieved on 2017-02-08.
- ^ SNS Neutron EDM Experiment Archived 2011-02-10 at the Wayback Machine. P25ext.lanl.gov. Retrieved on 2012-08-16.
- ^ Measurement of the Neutron Electric Dipole Moment Archived 2011-08-23 at the Wayback Machine. Nrd.pnpi.spb.ru. Retrieved on 2012-08-16.
- ^ Kisamori, K.; et al. (2016). «Candidate Resonant Tetraneutron State Populated by the He4(He8,Be8) Reaction». Physical Review Letters. 116 (5): 052501. Bibcode:2016PhRvL.116e2501K. doi:10.1103/PhysRevLett.116.052501. PMID 26894705.
- ^ «Physicists find signs of four-neutron nucleus». 2016-02-24. Archived from the original on 2017-07-29. Retrieved 2017-06-27.
- ^ Orr, Nigel (2016-02-03). «Can Four Neutrons Tango?». Physics. 9: 14. Bibcode:2016PhyOJ…9…14O. doi:10.1103/Physics.9.14.
- ^ Spyrou, A.; et al. (2012). «First Observation of Ground State Dineutron Decay: 16Be». Physical Review Letters. 108 (10): 102501. Bibcode:2012PhRvL.108j2501S. doi:10.1103/PhysRevLett.108.102501. PMID 22463404.
- ^ Llanes-Estrada, Felipe J.; Moreno Navarro, Gaspar (2012). «Cubic neutrons». Modern Physics Letters A. 27 (6): 1250033–1–1250033–7. arXiv:1108.1859. Bibcode:2012MPLA…2750033L. doi:10.1142/S0217732312500332. S2CID 118407306.
- ^ Knoll, Glenn F. (1979). «Ch. 14». Radiation Detection and Measurement. John Wiley & Sons. ISBN 978-0471495451.
- ^ Ghosh, P.; W. Fu; M. J. Harrison; P. K. Doyle; N. S. Edwards; J. A. Roberts; D. S. McGregor (2018). «A high-efficiency, low-Ĉerenkov Micro-Layered Fast-Neutron Detector for the TREAT hodoscope». Nuclear Instruments and Methods in Physics: A. 904: 100–106. Bibcode:2018NIMPA.904..100G. doi:10.1016/j.nima.2018.07.035. S2CID 126130994. Archived from the original on 2022-04-12. Retrieved 2020-04-14.
- ^ Ghosh, P.; D. M. Nichols; W. Fu; J. A. Roberts; D. S. McGregor (2020). «Gamma-Ray Rejection of the SiPM-coupled Micro-Layered Fast-Neutron Detector». 2019 IEEE Nuclear Science Symposium and Medical Imaging Conference (NSS/MIC): 1–3. doi:10.1109/NSS/MIC42101.2019.9059869. ISBN 978-1-7281-4164-0. S2CID 204877955.
- ^ Köhn, C.; Ebert, U. (2015). «Calculation of beams of positrons, neutrons and protons associated with terrestrial gamma-ray flashes» (PDF). Journal of Geophysical Research: Atmospheres. 23 (4): 1620–1635. Bibcode:2015JGRD..120.1620K. doi:10.1002/2014JD022229. Archived (PDF) from the original on 2019-12-23. Retrieved 2019-08-25.
- ^ Köhn, C.; Diniz, G.; Harakeh, Muhsin (2017). «Production mechanisms of leptons, photons, and hadrons and their possible feedback close to lightning leaders». Journal of Geophysical Research: Atmospheres. 122 (2): 1365–1383. Bibcode:2017JGRD..122.1365K. doi:10.1002/2016JD025445. PMC 5349290. PMID 28357174.
- ^ Clowdsley, MS; Wilson, JW; Kim, MH; Singleterry, RC; Tripathi, RK; Heinbockel, JH; Badavi, FF; Shinn, JL (2001). «Neutron Environments on the Martian Surface» (PDF). Physica Medica. 17 (Suppl 1): 94–96. PMID 11770546. Archived from the original (PDF) on 2005-02-25.
- ^ Byrne, J. Neutrons, Nuclei, and Matter, Dover Publications, Mineola, New York, 2011, ISBN 0486482383, pp. 32–33.
- ^ «Isotopes and Radioactivity Tutorial». Archived from the original on 2020-02-14. Retrieved 2020-04-16.
- ^ Science/Nature |Q&A: Nuclear fusion reactor Archived 2022-02-25 at the Wayback Machine. BBC News (2006-02-06). Retrieved on 2010-12-04.
- ^ Byrne, J. Neutrons, Nuclei, and Matter, Dover Publications, Mineola, New York, 2011, ISBN 0486482383, p. 453.
- ^ Kumakhov, M.A.; Sharov, V.A. (1992). «A neutron lens». Nature. 357 (6377): 390–391. Bibcode:1992Natur.357..390K. doi:10.1038/357390a0. S2CID 37062511.
- ^ Physorg.com, «New Way of ‘Seeing’: A ‘Neutron Microscope'» Archived 2012-01-24 at the Wayback Machine. Physorg.com (2004-07-30). Retrieved on 2012-08-16.
- ^ «NASA Develops a Nugget to Search for Life in Space» Archived 2014-03-08 at the Wayback Machine. NASA.gov (2007-11-30). Retrieved on 2012-08-16.
- ^ Ioffe, A.; Dabagov, S.; Kumakhov, M. (1995-01-01). «Effective neutron bending at large angles». Neutron News. 6 (3): 20–21. doi:10.1080/10448639508217696. ISSN 1044-8632.
- ^ Hall EJ (2000). Radiobiology for the Radiologist. Lippincott Williams & Wilkins; 5th edition
- ^ Johns HE and Cunningham JR (1978). The Physics of Radiology. Charles C Thomas 3rd edition
- ^ brian.maranville@nist.gov (2017-04-17). «How neutrons are useful». NIST. Archived from the original on 2021-01-25. Retrieved 2021-01-21.
- ^ E Hadden; Y Iso; A Kume; K Umemoto; T Jenke; M Fally; J Klepp; Y Tomita (2022). «HIGHLY EFFICIENT HOLOGRAPHIC OPTICAL ELEMENTS FOR COLD NEUTRON EXPERIMENTS». doi:10.13140/RG.2.2.26033.04963.
- ^ Hadden, Elhoucine; Iso, Yuko; Kume, Atsushi; Umemoto, Koichi; Jenke, Tobias; Fally, Martin; Klepp, Jürgen; Tomita, Yasuo (2022-05-24). McLeod, Robert R; Tomita, Yasuo; Sheridan, John T; Pascual Villalobos, Inmaculada (eds.). «Nanodiamond-based nanoparticle-polymer composite gratings with extremely large neutron refractive index modulation». Photosensitive Materials and Their Applications II. SPIE. 12151: 70–76. Bibcode:2022SPIE12151E..09H. doi:10.1117/12.2623661. ISBN 9781510651784. S2CID 249056691.
- ^ B. Lauss (May 2012). «Startup of the high-intensity ultracold neutron source at the Paul Scherrer Institute». Hyperfine Interact. 211 (1): 21–25. arXiv:1202.6003. Bibcode:2012HyInt.211…21L. doi:10.1007/s10751-012-0578-7. S2CID 119164071.
- ^ R. Golub & J. M. Pendlebury (1977). «The interaction of Ultra-Cold Neutrons (UCN) with liquid helium and a superthermal UCN source». Phys. Lett. A. 62 (5): 337–339. Bibcode:1977PhLA…62..337G. doi:10.1016/0375-9601(77)90434-0.
- ^ A. Steyerl; H. Nagel; F.-X. Schreiber; K.-A. Steinhauser; R. Gähler; W. Gläser; P. Ageron; J. M. Astruc; W. Drexel; G. Gervais & W. Mampe (1986). «A new source of cold and ultracold neutrons». Phys. Lett. A. 116 (7): 347–352. Bibcode:1986PhLA..116..347S. doi:10.1016/0375-9601(86)90587-6.
- ^ Stefan Döge; Jürgen Hingerl & Christoph Morkel (Feb 2020). «Measured velocity spectra and neutron densities of the PF2 ultracold-neutron beam ports at the Institut Laue–Langevin». Nucl. Instrum. Methods A. 953: 163112. arXiv:2001.04538. Bibcode:2020NIMPA.95363112D. doi:10.1016/j.nima.2019.163112. S2CID 209942845. Archived from the original on 2021-02-24. Retrieved 2020-04-24.
- ^ Freeman, Tami (May 23, 2008). «Facing up to secondary neutrons». Medical Physics Web. Archived from the original on 2010-12-20. Retrieved 2011-02-08.
- ^ Heilbronn, L.; Nakamura, T; Iwata, Y; Kurosawa, T; Iwase, H; Townsend, LW (2005). «Expand+Overview of secondary neutron production relevant to shielding in space». Radiation Protection Dosimetry. 116 (1–4): 140–143. doi:10.1093/rpd/nci033. PMID 16604615. Archived from the original on 2019-01-26. Retrieved 2019-01-25.
Further reading[edit]
- James Byrne, Neutrons, Nuclei and Matter: An Exploration of the Physics of Slow Neutrons. Mineola, New York: Dover Publications, 2011. ISBN 0486482383.
- Abraham Pais, Inward Bound, Oxford: Oxford University Press, 1986. ISBN 0198519974.
- Sin-Itiro Tomonaga, The Story of Spin, The University of Chicago Press, 1997
- Herwig Schopper, Weak interactions and nuclear beta decay, Publisher, North-Holland Pub. Co., 1966.
- Annotated bibliography for neutrons from the Alsos Digital Library for Nuclear Issues

The quark content of the neutron. The color assignment of individual quarks is arbitrary, but all three colors must be present. Forces between quarks are mediated by gluons. |
|
| Classification | Baryon |
|---|---|
| Composition | 1 up quark, 2 down quarks |
| Statistics | Fermionic |
| Family | Hadron |
| Interactions | Gravity, weak, strong, electromagnetic |
| Symbol | n , n0 , N0 |
| Antiparticle | Antineutron |
| Theorized | Ernest Rutherford[1] (1920) |
| Discovered | James Chadwick[2] (1932) |
| Mass | 1.67492749804(95)×10−27 kg[3] 939.56542052(54) MeV/c2[3] 1.00866491588(49) Da[4] |
| Mean lifetime | 879.4(6) s (free)[5] |
| Electric charge | 0 e (−2±  ×10−22 e (experimental limits)[6] ×10−22 e (experimental limits)[6] |
| Electric dipole moment | < 2.9×10−26 e⋅cm (experimental upper limit) |
| Electric polarizability | 1.16(15)×10−3 fm3 |
| Magnetic moment | −0.96623650(23)×10−26 J·T−1[4] −1.04187563(25)×10−3 μB[4] −1.91304273(45) μN[4] |
| Magnetic polarizability | 3.7(20)×10−4 fm3 |
| Spin | 1/2 |
| Isospin | −1/2 |
| Parity | +1 |
| Condensed | I(JP) = 1/2(1/2+) |
The neutron is a subatomic particle, symbol
n
or
n0
, which has a neutral (not positive or negative) charge, and a mass slightly greater than that of a proton. Protons and neutrons constitute the nuclei of atoms. Since protons and neutrons behave similarly within the nucleus, and each has a mass of approximately one atomic mass unit, they are both referred to as nucleons.[7] Their properties and interactions are described by nuclear physics. Protons and neutrons are not elementary particles; each is composed of three quarks.
The chemical properties of an atom are mostly determined by the configuration of electrons that orbit the atom’s heavy nucleus. The electron configuration is determined by the charge of the nucleus, which is determined by the number of protons, or atomic number. The number of neutrons is the neutron number. Neutrons do not affect the electron configuration, but the sum of atomic and neutron numbers is the mass of the nucleus.
Atoms of a chemical element that differ only in neutron number are called isotopes. For example, carbon, with atomic number 6, has an abundant isotope carbon-12 with 6 neutrons and a rare isotope carbon-13 with 7 neutrons. Some elements occur in nature with only one stable isotope, such as fluorine; Other elements occur with many stable isotopes, such as tin with ten stable isotopes, and some elements such as technetium have no stable isotope.
The properties of an atomic nucleus depend on both atomic and neutron numbers. With their positive charge, the protons within the nucleus are repelled by the long-range electromagnetic force, but the much stronger, but short-range, nuclear force binds the nucleons closely together. Neutrons are required for the stability of nuclei, with the exception of the single-proton hydrogen nucleus. Neutrons are produced copiously in nuclear fission and fusion. They are a primary contributor to the nucleosynthesis of chemical elements within stars through fission, fusion, and neutron capture processes.
The neutron is essential to the production of nuclear power. In the decade after the neutron was discovered by James Chadwick in 1932,[8] neutrons were used to induce many different types of nuclear transmutations. With the discovery of nuclear fission in 1938,[9] it was quickly realized that, if a fission event produced neutrons, each of these neutrons might cause further fission events, in a cascade known as a nuclear chain reaction.[10] These events and findings led to the first self-sustaining nuclear reactor (Chicago Pile-1, 1942) and the first nuclear weapon (Trinity, 1945).
Dedicated neutron sources like neutron generators, research reactors and spallation sources produce free neutrons for use in irradiation and in neutron scattering experiments. A free neutron spontaneously decays to a proton, an electron, and an antineutrino, with a mean lifetime of about 15 minutes.[11] Free neutrons do not directly ionize atoms, but they do indirectly cause ionizing radiation, so they can be a biological hazard, depending on dose.[10] A small natural «neutron background» flux of free neutrons exists on Earth, caused by cosmic ray showers, and by the natural radioactivity of spontaneously fissionable elements in the Earth’s crust.[12]
Description[edit]
An atomic nucleus is formed by a number of protons, Z (the atomic number), and a number of neutrons, N (the neutron number), bound together by the nuclear force. The atomic number determines the chemical properties of the atom, and the neutron number determines the isotope or nuclide.[10] The terms isotope and nuclide are often used synonymously, but they refer to chemical and nuclear properties, respectively. Isotopes are nuclides with the same atomic number, but different neutron number. Nuclides with the same neutron number, but different atomic number, are called isotones. The atomic mass number, A, is equal to the sum of atomic and neutron numbers. Nuclides with the same atomic mass number, but different atomic and neutron numbers, are called isobars.
The nucleus of the most common isotope of the hydrogen atom (with the chemical symbol 1H) is a lone proton. The nuclei of the heavy hydrogen isotopes deuterium (D or 2H) and tritium (T or 3H) contain one proton bound to one and two neutrons, respectively. All other types of atomic nuclei are composed of two or more protons and various numbers of neutrons. The most common nuclide of the common chemical element lead, 208Pb, has 82 protons and 126 neutrons, for example. The table of nuclides comprises all the known nuclides. Even though it is not a chemical element, the neutron is included in this table.[13]
The free neutron has a mass of 939565413.3 eV/c2, or 1.674927471×10−27 kg, or 1.00866491588 Da.[4] The neutron has a mean square radius of about 0.8×10−15 m, or 0.8 fm,[14] and it is a spin-½ fermion.[15] The neutron has no measurable electric charge. With its positive electric charge, the proton is directly influenced by electric fields, whereas the neutron is unaffected by electric fields. But the neutron has a magnetic moment, so the neutron is influenced by magnetic fields. The neutron’s magnetic moment has a negative value, because its orientation is opposite to the neutron’s spin.[16]
A free neutron is unstable, decaying to a proton, electron and antineutrino with a mean lifetime of just under 15 minutes (879.6±0.8 s).[5] This radioactive decay, known as beta decay, is possible because the mass of the neutron is slightly greater than that of the proton. The free proton is stable. However, neutrons or protons bound in a nucleus can be stable or unstable, depending on the nuclide. Beta decay, in which neutrons decay to protons, or vice versa, is governed by the weak force, and it requires the emission or absorption of electrons and neutrinos, or their antiparticles.
Nuclear fission caused by absorption of a neutron by uranium-235. The heavy nuclide fragments into lighter components and additional neutrons.
Protons and neutrons behave almost identically under the influence of the nuclear force within the nucleus. The concept of isospin, in which the proton and neutron are viewed as two quantum states of the same particle, is used to model the interactions of nucleons by the nuclear or weak forces. Because of the strength of the nuclear force at short distances, the binding energy of nucleons is more than seven orders of magnitude larger than the electromagnetic energy binding electrons in atoms. Nuclear reactions (such as nuclear fission) therefore have an energy density that is more than ten million times that of chemical reactions. Because of the mass–energy equivalence, nuclear binding energies reduce the mass of nuclei. Ultimately, the ability of the nuclear force to store energy arising from the electromagnetic repulsion of nuclear components is the basis for most of the energy that makes nuclear reactors or bombs possible. In nuclear fission, the absorption of a neutron by a heavy nuclide (e.g., uranium-235) causes the nuclide to become unstable and break into light nuclides and additional neutrons. The positively charged light nuclides then repel, releasing electromagnetic potential energy.
The neutron is classified as a hadron, because it is a composite particle made of quarks. The neutron is also classified as a baryon, because it is composed of three valence quarks.[17] The finite size of the neutron and its magnetic moment both indicate that the neutron is a composite, rather than elementary, particle. A neutron contains two down quarks with charge −1/3e and one up quark with charge +2/3e.
Like protons, the quarks of the neutron are held together by the strong force, mediated by gluons.[18] The nuclear force results from secondary effects of the more fundamental strong force.
Discovery[edit]
The story of the discovery of the neutron and its properties is central to the extraordinary developments in atomic physics that occurred in the first half of the 20th century, leading ultimately to the atomic bomb in 1945. In the 1911 Rutherford model, the atom consisted of a small positively charged massive nucleus surrounded by a much larger cloud of negatively charged electrons. In 1920, Ernest Rutherford suggested that the nucleus consisted of positive protons and neutrally charged particles, suggested to be a proton and an electron bound in some way.[19] Electrons were assumed to reside within the nucleus because it was known that beta radiation consisted of electrons emitted from the nucleus.[19]
About the time Rutherford suggested the neutral proton-electron composite, several other publications appeared making similar suggestions, and in 1921 the American chemist W.D. Harkins first named the hypothetical particle a «neutron».[20][21] The name derives from the Latin root for neutralis (neuter) and the Greek suffix -on (a suffix used in the names of subatomic particles, i.e. electron and proton).[22][23] References to the word neutron in connection with the atom can be found in the literature as early as 1899, however.[21]
Throughout the 1920s, physicists assumed that the atomic nucleus was composed of protons and «nuclear electrons»[24][25] but there were obvious problems. It was difficult to reconcile the proton–electron model for nuclei with the Heisenberg uncertainty relation of quantum mechanics.[26][27] The Klein paradox,[28] discovered by Oskar Klein in 1928, presented further quantum mechanical objections to the notion of an electron confined within a nucleus.[26] Observed properties of atoms and molecules were inconsistent with the nuclear spin expected from the proton–electron hypothesis. Both protons and electrons carry an intrinsic spin of 1/2ħ. Isotopes of the same species (i.e. having the same number of protons) can have both integer or fractional spin, i.e. the neutron spin must be also fractional (1/2ħ). But there is no way to arrange the spins of an electron and a proton (supposed to bond to form a neutron) to get the fractional spin of a neutron.
In 1931, Walther Bothe and Herbert Becker found that if alpha particle radiation from polonium fell on beryllium, boron, or lithium, an unusually penetrating radiation was produced. The radiation was not influenced by an electric field, so Bothe and Becker assumed it was gamma radiation.[29][30] The following year Irène Joliot-Curie and Frédéric Joliot-Curie in Paris showed that if this «gamma» radiation fell on paraffin, or any other hydrogen-containing compound, it ejected protons of very high energy.[31] Neither Rutherford nor James Chadwick at the Cavendish Laboratory in Cambridge were convinced by the gamma ray interpretation.[32] Chadwick quickly performed a series of experiments that showed that the new radiation consisted of uncharged particles with about the same mass as the proton.[8][33][34] These particles were neutrons. Chadwick won the 1935 Nobel Prize in Physics for this discovery.[2]
Models depicting the nucleus and electron energy levels in hydrogen, helium, lithium, and neon atoms. In reality, the diameter of the nucleus is about 100,000 times smaller than the diameter of the atom.
Models for an atomic nucleus consisting of protons and neutrons were quickly developed by Werner Heisenberg[35][36][37] and others.[38][39] The proton–neutron model explained the puzzle of nuclear spins. The origins of beta radiation were explained by Enrico Fermi in 1934 by the process of beta decay, in which the neutron decays to a proton by creating an electron and a (at the time undiscovered) neutrino.[40] In 1935, Chadwick and his doctoral student Maurice Goldhaber reported the first accurate measurement of the mass of the neutron.[41][42]
By 1934, Fermi had bombarded heavier elements with neutrons to induce radioactivity in elements of high atomic number. In 1938, Fermi received the Nobel Prize in Physics «for his demonstrations of the existence of new radioactive elements produced by neutron irradiation, and for his related discovery of nuclear reactions brought about by slow neutrons».[43] In 1938 Otto Hahn, Lise Meitner, and Fritz Strassmann discovered nuclear fission, or the fractionation of uranium nuclei into light elements, induced by neutron bombardment.[44][45][46] In 1945 Hahn received the 1944 Nobel Prize in Chemistry «for his discovery of the fission of heavy atomic nuclei».[47][48][49] The discovery of nuclear fission would lead to the development of nuclear power and the atomic bomb by the end of World War II.
Beta decay and the stability of the nucleus[edit]
Neutrons are a necessary constituent of any atomic nucleus that contains more than one proton. Interacting protons have a mutual electromagnetic repulsion that is stronger than their attractive nuclear interaction, so proton-only nuclei are unstable (see diproton and neutron–proton ratio).[50] Neutrons bind with protons and one another in the nucleus via the nuclear force, effectively moderating the repulsive forces between the protons and stabilizing the nucleus.
Within the nucleus, neutrons can decay to protons, or vice versa. This process is called beta decay, and, for the neutron, it requires the emission of an electron and an anti-neutrino:
n0
→
p+
+
e−
+
ν
e
where
p+
,
e−
, and
ν
e denote the proton, electron and electron antineutrino products, respectively.[51] The decay of the proton to a neutron is similar:
p+
→
n0
+
e+
+
ν
e
where
n0
,
e+
, and
ν
e denote the neutron, positron and electron neutrino products, respectively. In these reactions, the original particle is not composed of the product particles; rather, the product particles are created at the instant of the reaction.
The neutrons and protons bound in a nucleus form a quantum mechanical system wherein each nucleon is bound in a particular, hierarchical quantum state. The emitted particles, that is, the decay products, carry away the energy excess as a nucleon falls from one quantum state to a lower energy state, while the proton (or neutron) changes to a neutron (or proton). Such decay processes can occur only if allowed by basic energy conservation and quantum mechanical constraints. The stability of nuclei is a consequence of these constraints.
«Free» neutrons or protons are nucleons that exist independently, free of any nucleus. Since the neutron is slightly more massive than a proton, the decay of a free neutron to a proton is allowed, while the decay of a free proton is energetically disallowed. A high-energy collision of a proton and an electron or neutrino can result in a neutron, however.
Free neutron decay[edit]
Outside the nucleus, free neutrons are unstable and have a mean lifetime of 879.6±0.8 s (about 14 minutes, 40 seconds); therefore the half-life for this process (which differs from the mean lifetime by a factor of ln(2) = 0.693) is 610.1±0.7 s (about 10 minutes, 10 seconds).[11][52] This decay is only possible because the mass of the proton is less than that of the neutron. By the mass-energy equivalence, when a neutron decays to a proton this way, a lower energy state is attained.
For the free neutron the decay energy for this process (based on the masses of the neutron, proton, and electron) is 0.782343 MeV. The maximal energy of the beta decay electron (in the process wherein the neutrino receives a vanishingly small amount of kinetic energy) has been measured at 0.782±0.013 MeV.[53] The latter number is not well-enough measured to determine the comparatively tiny rest mass of the neutrino (which must in theory be subtracted from the maximal electron kinetic energy) as well as neutrino mass is constrained by many other methods.
A small fraction (about one in 1000) of free neutrons decay with the same products, but add an extra particle in the form of an emitted gamma ray:
n0
→
p+
+
e−
+
ν
e +
γ
This gamma ray may be thought of as an «internal bremsstrahlung» that arises from the electromagnetic interaction of the emitted beta particle with the proton. Internal bremsstrahlung gamma ray production is also a minor feature of beta decays of bound neutrons (as discussed below).
A schematic of the nucleus of an atom indicating
β−
radiation, the emission of a fast electron from the nucleus (the accompanying antineutrino is omitted). In the Rutherford model for the nucleus, red spheres were protons with positive charge and blue spheres were protons tightly bound to an electron with no net charge.
The inset shows beta decay of a free neutron as it is understood today; an electron and antineutrino are created in this process.
A very small minority of neutron decays (about four per million) are so-called «two-body (neutron) decays», in which a proton, electron and antineutrino are produced as usual, but the electron fails to gain the 13.6 eV necessary energy to escape the proton (the ionization energy of hydrogen), and therefore simply remains bound to it, as a neutral hydrogen atom (one of the «two bodies»). In this type of free neutron decay, almost all of the neutron decay energy is carried off by the antineutrino (the other «body»). (The hydrogen atom recoils with a speed of only about (decay energy)/(hydrogen rest energy) times the speed of light, or 250 km/s.)
Bound neutron decay[edit]
While a free neutron has a half life of about 10.2 min, most neutrons within nuclei are stable. According to the nuclear shell model, the protons and neutrons of a nuclide are a quantum mechanical system organized into discrete energy levels with unique quantum numbers. For a neutron to decay, the resulting proton requires an available state at lower energy than the initial neutron state. In stable nuclei the possible lower energy states are all filled, meaning each state is occupied by a pair of protons, one with spin up, another with spin down. When all available proton states are filled, the Pauli exclusion principle disallows the decay of a neutron to a proton within stable nuclei. The situation is similar to electrons of an atom, where electrons that occupy distinct atomic orbitals are prevented by the exclusion principle from decaying to lower, already-filled, energy states, with the emission of a photon.
Neutrons in unstable nuclei can decay by beta decay as described above. In this case, an energetically allowed quantum state is available for the proton resulting from the decay. One example of this decay is carbon-14 (6 protons, 8 neutrons) that decays to nitrogen-14 (7 protons, 7 neutrons) with a half-life of about 5,730 years.
Similarly, a proton inside a nucleus can decay into a neutron, if an energetically allowed quantum state is available for the neutron.
The transformation of a proton to a neutron inside of a nucleus is also possible through electron capture:
p+
+
e−
→
n0
+
ν
e
Positron capture by neutrons in nuclei that contain an excess of neutrons is also possible, but is hindered because positrons are repelled by the positive nucleus, and quickly annihilate when they encounter electrons. Similar, but far more rare, reactions involve the capture of a neutrino by a nucleon in inverse beta decay.
Competition of beta decay types[edit]
Three types of beta decay in competition are illustrated by the single isotope copper-64 (29 protons, 35 neutrons), which has a half-life of about 12.7 hours. This isotope has one unpaired proton and one unpaired neutron, so either the proton or the neutron can decay. This particular nuclide is almost equally likely to undergo proton decay (by positron emission, 18% or by electron capture, 43%) or neutron decay (by electron emission, 39%).
Decay of the neutron by elementary particle physics[edit]
Within the theoretical framework of Standard Model for particle physics, the neutron is composed of two down quarks and an up quark. The only possible decay mode for the neutron that conserves baryon number is for one of the neutron’s quarks to change flavour via the weak interaction. The decay of one of the neutron’s down quarks into a lighter up quark can be achieved by the emission of a W boson. By this process, the Standard Model description of beta decay, the neutron decays into a proton (which contains one down and two up quarks), an electron, and an electron antineutrino.
The decay of the proton to a neutron occurs similarly through the electroweak force. The decay of one of the proton’s up quarks into a down quark can be achieved by the emission of a W boson. The proton decays into a neutron, a positron, and an electron neutrino. This reaction can only occur within an atomic nucleus which has a quantum state at lower energy available for the created neutron.
Intrinsic properties[edit]
Mass[edit]
The mass of a neutron cannot be directly determined by mass spectrometry since it has no electric charge. But since the masses of a proton and of a deuteron can be measured with a mass spectrometer, the mass of a neutron can be deduced by subtracting proton mass from deuteron mass, with the difference being the mass of the neutron plus the binding energy of deuterium (expressed as a positive emitted energy). The latter can be directly measured by measuring the energy (

The energy of the gamma ray can be measured to high precision by X-ray diffraction techniques, as was first done by Bell and Elliot in 1948. The best modern (1986) values for neutron mass by this technique are provided by Greene, et al.[54] These give a neutron mass of:
- mneutron = 1.008644904(14) Da
The value for the neutron mass in MeV is less accurately known, due to less accuracy in the known conversion of Da to MeV/c2:[55]
- mneutron = 939.56563(28) MeV/c2.
Another method to determine the mass of a neutron starts from the beta decay of the neutron, when the momenta of the resulting proton and electron are measured.
Electric charge[edit]
The total electric charge of the neutron is 0 e. This zero value has been tested experimentally, and the present experimental limit for the charge of the neutron is −2(8)×10−22 e,[6] or −3(13)×10−41 C. This value is consistent with zero, given the experimental uncertainties (indicated in parentheses). By comparison, the charge of the proton is +1 e.
Magnetic moment[edit]
Even though the neutron is a neutral particle, the magnetic moment of a neutron is not zero. The neutron is not affected by electric fields, but it is affected by magnetic fields. The value for the neutron’s magnetic moment was first directly measured by Luis Alvarez and Felix Bloch at Berkeley, California, in 1940.[56] Alvarez and Bloch determined the magnetic moment of the neutron to be μn= −1.93(2) μN, where μN is the nuclear magneton.
The magnetic moment of the neutron is an indication of its quark substructure and internal charge distribution.[57] In the quark model for hadrons, the neutron is composed of one up quark (charge +2/3 e) and two down quarks (charge −1/3 e).[57] The magnetic moment of the neutron can be modeled as a sum of the magnetic moments of the constituent quarks.[58] The calculation assumes that the quarks behave like pointlike Dirac particles, each having their own magnetic moment. Simplistically, the magnetic moment of the neutron can be viewed as resulting from the vector sum of the three quark magnetic moments, plus the orbital magnetic moments caused by the movement of the three charged quarks within the neutron.
In one of the early successes of the Standard Model in 1964 Mirza A.B. Beg, Benjamin W. Lee, and Abraham Pais theoretically calculated the ratio of proton to neutron magnetic moments to be −3/2, which agrees with the experimental value to within 3%.[59][60][61] The measured value for this ratio is −1.45989805(34).[4]
The above treatment compares neutrons with protons, allowing the complex behavior of quarks to be subtracted out between models, and merely exploring what the effects would be of differing quark charges (or quark type). Such calculations are enough to show that the interior of neutrons is very much like that of protons, save for the difference in quark composition with a down quark in the neutron replacing an up quark in the proton.
The neutron magnetic moment can be roughly computed by assuming a simple nonrelativistic, quantum mechanical wavefunction for baryons composed of three quarks. A straightforward calculation gives fairly accurate estimates for the magnetic moments of neutrons, protons, and other baryons.[58] For a neutron, the result of this calculation is that the magnetic moment of the neutron is given by μn= 4/3 μd − 1/3 μu, where μd and μu are the magnetic moments for the down and up quarks, respectively. This result combines the intrinsic magnetic moments of the quarks with their orbital magnetic moments, and assumes the three quarks are in a particular, dominant quantum state.
| Baryon | Magnetic moment of quark model |
Computed (  ) )
|
Observed (  ) )
|
|---|---|---|---|
| p | 4/3 μu − 1/3 μd | 2.79 | 2.793 |
| n | 4/3 μd − 1/3 μu | −1.86 | −1.913 |
The results of this calculation are encouraging, but the masses of the up or down quarks were assumed to be 1/3 the mass of a nucleon.[58] The masses of the quarks are actually only about 1% that of a nucleon.[62] The discrepancy stems from the complexity of the Standard Model for nucleons, where most of their mass originates in the gluon fields, virtual particles, and their associated energy that are essential aspects of the strong force.[62][63] Furthermore, the complex system of quarks and gluons that constitute a neutron requires a relativistic treatment.[64] But the nucleon magnetic moment has been successfully computed numerically from first principles, including all of the effects mentioned and using more realistic values for the quark masses. The calculation gave results that were in fair agreement with measurement, but it required significant computing resources.[65][66]
Spin[edit]
The neutron is a spin 1/2 particle, that is, it is a fermion with intrinsic angular momentum equal to 1/2 ħ, where ħ is the reduced Planck constant. For many years after the discovery of the neutron, its exact spin was ambiguous. Although it was assumed to be a spin 1/2 Dirac particle, the possibility that the neutron was a spin 3/2 particle lingered. The interactions of the neutron’s magnetic moment with an external magnetic field were exploited to finally determine the spin of the neutron.[67] In 1949, Hughes and Burgy measured neutrons reflected from a ferromagnetic mirror and found that the angular distribution of the reflections was consistent with spin 1/2.[68] In 1954, Sherwood, Stephenson, and Bernstein employed neutrons in a Stern–Gerlach experiment that used a magnetic field to separate the neutron spin states. They recorded two such spin states, consistent with a spin 1/2 particle.[67][69]
As a fermion, the neutron is subject to the Pauli exclusion principle; two neutrons cannot have the same quantum numbers. This is the source of the degeneracy pressure which makes neutron stars possible.
Structure and geometry of charge distribution[edit]
An article published in 2007 featuring a model-independent analysis concluded that the neutron has a negatively charged exterior, a positively charged middle, and a negative core.[70] In a simplified classical view, the negative «skin» of the neutron assists it to be attracted to the protons with which it interacts in the nucleus; but the main attraction between neutrons and protons is via the nuclear force, which does not involve electric charge.
The simplified classical view of the neutron’s charge distribution also «explains» the fact that the neutron magnetic dipole points in the opposite direction from its spin angular momentum vector (as compared to the proton). This gives the neutron, in effect, a magnetic moment which resembles a negatively charged particle. This can be reconciled classically with a neutral neutron composed of a charge distribution in which the negative sub-parts of the neutron have a larger average radius of distribution, and therefore contribute more to the particle’s magnetic dipole moment, than do the positive parts that are, on average, nearer the core.
Electric dipole moment[edit]
The Standard Model of particle physics predicts a tiny separation of positive and negative charge within the neutron leading to a permanent electric dipole moment.[71] But the predicted value is well below the current sensitivity of experiments. From several unsolved puzzles in particle physics, it is clear that the Standard Model is not the final and full description of all particles and their interactions. New theories going beyond the Standard Model generally lead to much larger predictions for the electric dipole moment of the neutron. Currently, there are at least four experiments trying to measure for the first time a finite neutron electric dipole moment, including:
- Cryogenic neutron EDM experiment being set up at the Institut Laue–Langevin[72]
- nEDM experiment under construction at the new UCN source at the Paul Scherrer Institute[73]
- nEDM experiment being envisaged at the Spallation Neutron Source[74][75]
- nEDM experiment being built at the Institut Laue–Langevin[76]
Antineutron[edit]
The antineutron is the antiparticle of the neutron. It was discovered by Bruce Cork in 1956, a year after the antiproton was discovered. CPT-symmetry puts strong constraints on the relative properties of particles and antiparticles, so studying antineutrons provides stringent tests on CPT-symmetry. The fractional difference in the masses of the neutron and antineutron is (9±6)×10−5. Since the difference is only about two standard deviations away from zero, this does not give any convincing evidence of CPT-violation.[11]
Neutron compounds[edit]
Dineutrons and tetraneutrons[edit]
The existence of stable clusters of 4 neutrons, or tetraneutrons, has been hypothesised by a team led by Francisco-Miguel Marqués at the CNRS Laboratory for Nuclear Physics based on observations of the disintegration of beryllium-14 nuclei. This is particularly interesting because current theory suggests that these clusters should not be stable.
In February 2016, Japanese physicist Susumu Shimoura of the University of Tokyo and co-workers reported they had observed the purported tetraneutrons for the first time experimentally.[77] Nuclear physicists around the world say this discovery, if confirmed, would be a milestone in the field of nuclear physics and certainly would deepen our understanding of the nuclear forces.[78][79]
The dineutron is another hypothetical particle. In 2012, Artemis Spyrou from Michigan State University and coworkers reported that they observed, for the first time, the dineutron emission in the decay of 16Be. The dineutron character is evidenced by a small emission angle between the two neutrons. The authors measured the two-neutron separation energy to be 1.35(10) MeV, in good agreement with shell model calculations, using standard interactions for this mass region.[80]
Neutronium and neutron stars[edit]
At extremely high pressures and temperatures, nucleons and electrons are believed to collapse into bulk neutronic matter, called neutronium. This is presumed to happen in neutron stars.
The extreme pressure inside a neutron star may deform the neutrons into a cubic symmetry, allowing tighter packing of neutrons.[81]
Detection[edit]
The common means of detecting a charged particle by looking for a track of ionization (such as in a cloud chamber) does not work for neutrons directly. Neutrons that elastically scatter off atoms can create an ionization track that is detectable, but the experiments are not as simple to carry out; other means for detecting neutrons, consisting of allowing them to interact with atomic nuclei, are more commonly used. The commonly used methods to detect neutrons can therefore be categorized according to the nuclear processes relied upon, mainly neutron capture or elastic scattering.[82]
Neutron detection by neutron capture[edit]
A common method for detecting neutrons involves converting the energy released from neutron capture reactions into electrical signals. Certain nuclides have a high neutron capture cross section, which is the probability of absorbing a neutron. Upon neutron capture, the compound nucleus emits more easily detectable radiation, for example an alpha particle, which is then detected. The nuclides 3
He
, 6
Li
, 10
B
, 233
U
, 235
U
, 237
Np
, and 239
Pu
are useful for this purpose.
Neutron detection by elastic scattering[edit]
Neutrons can elastically scatter off nuclei, causing the struck nucleus to recoil. Kinematically, a neutron can transfer more energy to a light nucleus such as hydrogen or helium than to a heavier nucleus. Detectors relying on elastic scattering are called fast neutron detectors. Recoiling nuclei can ionize and excite further atoms through collisions. Charge and/or scintillation light produced in this way can be collected to produce a detected signal. A major challenge in fast neutron detection is discerning such signals from erroneous signals produced by gamma radiation in the same detector. Methods such as pulse shape discrimination can be used in distinguishing neutron signals from gamma-ray signals, although certain inorganic scintillator-based detectors have been developed [83][84] to selectively detect neutrons in mixed radiation fields inherently without any additional techniques.
Fast neutron detectors have the advantage of not requiring a moderator, and are therefore capable of measuring the neutron’s energy, time of arrival, and in certain cases direction of incidence.
Sources and production[edit]
Free neutrons are unstable, although they have the longest half-life of any unstable subatomic particle by several orders of magnitude. Their half-life is still only about 10 minutes, so they can be obtained only from sources that produce them continuously.
Natural neutron background. A small natural background flux of free neutrons exists everywhere on Earth. In the atmosphere and deep into the ocean, the «neutron background» is caused by muons produced by cosmic ray interaction with the atmosphere. These high-energy muons are capable of penetration to considerable depths in water and soil. There, in striking atomic nuclei, among other reactions they induce spallation reactions in which a neutron is liberated from the nucleus. Within the Earth’s crust a second source is neutrons produced primarily by spontaneous fission of uranium and thorium present in crustal minerals. The neutron background is not strong enough to be a biological hazard, but it is of importance to very high resolution particle detectors that are looking for very rare events, such as (hypothesized) interactions that might be caused by particles of dark matter.[12] Recent research has shown that even thunderstorms can produce neutrons with energies of up to several tens of MeV.[85] Recent research has shown that the fluence of these neutrons lies between 10−9 and 10−13 per ms and per m2 depending on the detection altitude. The energy of most of these neutrons, even with initial energies of 20 MeV, decreases down to the keV range within 1 ms.[86]
Even stronger neutron background radiation is produced at the surface of Mars, where the atmosphere is thick enough to generate neutrons from cosmic ray muon production and neutron-spallation, but not thick enough to provide significant protection from the neutrons produced. These neutrons not only produce a Martian surface neutron radiation hazard from direct downward-going neutron radiation but may also produce a significant hazard from reflection of neutrons from the Martian surface, which will produce reflected neutron radiation penetrating upward into a Martian craft or habitat from the floor.[87]
Sources of neutrons for research. These include certain types of radioactive decay (spontaneous fission and neutron emission), and from certain nuclear reactions. Convenient nuclear reactions include tabletop reactions such as natural alpha and gamma bombardment of certain nuclides, often beryllium or deuterium, and induced nuclear fission, such as occurs in nuclear reactors. In addition, high-energy nuclear reactions (such as occur in cosmic radiation showers or accelerator collisions) also produce neutrons from disintegration of target nuclei. Small (tabletop) particle accelerators optimized to produce free neutrons in this way, are called neutron generators.
In practice, the most commonly used small laboratory sources of neutrons use radioactive decay to power neutron production. One noted neutron-producing radioisotope, californium-252 decays (half-life 2.65 years) by spontaneous fission 3% of the time with production of 3.7 neutrons per fission, and is used alone as a neutron source from this process. Nuclear reaction sources (that involve two materials) powered by radioisotopes use an alpha decay source plus a beryllium target, or else a source of high-energy gamma radiation from a source that undergoes beta decay followed by gamma decay, which produces photoneutrons on interaction of the high-energy gamma ray with ordinary stable beryllium, or else with the deuterium in heavy water. A popular source of the latter type is radioactive antimony-124 plus beryllium, a system with a half-life of 60.9 days, which can be constructed from natural antimony (which is 42.8% stable antimony-123) by activating it with neutrons in a nuclear reactor, then transported to where the neutron source is needed.[88]
Nuclear fission reactors naturally produce free neutrons; their role is to sustain the energy-producing chain reaction. The intense neutron radiation can also be used to produce various radioisotopes through the process of neutron activation, which is a type of neutron capture.
Experimental nuclear fusion reactors produce free neutrons as a waste product. But it is these neutrons that possess most of the energy, and converting that energy to a useful form has proved a difficult engineering challenge. Fusion reactors that generate neutrons are likely to create radioactive waste, but the waste is composed of neutron-activated lighter isotopes, which have relatively short (50–100 years) decay periods as compared to typical half-lives of 10,000 years[89] for fission waste, which is long due primarily to the long half-life of alpha-emitting transuranic actinides.[90] Some nuclear fusion-fission hybrids are proposed to make use of those neutrons to either maintain a subcritical reactor or to aid in nuclear transmutation of harmful long lived nuclear waste to shorter lived or stable nuclides.
Neutron beams and modification of beams after production[edit]
Free neutron beams are obtained from neutron sources by neutron transport. For access to intense neutron sources, researchers must go to a specialized neutron facility that operates a research reactor or a spallation source.
The neutron’s lack of total electric charge makes it difficult to steer or accelerate them. Charged particles can be accelerated, decelerated, or deflected by electric or magnetic fields. These methods have little effect on neutrons. But some effects may be attained by use of inhomogeneous magnetic fields because of the neutron’s magnetic moment. Neutrons can be controlled by methods that include moderation, reflection, and velocity selection. Thermal neutrons can be polarized by transmission through magnetic materials in a method analogous to the Faraday effect for photons. Cold neutrons of wavelengths of 6–7 angstroms can be produced in beams of a high degree of polarization, by use of magnetic mirrors and magnetized interference filters.[91]
Applications[edit]
The neutron plays an important role in many nuclear reactions. For example, neutron capture often results in neutron activation, inducing radioactivity. In particular, knowledge of neutrons and their behavior has been important in the development of nuclear reactors and nuclear weapons. The fissioning of elements like uranium-235 and plutonium-239 is caused by their absorption of neutrons.
Cold, thermal, and hot neutron radiation is commonly employed in neutron scattering facilities, where the radiation is used in a similar way one uses X-rays for the analysis of condensed matter. Neutrons are complementary to the latter in terms of atomic contrasts by different scattering cross sections; sensitivity to magnetism; energy range for inelastic neutron spectroscopy; and deep penetration into matter.
The development of «neutron lenses» based on total internal reflection within hollow glass capillary tubes or by reflection from dimpled aluminum plates has driven ongoing research into neutron microscopy and neutron/gamma ray tomography.[92][93][94][95]
A major use of neutrons is to excite delayed and prompt gamma rays from elements in materials. This forms the basis of neutron activation analysis (NAA) and prompt gamma neutron activation analysis (PGNAA). NAA is most often used to analyze small samples of materials in a nuclear reactor whilst PGNAA is most often used to analyze subterranean rocks around bore holes and industrial bulk materials on conveyor belts.
Another use of neutron emitters is the detection of light nuclei, in particular the hydrogen found in water molecules. When a fast neutron collides with a light nucleus, it loses a large fraction of its energy. By measuring the rate at which slow neutrons return to the probe after reflecting off of hydrogen nuclei, a neutron probe may determine the water content in soil.
Medical therapies[edit]
Because neutron radiation is both penetrating and ionizing, it can be exploited for medical treatments. However, neutron radiation can have the unfortunate side-effect of leaving the affected area radioactive. Neutron tomography is therefore not a viable medical application.
Fast neutron therapy uses high-energy neutrons typically greater than 20 MeV to treat cancer. Radiation therapy of cancers is based upon the biological response of cells to ionizing radiation. If radiation is delivered in small sessions to damage cancerous areas, normal tissue will have time to repair itself, while tumor cells often cannot.[96] Neutron radiation can deliver energy to a cancerous region at a rate an order of magnitude larger than gamma radiation.[97]
Beams of low-energy neutrons are used in boron neutron capture therapy to treat cancer. In boron neutron capture therapy, the patient is given a drug that contains boron and that preferentially accumulates in the tumor to be targeted. The tumor is then bombarded with very low-energy neutrons (although often higher than thermal energy) which are captured by the boron-10 isotope in the boron, which produces an excited state of boron-11 that then decays to produce lithium-7 and an alpha particle that have sufficient energy to kill the malignant cell, but insufficient range to damage nearby cells. For such a therapy to be applied to the treatment of cancer, a neutron source having an intensity of the order of a thousand million (109) neutrons per second per cm2 is preferred. Such fluxes require a research nuclear reactor.
Protection[edit]
Exposure to free neutrons can be hazardous, since the interaction of neutrons with molecules in the body can cause disruption to molecules and atoms, and can also cause reactions that give rise to other forms of radiation (such as protons). The normal precautions of radiation protection apply: Avoid exposure, stay as far from the source as possible, and keep exposure time to a minimum. But particular thought must be given to how to protect from neutron exposure. For other types of radiation, e.g., alpha particles, beta particles, or gamma rays, material of a high atomic number and with high density makes for good shielding; frequently, lead is used. However, this approach will not work with neutrons, since the absorption of neutrons does not increase straightforwardly with atomic number, as it does with alpha, beta, and gamma radiation. Instead one needs to look at the particular interactions neutrons have with matter (see the section on detection above). For example, hydrogen-rich materials are often used to shield against neutrons, since ordinary hydrogen both scatters and slows neutrons. This often means that simple concrete blocks or even paraffin-loaded plastic blocks afford better protection from neutrons than do far more dense materials. After slowing, neutrons may then be absorbed with an isotope that has high affinity for slow neutrons without causing secondary capture radiation, such as lithium-6.
Hydrogen-rich ordinary water affects neutron absorption in nuclear fission reactors: Usually, neutrons are so strongly absorbed by normal water that fuel enrichment with fissionable isotope is required.[clarification needed] The deuterium in heavy water has a very much lower absorption affinity for neutrons than does protium (normal light hydrogen). Deuterium is, therefore, used in CANDU-type reactors, in order to slow (moderate) neutron velocity, to increase the probability of nuclear fission compared to neutron capture.
Neutron temperature[edit]
Thermal neutrons[edit]
Thermal neutrons are free neutrons whose energies have a Maxwell–Boltzmann distribution with kT = 0.0253 eV (4.0×10−21 J) at room temperature. This gives characteristic (not average, or median) speed of 2.2 km/s. The name ‘thermal’ comes from their energy being that of the room temperature gas or material they are permeating. (see kinetic theory for energies and speeds of molecules). After a number of collisions (often in the range of 10–20) with nuclei, neutrons arrive at this energy level, provided that they are not absorbed.
In many substances, thermal neutron reactions show a much larger effective cross-section than reactions involving faster neutrons, and thermal neutrons can therefore be absorbed more readily (i.e., with higher probability) by any atomic nuclei that they collide with, creating a heavier – and often unstable – isotope of the chemical element as a result.
Most fission reactors use a neutron moderator to slow down, or thermalize the neutrons that are emitted by nuclear fission so that they are more easily captured, causing further fission. Others, called fast breeder reactors, use fission energy neutrons directly.
Cold neutrons[edit]
Cold neutrons are thermal neutrons that have been equilibrated in a very cold substance such as liquid deuterium. Such a cold source is placed in the moderator of a research reactor or spallation source. Cold neutrons are particularly valuable for neutron scattering experiments.[98]
The use of cold and very cold neutrons (VCN) have been a bit limited compared to the use of thermal neutrons due to the relatively lower flux and lack in optical components. However, Innovative solutions have been proposed to offer more options to the scientific community to promote the use of VCN.[99][100]
Cold neutron source providing neutrons at about the temperature of liquid hydrogen
Ultracold neutrons[edit]
Ultracold neutrons are produced by inelastic scattering of cold neutrons in substances with a low neutron absorption cross section at a temperature of a few kelvins, such as solid deuterium[101] or superfluid helium.[102] An alternative production method is the mechanical deceleration of cold neutrons exploiting the Doppler shift.[103][104]
Fission energy neutrons[edit]
A fast neutron is a free neutron with a kinetic energy level close to 1 MeV (1.6×10−13 J), hence a speed of ~14000 km/s (~ 5% of the speed of light). They are named fission energy or fast neutrons to distinguish them from lower-energy thermal neutrons, and high-energy neutrons produced in cosmic showers or accelerators. Fast neutrons are produced by nuclear processes such as nuclear fission. Neutrons produced in fission, as noted above, have a Maxwell–Boltzmann distribution of kinetic energies from 0 to ~14 MeV, a mean energy of 2 MeV (for 235U fission neutrons), and a mode of only 0.75 MeV, which means that more than half of them do not qualify as fast (and thus have almost no chance of initiating fission in fertile materials, such as 238U and 232Th).
Fast neutrons can be made into thermal neutrons via a process called moderation. This is done with a neutron moderator. In reactors, typically heavy water, light water, or graphite are used to moderate neutrons.
Fusion neutrons[edit]
The fusion reaction rate increases rapidly with temperature until it maximizes and then gradually drops off. The D–T rate peaks at a lower temperature (about 70 keV, or 800 million kelvins) and at a higher value than other reactions commonly considered for fusion energy.
D–T (deuterium–tritium) fusion is the fusion reaction that produces the most energetic neutrons, with 14.1 MeV of kinetic energy and traveling at 17% of the speed of light. D–T fusion is also the easiest fusion reaction to ignite, reaching near-peak rates even when the deuterium and tritium nuclei have only a thousandth as much kinetic energy as the 14.1 MeV that will be produced.
14.1 MeV neutrons have about 10 times as much energy as fission neutrons, and are very effective at fissioning even non-fissile heavy nuclei, and these high-energy fissions produce more neutrons on average than fissions by lower-energy neutrons. This makes D–T fusion neutron sources such as proposed tokamak power reactors useful for transmutation of transuranic waste. 14.1 MeV neutrons can also produce neutrons by knocking them loose from nuclei.
On the other hand, these very high-energy neutrons are less likely to simply be captured without causing fission or spallation. For these reasons, nuclear weapon design extensively uses D–T fusion 14.1 MeV neutrons to cause more fission. Fusion neutrons are able to cause fission in ordinarily non-fissile materials, such as depleted uranium (uranium-238), and these materials have been used in the jackets of thermonuclear weapons. Fusion neutrons also can cause fission in substances that are unsuitable or difficult to make into primary fission bombs, such as reactor grade plutonium. This physical fact thus causes ordinary non-weapons grade materials to become of concern in certain nuclear proliferation discussions and treaties.
Other fusion reactions produce much less energetic neutrons. D–D fusion produces a 2.45 MeV neutron and helium-3 half of the time, and produces tritium and a proton but no neutron the rest of the time. D–3He fusion produces no neutron.
Intermediate-energy neutrons[edit]
A fission energy neutron that has slowed down but not yet reached thermal energies is called an epithermal neutron.
Cross sections for both capture and fission reactions often have multiple resonance peaks at specific energies in the epithermal energy range. These are of less significance in a fast-neutron reactor, where most neutrons are absorbed before slowing down to this range, or in a well-moderated thermal reactor, where epithermal neutrons interact mostly with moderator nuclei, not with either fissile or fertile actinide nuclides. But in a partially moderated reactor with more interactions of epithermal neutrons with heavy metal nuclei, there are greater possibilities for transient changes in reactivity that might make reactor control more difficult.
Ratios of capture reactions to fission reactions are also worse (more captures without fission) in most nuclear fuels such as plutonium-239, making epithermal-spectrum reactors using these fuels less desirable, as captures not only waste the one neutron captured but also usually result in a nuclide that is not fissile with thermal or epithermal neutrons, though still fissionable with fast neutrons. The exception is uranium-233 of the thorium cycle, which has good capture-fission ratios at all neutron energies.
High-energy neutrons[edit]
High-energy neutrons have much more energy than fission energy neutrons and are generated as secondary particles by particle accelerators or in the atmosphere from cosmic rays. These high-energy neutrons are extremely efficient at ionization and far more likely to cause cell death than X-rays or protons.[105][106]
See also[edit]
Wikimedia Commons has media related to Neutrons.
- Ionizing radiation
- Isotope
- List of particles
- Neutron radiation and the Sievert radiation scale
- Neutronium
- Nuclear reaction
- Nucleosynthesis
- Neutron capture nucleosynthesis
- R-process
- S-process
- Thermal reactor
Neutron sources[edit]
- Neutron generator
- Neutron source
Processes involving neutrons[edit]
- Neutron bomb
- Neutron diffraction
- Neutron flux
- Neutron transport
- Cosmogenic radionuclide dating
References[edit]
- ^ Ernest Rutherford Archived 2011-08-03 at the Wayback Machine. Chemed.chem.purdue.edu. Retrieved on 2012-08-16.
- ^ a b 1935 Nobel Prize in Physics Archived 2017-10-03 at the Wayback Machine. Nobelprize.org. Retrieved on 2012-08-16.
- ^ a b «2018 CODATA recommended values» https://physics.nist.gov/cuu/Constants/index.html Archived 2018-01-22 at the Wayback Machine
- ^ a b c d e f Mohr, P.J.; Taylor, B.N. and Newell, D.B. (2014), «The 2014 CODATA Recommended Values of the Fundamental Physical Constants» Archived 2013-10-09 at the Wayback Machine (Web Version 7.0). The database was developed by J. Baker, M. Douma, and S. Kotochigova. (2014). National Institute of Standards and Technology, Gaithersburg, Maryland 20899.
- ^ a b Zyla, P. A. (2020). «n MEAN LIFE». PDG Live: 2020 Review of Particle Physics. Particle Data Group. Archived from the original on 17 January 2021. Retrieved 25 February 2021.
- ^ a b Olive, K.A.; (Particle Data Group); et al. (2014). «Review of Particle Physics» (PDF). Chinese Physics C. 38 (9): 1–708. arXiv:1412.1408. Bibcode:2014ChPhC..38i0001O. doi:10.1088/1674-1137/38/9/090001. PMID 10020536. S2CID 118395784. Archived (PDF) from the original on 2020-06-01. Retrieved 2017-10-26.
- ^ Thomas, A.W.; Weise, W. (2001), The Structure of the Nucleon, Wiley-WCH, Berlin, ISBN 978-3-527-40297-7
- ^ a b
Chadwick, James (1932). «Possible Existence of a Neutron». Nature. 129 (3252): 312. Bibcode:1932Natur.129Q.312C. doi:10.1038/129312a0. S2CID 4076465. - ^ Hahn, O. & Strassmann, F. (1939). «Über den Nachweis und das Verhalten der bei der Bestrahlung des Urans mittels Neutronen entstehenden Erdalkalimetalle» [On the detection and characteristics of the alkaline earth metals formed by irradiation of uranium with neutrons]. Die Naturwissenschaften. 27 (1): 11–15. Bibcode:1939NW…..27…11H. doi:10.1007/BF01488241. S2CID 5920336.
- ^ a b c Glasstone, Samuel; Dolan, Philip J., eds. (1977), The Effects of Nuclear Weapons (3rd ed.), U.S. Dept. of Defense and Energy Research and Development Administration, U.S. Government Printing Office, ISBN 978-1-60322-016-3
- ^ a b c Nakamura, K (2010). «Review of Particle Physics». Journal of Physics G. 37 (7A): 1–708. Bibcode:2010JPhG…37g5021N. doi:10.1088/0954-3899/37/7A/075021. PMID 10020536. PDF with 2011 partial update for the 2012 edition Archived 2012-09-20 at the Wayback Machine.
The exact value of the mean lifetime is still uncertain, due to conflicting results from experiments.
The Particle Data Group reports values up to six seconds apart (more than four standard deviations), commenting that «our 2006, 2008, and 2010 Reviews stayed with 885.7±0.8 s; but we noted that in light of SEREBROV 05 our value should be regarded as suspect until further experiments clarified matters. Since our 2010 Review, PICHLMAIER 10 has obtained a mean life of 880.7±1.8 s, closer to the value of SEREBROV 05 than to our average. And SEREBROV 10B[…] claims their values should be lowered by about 6 s, which would bring them into line with the two lower values. But those re-evaluations have not received an enthusiastic response from the experimenters in question; and in any case the Particle Data Group would have to await published changes (by those experimenters) of published values.At this point, we can think of nothing better to do than to average the seven best but discordant measurements, getting 881.5±1.5 s. Note that the error includes a scale factor of 2.7. This is a jump of 4.2 old (and 2.8 new) standard deviations. This state of affairs is a particularly unhappy one, because the value is so important. We again call upon the experimenters to clear this up.»
- ^ a b
Carson, M.J.; et al. (2004). «Neutron background in large-scale xenon detectors for dark matter searches». Astroparticle Physics. 21 (6): 667–687. arXiv:hep-ex/0404042. Bibcode:2004APh….21..667C. doi:10.1016/j.astropartphys.2004.05.001. S2CID 17887096. - ^ Nudat 2 Archived 2009-08-17 at the Wayback Machine. Nndc.bnl.gov. Retrieved on 2010-12-04.
- ^ Povh, B.; Rith, K.; Scholz, C.; Zetsche, F. (2002). Particles and Nuclei: An Introduction to the Physical Concepts. Berlin: Springer-Verlag. p. 73. ISBN 978-3-540-43823-6.
- ^
Basdevant, J.-L.; Rich, J.; Spiro, M. (2005). Fundamentals in Nuclear Physics. Springer. p. 155. ISBN 978-0-387-01672-6. - ^ Tipler, Paul Allen; Llewellyn, Ralph A. (2002). Modern Physics (4 ed.). Macmillan. p. 310. ISBN 978-0-7167-4345-3. Archived from the original on 2022-04-07. Retrieved 2020-08-27.
- ^
Adair, R.K. (1989). The Great Design: Particles, Fields, and Creation. Oxford University Press. p. 214. Bibcode:1988gdpf.book…..A. - ^
Cottingham, W.N.; Greenwood, D.A. (1986). An Introduction to Nuclear Physics. Cambridge University Press. ISBN 9780521657334. - ^ a b
Rutherford, E. (1920). «Nuclear Constitution of Atoms». Proceedings of the Royal Society A. 97 (686): 374–400. Bibcode:1920RSPSA..97..374R. doi:10.1098/rspa.1920.0040. - ^ Harkins, William (1921). «The constitution and stability of atomic nuclei. (A contribution to the subject of inorganic evolution.)». Philos. Mag. 42 (249): 305. doi:10.1080/14786442108633770.
- ^ a b Feather, N. (1960). «A history of neutrons and nuclei. Part 1». Contemporary Physics. 1 (3): 191–203. Bibcode:1960ConPh…1..191F. doi:10.1080/00107516008202611.
- ^ Pauli, Wolfgang; Hermann, A.; Meyenn, K.v; Weisskopff, V.F (1985). «Das Jahr 1932 Die Entdeckung des Neutrons». Wolfgang Pauli. Sources in the History of Mathematics and Physical Sciences. Vol. 6. pp. 105–144. doi:10.1007/978-3-540-78801-0_3. ISBN 978-3-540-13609-5.
- ^ Hendry, John, ed. (1984). Cambridge Physics in the Thirties. Bristol: Adam Hilger. ISBN 978-0852747612.
- ^ Brown, Laurie M. (1978). «The idea of the neutrino». Physics Today. 31 (9): 23–28. Bibcode:1978PhT….31i..23B. doi:10.1063/1.2995181.
- ^ Friedlander G., Kennedy J.W. and Miller J.M. (1964) Nuclear and Radiochemistry (2nd edition), Wiley, pp. 22–23 and 38–39
- ^ a b Stuewer, Roger H. (1985). «Niels Bohr and Nuclear Physics». In French, A.P.; Kennedy, P.J. (eds.). Niels Bohr: A Centenary Volume. Harvard University Press. pp. 197–220. ISBN 978-0674624160.
- ^ Pais, Abraham (1986). Inward Bound. Oxford: Oxford University Press. p. 299. ISBN 978-0198519973.
- ^ Klein, O. (1929). «Die Reflexion von Elektronen an einem Potentialsprung nach der relativistischen Dynamik von Dirac». Zeitschrift für Physik. 53 (3–4): 157–165. Bibcode:1929ZPhy…53..157K. doi:10.1007/BF01339716. S2CID 121771000.
- ^ Bothe, W.; Becker, H. (1930). «Künstliche Erregung von Kern-γ-Strahlen» [Artificial excitation of nuclear γ-radiation]. Zeitschrift für Physik. 66 (5–6): 289–306. Bibcode:1930ZPhy…66..289B. doi:10.1007/BF01390908. S2CID 122888356.
- ^ Becker, H.; Bothe, W. (1932). «Die in Bor und Beryllium erregten γ-Strahlen» [Γ-rays excited in boron and beryllium]. Zeitschrift für Physik. 76 (7–8): 421–438. Bibcode:1932ZPhy…76..421B. doi:10.1007/BF01336726. S2CID 121188471.
- ^ Joliot-Curie, Irène & Joliot, Frédéric (1932). «Émission de protons de grande vitesse par les substances hydrogénées sous l’influence des rayons γ très pénétrants» [Emission of high-speed protons by hydrogenated substances under the influence of very penetrating γ-rays]. Comptes Rendus. 194: 273. Archived from the original on 2022-03-04. Retrieved 2012-06-16.
- ^ Brown, Andrew (1997). The Neutron and the Bomb: A Biography of Sir James Chadwick. Oxford University Press. ISBN 978-0-19-853992-6.
- ^ «Atop the Physics Wave: Rutherford Back in Cambridge, 1919–1937». Rutherford’s Nuclear World. American Institute of Physics. 2011–2014. Archived from the original on 21 October 2014. Retrieved 19 August 2014.
- ^ Chadwick, J. (1933). «Bakerian Lecture. The Neutron». Proceedings of the Royal Society A. 142 (846): 1–25. Bibcode:1933RSPSA.142….1C. doi:10.1098/rspa.1933.0152.
- ^ Heisenberg, W. (1932). «Über den Bau der Atomkerne. I». Zeitschrift für Physik. 77 (1–2): 1–11. Bibcode:1932ZPhy…77….1H. doi:10.1007/BF01342433. S2CID 186218053.
- ^ Heisenberg, W. (1932). «Über den Bau der Atomkerne. II». Zeitschrift für Physik. 78 (3–4): 156–164. Bibcode:1932ZPhy…78..156H. doi:10.1007/BF01337585. S2CID 186221789.
- ^ Heisenberg, W. (1933). «Über den Bau der Atomkerne. III». Zeitschrift für Physik. 80 (9–10): 587–596. Bibcode:1933ZPhy…80..587H. doi:10.1007/BF01335696. S2CID 126422047.
- ^ Iwanenko, D. (1932). «The Neutron Hypothesis». Nature. 129 (3265): 798. Bibcode:1932Natur.129..798I. doi:10.1038/129798d0. S2CID 4096734.
- ^ Miller A.I. (1995) Early Quantum Electrodynamics: A Sourcebook, Cambridge University Press, Cambridge, ISBN 0521568919, pp. 84–88.
- ^ Wilson, Fred L. (1968). «Fermi’s Theory of Beta Decay». American Journal of Physics. 36 (12): 1150–1160. Bibcode:1968AmJPh..36.1150W. doi:10.1119/1.1974382.
- ^ Chadwick, J.; Goldhaber, M. (1934). «A nuclear photo-effect: disintegration of the diplon by gamma rays». Nature. 134 (3381): 237–238. Bibcode:1934Natur.134..237C. doi:10.1038/134237a0. S2CID 4137231.
- ^ Chadwick, J.; Goldhaber, M. (1935). «A nuclear photoelectric effect». Proceedings of the Royal Society of London A. 151 (873): 479–493. Bibcode:1935RSPSA.151..479C. doi:10.1098/rspa.1935.0162.
- ^ Cooper, Dan (1999). Enrico Fermi: And the Revolutions in Modern physics. New York: Oxford University Press. ISBN 978-0-19-511762-2. OCLC 39508200.
- ^ Hahn, O. (1958). «The Discovery of Fission». Scientific American. 198 (2): 76–84. Bibcode:1958SciAm.198b..76H. doi:10.1038/scientificamerican0258-76.
- ^ Rife, Patricia (1999). Lise Meitner and the dawn of the nuclear age. Basel, Switzerland: Birkhäuser. ISBN 978-0-8176-3732-3.
- ^ Hahn, O.; Strassmann, F. (10 February 1939). «Proof of the Formation of Active Isotopes of Barium from Uranium and Thorium Irradiated with Neutrons; Proof of the Existence of More Active Fragments Produced by Uranium Fission». Die Naturwissenschaften. 27 (6): 89–95. Bibcode:1939NW…..27…89H. doi:10.1007/BF01488988. S2CID 33512939.
- ^ «The Nobel Prize in Chemistry 1944». Nobel Foundation. Archived from the original on 2018-12-26. Retrieved 2007-12-17.
- ^ Bernstein, Jeremy (2001). Hitler’s uranium club: the secret recordings at Farm Hall. New York: Copernicus. p. 281. ISBN 978-0-387-95089-1.
- ^ «The Nobel Prize in Chemistry 1944: Presentation Speech». Nobel Foundation. Archived from the original on 2007-10-25. Retrieved 2008-01-03.
- ^ Sir James Chadwick’s Discovery of Neutrons Archived 2011-10-26 at the Wayback Machine. ANS Nuclear Cafe. Retrieved on 2012-08-16.
- ^ Particle Data Group Summary Data Table on Baryons Archived 2011-09-10 at the Wayback Machine. lbl.gov (2007). Retrieved on 2012-08-16.
- ^ Tanabashi, M. (2018). «The Review of Particle Physics». Physical Review D. 54 (1): 1653. doi:10.1103/physrevd.54.1. PMID 10020536. Archived from the original on 2020-10-19. Retrieved 2020-10-14.
- ^ Basic Ideas and Concepts in Nuclear Physics: An Introductory Approach, Third Edition; K. Heyde Taylor & Francis 2004. Print ISBN 978-0-7503-0980-6, 978-1-4200-5494-1. doi:10.1201/9781420054941. full text[permanent dead link]
- ^ Greene, GL; et al. (1986). «New determination of the deuteron binding energy and the neutron mass». Physical Review Letters. 56 (8): 819–822. Bibcode:1986PhRvL..56..819G. doi:10.1103/PhysRevLett.56.819. PMID 10033294.
- ^ Byrne, J. Neutrons, Nuclei, and Matter, Dover Publications, Mineola, New York, 2011, ISBN 0486482383, pp. 18–19
- ^ Alvarez, L.W; Bloch, F. (1940). «A quantitative determination of the neutron magnetic moment in absolute nuclear magnetons». Physical Review. 57 (2): 111–122. Bibcode:1940PhRv…57..111A. doi:10.1103/physrev.57.111.
- ^ a b Gell, Y.; Lichtenberg, D.B. (1969). «Quark model and the magnetic moments of proton and neutron». Il Nuovo Cimento A. Series 10. 61 (1): 27–40. Bibcode:1969NCimA..61…27G. doi:10.1007/BF02760010. S2CID 123822660.
- ^ a b c Perkins, Donald H. (1982). Introduction to High Energy Physics. Addison Wesley, Reading, Massachusetts. pp. 201–202. ISBN 978-0-201-05757-7.
- ^
Greenberg, O.W. (2009), «Color charge degree of freedom in particle physics», Compendium of Quantum Physics, Springer Berlin Heidelberg, pp. 109–111, arXiv:0805.0289, doi:10.1007/978-3-540-70626-7_32, ISBN 978-3-540-70622-9, S2CID 17512393 - ^ Beg, M.A.B.; Lee, B.W.; Pais, A. (1964). «SU(6) and electromagnetic interactions». Physical Review Letters. 13 (16): 514–517, erratum 650. Bibcode:1964PhRvL..13..514B. doi:10.1103/physrevlett.13.514.
- ^ Sakita, B. (1964). «Electromagnetic properties of baryons in the supermultiplet scheme of elementary particles». Physical Review Letters. 13 (21): 643–646. Bibcode:1964PhRvL..13..643S. doi:10.1103/physrevlett.13.643.
- ^ a b Cho, Adrian (2 April 2010). «Mass of the Common Quark Finally Nailed Down». Science. American Association for the Advancement of Science. Archived from the original on 27 August 2015. Retrieved 27 September 2014.
- ^ Wilczek, F. (2003). «The Origin of Mass» (PDF). MIT Physics Annual: 24–35. Archived (PDF) from the original on June 20, 2015. Retrieved May 8, 2015.
- ^
Ji, Xiangdong (1995). «A QCD Analysis of the Mass Structure of the Nucleon». Physical Review Letters. 74 (7): 1071–1074. arXiv:hep-ph/9410274. Bibcode:1995PhRvL..74.1071J. doi:10.1103/PhysRevLett.74.1071. PMID 10058927. S2CID 15148740. - ^ Martinelli, G.; Parisi, G.; Petronzio, R.; Rapuano, F. (1982). «The proton and neutron magnetic moments in lattice QCD» (PDF). Physics Letters B. 116 (6): 434–436. Bibcode:1982PhLB..116..434M. doi:10.1016/0370-2693(82)90162-9. Archived (PDF) from the original on 2020-04-20. Retrieved 2019-08-25.
- ^ Kincade, Kathy (2 February 2015). «Pinpointing the magnetic moments of nuclear matter». Phys.org. Archived from the original on 2 May 2015. Retrieved May 8, 2015.
- ^ a b J. Byrne (2011). Neutrons, Nuclei and Matter: An exploration of the physics of slow neutrons. Mineola, NY: Dover Publications. pp. 28–31. ISBN 978-0486482385.
- ^ Hughes, D.J.; Burgy, M.T. (1949). «Reflection and polarization of neutrons by magnetized mirrors» (PDF). Physical Review. 76 (9): 1413–1414. Bibcode:1949PhRv…76.1413H. doi:10.1103/PhysRev.76.1413. Archived from the original (PDF) on 2016-08-13. Retrieved 2016-06-26.
- ^ Sherwood, J.E.; Stephenson, T.E.; Bernstein, S. (1954). «Stern-Gerlach experiment on polarized neutrons». Physical Review. 96 (6): 1546–1548. Bibcode:1954PhRv…96.1546S. doi:10.1103/PhysRev.96.1546.
- ^
Miller, G.A. (2007). «Charge Densities of the Neutron and Proton». Physical Review Letters. 99 (11): 112001. arXiv:0705.2409. Bibcode:2007PhRvL..99k2001M. doi:10.1103/PhysRevLett.99.112001. PMID 17930428. S2CID 119120565. - ^ «Pear-shaped particles probe big-bang mystery» (Press release). University of Sussex. 20 February 2006. Archived from the original on 2011-06-07. Retrieved 2009-12-14.
- ^ A cryogenic experiment to search for the EDM of the neutron Archived 2012-02-16 at the Wayback Machine. Hepwww.rl.ac.uk. Retrieved on 2012-08-16.
- ^ Search for the neutron electric dipole moment: nEDM Archived 2015-09-25 at the Wayback Machine. Nedm.web.psi.ch (2001-09-12). Retrieved on 2012-08-16.
- ^ US nEDM ORNL experiment public page Archived 2017-04-30 at the Wayback Machine. Retrieved on 2017-02-08.
- ^ SNS Neutron EDM Experiment Archived 2011-02-10 at the Wayback Machine. P25ext.lanl.gov. Retrieved on 2012-08-16.
- ^ Measurement of the Neutron Electric Dipole Moment Archived 2011-08-23 at the Wayback Machine. Nrd.pnpi.spb.ru. Retrieved on 2012-08-16.
- ^ Kisamori, K.; et al. (2016). «Candidate Resonant Tetraneutron State Populated by the He4(He8,Be8) Reaction». Physical Review Letters. 116 (5): 052501. Bibcode:2016PhRvL.116e2501K. doi:10.1103/PhysRevLett.116.052501. PMID 26894705.
- ^ «Physicists find signs of four-neutron nucleus». 2016-02-24. Archived from the original on 2017-07-29. Retrieved 2017-06-27.
- ^ Orr, Nigel (2016-02-03). «Can Four Neutrons Tango?». Physics. 9: 14. Bibcode:2016PhyOJ…9…14O. doi:10.1103/Physics.9.14.
- ^ Spyrou, A.; et al. (2012). «First Observation of Ground State Dineutron Decay: 16Be». Physical Review Letters. 108 (10): 102501. Bibcode:2012PhRvL.108j2501S. doi:10.1103/PhysRevLett.108.102501. PMID 22463404.
- ^ Llanes-Estrada, Felipe J.; Moreno Navarro, Gaspar (2012). «Cubic neutrons». Modern Physics Letters A. 27 (6): 1250033–1–1250033–7. arXiv:1108.1859. Bibcode:2012MPLA…2750033L. doi:10.1142/S0217732312500332. S2CID 118407306.
- ^ Knoll, Glenn F. (1979). «Ch. 14». Radiation Detection and Measurement. John Wiley & Sons. ISBN 978-0471495451.
- ^ Ghosh, P.; W. Fu; M. J. Harrison; P. K. Doyle; N. S. Edwards; J. A. Roberts; D. S. McGregor (2018). «A high-efficiency, low-Ĉerenkov Micro-Layered Fast-Neutron Detector for the TREAT hodoscope». Nuclear Instruments and Methods in Physics: A. 904: 100–106. Bibcode:2018NIMPA.904..100G. doi:10.1016/j.nima.2018.07.035. S2CID 126130994. Archived from the original on 2022-04-12. Retrieved 2020-04-14.
- ^ Ghosh, P.; D. M. Nichols; W. Fu; J. A. Roberts; D. S. McGregor (2020). «Gamma-Ray Rejection of the SiPM-coupled Micro-Layered Fast-Neutron Detector». 2019 IEEE Nuclear Science Symposium and Medical Imaging Conference (NSS/MIC): 1–3. doi:10.1109/NSS/MIC42101.2019.9059869. ISBN 978-1-7281-4164-0. S2CID 204877955.
- ^ Köhn, C.; Ebert, U. (2015). «Calculation of beams of positrons, neutrons and protons associated with terrestrial gamma-ray flashes» (PDF). Journal of Geophysical Research: Atmospheres. 23 (4): 1620–1635. Bibcode:2015JGRD..120.1620K. doi:10.1002/2014JD022229. Archived (PDF) from the original on 2019-12-23. Retrieved 2019-08-25.
- ^ Köhn, C.; Diniz, G.; Harakeh, Muhsin (2017). «Production mechanisms of leptons, photons, and hadrons and their possible feedback close to lightning leaders». Journal of Geophysical Research: Atmospheres. 122 (2): 1365–1383. Bibcode:2017JGRD..122.1365K. doi:10.1002/2016JD025445. PMC 5349290. PMID 28357174.
- ^ Clowdsley, MS; Wilson, JW; Kim, MH; Singleterry, RC; Tripathi, RK; Heinbockel, JH; Badavi, FF; Shinn, JL (2001). «Neutron Environments on the Martian Surface» (PDF). Physica Medica. 17 (Suppl 1): 94–96. PMID 11770546. Archived from the original (PDF) on 2005-02-25.
- ^ Byrne, J. Neutrons, Nuclei, and Matter, Dover Publications, Mineola, New York, 2011, ISBN 0486482383, pp. 32–33.
- ^ «Isotopes and Radioactivity Tutorial». Archived from the original on 2020-02-14. Retrieved 2020-04-16.
- ^ Science/Nature |Q&A: Nuclear fusion reactor Archived 2022-02-25 at the Wayback Machine. BBC News (2006-02-06). Retrieved on 2010-12-04.
- ^ Byrne, J. Neutrons, Nuclei, and Matter, Dover Publications, Mineola, New York, 2011, ISBN 0486482383, p. 453.
- ^ Kumakhov, M.A.; Sharov, V.A. (1992). «A neutron lens». Nature. 357 (6377): 390–391. Bibcode:1992Natur.357..390K. doi:10.1038/357390a0. S2CID 37062511.
- ^ Physorg.com, «New Way of ‘Seeing’: A ‘Neutron Microscope'» Archived 2012-01-24 at the Wayback Machine. Physorg.com (2004-07-30). Retrieved on 2012-08-16.
- ^ «NASA Develops a Nugget to Search for Life in Space» Archived 2014-03-08 at the Wayback Machine. NASA.gov (2007-11-30). Retrieved on 2012-08-16.
- ^ Ioffe, A.; Dabagov, S.; Kumakhov, M. (1995-01-01). «Effective neutron bending at large angles». Neutron News. 6 (3): 20–21. doi:10.1080/10448639508217696. ISSN 1044-8632.
- ^ Hall EJ (2000). Radiobiology for the Radiologist. Lippincott Williams & Wilkins; 5th edition
- ^ Johns HE and Cunningham JR (1978). The Physics of Radiology. Charles C Thomas 3rd edition
- ^ brian.maranville@nist.gov (2017-04-17). «How neutrons are useful». NIST. Archived from the original on 2021-01-25. Retrieved 2021-01-21.
- ^ E Hadden; Y Iso; A Kume; K Umemoto; T Jenke; M Fally; J Klepp; Y Tomita (2022). «HIGHLY EFFICIENT HOLOGRAPHIC OPTICAL ELEMENTS FOR COLD NEUTRON EXPERIMENTS». doi:10.13140/RG.2.2.26033.04963.
- ^ Hadden, Elhoucine; Iso, Yuko; Kume, Atsushi; Umemoto, Koichi; Jenke, Tobias; Fally, Martin; Klepp, Jürgen; Tomita, Yasuo (2022-05-24). McLeod, Robert R; Tomita, Yasuo; Sheridan, John T; Pascual Villalobos, Inmaculada (eds.). «Nanodiamond-based nanoparticle-polymer composite gratings with extremely large neutron refractive index modulation». Photosensitive Materials and Their Applications II. SPIE. 12151: 70–76. Bibcode:2022SPIE12151E..09H. doi:10.1117/12.2623661. ISBN 9781510651784. S2CID 249056691.
- ^ B. Lauss (May 2012). «Startup of the high-intensity ultracold neutron source at the Paul Scherrer Institute». Hyperfine Interact. 211 (1): 21–25. arXiv:1202.6003. Bibcode:2012HyInt.211…21L. doi:10.1007/s10751-012-0578-7. S2CID 119164071.
- ^ R. Golub & J. M. Pendlebury (1977). «The interaction of Ultra-Cold Neutrons (UCN) with liquid helium and a superthermal UCN source». Phys. Lett. A. 62 (5): 337–339. Bibcode:1977PhLA…62..337G. doi:10.1016/0375-9601(77)90434-0.
- ^ A. Steyerl; H. Nagel; F.-X. Schreiber; K.-A. Steinhauser; R. Gähler; W. Gläser; P. Ageron; J. M. Astruc; W. Drexel; G. Gervais & W. Mampe (1986). «A new source of cold and ultracold neutrons». Phys. Lett. A. 116 (7): 347–352. Bibcode:1986PhLA..116..347S. doi:10.1016/0375-9601(86)90587-6.
- ^ Stefan Döge; Jürgen Hingerl & Christoph Morkel (Feb 2020). «Measured velocity spectra and neutron densities of the PF2 ultracold-neutron beam ports at the Institut Laue–Langevin». Nucl. Instrum. Methods A. 953: 163112. arXiv:2001.04538. Bibcode:2020NIMPA.95363112D. doi:10.1016/j.nima.2019.163112. S2CID 209942845. Archived from the original on 2021-02-24. Retrieved 2020-04-24.
- ^ Freeman, Tami (May 23, 2008). «Facing up to secondary neutrons». Medical Physics Web. Archived from the original on 2010-12-20. Retrieved 2011-02-08.
- ^ Heilbronn, L.; Nakamura, T; Iwata, Y; Kurosawa, T; Iwase, H; Townsend, LW (2005). «Expand+Overview of secondary neutron production relevant to shielding in space». Radiation Protection Dosimetry. 116 (1–4): 140–143. doi:10.1093/rpd/nci033. PMID 16604615. Archived from the original on 2019-01-26. Retrieved 2019-01-25.
Further reading[edit]
- James Byrne, Neutrons, Nuclei and Matter: An Exploration of the Physics of Slow Neutrons. Mineola, New York: Dover Publications, 2011. ISBN 0486482383.
- Abraham Pais, Inward Bound, Oxford: Oxford University Press, 1986. ISBN 0198519974.
- Sin-Itiro Tomonaga, The Story of Spin, The University of Chicago Press, 1997
- Herwig Schopper, Weak interactions and nuclear beta decay, Publisher, North-Holland Pub. Co., 1966.
- Annotated bibliography for neutrons from the Alsos Digital Library for Nuclear Issues
Нейтро́н (от лат. neuter — ни тот, ни другой) — элементарная частица, не имеющая электрического заряда. Нейтрон является фермионом и принадлежит к классу барионов. Атомные ядра состоят из нейтронов и протонов.
Содержание
- 1 Открытие
- 2 Основные характеристики
- 3 Строение и распад
- 4 Другие свойства
- 5 Направления исследований в физике нейтронов
- 6 См. также
- 7 Примечания
- 8 Литература
- 9 Ссылки
Открытие
Открытие нейтрона (1932) принадлежит физику Дж. Чедвику, за это открытие он получил Нобелевскую премию по физике в 1935 году.
В 1930 г. В. А. Амбарцумян и Д. Д. Иваненко показали, что ядро не может, как считалось в то время, состоять из протонов и электронов, что электроны, вылетающие из ядра при бета-распаде, рождаются в момент распада, и что кроме протонов, в ядре должны присутствовать некие нейтральные частицы.[3][4]
В 1930 Вальтер Боте и Г. Бекер, работавшие в Германии, обнаружили, что если высокоэнергетичные альфа-частицы, испускаемые полонием-210, попадают на некоторые лёгкие элементы, в особенности на бериллий или литий, образуется излучение с необычно большой проникающей способностью. Сначала считалось, что это — гамма-излучение, но выяснилось, что оно обладает гораздо большей проникающей способностью, чем все известные гамма-лучи, и результаты эксперимента не могут быть таким образом интерпретированы. Важный вклад сделали в 1932 Ирен и Фредерик Жолио-Кюри. Они показали, что если это неизвестное излучение попадает на парафин или любое другое соединение, богатое водородом, образуются протоны высоких энергий. Само по себе это ничему не противоречило, но численные результаты приводили к нестыковкам в теории. Позднее в том же 1932 году английский физик Джеймс Чедвик провёл серию экспериментов, в которых он показал, что гамма-лучевая гипотеза несостоятельна. Он предположил, что это излучение состоит из незаряженных частиц с массой, близкой к массе протона, и произвёл серию экспериментов, подтвердивших эту гипотезу. Эти незаряженные частицы были названы нейтронами от латинского корня neutral и обычного для частиц суффикса on (он). В том же 1932 г. Д. Д. Иваненко[5] и затем В. Гейзенберг предположили, что атомное ядро состоит из протонов и нейтронов.
Основные характеристики
- Масса (примерно на 0,1378 % больше, чем масса протона; приведены рекомендованные значения CODATA 2010 года, в скобках указана погрешность величины в единицах последней значимой цифры, одно стандартное отклонение):
- 939,565378(21) МэВ;[6]
- 1,00866491600(43) а. е. м.;[7]
- 1,674927351(74)·10−27 кг;[8]
- 1838,6836601(16) массы электрона[9].
- Спин: 1/2 (фермион).
- Время жизни в свободном состоянии: 880.1 ± 1.1 секунды[2] (период полураспада — 611 секунд).
- Магнитный момент: −1,91304272(45) ядерного магнетона.[10]
Несмотря на нулевой электрический заряд, нейтрон не является истинно нейтральной частицей. Античастицей нейтрона является антинейтрон, который не совпадает с самим нейтроном.
Строение и распад
кварковая структура нейтрона
Считается надёжно установленным, что нейтрон является связанным состоянием трёх кварков: одного «верхнего» (u) и двух «нижних» (d) кварков (кварковая структура udd). Близость значений масс протона и нейтрона обусловлена свойством приближённой изотопической инвариантности: в протоне (кварковая структура uud) один d-кварк заменяется на u-кварк, но поскольку массы этих кварков очень близки, такая замена слабо сказывается на массе составной частицы.
Поскольку нейтрон тяжелее протона, то он может распадаться в свободном состоянии. Единственным каналом распада, разрешённым законом сохранения энергии и законами сохранения электрического заряда, барионного и лептонного квантовых чисел, является бета-распад нейтрона на протон, электрон и электронное антинейтрино (а также, возможно, гамма-квант). Поскольку этот распад идёт с образованием лептонов и изменением аромата кварков, то он обязан происходить только за счёт слабого взаимодействия. Однако, ввиду специфических свойств слабого взаимодействия, скорость этой реакции аномально мала из-за крайне малого энерговыделения (разности масс начальных и конечных частиц). Именно этим объясняется тот факт, что нейтрон является настоящим долгожителем среди элементарных частиц: его время жизни, приблизительно равное 15 минутам, примерно в миллиард раз больше времени жизни мюона — следующей за нейтроном метастабильной частице по времени жизни.
Кроме того, разница масс между протоном и нейтроном около 1,3 МэВ невелика по меркам ядерной физики. В результате, в ядрах нейтрон может находиться в более глубокой потенциальной яме, чем протон, и потому бета-распад нейтрона оказывается энергетически невыгодным. Это приводит к тому, что в ядрах нейтрон может быть стабильным. Более того, в нейтроно-дефицитных ядрах происходит распад протона в нейтрон (с захватом орбитального электрона или вылетом позитрона).
Другие свойства
Изоспины нейтрона и протона одинаковы (1/2), но их проекции противоположны по знаку. Проекция изоспина нейтрона по соглашению в физике элементарных частиц принимается равной −1/2, в ядерной физике +1/2 (поскольку в большинстве ядер нейтронов больше, чем протонов, это соглашение позволяет избегать отрицательных суммарных проекций изоспина).
Нейтрон — единственная[источник не указан 574 дня] из имеющих массу покоя элементарных частиц, для которой непосредственно наблюдалось гравитационное взаимодействие — искривление в поле земного тяготения траектории хорошо коллимированного пучка холодных нейтронов. Измеренное гравитационное ускорение нейтронов в пределах точности эксперимента совпадает с гравитационным ускорением макроскопических тел.
При огромном давлении внутри нейтронной звезды нейтроны могут деформироваться вплоть до того, что приобретают форму куба[11].
Направления исследований в физике нейтронов
Фундаментальные исследования:
- возможность существования тетранейтронов и иных связанных состояний из одних только нейтронов
- поиск возможных нейтрон-антинейтронных осцилляций
- поиск электрического дипольного момента нейтрона
- изучение свойств сильно нейтроно-избыточных лёгких ядер
Прикладные исследования:
- получение и хранение холодных нейтронов
- влияние потоков нейтронов на живые ткани и организмы
- влияние сверхмощных потоков нейтронов на свойства материалов
- изучение распространения нейтронов в различных средах
- изучение различных типов структуры в физике конденсированных сред
- нейтронно-дифракционный анализ
- нейтронно-активационный анализ
См. также
- Спектр нейтронов
- Бета-распад нейтрона
- Свободный нейтрон
- Нейтронное излучение
- Нейтронная физика
- Нейтронная оптика
- Нейтроний
- Нейтронная звезда
- Ядерная реакция
- Наведённая радиоактивность
Примечания
- ↑ 1 2 3 http://physics.nist.gov/cuu/Constants/Table/allascii.txt Fundamental Physical Constants — Complete Listing
- ↑ 1 2 J. Beringer et al. (Particle Data Group), Phys. Rev. D86, 010001 (2012) http://pdg.lbl.gov/2012/tables/rpp2012-sum-baryons.pdf
- ↑ Ambarzumian, V., Iwanenko, D. Les électrons inobservables et les rayons // Compt. Rend. Acad Sci. Paris. — 1930. — Т. 190. — С. 582.
- ↑ V. A. Ambartsumian — a life in science // Astrophysics. — 2008. — Т. 51. — С. 280-293. — DOI:10.1007/s10511-008-9016-6
- ↑ Iwanenko, D. The neutron hypothesis // Nature. — 1932. — В. 3265. — Т. 129. — № (28 May 1932). — С. 798. — ISSN 0028-0836. — DOI:10.1007/s10511-008-9016-6
- ↑ CODATA Value: proton mass energy equivalent in MeV.
- ↑ CODATA Value: neutron mass in u.
- ↑ CODATA Value: neutron mass.
- ↑ CODATA Value: neutron-electron mass ratio.
- ↑ CODATA Value: neutron magnetic moment to nuclear magneton ratio.
- ↑ Felipe J. Llanes-Estrada, Gaspar Moreno Navarro. (2011), «Cubic neutrons», arΧiv:1108.1859v1 [nucl-th]
Литература
- Dirk Dubbers, Michael G. Schmidt The neutron and its role in cosmology and particle physics (англ.) // Rev. Mod. Phys.. — 2011. — Vol. 83. — P. 1111–1171. — DOI:10.1103/RevModPhys.83.1111
Ссылки
- Экспериментальные свойства нейтронов (сайт Particle Data Group, англ.).
- CODATA Internationally recommended values of the Fundamental Physical Constants (2010).
|
|
В этой статье не хватает ссылок на источники информации.
Информация должна быть проверяема, иначе она может быть поставлена под сомнение и удалена. |
НЕЙТРО́Н (от лат. neuter – ни тот ни другой; символ n), элементарная частица с нулевым электрич. зарядом и массой, незначительно большей массы протона. Н. является фермионом и входит в группу барионов. Наряду с протоном Н. относится к нуклонам и входит в состав атомных ядер. Открыт в 1932 Дж. Чедвиком. Т. к. Н. электрически нейтрален, он легко проникает в атомные ядра при любой энергии и с большой вероятностью вызывает ядерные реакции. Способность Н. вызывать деление тяжёлых ядер в цепной ядерной реакции послужила основой для создания ядерного оружия и ядерной энергетики. Масса Н. $$m_n=939,565379(21): МэВ=1,00866491600(43): а. е. м.=1,674927351(74)·10^{–24}: г.$$
Н. тяжелее протона на $1,293332: МэВ$. Спин Н. равен 1/2. В свободном состоянии Н. нестабилен – распадается на протон, электрон и антинейтрино (см. Бета-распад нейтрона); время жизни составляет $885,7(8)$ с. В связанном состоянии в составе стабильных ядер Н. стабилен. Несмотря на электронейтральность Н., его магнитный момент существенно отличен от нуля: $μ_n=–1,91304272(45)μ_{яд}$, где $μ_{яд}$ – ядерный магнетон, знак магнитного момента определяется относительно направления его спина. Отношение к магнитному моменту протона равно примерно –2/3, что согласуется с кварковой структурой нуклонов.
Статич. электрич. дипольный момент точечной частицы должен быть тождественно равен нулю. Стандартная модель элементарных частиц предполагает малое разделение положительного и отрицательного заряда внутри Н. и предсказывает существование электрич. дипольного момента, но его расчётная величина мала и остаётся за пределами эксперим. обнаружения.
Согласно совр. кварковой модели, Н. состоит из трёх кварков: одного $u$-кварка с электрич. зарядом +2/3$e$ и двух $d$-кварков с зарядами –1/3$e$, связанных между собой глюонами. Квантовые числа Н. целиком определяются набором составляющих его кварков, а пространственная структура – динамикой взаимодействия кварков и глюонов. Особенностью этого взаимодействия является его рост с увеличением расстояния, так что размер Н. ограничен областью порядка 10–13 см – областью конфайнмента кварков. Античастица Н. – антинейтрон $(ñ)$ – открыт в 1956; в пределах точности измерений массы обеих частиц равны.
Свободные Н. в природе образуются в ядерных реакциях, вызываемых $α$-частицами радиоактивного распада, космич. лучами, и в результате спонтанного или вынужденного деления тяжёлых ядер. Искусств. источники Н. – ядерные реакторы, ядерные взрывы, ускорители протонов и электронов с мишенями из тяжёлых элементов (см. Нейтронные источники).
Поскольку Н. не имеет электрич. заряда, детектирование его посредством ионизации атомов вещества невозможно. Для регистрации Н. обычно используются два косвенных метода: захват Н. атомными ядрами с последующим излучением ядром $α$-частицы или $γ$-кванта и рассеяние Н. на ядрах вещества с последующей регистрацией ядра отдачи (см. Нейтронные детекторы).
Методы использования Н. в науч. и прикладных исследованиях зависят от их кинетич. энергии. Н. с кинетич. энергией св. 100 кэВ называют быстрыми, с энергией до 100 кэВ – медленными. Быстрые Н. образуются в ядерных реакциях при бомбардировке разл. ядер заряженными частицами или $γ$-квантами высокой энергии, а также при делении ядер. Медленные нейтроны подразделяют на промежуточные (с энергиями 104–105 эВ), резонансные (0,5–104 эВ), тепловые нейтроны (5·10–3–0,5 эВ), холодные нейтроны (10–7–5·10–3 эВ) и ультрахолодные нейтроны (<10–7 эВ). Тепловые Н. с большой вероятностью захватываются веществом с образованием, как правило, нестабильных, более тяжёлых изотопов атомных ядер. Холодные Н. образуются из тепловых при прохождении через холодные вещества, напр. через жидкий дейтерий. Ультрахолодные нейтроны формируются при упругом рассеянии на твёрдом дейтерии или на жидком сверхтекучем гелии.
Для медленных Н. определяющим фактором становятся их волновые свойства. Н. с длиной волны, близкой к межатомным расстояниям (ок. 0,1 нм), являются важнейшим средством исследования структуры твёрдых тел (см. Нейтронография структурная). Медленные нейтроны, подобно фотонам, рассеиваясь на атомах твёрдого вещества, интерферируют (см. Нейтронная интерферометрия). Структура наблюдаемой дифракции нейтронов связана со строением исследуемой среды. Наличие у Н. магнитного момента делает пучки поляризованных нейтронов чрезвычайно чувствительным инструментом для исследования распределения намагниченности в веществе.
Особенностью взаимодействия Н. с веществом является показатель преломления, меньший единицы. Благодаря этому Н., падающие из вакуума на границу вещества, могут испытывать полное внутр. отражение. Ультрахолодные Н. при скорости менее 5–8 м/с испытывают полное внутр. отражение от границы с углеродом, никелем, бериллием и др. при любом угле падения и могут удерживаться в замкнутых объёмах. Это свойство ультрaхолодных Н. используется в экспериментах и позволяет реализовать нейтронно-оптич. устройства, аналоги оптич. линз и призм (см. Нейтронная оптика).
Способность Н. при облучении вещества вызывать последующее излучение $γ$-квантов используется для активационного анализа. Спектр испущенных $γ$-квантов сопоставляется с таблицей линий излучения известных химич. элементов и позволяет с высокой точностью определить химич. состав вещества.
Н. – одна из немногих элементарных частиц, падение которой в гравитац. поле Земли можно наблюдать экспериментально. Прямое измерение ускорения свободного падения для Н. выполнено с погрешностью 0,3% и не отличается от ускорения свободного падения для макроскопич. тел. Гравитац. ускорение и замедление Н. широко используются в опытах с ультрахолодными нейтронами.
Согласно совр. представлениям, в модели горячей Вселенной (см. Горячей Вселенной теория) образование барионов, в т. ч. протонов и H., происходит в первые минуты жизни Вселенной. В дальнейшем некоторая часть Н., не успевших распасться, захватывается протонами с образованием ядер $ce{^4He}$. По астрономич. оценкам, 15% видимого вещества Вселенной представлено Н., входящими в состав ядер $ce{^4He}$.
Субатомная частица без электрического заряда
 кварк, содержащийся в нейтроне. Назначение цвета отдельных кварков произвольно, но все три цвета должны присутствовать. Силы между кварками опосредуются глюонами. кварк, содержащийся в нейтроне. Назначение цвета отдельных кварков произвольно, но все три цвета должны присутствовать. Силы между кварками опосредуются глюонами. |
|
| Классификация | Барион |
|---|---|
| Состав | 1 верхний кварк, 2 нижние кварки |
| Статистика | Фермионные |
| взаимодействия | Гравитация, слабая, сильная, электромагнитная |
| Символ | . n.,. n.,. N. |
| Античастица | Антинейтрон |
| Теоретически | Эрнест Резерфорд (1920) |
| Обнаружен | Джеймс Чедвик (1932) |
| Масса | 1,67492749804 (95) × 10 kg. 939,56542052 (54) МэВ / c. 1,00866491588 (49) u |
| Средний срок службы | 881,5 (15) с (в свободном состоянии) |
| Электрический заряд | 0 e. (−2 ±  × 10 e (экспериментальные пределы) × 10 e (экспериментальные пределы) |
| Электрический дипольный момент | < 2.9×10 e⋅cm (experimental upper limit) |
| Электрическая поляризуемость | 1,16 (15) × 10 фм |
| Магнитный момент | −0,96623650 (23) × 10 J ·T. −1,04187563 (25) × 10 μB. — 1,91304273 (45) μN |
| Магнитная поляризуемость | 3,7 (20) × 10 фм |
| Спин | 1/2 |
| Изоспин | -1/2 |
| Четность | +1 |
| Конденсированный | I (J ) = 1/2 (1/2) |
нейтрон — это субатомная частица, символ. n. или. n., которая имеет нейтральный (не положительный или негр активный) заряд и масса немного больше, чем у протона. Протоны и нейтроны составляют ядра из атомов. Поскольку протоны и нейтроны ведут себя в ядре одинаково и каждый имеет массу приблизительно одну атомную единицу массы, они оба упоминаются как нуклоны. Их свойства и взаимодействия описываются ядерной физикой.
химические свойства атома в основном определяются конфигурацией электронов, которые вращаются вокруг атома тяжелое ядро. Электронная конфигурация определяется зарядом ядра, задаваемым числом протонов или атомным номером. Нейтроны не влияют на электронную конфигурацию, но сумма атомного номера и количества нейтронов, или нейтронное число, составляет массу ядра.
Атомы химического элемента, которые отличаются только числом нейтронов, называются изотопами. Например, углерод с атомным номером 6 содержит обильный изотоп углерод-12 с 6 нейтронами и редкий изотоп углерод-13 с 7 нейтронами. Некоторые элементы встречаются в природе только с одним стабильным изотопом, например, фтором. Другие элементы встречаются со многими стабильными изотопами, например олово с десятью стабильными изотопами.
Свойства атомного ядра зависят как от атомного, так и от нейтронного числа. Обладая положительным зарядом, протоны в ядре отталкиваются дальнодействующей электромагнитной силой, но гораздо более сильная, но короткодействующая ядерная сила тесно связывает нуклоны друг с другом. Нейтроны необходимы для стабильности ядер, за исключением однопротонного ядра водорода. Нейтроны в больших количествах образуются при делении ядер и синтезе. Они вносят основной вклад в нуклеосинтез химических элементов внутри звезд посредством процессов деления, слияния и нейтронного захвата.
Нейтрон необходим для производства ядерной энергии. Спустя десятилетие после того, как нейтрон был открыт Джеймсом Чедвиком в 1932 году, нейтроны были использованы для индукции многих различных типов ядерных трансмутаций. С открытием ядерного деления в 1938 году было быстро осознано, что если в результате деления будут образовываться нейтроны, каждый из этих нейтронов может вызвать дальнейшие процессы деления в каскаде, известном как цепная ядерная реакция.. Эти события и открытия привели к созданию первого автономного ядерного реактора (Chicago Pile-1, 1942) и первого ядерного оружия (Trinity, 1945).
Свободные нейтроны, хотя и не непосредственно ионизируют атомы, вызывают ионизирующее излучение. Как таковые, они могут представлять биологическую опасность в зависимости от дозы. На Земле существует небольшой естественный «нейтронный фон» поток свободных нейтронов, вызванный ливнями космических лучей и естественной радиоактивностью спонтанно делящихся элементов в земной коре. Выделенные источники нейтронов, такие как генераторы нейтронов, исследовательские реакторы и источники расщепления, производят свободные нейтроны для использования в облучении и в экспериментах по рассеянию нейтронов.
Содержание
- 1 Описание
- 2 Discovery
- 3 Бета-распад и стабильность ядра
- 3.1 Распад свободного нейтрона
- 3.2 Распад связанного нейтрона
- 3.3 Конкуренция типов бета-распада
- 4 Распад нейтрона по физике элементарных частиц
- 5 Внутренние свойства
- 5.1 Масса
- 5.2 Электрический заряд
- 5.3 Магнитный момент
- 5.4 Спин
- 5.5 Структура и геометрия распределения заряда
- 5.6 Электрический дипольный момент
- 5.7 Антинейтрон
- 6 Нейтронные соединения
- 6.1 Динейтроны и тетранейтроны
- 6.2 Нейтрониевые и нейтронные звезды
- 7 Обнаружение
- 7.1 Детектирование нейтронов путем захвата нейтронов
- 7.2 Обнаружение нейтронов посредством упругого рассеяния
- 8 Источники и производство
- 8.1 Нейтронные пучки и модификация пучков после производства
- 9 Области применения
- 10 Медицинские методы лечения
- 11 Защита
- 12 Температура нейтронов
- 12.1 Тепловые нейтроны
- 12,2 Холодные нейтроны
- 12,3 Ультрахолодные нейтроны
- 12,4 Нейтроны с энергией деления
- 12,5 Нейтроны термоядерного синтеза
- 12,6 Промежуточные Нейтроны с высокой энергией
- 12.7 Нейтроны высоких энергий
- 13 См. также
- 13.1 Источники нейтронов
- 13.2 Процессы с участием нейтронов
- 14 Ссылки
- 15 Дополнительная литература
Описание
Атомное ядро образовано количеством протонов Z (атомный номер ) и количеством нейтронов N (нейтронное число ), связанные вместе ядерной силой. Атомный номер определяет химические свойства атома, а нейтронный номер определяет изотоп или нуклид. Термины изотоп и нуклид часто используются как синонимы, но они относятся к химическим и ядерным свойствам соответственно. Изотопы — это нуклиды с одним и тем же атомным номером, но с другим числом нейтронов. Нуклиды с одинаковым числом нейтронов, но разным атомным номером, называются изотонами. Атомное массовое число, A, равно сумме атомного и нейтронного номеров. Нуклиды с одинаковыми атомными массовыми числами, но разными атомными и нейтронными числами, называются изобарами.
Ядро наиболее распространенного изотопа атома водорода (с химический символ H) — одиночный протон. Ядра тяжелых изотопов водорода дейтерия (D или H) и трития (T или H) содержат один протон, связанный с одним и двумя нейтронами, соответственно. Все другие типы атомных ядер состоят из двух или более протонов и различного количества нейтронов. Например, самый распространенный нуклид обычного химического элемента свинца, Pb, имеет 82 протона и 126 нейтронов. Таблица нуклидов включает все известные нуклиды. Несмотря на то, что это не химический элемент, нейтрон включен в эту таблицу.
Свободный нейтрон имеет массу 939,565,413,3 эВ / c, или 1,674927471 × 10 кг или 1.00866491588 u. Нейтрон имеет средний квадрат радиус примерно 0,8 × 10 м, или 0,8 фм, и это спин-½ фермион. У нейтрона нет измеримого электрического заряда. Обладая положительным электрическим зарядом, протон испытывает непосредственное влияние электрических полей, тогда как на нейтрон электрические поля не действуют. Однако нейтрон имеет магнитный момент , поэтому на нейтрон влияют магнитные поля. Магнитный момент нейтрона имеет отрицательное значение, поскольку его ориентация противоположна спину нейтрона.
Свободный нейтрон нестабилен, распадается на протон, электрон и антинейтрино со средним сроком службы чуть менее 15 минут (879,6 ± 0,8 с). Этот радиоактивный распад, известный как бета-распад, возможен, потому что масса нейтрона немного больше, чем масса протона. Свободный протон стабилен. Однако нейтроны или протоны, связанные в ядре, могут быть стабильными или нестабильными, в зависимости от нуклида . Бета-распад, при котором нейтроны распадаются на протоны или наоборот, регулируется слабой силой и требует испускания или поглощения электронов и нейтрино или их античастиц.

Протоны и нейтроны ведут себя почти одинаково под влиянием ядерной силы внутри ядра. Концепция изоспина, в которой протон и нейтрон рассматриваются как два квантовых состояния одной и той же частицы, используется для моделирования взаимодействий нуклонов посредством ядерных или слабых сил. Из-за силы ядерной силы на коротких расстояниях, энергия связи нуклонов более чем на семь порядков больше, чем электромагнитная энергия, связывающая электроны в атомах. Ядерные реакции (такие как деление ядер ), следовательно, имеют плотность энергии, которая более чем в десять миллионов раз превышает плотность химических реакций. Из-за эквивалентности массы и энергии, энергии связи ядер уменьшают массу ядер. В конечном счете, способность ядерных сил накапливать энергию, возникающую в результате электромагнитного отталкивания ядерных компонентов, является основой большей части энергии, которая делает возможными ядерные реакторы или бомбы. При ядерном делении поглощение нейтрона тяжелым нуклидом (например, уран-235 ) приводит к тому, что нуклид становится нестабильным и распадается на легкие нуклиды и дополнительные нейтроны. Положительно заряженные легкие нуклиды затем отталкиваются, высвобождая электромагнитную потенциальную энергию.
. Нейтрон классифицируется как адрон, потому что это составная частица, состоящая из кварков.. Нейтрон также классифицируется как барион, потому что он состоит из трех валентных кварков . Конечный размер нейтрона и его магнитный момент указывают на то, что нейтрон является составной, а не элементарной частицей. Нейтрон содержит два нижних кварка с зарядом — ⁄ 3e и один верхний кварк с зарядом + ⁄ 3 e.
Подобно протонам кварки нейтрона удерживаются вместе сильной силой, опосредованной глюонами. Ядерное взаимодействие является результатом вторичных эффектов более фундаментального сильного взаимодействия.
Discovery
История открытия нейтрона и его свойств занимает центральное место в необычных достижениях в атомной физике, которые произошли в Первая половина 20-го века, что в конечном итоге привело к атомной бомбе в 1945 году. В модели Резерфорда 1911 года атом состоял из небольшого положительно заряженного массивного ядра, окруженного гораздо большим облаком отрицательно заряженных электронов. В 1920 году Резерфорд предположил, что ядро состоит из положительных протонов и нейтрально заряженных частиц, предположительно, это протон и электрон, каким-то образом связанные. Предполагалось, что электроны находятся внутри ядра, потому что было известно, что бета-излучение состоит из электронов, испускаемых ядром. Резерфорд назвал эти незаряженные частицы нейтронами, используя латинский корень для нейтралис (средний) и греческий суффикс -on (суффикс, используемый в названиях субатомных частиц, то есть электрона и протона).. Ссылки на слово нейтрон в связи с атомом можно найти в литературе еще в 1899 году.
На протяжении 1920-х годов физики предполагали, что атомное ядро состоит из протонов и «ядерных электронов», но там были очевидные проблемы. Было трудно согласовать протон-электронную модель ядер с соотношением неопределенностей Гейзенберга квантовой механики. Парадокс Клейна, открытый Оскаром Клейном в 1928 году, представил дальнейшие квантово-механические возражения против идеи электрона, заключенного в ядре. Наблюдаемые свойства атомов и молекул не соответствовали ядерному спину, ожидаемому из протон-электронной гипотезы. И протоны, и электроны несут собственный спин 1/2. Изотопы одного и того же вида (т.е. имеющие одинаковое количество протонов) могут иметь как целочисленный, так и дробный спин, то есть спин нейтрона также должен быть дробным (½ ħ). Однако нет способа расположить спины электрона и протона (предполагается, что они соединяются с образованием нейтрона), чтобы получить дробный спин нейтрона.
В 1931 году Вальтер Боте и Герберт Беккер обнаружили, что если альфа-частица излучение полония падает на бериллий, бор или литий, было получено необычно проникающее излучение. На излучение не влияло электрическое поле, поэтому Боте и Беккер предположили, что это было гамма-излучение. В следующем году Ирен Жолио-Кюри и Фредерик Жолио-Кюри в Париже показали, что если это «гамма-излучение попадет на парафин или любой другой водород -содержащее соединение, оно выбрасывает протоны очень высокой энергии. Ни Резерфорд, ни Джеймс Чедвик из Кавендишской лаборатории в Кембридже не были убеждены в интерпретации гамма-лучей. Чедвик быстро провел серию экспериментов, которые показали, что новое излучение состоит из незаряженных частиц с массой примерно такой же, как и у протона. Эти частицы были нейтронами. За это открытие Чедвик в 1935 г. получил Нобелевскую премию по физике.

Модели атомного ядра, состоящие из протонов и нейтронов, были быстро разработаны Вернером Гейзенбергом и другими. Протон-нейтронная модель объяснила загадку ядерных спинов. Энрико Ферми в 1934 г. объяснил происхождение бета-излучения процессом бета-распада, в котором нейтрон распадается на протон, создавая электрон и (пока не обнаруженный) нейтрино. В 1935 году Чедвик и его докторант Морис Голдхабер сообщили о первом точном измерении массы нейтрона.
К 1934 году Ферми бомбардировал более тяжелые элементы нейтронами, чтобы вызвать радиоактивность в элементах высокий атомный номер. В 1938 году Ферми получил Нобелевскую премию по физике «за демонстрацию существования новых радиоактивных элементов, производимых нейтронным облучением, а также за связанное с ним открытие ядерных реакций, вызываемых медленными нейтронами». В 1938 Отто Хан, Лиз Мейтнер и Фриц Штрассманн открыли ядерное деление, или разделение ядер урана на легкие элементы, вызванное нейтронной бомбардировкой. В 1945 году Хан получил в 1944 г. Нобелевскую премию по химии «за открытие деления тяжелых ядер атомов». Открытие ядерного деления приведет к развитию ядерной энергетики и атомной бомбы к концу Второй мировой войны.
Бета-распад и стабильность ядра
Поскольку взаимодействующие протоны имеют взаимное электромагнитное отталкивание, которое сильнее их притягивающего ядерного взаимодействия, нейтроны являются необходимой составляющей любого атомного ядра, которое содержит более одного протона (см. дипротон и нейтрон-протонное отношение ). Нейтроны связываются с протонами и друг с другом в ядре посредством ядерной силы, эффективно уменьшая силы отталкивания между протонами и стабилизируя ядро.
Нейтроны и протоны, связанные в ядре, образуют квантово-механическую систему, в которой каждый нуклон связан в определенном иерархическом квантовом состоянии. Протоны могут распадаться на нейтроны или наоборот внутри ядра. Этот процесс, называемый бета-распадом, требует испускания электрона или позитрона и связанного с ним нейтрино. Эти испускаемые частицы уносят избыток энергии, когда нуклон падает из одного квантового состояния в состояние с более низкой энергией, в то время как протон (или нейтрон) превращается в нейтрон (или протон). Такие процессы распада могут происходить только в том случае, если это разрешено основными законами сохранения энергии и квантово-механическими ограничениями. От этих ограничений зависит стабильность ядер.
Распад свободного нейтрона
Вне ядра свободные нейтроны нестабильны и имеют среднее время жизни 879,6 ± 0,8 с (около 14 минут 40 секунд); поэтому период полураспада для этого процесса (который отличается от среднего времени жизни на коэффициент ln (2) = 0,693) составляет 610,1 ± 0,7 с (около 10 минут, 10 секунд). Этот распад возможен только потому, что масса протона меньше массы нейтрона. По эквивалентности массы и энергии, когда нейтрон распадается на протон таким образом, он достигает более низкого энергетического состояния. Бета-распад нейтрона, описанный выше, может быть обозначен радиоактивным распадом :
- . n. →. p. +. e. +. ν. e
, где. p.,. e. и. ν. eобозначают протон, электрон и электронный антинейтрино, соответственно. Для свободного нейтрона энергия распада для этого процесса (основанная на массах нейтрона, протона и электрона) составляет 0,782343 МэВ. Максимальная энергия бета-распада электрона (в процессе, когда нейтрино получает исчезающе малую кинетическую энергию) была измерена на уровне 0,782 ± 0,013 МэВ. Последнее число недостаточно хорошо измерено, чтобы определить сравнительно крошечную массу покоя нейтрино (которую теоретически необходимо вычесть из максимальной кинетической энергии электрона), так как масса нейтрино ограничивается многими другими методами.
Небольшая часть (примерно одна из 1000) свободных нейтронов распадается с теми же продуктами, но с добавлением дополнительной частицы в виде испускаемого гамма-луча:
- . n. →. p. +. e. +. ν. e+. γ.
Этот гамма-луч можно рассматривать как «внутреннее тормозное излучение », которое возникает в результате электромагнитного взаимодействия испускаемой бета-частицы с протоном. Образование внутреннего тормозного гамма-излучения также является второстепенным признаком бета-распада связанных нейтронов (как обсуждается ниже).
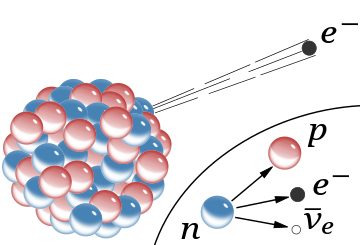
Очень небольшая часть нейтронных распадов (около четырех на миллион) — это так называемые «двухчастичные (нейтронные) распады», в которых образуются протон, электрон и антинейтрино как обычно, но электрон не может набрать энергию 13,6 эВ, необходимую для выхода из протона (энергия ионизации водорода ), и поэтому просто остается связанным с ним как нейтральный атом водорода (одно из «двух тел»). В этом типе распада свободного нейтрона почти вся энергия распада нейтрона уносится антинейтрино (другим «телом»). (Атом водорода отскакивает со скоростью, умноженной на (энергия распада) / (энергия покоя водорода) скорости света, или 250 км / с.)
Превращение свободного протона в нейтрон ( плюс позитрон и нейтрино) энергетически невозможно, так как свободный нейтрон имеет большую массу, чем свободный протон. Но столкновение протона с электроном или нейтрино при высоких энергиях может привести к образованию нейтрона.
Распад связанного нейтрона
В то время как свободный нейтрон имеет период полураспада около 10,2 мин, большинство нейтронов в ядрах стабильны. Согласно модели ядерной оболочки, протоны и нейтроны нуклида представляют собой квантово-механическую систему, организованную в дискретные энергетические уровни с уникальными квантовые числа. Для распада нейтрона образовавшемуся протону требуется доступное состояние с меньшей энергией, чем исходное состояние нейтрона. В стабильных ядрах все возможные состояния с более низкой энергией заполнены, что означает, что каждое из них занято двумя протонами со спином вверх и вниз. Принцип исключения Паули поэтому запрещает распад нейтрона на протон в стабильных ядрах. Ситуация аналогична электронам в атоме, где электроны имеют различные атомные орбитали и не могут распадаться на более низкие энергетические состояния с испусканием фотона в соответствии с принципом исключения.
Нейтроны в нестабильных ядрах могут распадаться посредством бета-распада, как описано выше. В этом случае для протона, образовавшегося в результате распада, доступно энергетически разрешенное квантовое состояние. Одним из примеров этого распада является углерод-14 (6 протонов, 8 нейтронов), который распадается до азота-14 (7 протонов, 7 нейтронов) с периодом полураспада около 5730 лет..
Внутри ядра протон может превратиться в нейтрон посредством обратного бета-распада, если для нейтрона доступно энергетически разрешенное квантовое состояние. Это преобразование происходит путем испускания позитрона и электронного нейтрино:
- . p. →. n. +. e. +. ν. e
Преобразование протона в нейтрон внутри ядра также возможно посредством захвата электрона :
- . p. +. e. →. n. +. ν. e
захвата позитрона нейтронами в ядрах, содержащих избыток нейтронов также возможен, но это затруднено, потому что позитроны отталкиваются положительным ядром и быстро аннигилируют при встрече с электронами.
Конкуренция типов бета-распада
Три конкурирующих типа бета-распада иллюстрируются одним изотопом меди-64 (29 протонов, 35 нейтронов), который имеет период полувыведения около 12,7 часов. У этого изотопа один неспаренный протон и один неспаренный нейтрон, поэтому либо протон, либо нейтрон могут распадаться. Этот конкретный нуклид почти с равной вероятностью подвергнется распаду протона (посредством эмиссии позитронов, 18% или захвата электронов, 43%) или нейтронного распада (посредством эмиссии электронов, 39%).
Распад нейтрона по физике элементарных частиц

В теоретических рамках Стандартной модели для физики элементарных частиц нейтрон состоит из двух нижних кварков и верхнего кварка. Единственно возможный режим распада нейтрона, который сохраняет барионное число, — это для одного из кварков нейтрона изменение аромата через слабое взаимодействие. Распад одного из нижних кварков нейтрона в более легкий верхний кварк может быть достигнут за счет испускания бозона W. Посредством этого процесса, описываемого Стандартной моделью бета-распада, нейтрон распадается на протон (который содержит один нижний и два верхних кварка), электрон и электронный антинейтрино.

Распад протона на нейтрон происходит аналогично через электрослабый сила. Распад одного из верхних кварков протона на нижний кварк может быть осуществлен путем испускания W-бозона. Протон распадается на нейтрон, позитрон и электронное нейтрино. Эта реакция может происходить только внутри атомного ядра, которое имеет квантовое состояние с более низкой энергией, доступной для созданного нейтрона.
Внутренние свойства
Масса
Масса нейтрона не может быть напрямую определена с помощью масс-спектрометрии из-за отсутствия электрического заряда. Однако, поскольку массы протона и дейтрона могут быть измерены с помощью масс-спектрометра, масса нейтрона может быть вычислена путем вычитания массы протона из массы дейтрона, с разницей в массе нейтрон плюс энергия связи дейтерия (выраженная как положительная излучаемая энергия). Последний может быть непосредственно измерен путем измерения энергии (B d { displaystyle B_ {d}}

- mn = md — mp + B d — E rd { displaystyle m_ {n} = m_ {d} -m_ {p} + B_ {d} -E_ {rd}}
Энергия гаммы луч может быть измерен с высокой точностью методами дифракции рентгеновских лучей, как это было впервые сделано Беллом и Эллиотом в 1948 году. Лучшие современные (1986) значения массы нейтрона с помощью этого метода предоставлены Greene et al. Они дают массу нейтрона:
- mнейтрон = 1,008644904 (14) u
Значение массы нейтрона в МэВ известно менее точно из-за меньшей точности известного преобразования u до МэВ:
- mнейтрон = 939,56563 (28) МэВ / c.
Другой метод определения массы нейтрона начинается с бета-распада нейтрона, когда импульсы образующегося протона и электрон измеряются.
Электрический заряд
Полный электрический заряд нейтрона равен 0 e. Это нулевое значение было проверено экспериментально, и текущий экспериментальный предел для заряда нейтрона составляет -2 (8) × 10 e, или -3 (13) × 10 C. Это значение соответствует нулю, учитывая экспериментальные неопределенности (указанные в скобках). Для сравнения, заряд протона равен +1 e.
Магнитный момент
. Хотя нейтрон является нейтральной частицей, магнитный момент нейтрона не равен нулю. На нейтрон не действуют электрические поля, но на него действуют магнитные поля. Магнитный момент нейтрона указывает на его кварковую субструктуру и распределение внутреннего заряда. Значение магнитного момента нейтрона было впервые непосредственно измерено Луисом Альваресом и Феликсом Блохом в Беркли, Калифорния в 1940 году. Альварес и Блох определили магнитный момент нейтрона должно быть μ n = −1.93 (2) μ N, где μ N — ядерный магнетон.
В кварковая модель для адронов, нейтрон состоит из одного верхнего кварка (заряд +2/3 e) и двух нижних кварков (заряд -1/3 e). Магнитный момент нейтрона можно смоделировать как сумму магнитных моментов составляющих кварков. Расчет предполагает, что кварки ведут себя как точечные дираковские частицы, каждая из которых имеет свой магнитный момент. Упрощенно, магнитный момент нейтрона можно рассматривать как результат векторной суммы трех магнитных моментов кварков плюс орбитальные магнитные моменты, вызванные движением трех заряженных кварков внутри нейтрона.
В одном из первых успехов Стандартной модели (теория SU (6), теперь понимаемая в терминах поведения кварков) в 1964 году Мирза А.Б. Бег, Бенджамин В. Ли и Абрахам Пайс теоретически рассчитали отношение магнитных моментов протона к нейтрону, равное −3/2, что согласуется с экспериментальным значением с точностью до 3%. Измеренное значение этого отношения составляет -1,45989805 (34). Противоречие квантово-механической основы этого расчета с принципом исключения Паули привело к открытию цветового заряда кварков Оскаром В.. Гринберг в 1964 году.
Вышеупомянутая трактовка сравнивает нейтроны с протонами, позволяя вычесть сложное поведение кварков между моделями и просто исследуя, каковы будут эффекты различных зарядов кварков (или кварков). тип). Таких вычислений достаточно, чтобы показать, что внутренняя часть нейтронов очень похожа на внутреннюю часть протонов, за исключением разницы в составе кварков с нижним кварком в нейтроне, заменяющим верхний кварк в протоне.
Магнитный момент нейтрона можно грубо вычислить, приняв простую нерелятивистскую, квантово-механическую волновую функцию для барионов, состоящих из трех кварков. Прямой расчет дает довольно точные оценки магнитных моментов нейтронов, протонов и других барионов. Для нейтрона конечным результатом этого расчета является то, что магнитный момент нейтрона определяется выражением μ n = 4/3 μ d — 1/3 μ u., где μ d и μ u — магнитные моменты для нижнего и верхнего кварков соответственно. Этот результат объединяет собственные магнитные моменты кварков с их орбитальными магнитными моментами и предполагает, что три кварка находятся в конкретном доминирующем квантовом состоянии.
| Барион | Магнитный момент. модели кварков | Вычислено. (μ N { displaystyle mu _ { mathrm {N}}} ) ) |
Наблюдаемое. (μ N { displaystyle mu _ { mathrm {N}}} ) ) |
|---|---|---|---|
| p | 4/3 μ u — 1/3 μ d | 2,79 | 2,793 |
| n | 4/3 μ d — 1/3 μ u | -1,86 | -1,913 |
Результаты этого расчета обнадеживают, но Предполагалось, что массы верхних или нижних кварков равны 1/3 массы нуклона. На самом деле массы кварков составляют всего около 1% от массы нуклона. Несоответствие проистекает из сложности Стандартной модели для нуклонов, где большая часть их массы происходит от полей глюонов, виртуальных частиц и связанной с ними энергии, которые являются важными аспектами сильного взаимодействия. Кроме того, сложная система кварков и глюонов, составляющих нейтрон требует релятивистского подхода. Магнитный момент нуклона был успешно вычислен численно из первых принципов, однако, включая все t Он упоминает эффекты и использует более реалистичные значения масс кварков. Расчет дал результаты, которые хорошо согласовывались с измерениями, но потребовали значительных вычислительных ресурсов.
Спин
Нейтрон — частица со спином 1/2, то есть фермион с собственным угловым моментом, равным 1/2 ħ, где ħ — приведенная постоянная Планка. В течение многих лет после открытия нейтрона его точный спин был неоднозначным. Хотя предполагалось, что это частица Дирака со спином 1/2 , возможность того, что нейтрон был частицей со спином 3/2, сохранялась. Взаимодействие магнитного момента нейтрона с внешним магнитным полем было использовано, чтобы окончательно определить спин нейтрона. В 1949 году Хьюз и Берги измерили нейтроны, отраженные от ферромагнитного зеркала, и обнаружили, что угловое распределение отражений соответствует спину 1/2. В 1954 году Шервуд, Стефенсон и Бернштейн использовали нейтроны в эксперименте Штерна-Герлаха, в котором для разделения спиновых состояний нейтронов использовалось магнитное поле. Они зарегистрировали два таких спиновых состояния, соответствующих частице со спином 1/2.
Как фермион, нейтрон подчиняется принципу исключения Паули ; два нейтрона не могут иметь одинаковые квантовые числа. Это источник давления вырождения, который делает возможными нейтронные звезды.
Структура и геометрия распределения заряда
В статье, опубликованной в 2007 году, с независимым от модели анализом сделан вывод о том, что нейтрон имеет отрицательно заряженную внешнюю поверхность, положительно заряженную середину и отрицательное ядро. В упрощенном классическом представлении отрицательная «кожа» нейтрона помогает ему притягиваться к протонам, с которыми он взаимодействует в ядре. (Однако основное притяжение между нейтронами и протонами осуществляется посредством ядерной силы, которая не связана с электрическим зарядом.)
Упрощенный классический взгляд на распределение заряда нейтрона также «объясняет» Дело в том, что магнитный диполь нейтрона направлен в противоположную сторону от вектора его спинового момента импульса (по сравнению с протоном). По сути, это придает нейтрону магнитный момент, который напоминает отрицательно заряженную частицу. Классически это может быть согласовано с нейтральным нейтроном, состоящим из распределения заряда, в котором отрицательные части нейтрона имеют больший средний радиус распределения и, следовательно, вносят больший вклад в магнитный дипольный момент частицы, чем положительные части, которые являются, в среднем, ближе к ядру.
Электрический дипольный момент
Стандартная модель физики элементарных частиц предсказывает крошечное разделение положительного и отрицательного заряда внутри нейтрона, что приводит к постоянному электрическому дипольному моменту. Однако предсказанное значение намного ниже текущей чувствительности экспериментов. Из нескольких нерешенных загадок в физике элементарных частиц становится ясно, что Стандартная модель не является окончательным и полным описанием всех частиц и их взаимодействий. Новые теории, выходящие за рамки Стандартной модели, обычно приводят к гораздо более крупным предсказаниям электрического дипольного момента нейтрона. В настоящее время проводится как минимум четыре эксперимента по измерению конечного электрического дипольного момента нейтрона, в том числе:
- Эксперимент EDM криогенного нейтрона, проводимый в Институте Лауэ – Ланжевена
- nEDM. эксперимент на новом источнике УХН в Институте Пола Шерера
- Эксперимент с nEDM планируется на Источник нейтронов расщепления
- Эксперимент с nEDM, проводимый в Институте Лауэ – Ланжевена
Антинейтрон
Антинейтрон — это античастица нейтрона. Он был открыт Брюсом Корком в 1956 году, через год после открытия антипротона. CPT-симметрия налагает сильные ограничения на относительные свойства частиц и античастиц, поэтому изучение антинейтронов обеспечивает строгие тесты на CPT-симметрию. Относительная разница масс нейтрона и антинейтрона составляет (9 ± 6) × 10. Поскольку разница составляет всего два стандартных отклонения от нуля, это не дает убедительных доказательств нарушения СРТ.
Нейтронные соединения
Динейтроны и тетранейтроны
Существование стабильных кластеров из 4 нейтронов, или тетранейтронов, было выдвинуто группой под руководством Франсиско-Мигеля Маркеса из Лаборатории ядерной физики CNRS на основании наблюдений за распадом бериллий -14 ядер. Это особенно интересно, потому что современная теория предполагает, что эти кластеры не должны быть стабильными.
В феврале 2016 года японский физик Сусуму Шимура из Токийского университета и его коллеги сообщили, что они впервые наблюдали предполагаемые тетранейтроны экспериментально. Физики-ядерщики всего мира говорят, что это открытие, если оно подтвердится, станет важной вехой в области ядерной физики и ядерной физики. безусловно, углубит наше понимание ядерных сил.
динейтрон — еще одна гипотетическая частица. В 2012 году Артемис Спайро из Университета штата Мичиган и его коллеги сообщили, что они впервые наблюдали эмиссию динейтрона при распаде Be. О динейтронном характере свидетельствует малый угол вылета между двумя нейтронами. Авторы измерили энергию разделения двух нейтронов, равную 1,35 (10) МэВ, что хорошо согласуется с расчетами модели оболочки, используя стандартные взаимодействия для этой области масс.
Нейтроний и нейтронные звезды
При При чрезвычайно высоких давлениях и температурах нуклоны и электроны, как полагают, коллапсируют в объемную нейтронную материю, называемую нейтронием. Предполагается, что это происходит в нейтронных звездах.
Экстремальное давление внутри нейтронной звезды может деформировать нейтроны до кубической симметрии, позволяя более плотно упаковывать нейтроны.
Обнаружение
Обычные средства обнаружения заряженной частицы путем поиска следа ионизации (например, в камере Вильсона ) не работают напрямую для нейтронов. Нейтроны, которые упруго рассеиваются на атомах, могут образовывать ионизационный трек, который можно обнаружить, но эксперименты не так просты; Чаще используются другие средства обнаружения нейтронов, заключающиеся в их взаимодействии с атомными ядрами. Таким образом, обычно используемые методы обнаружения нейтронов можно разделить на категории в соответствии с ядерными процессами, на которые опираются, в основном захват нейтронов или упругое рассеяние.
Обнаружение нейтронов путем захвата нейтронов
Метод обнаружения нейтронов включает преобразование энергии, высвобождаемой в реакциях захвата нейтронов, в электрические сигналы. Некоторые нуклиды имеют высокое сечение захвата нейтрона , которое представляет собой вероятность поглощения нейтрона. При захвате нейтронов составное ядро испускает более легко обнаруживаемое излучение, например альфа-частицу, которое затем обнаруживается. Для этой цели пригодны нуклиды. He.,. Li.,. B.,. U.,. U.,. Np. и. Pu..
Обнаружение нейтронов с помощью упругого рассеяния
Нейтроны могут упруго рассеиваться от ядер, вызывая отдачу пораженного ядра. Кинематически нейтрон может передать больше энергии легкому ядру, такому как водород или гелий, чем более тяжелому ядру. Детекторы, основанные на упругом рассеянии, называются детекторами быстрых нейтронов. Ядра отдачи могут ионизировать и возбуждать другие атомы посредством столкновений. Полученный таким образом заряд и / или сцинтилляционный свет можно собрать для получения детектируемого сигнала. Основная проблема при обнаружении быстрых нейтронов — отличить такие сигналы от ошибочных сигналов, создаваемых гамма-излучением в том же детекторе. Такие методы, как распознавание формы импульса, могут использоваться для различения нейтронных сигналов от сигналов гамма-излучения, хотя некоторые детекторы на основе неорганических сцинтилляторов были разработаны для избирательного обнаружения нейтронов в полях смешанного излучения, по сути, без каких-либо дополнительных методов.
Детекторы быстрых нейтронов имеют то преимущество, что не требуют замедлителя и, следовательно, способны измерять энергию нейтрона, время прибытия и, в некоторых случаях, направление падения.
Источники и производство
Свободные нейтроны нестабильны, хотя у них самый длительный период полураспада из всех нестабильных субатомных частиц на несколько порядков. Однако их период полураспада составляет всего около 10 минут, поэтому их можно получить только из источников, которые производят их непрерывно.
Естественный нейтронный фон. Небольшой естественный фоновый поток свободных нейтронов существует повсюду на Земле. В атмосфере и глубоко в океане «нейтронный фон» вызывается мюонами, возникающими при взаимодействии космических лучей с атмосферой. Эти высокоэнергетические мюоны способны проникать на значительные глубины в воду и почву. Там, поражая атомные ядра, среди других реакций они вызывают реакции расщепления, в которых нейтрон высвобождается из ядра. В земной коре вторым источником являются нейтроны, образующиеся в основном в результате спонтанного деления урана и тория, присутствующих в минералах земной коры. Нейтронный фон недостаточно силен, чтобы представлять биологическую опасность, но он важен для детекторов частиц с очень высоким разрешением, которые ищут очень редкие события, такие как (предполагаемые) взаимодействия, которые могут быть вызваны частицами темной материи.. Недавние исследования показали, что даже грозы могут производить нейтроны с энергией до нескольких десятков МэВ. Недавние исследования показали, что флюенс этих нейтронов составляет от 10 до 10 на мс и на м в зависимости от высоты обнаружения. Энергия большинства этих нейтронов, даже с начальной энергией 20 МэВ, снижается до диапазона кэВ в течение 1 мс.
Еще более сильное нейтронное фоновое излучение возникает на поверхности Марса, где атмосфера толстая. достаточно, чтобы генерировать нейтроны от образования мюонов космических лучей и отщепления нейтронов, но не достаточно толстым, чтобы обеспечить значительную защиту от образовавшихся нейтронов. Эти нейтроны не только создают опасность нейтронного излучения на поверхности Марса из-за прямого нисходящего нейтронного излучения, но также могут создавать значительную опасность из-за отражения нейтронов от поверхности Марса, что приведет к возникновению отраженного нейтронного излучения, проникающего вверх в марсианский корабль или среду обитания с моря. этаж.
Источники нейтронов для исследований. К ним относятся определенные типы радиоактивного распада (спонтанное деление и нейтронное излучение ), а также некоторые ядерные реакции. Удобные ядерные реакции включают в себя настольные реакции, такие как естественная альфа- и гамма-бомбардировка определенных нуклидов, часто бериллия или дейтерия, и индуцированное ядерное деление, такое как происходит в ядерных реакторах. Кроме того, высокоэнергетические ядерные реакции (такие как ливни космической радиации или столкновения ускорителей) также производят нейтроны в результате распада ядер-мишеней. Небольшие (настольные) ускорители элементарных частиц, оптимизированные для получения свободных нейтронов таким образом, называются нейтронными генераторами.
На практике наиболее часто используемые небольшие лабораторные источники нейтронов используют радиоактивный распад для производства нейтронов.. Один отметил вырабатывающий нейтроны радиоизотоп, калифорний -252 распада (период полураспада 2,65 года) в результате спонтанного деления в 3% случаев с образованием 3,7 нейтронов на деление, и используется только как источник нейтронов от этого процесса. источники ядерной реакции (состоящие из двух материалов), работающие на радиоизотопах, используют источник альфа-распада плюс бериллиевую мишень или источник высокоэнергетического гамма-излучения от источника, подвергающегося бета-распад с последующим гамма-распадом, который производит фотонейтроны при взаимодействии высокоэнергетического гамма-кванта с обычным стабильным бериллием или с дейтерий в тяжелой воде. Популярным источником последнего типа является радиоактивный сурьма-124 плюс бериллий, система с периодом полураспада 60,9 дней, которую можно построить из природной сурьмы (что составляет 42,8%). стабильная сурьма-123), активируя ее нейтронами в ядерном реакторе, а затем транспортируют туда, где необходим источник нейтронов.

Реакторы ядерного деления естественным образом производят свободные нейтроны; их роль — поддерживать производящую энергию цепную реакцию. Интенсивное нейтронное излучение также может быть использовано для получения различных радиоизотопов в процессе активации нейтронов, который представляет собой тип захвата нейтронов.
Экспериментальный ядерный синтез. реакторы производят свободные нейтроны как отходы. Однако именно эти нейтроны обладают большей частью энергии, и преобразование этой энергии в полезную форму оказалось сложной инженерной задачей. В термоядерных реакторах, вырабатывающих нейтроны, вероятно, образуются радиоактивные отходы, но отходы состоят из активированных нейтронами более легких изотопов, которые имеют относительно короткие периоды распада (50–100 лет) по сравнению с типичным периодом полураспада в 10 000 лет для отходов деления. который является долгим в первую очередь из-за длительного периода полураспада трансурановых актинидов, излучающих альфа.
Нейтронные пучки и модификация пучков после получения
Свободные нейтронные пучки получают от источников нейтронов посредством переноса нейтронов. Чтобы получить доступ к интенсивным источникам нейтронов, исследователи должны обратиться в специализированную нейтронную установку, на которой работает исследовательский реактор или расщепленный источник.
Отсутствие у нейтронов полного электрического заряда затрудняет их управление или ускорение. Заряженные частицы могут ускоряться, замедляться или отклоняться электрическими или магнитными полями. Эти методы мало влияют на нейтроны. Однако некоторые эффекты могут быть достигнуты при использовании неоднородных магнитных полей из-за магнитного момента нейтрона. Нейтронами можно управлять с помощью методов, включающих в себя замедление, отражение и выбор скорости. Тепловые нейтроны могут быть поляризованы путем прохождения через магнитные материалы способом, аналогичным эффекту Фарадея для фотонов. Холодные нейтроны с длинами волн 6–7 ангстрем могут образовываться в пучках с высокой степенью поляризации с помощью магнитных зеркал и намагниченных интерференционных фильтров.
Применения
Нейтрон играет важную роль во многих ядерных реакциях. Например, захват нейтронов часто приводит к активации нейтронов, вызывая радиоактивность. В частности, знание нейтронов и их поведения было важным при разработке ядерных реакторов и ядерного оружия. Деление таких элементов, как уран-235 и плутоний-239, вызвано их поглощением нейтронов.
Холодное, тепловое и горячее нейтронное излучение обычно используется в устройствах для рассеяния нейтронов, где излучение используется аналогичным образом, как используется X- лучи для анализа конденсированного состояния. Нейтроны дополняют последние с точки зрения атомных контрастов различными сечениями рассеяния ; чувствительность к магнетизму; диапазон энергий для неупругой нейтронной спектроскопии; и глубокое проникновение в материю.
Разработка «нейтронных линз», основанных на полном внутреннем отражении внутри полых стеклянных капиллярных трубок или отражении от алюминиевых пластин с углублениями, стимулировала продолжающиеся исследования в области нейтронной микроскопии и нейтронной / гамма-томографии.
В основном нейтроны используются для возбуждения запаздывающих и побуждающих гамма-лучей от элементов в материалах. Это составляет основу нейтронно-активационного анализа (NAA) и мгновенного активационного гамма-нейтронного анализа (PGNAA). NAA чаще всего используется для анализа небольших образцов материалов в ядерном реакторе , в то время как PGNAA чаще всего используется для анализа подземных горных пород вокруг скважин и промышленных сыпучих материалов на конвейерных лентах.
Еще одно применение нейтронных излучателей — обнаружение легких ядер, в частности водорода, содержащегося в молекулах воды. Когда быстрый нейтрон сталкивается с легким ядром, он теряет большую часть своей энергии. Измеряя скорость, с которой медленные нейтроны возвращаются в зонд после отражения от ядер водорода, нейтронный зонд может определять содержание воды в почве.
Лечебные методы лечения
Поскольку нейтронное излучение проникающее и ионизирующее, оно может быть использовано для лечения. Однако нейтронное излучение может иметь нежелательный побочный эффект, поскольку пораженный участок остается радиоактивным. Нейтронная томография поэтому не является жизнеспособным медицинским приложением.
Терапия быстрыми нейтронами использует нейтроны высокой энергии, обычно более 20 МэВ, для лечения рака. Лучевая терапия рака основана на биологической реакции клеток на ионизирующее излучение. Если излучение доставляется небольшими сеансами для повреждения раковых участков, нормальные ткани успевают восстановиться, а опухолевые клетки часто не могут. Нейтронное излучение может доставлять энергию в злокачественную область со скоростью, на порядок превышающей гамма-излучение.
Пучки нейтронов низкой энергии используются в терапии с захватом бора для лечения рака. При терапии с захватом бора пациенту дают лекарство, которое содержит бор и который предпочтительно накапливается в опухоли, на которую она направлена. Затем опухоль бомбардируется нейтронами очень низкой энергии (хотя часто с более высокой, чем тепловая энергия), которые захватываются изотопом бор-10 в боре, что создает возбужденное состояние бор-11, который затем распадается. для производства лития-7 и альфа-частицы, обладающих достаточной энергией для уничтожения злокачественной клетки, но недостаточной дальностью действия для повреждения соседних клеток. Для применения такой терапии для лечения рака предпочтительным является источник нейтронов с интенсивностью порядка тысячи миллионов (10) нейтронов в секунду на 1 см. Такие потоки требуют исследовательского ядерного реактора.
Защита
Воздействие свободных нейтронов может быть опасным, поскольку взаимодействие нейтронов с молекулами в организме может вызвать разрушение молекул и атомов, а также может вызывать реакции, вызывающие другие формы излучения (например, протоны). Применяются обычные меры радиационной защиты: избегать облучения, держаться как можно дальше от источника и сводить время воздействия к минимуму. Однако следует подумать о том, как защитить себя от воздействия нейтронов. Для других типов излучения, например, альфа-частиц, бета-частиц или гамма-лучей, материал с высоким атомным номером и высокой плотностью обеспечивает хорошее экранирование. ; часто используется отведение. Однако этот подход не будет работать с нейтронами, поскольку поглощение нейтронов не увеличивается напрямую с атомным номером, как это происходит с альфа-, бета- и гамма-излучением. Вместо этого нужно посмотреть на конкретные взаимодействия нейтронов с веществом (см. Раздел об обнаружении выше). Например, водород -содержащие материалы часто используются для защиты от нейтронов, так как обычный водород и рассеивает, и замедляет нейтроны. Это часто означает, что простые бетонные блоки или даже наполненные парафином пластиковые блоки обеспечивают лучшую защиту от нейтронов, чем гораздо более плотные материалы. После замедления нейтроны могут быть поглощены изотопом, который имеет высокое сродство к медленным нейтронам, не вызывая вторичного захватывающего излучения, такого как литий-6.
Богатая водородом обычная вода влияет на поглощение нейтронов в реакторах ядерного деления : Обычно нейтроны настолько сильно поглощаются обычной водой, что требуется обогащение топлива делящимся изотопом. дейтерий в тяжелой воде имеет гораздо более низкое сродство к поглощению нейтронов, чем протий (нормальный легкий водород). Следовательно, дейтерий используется в реакторах типа CANDU, чтобы замедлить (умеренную ) скорость нейтронов, чтобы увеличить вероятность ядерного деления по сравнению с захват нейтронов.
Температура нейтронов
Тепловые нейтроны
Тепловые нейтроны — это свободные нейтроны, энергии которых имеют распределение Максвелла – Больцмана с kT = 0,0253 эВ (4,0 × 10 Дж ) при комнатной температуре. Это дает характеристическую (не среднюю и не среднюю) скорость 2,2 км / с. Название «термический» происходит от того, что их энергия — это энергия газа или материала комнатной температуры, через которые они проникают. (энергии и скорости молекул см. в кинетической теории ). После ряда столкновений (часто в диапазоне 10–20) с ядрами нейтроны попадают на этот энергетический уровень при условии, что они не поглощаются.
Во многих веществах реакции тепловых нейтронов имеют гораздо большее эффективное сечение, чем реакции с участием более быстрых нейтронов, и поэтому тепловые нейтроны могут легче (т. Е. С большей вероятностью) поглощаться любыми атомными ядрами., с которым они сталкиваются, создавая в результате более тяжелый — и часто нестабильный — изотоп химического элемента.
В большинстве реакторов деления используется замедлитель нейтронов для замедления или термализации нейтронов, испускаемых ядерным делением, чтобы они были больше легко захватывается, вызывая дальнейшее деление. Другие, называемые реакторами на быстрых нейтронах, напрямую используют нейтроны энергии деления.
Холодные нейтроны
Холодные нейтроны — это тепловые нейтроны, уравновешенные в очень холодном веществе, таком как жидкий дейтерий. Такой источник холода помещается в замедлитель исследовательского реактора или источник скола. Холодные нейтроны особенно ценны для экспериментов по рассеянию нейтронов.

Ультрахолодные нейтроны
Ультрахолодные нейтроны получаются путем неупругого рассеяния холодные нейтроны в веществах с низким сечением поглощения нейтронов при температуре в несколько кельвинов, таких как твердый дейтерий или сверхтекучий гелий. Альтернативным методом производства является механическое замедление холодных нейтронов с использованием доплеровского сдвига.
Нейтроны с энергией деления
Быстрый нейтрон — это свободный нейтрон с уровнем кинетической энергии, близким к 1 МэВ (1,6 × 10 J ), следовательно, скорость ~ 14000 км / с (~ 5% скорости света). Их называют энергией деления или быстрыми нейтронами, чтобы отличить их от тепловых нейтронов с более низкой энергией и нейтронов высокой энергии, образующихся в космических ливнях или ускорителях. Быстрые нейтроны производятся ядерными процессами, такими как деление ядра. Нейтроны, образующиеся при делении, как отмечалось выше, имеют распределение Максвелла – Больцмана кинетических энергий от 0 до ~ 14 МэВ, среднюю энергию 2 МэВ (для нейтронов деления U) и режим . всего 0,75 МэВ, что означает, что более половины из них не квалифицируются как быстрые (и, таким образом, почти не имеют шансов инициировать деление в фертильных материалах, таких как U и Th).
Быстрые нейтроны можно превратить в тепловые нейтроны с помощью процесса, называемого замедлением. Это делается с помощью замедлителя нейтронов. В реакторах для замедления нейтронов обычно используются тяжелая вода, легкая вода или графит.
Нейтроны термоядерного синтеза

D – T (дейтерий — тритий ) термоядерный синтез — это реакция синтеза, которая производит нейтроны с наибольшей энергией, с 14,1 МэВ из кинетической энергии и движущиеся со скоростью 17% скорость света. D – T-синтез также является самой простой реакцией синтеза для воспламенения, достигающей почти пиковых скоростей, даже когда ядра дейтерия и трития имеют кинетическую энергию в тысячную раз меньше, чем 14,1 МэВ, которые будут произведены.
14,1 МэВ нейтроны имеют примерно в 10 раз больше энергии, чем нейтроны деления, и очень эффективны при делении даже не делящихся тяжелых ядер, и эти высокоэнергетические деление дает в среднем больше нейтронов, чем деление нейтронами с меньшей энергией. Это делает источники нейтронов термоядерного синтеза, такие как предлагаемые токамак энергетические реакторы, полезными для трансмутации трансурановых отходов. Нейтроны с энергией 14,1 МэВ также могут производить нейтроны, выбивая их из ядер.
. С другой стороны, эти нейтроны очень высоких энергий с меньшей вероятностью просто будут захвачены, не вызывая деления или расщепления. По этим причинам конструкция ядерного оружия широко использует D – T-синтез нейтронов с энергией 14,1 МэВ, чтобы вызвать большее деление. Нейтроны термоядерного синтеза способны вызывать деление в обычно неделящихся материалах, таких как обедненный уран (уран-238), и эти материалы использовались в оболочках термоядерного оружия. Нейтроны термоядерного синтеза также могут вызывать деление в веществах, которые непригодны или трудны для превращения в бомбы первичного деления, таких как плутоний реакторного качества. Этот физический факт, таким образом, заставляет обычные материалы, не относящиеся к оружию, вызывать озабоченность в некоторых обсуждениях и договорах ядерного распространения.
Другие реакции синтеза производят нейтроны с гораздо меньшей энергией. D – D термоядерный синтез производит нейтрон с энергией 2,45 МэВ и гелий-3 половину времени и производит тритий и протон, но не нейтрон в остальное время. Синтез D – He не производит нейтронов.
Нейтроны промежуточной энергии

Нейтрон энергии деления, который замедлился, но еще не достиг тепловой энергии называется эпитепловым нейтроном.
Сечения для реакций захвата и деления часто имеют множественные резонансные пики при определенных энергиях в надтепловом диапазоне энергий. Они имеют меньшее значение в реакторе на быстрых нейтронах, где большая часть нейтронов поглощается перед замедлением до этого диапазона, или в охлаждаемом тепловом реакторе, где надтепловые нейтроны взаимодействуют в основном с ядрами замедлителей, а не с делящимися или плодородными нуклидами актинидов. Однако в реакторе с частичным замедлителем с большим количеством взаимодействий надтепловых нейтронов с ядрами тяжелых металлов существует больше возможностей для переходных изменений реактивности, которые могут затруднить управление реактором.
Отношение реакций захвата к реакциям деления также хуже (больше захватов без деления) в большинстве ядерных топлив, таких как плутоний-239, что делает реакторы эпитеплового спектра, использующие эти виды топлива менее желательны, так как улавливает не только один нейтрон, но и обычно приводит к нуклиду, который не делящийся с тепловыми или надтепловыми нейтронами, но все же делится с быстрыми нейтронами. Исключением является уран-233 из ториевого цикла, который имеет хорошие отношения захвата-деления при всех энергиях нейтронов.
Нейтроны высоких энергий
Нейтроны высоких энергий имеют гораздо большую энергию, чем нейтроны энергии деления, и генерируются в виде вторичных частиц ускорителями частиц или в атмосфере из космические лучи. Эти нейтроны высокой энергии чрезвычайно эффективны при ионизации и с гораздо большей вероятностью могут вызвать смерть клетки, чем рентгеновские лучи или протоны.
См. также
| Викискладе есть материалы, связанные с нейтронами. |
- Ионизирующим излучением
- Изотопом
- Список частиц
- Магнитным моментом нейтрона
- Нейтронным излучением и Шкала излучения Сиверта
- Нейтроний
- Ядерная реакция
- Нуклеосинтез
- Нуклеосинтез нейтронного захвата
- R-процесс
- S-процесс
- Тепловой реактор
Источники нейтронов
- Генератор нейтронов
- Источник нейтронов
Процессы с участием нейтронов
- Нейтронная бомба
- Дифракция нейтронов
- Поток нейтронов
- Транспорт нейтронов
- Датирование космогенных радионуклидов
Ссылки
Дополнительная литература
- Джеймс Бирн, Нейтроны, ядра и материя: исследование физики медленных нейтронов. Mineola, New York: Dover Publications, 2011. ISBN 0486482383.
- Abraham Pais, Inward Bound, Oxford: Oxford University Press, 1986. ISBN 0198519974.
- Син-Итиро Томонага, The Story of Spin, The University of Chicago Press, 1997
- Хервиг Шоппер, Слабые взаимодействия и бета-распад ядер, Издательство, Север -Holland Pub. Co., 1966.
- Аннотированная библиография по нейтронам из Цифровой библиотеки по ядерным вопросам Алсос
Нейтрон — элементарная частица, не имеющая электрического заряда.
Константы нейтрона.
| Название | Значение | Обозначение | Размерность |
| Масса покоя | 1.67492861 | тп | 10-27 кг |
| Масса покоя в атомных единицах массы | 1.00866490414 | тп | а.е.м. |
| Масса покоя в электронвольтах | 939.5656328 | тп – тпс2/{е} | МэВ |
| Отношение массы нейтрона к массе электрона | 1838.6836624 | mp/me | МэВ |
| Отношение массы нейтрона к массе протона | 1.0013784049 | mn/mp | МэВ |
| Молярная масса | 1.00866490414 | М(п) | 10-3 кг/моль |
| Комптоновская длина волны | 1.3195911012 | λcn – h/mn | 10-15M |
| Магнитный момент | 0.966237074 | μn /μb | 10-16м |
| Магнитный момент нейтрона в магнетонах Бора | 1.0418756325 | μn /μN | 10-26 Дж×Тл-1 |
| Магнитный момент нейтрона в ядерных магнетонах | 1.9130427545 | μn /μN | 10–3 |
| Отношение магнитного момента нейтрона к магнитному моменту электрона | 1.0406688225 | μn /μb | 10–3 |
| Отношение магнитного момента нейтрона к магнитному моменту протона | 0.684 | μn /μΡ | 10–3 |
Нейтрон – элементарная частица, которая собственным электрическим зарядом не обладает. Отсюда и название этой частицы, которая с латинского языка переводится дословно «ни тот, ни другой». Из определенного числа нейтронов (а также из протонов) состоят атомные ядра.
Нейтрон: открытие
Впервые о существовании нейтрона люди узнали в 1932 году. Случилось это благодаря ученому Дж. Чедвику. Важный вклад в науку был оценен, и спустя три года физик получил за свое открытие Нобелевскую премию.
Но еще в 1930 году ученые Д. Иваненко и В. Амбарцумян на опытах доказали, что ядро молекулы из протонов и электронов не состоит (а именно так считалось ранее). Они показали, что электроны, которые отделяются от ядра в момент бета-распада, собственно в этот момент и рождаются. Так что, помимо протонов, справедливо считали ученые, в ядре присутствуют нейтрально заряженные (а именно, не заряженные вовсе) частицы.
Нейтрон: данные
Масса нейтрона больше, нежели протоновая масса, примерно на 0, 14 процентов. Спин нейтрона 12. Период полураспада нейтрона – 614 секунд. А время жизни нейтрона в свободном состоянии примерно 885, 8 секунды.
Хот нейтрон и не обладает электрическим зарядом, подлинно нейтральной атомной частицей он не является. У него есть антипод или античастица – соответственно, антинейтрон.
Кварковая структура нейтрона
50. Открытие нейтрона [9:26]
Нейтрон — это субатомная частица, которая не имеет электрического заряда, а масса чуть больше массы протона.
Ядра всех атомов состоят из протонов и нейтронов, которые имеют общее название — нуклоны. Количество протонов в ядре определяет атомный номер, а значит и химический элемент. Количество нейтронов определяет изотоп химического элемента. Например, углерод-12 имеет 6 протонов и 6 нейтронов, в то время как углерод-14 имеет 6 протонов и 8 нейтронов.
Нейтрон состоит из двух нижних кварков и одного верхнего кварка, и поэтому он является барионом и имеет спин ½. Масса нейтрона составляет 939 573 МэВ, либо 1.008 664 915 (78) а.е.м., либо 1,6749 × 10−27. Античастицей нейтрона является антинейтрон.
Стабильность нейтрона и бета-распад[править]
Если нейтрон находится за пределами ядра (свободный нейтрон), он является нестабильным и его время жизни составляет 885.7 ± 0.8 секунд (около 15 минут), при этом, чтобы стать протоном, нейтрон выпускает электрон и антинейтрин: [math]displaystyle{ hbox{n}tohbox{p}+hbox{e}^-+overline{nu}_{mathrm{e}} }[/math]. Этот вид распада, известный как бета-распад, возможен также и для нейтрона, что находится внутри нестабильных ядер.
Протоны, содержащиеся внутри ядра, также могут трансформироваться в нейтроны путем бета-распада. В этом случае, превращение сопровождается эмиссией позитронов (антиэлектрона) и нейтрино (вместо антинейтрина): [math]displaystyle{ hbox{p}tohbox{n}+hbox{e}^{+}+{nu}_{mathrm{e}} }[/math]. Превращение протона в нейтрон внутри ядра возможно и путем захвата электрона (электронный захват): [math]displaystyle{ hbox{p}+hbox{e}^{-}tohbox{n}+{nu}_{mathrm{e}} }[/math]. Захват нейтронов позитронов в ядрах (позитронный захват), что имеют избыточные нейтроны, также возможен, однако маловероятен, ведь позитроны отталкиваются ядрами, и, более того, быстро аннигилируют, когда встречают отрицательные электроны.
Если нейтроны удерживаются внутри ядра, неустойчивость отдельного нейтрона уравновешивается неустойчивостью, которой будет обладать атом в целом, если возникнет дополнительный протон, который вступит во взаимодействие отталкивания с другими протонами, которые уже существовали в ядре. Поэтому, когда свободные нейтроны являются нестабильными, связанные нейтроны необязательно являются неустойчивыми. Аналогичным образом можно объяснить, почему протоны, которые являются стабильными в пустом пространстве, могут превращаться в нейтроны, когда находятся в ядре.
Бета-распад и электронный захват являются типами радиоактивного распада и оба происходят благодаря слабому взаимодействию.
Взаимодействие[править]
Нейтрон участвует во всех четырех типах фундаментальных взаимодействий: электромагнитном, слабом, сильном и гравитационным взаимодействиях.
Гравитация действует на нейтрон, как и на любое энергетическое тело, однако гравитация настолько слаба, что ее можно не учитывать при экспериментах по физике частиц.
Самым значимым для нейтрона является сильное взаимодействие. Это взаимодействие отвечает за удерживание трех кварков у отдельной частицы. Остаточная мощная сила ответственна за удерживание нейтронов и протонов вместе в ядрах. Эта ядерная сила играет первостепенную роль, когда нейтроны проходят через материю. В отличие от заряженных частиц или фотонов, нейтрон не может терять энергию благодаря ионизации атомов. Наоборот, нейтрон беспрепятственно движется к лобовому столкновению с атомным ядром. Из-за этого нейтронное излучение является чрезвычайно проникающим.
Регистрация[править]
Общие способы регистрации заряженных частиц, если смотреть след ионизации (например в камере Вильсона) не подходят для нейтронов напрямую. Нейтроны, что упруго рассеиваются на атомах, могут оставлять ионизационный след, который можно зарегистрировать, но не так просто осуществить такой эксперимент; обычно используют другие методы регистрации нейтронов, они основаны на взаимодействии нейтронов с атомными ядрами.
Общий метод регистрации нейтронов заключается в превращении выделенной в ходе реакций энергии в электрические сигналы. Для такой цели полезными являются изотопы ³He, 6Li, 10B, 233U, 235U, 237Np и 239Pu.
Использование[править]
Нейтроны играют важную роль во многих ядерных реакциях. Например, нейтронный захват часто приводит к нейтронному возбуждению, вызывая радиоактивность. В частности, знания о нейтронах и их поведении важны при разработке ядерных реакторов и ядерного оружия.
Ссылки[править]
- Экспериментальные свойства нейтронов (сайт Particle Data Group, англ.).
- CODATA Internationally recommended values of the Fundamental Physical Constants (2010).
(n) (от лат. neuter — ни тот, ни другой) — элементарная частица с нулевым электрич. зарядом и массой, незначительно большей массы протона. Наряду с протоном под общим назв. нуклон входит в состав атомных ядер. H. имеет спин 1/2 и, следовательно, подчиняется Ферми — Дирака статистике (является фермионом). Принадлежит к семейству адра-нов; обладает барионным числом B=1, т. е. входит в группу барионов.
Открыт в 1932 Дж. Чедвиком (J. Chadwick), показавшим, что жёсткое проникающее излучение, возникающее при бомбардировке ядер бериллия a-частицами, состоит из электрически нейтральных частиц с массой, примерно равной протонной. В 1932 Д. Д. Иваненко и В. Гей-зенберг (W. Heisenberg) выдвинули гипотезу о том, что атомные ядра состоят из протонов и H. В отличие от заряж. частиц, H. легко проникает в ядра при любой энергии и с большой вероятностью вызывает ядерные реакции захвата (n,g), (n,a), (n, p), если баланс энергии в реакции положительный. Вероятность экзотермич. ядерной реакции увеличивается при замедлении H. обратно пропорц. его скорости. Увеличение вероятности реакций захвата H. при их замедлении в водородсодержащих средах было обнаружено Э. Ферми (E. Fermi) с сотрудниками в 1934. Способность H. вызывать деление тяжёлых ядер, открытая О. Ганом (О. Hahn) и Ф. Штрасманом (F. Strassman) в 1938 (см. Деление ядер), послужила основой для создания ядерного оружия и ядерной энергетики. Своеобразие взаимодействия с веществом медленных H., имеющих де-бройлевскую длину волны порядка атомных расстояний (резонансные эффекты, дифракция и т. д.), служит основой широкого использования нейтронных пучков в физике твёрдого тела. (Классификацию H. по энергиям — быстрые, медленные, тепловые, холодные, ультрахолодные — см. в ст. Нейтронная физика.)
В свободном состоянии H. нестабилен — испытывает B-распад; n 
Основные характеристики H.
Масса H. т п =939,5731(27) МэВ/с 2 = = 1,008664967(34) ат. ед. массы 


Электрический заряд H. Qn =0. Наиболее точные прямые измерения Qn выполнены по отклонению пучков холодных либо ультрахолодных H. в электростатич. поле: Qn<= 3·10-21 е (е — заряд электрона). Косв. данные по электрич. нейтральности мак-роскопич. кол-ва газа дают Qn <= 2·10-22 е.
Спин H. J = 1/2 был определён из прямых опытов по расщеплению пучка H. в неоднородном магн. поле на две компоненты [в общем случае число компонент равно (2J + 1)].
Внутренняя чётностьH. положительная. Изотопический спин I =1/2, при этом проекция изотопич. спина H. I3 = —1/2. В рамках SU(3)-симметрии H. входит в октет барионов (см. Унитарная симметрия).
Магнитный момент H. Несмотря на электронейтральность H., его магн. момент существенно отличен от нуля: mn = — 1,91304184(88)m Я, где m Я = е

Электрический дипольный момент H. Динамический, т. е. индуцированный, ди-польный момент H. может возникать в сильном электрич. поле, напр. при рассеянии H. на тяжёлом ядре, либо при рассеянии g-квантов на дейтроне. Изменение энергии частицы в электрич. поле определяется соотношением D

Статич. электрич. дипольный момент (ЭДМ) элементарной частицы должен быть тождественно равен нулю, если взаимодействия, к-рые она испытывает, инвариантны относительно обращения времени(T -инвариант-ны). ЭДМ отличен от нуля, если T -инвариантность нарушена, что, согласно теореме CPT (т. зарядового сопряжения, пространственной инверсии и обращения времени), эквивалентно нарушению СР -ин-вариантности. Хотя нарушение СР -инвариантности было обнаружено ещё в 1964 в распаде K0L -мезона, до сих пор СР -неинвариантные эффекты для др. частиц (или систем) не наблюдались. В совр. объединённых калибровочных теориях элементарных частиц нарушение T (или CP )-инвариантности может иметь место в электрослабом взаимодействии, хотя величина эффекта крайне мала. Разл. модели нарушения СР -инвариант-ности предсказывают величину ЭДМ H. на уровне (10-24-10-32) е . см. Из-за своей электрич. нейтральности H.- весьма удобный объект для поисков СР -не-инвариантности. Наиб. чувствительный и надёжный метод — метод ЯМР с электрич. полем, наложенным на магн. иоле. Изменение направления электрич. поля при сохранении всех остальных характеристик резонансного спектрометра ЯМР вызывает смещение частоты ЯМР на величину Dv = —4dЕ, где d — ЭДМ. Для d ~10-25 е . см Dv ~10-6 Гц. Используя метод удержания ультрахолодных H. в ЯМР-спектрометре, удаётся достичь такой чувствительности. Полученное наиб. точное ограничение на ЭДМ H.: dn<= 2·10-25 е . см .
Структура H.
H. наряду с протоном принадлежит к легчайшим барионам. По совр. представлениям, ои состоит из трёх легчайших валентных кварков (двух d -кварков и одного u -кварка) трёх цветов, образующих бесцветную комбинацию. Кроме валентных кварков и связывающих их глюонов нуклон содержит «море» виртуальных пар кварк — антикварк, в т. ч. тяжёлых (странных, очарованных и т. д.). Квантовые числа H. целиком определяются набором валентных кварков, а пространств. структура — динамикой взаимодействия кварков и глюонов. Особенностью этого взаимодействия является рост эфф. константы взаимодействия ( эффективного заряда )с увеличением расстояния, так что размер области взаимодействия ограничен областью т. н. кон-файнмента кварков — областью невылетания цветных объектов, радиус которой ~10-13 см (см. Удержание цвета).
Последоват. описание структуры адронов на основе совр. теории сильного взаимодействия — квантовой хромодинамики — пока встречает теоретич. трудности, однако для мн. задач вполне удовлетворит. результаты даёт описание взаимодействия нуклонов, представляемых как элементарные объекты, посредством обмена мезонами. Эксперим. исследование пространств. структуры H. выполняется с помощью рассеяния высокоэнергичных лептонов (электронов, мюонов, нейтрино, рассматриваемых в совр. теории как точечные частицы) на дейтронах. Вклад рассеяния на протоне измеряется в отд. эксперименте и может быть вычтен с помощью определ. вычислит. процедуры.
Упругое и квазиупругое (с расщеплением дейтрона) рассеяние электронов на дейтроне позволяет найти распределение плотности электрич. заряда и магн. момента H. (формфакторH.). Согласно эксперименту, распределение плотности магн. момента H. с точностью порядка неск. процентов совпадает с распределением плотности электрич. заряда протона и имеет среднеквадратичный радиус ~0,8·10-13 см (0,8 Ф). Магн. форм-фактор H. довольно хорошо описывается т. н. диполь-ной ф-лой GMn= mn(1 + q2/0,71)-2, где q2— квадрат переданного импульса в единицах (ГэВ/с)2.
Более сложен вопрос о величине электрич. (зарядового) формфактора H. GEn. Из экспериментов по рассеянию на дейтроне можно сделать заключение, что GEn (q2) <= 0,1 в интервале квадратов переданных импульсов (0-1) (ГэВ/с)2. При q2
В глубоко неупругом процессе рассеяния (взаимодействия с рождением многих вторичных адронов, преим. пионов) налетающая точечная частица (лептон) взаимодействует непосредственно с точечными компонентами нуклона — кварками. Кварковый состав H. (ddu )наиб. наглядно выявляется в экспериментах с взаимодействием нейтрино и антинейтрино высоких энергий с протонной и нейтронной (в составе дейтерия) мишенями. Напр., полное сечение s реакции vmn 


Взаимодействия H.
Сильное взаимодействие H. с нуклонами. Следствием изотопич. инвариантности является равенство сечений нейтрон-нейтронного и протон-протонного взаимодействия, если в последнем случае учесть вклад кулонов-ского взаимодействия. На кварк-глюонном уровне изотопич. симметрия является следствием малой разности масс d- и u -кварков (при малости самой массы кварков). Этим же объясняется малость разности масс протона и H., а также величина и знак этой разности (d- кварк тяжелее u -кварка).
При низких энергиях (до 15 МэВ) рассеяние H. на протоне изотропно в системе центра масс, т. е. взаимодействие определяется в осн. S -волной (относит. движением с орбит. моментом L= 0). Для S -волнового взаимодействия сечение рассеяния может быть охарактеризовано двумя параметрами — эфф. радиусом потенциала взаимодействия и длиной рассеяния. Зависимость от относит. направления спинов H. и протона удваивает число параметров, т. к. длины рассеяния для синглетного (полный спин системы 0) и триплетного (полный спин 1) состояний различны (отличаются в неск. раз). Совр. значения длин рассеяния и эфф. радиусов (в Ф): = 1,70(3), ros = 2,67(3). Параметры np-рассеяния не могут быть непосредственно сопоставлены с рр- и nn-рассеянием, поскольку системы рр и nn в соответствии с Паули принципом не могут находиться в триплетом состоянии. Синглетная длина рр-рассеяния равна: а рр = -7,815(8) Ф, r0 = 2,758 Ф. Расчёт кулоновского вклада в a рр позволяет получить чисто ядерную длину рр-рассеяния a яpp , к-рая оказывается равной -17,25 Ф. Согласно изотопич. инвариантности, а яpp = аnn. Определение параметров nn-рассеяния — сложная проблема, т. к. прямое взаимодействие свободных H. до сих пор не наблюдалось из-за трудности эксперимента. Предложено неск. вариантов эксперимента по поиску прямого nn-рассеяния в пучках высокопоточных импульсных или стационарных реакторов.
Наиб. определённые сведения об а пп. получены при исследовании реакции p-d 

На раннем этапе развития ядерной физики большую роль для понимания свойств ядерных сил сыграли осн. характеристики дейтрона. Дейтрон является связанным триплетным состоянием пр с энергией связи -2,224 МэВ. Синглетное состояние пр имеет положит. энергию связи 64 кэВ и является резонансом. Др. ре-зонансов и связанных состояний в области низких энергий в np-системе нет. Эти два параметра позволяют определить потенциал нуклон-нуклонного взаимодействия и радиус ядерных сил. Наличие у дейтрона квад-рупольного электрич. момента Q =2,859.10-27 см 2 приводит к выводу о существовании тензорных ядерных сил.
Радиац. захват H. протоном, nр 

Изотопич. инвариантность ядерных сил и известная энергия связи синглетного np-состояния позволяют обосновать отсутствие связанного nn-состояния (ди-нейтрона). Эксперим. поиски такого состояния в реакциях типа А + В 
При больших энергиях нуклон-нуклонного взаимодействия его характер меняется. При энергиях падающих нуклонов (200-400) МэВ, соответствующих их сближению на расстояния ~0,3 Ф, во взаимодействии проявляются отталкиват. силы. Это явление обычно сопоставляется с существованием жёсткой отталкивающей сердцевины (кора) у нуклонов и приписывается доминирующей роли на малых расстояниях обмена тяжёлыми векторными мезонами, напр. w-мезонами. Такое объяснение не единственно возможное. В модели «кварковых мешков» (см. Кварковые модели )это же явление объясняется слиянием на малых расстояниях двух нуклонов в один шестикварковый мешок, свойства к-рого качественно отличаются от свойств индивидуальных нуклонов; это приводит к тому, что экспериментально не наблюдаются два индивидуальных нуклона на малых расстояниях.
При более высоких энергиях взаимодействия становятся существенно неупругими и сопровождаются множеств. рождением p-мезонов и более тяжёлых частиц (см. Множественные процессы). Свойства кварков и глюонов при этом играют определяющую роль в динамике взаимодействия, вызывая образование струй вторичных адронов (см. Струя адронная )и др.
Взаимодействие H. с ядрами и с веществом. Как и при взаимодействии с протоном, взаимодействие H. с ядрами описывается достаточно короткодействующими силами по сравнению с де-бройлевской длиной волны H. Для малых энергий 

Для медленных H. определяющими становятся его волновые свойства, когерентное взаимодействие с упорядоченными конденсиров. средами. H. с длиной волны, близкой к межатомным расстояниям, являются важнейшим средством исследования структуры твёрдых тел и динамики возбуждения в них. Наличие у H. магн. момента делает пучки поляризов. H. чрезвычайно чувствит. инструментом для исследования распределения намагниченности в веществе (см. Нейтронография).
Особенностью взаимодействия H. с большинством ядер является положит. длина рассеяния, что приводит к коэф. преломления < 1. Благодаря этому H., падающие из вакуума на границу вещества, могут испытывать полное внутр. отражение. При скорости u.< (5-8) м/с (ультрахолодные H.) H. испытывают полное отражение от границы с углеродом, никелем, бериллием и др. при любом угле падения и могут удерживаться в замкнутых объёмах. Это свойство ультрахолодных H. широко используется в экспериментах (напр., для поиска ЭДМ H.) и позволяет реализовать нейтронооптич. устройства (см. Нейтронная оптика).
H. и слабое (электрослабое) взаимодействие. Важным источником сведений об электрослабом взаимодействии является b-распад свободного H. 



Распад свободного H. с учётом кинематич. параметров описывается двумя константами — векторной GV, являющейся вследствие векторного тока сохранения универс. константой слабого взаимодействия, и аксиально-векторной GA, величина к-рой определяется динамикой сильно взаимодействующих компонент нуклона — кварков и глюонов. Волновые ф-ции начального H. и конечного протона и матричный элемент перехода n 
Время жизни H. без учёта нек-рых поправок равно: tn = k(G2V + 3G2A)-1, где k включает кинематич. факторы и зависящие от граничной энергии b-распада кулонов-ские поправки и радиационные поправки.
Вероятность распада поляризов. H. со спином S, энергиями и импульсами электрона и антинейтрино 

Коэф. корреляции a, А, В, D могут быть представлены в виде ф-ции от параметра а =(GA/GV,)exp(if). Фаза f отлична от нуля или p, если T -инвариантность нарушена. В табл. приведены эксперим. значения для этих коэф. и вытекающие из них значения a и f.
Имеется заметное отличие данных разл. экспериментов для т n, достигающее неск. процентов.
Описание электрослабого взаимодействия с участием H. при более высоких энергиях гораздо сложнее из-за необходимости учитывать структуру нуклонов. Напр., m— -захват, m—p 
1) Распады гиперонов L 



2) Слабое взаимодействие n — n или n — p, к-рое проявляется как ядерные силы, не сохраняющие пространств. чётность. Обычная величина обусловленных ими эффектов порядка 10-6-10-7.
Взаимодействие H. со средними и тяжёлыми ядрами имеет ряд особенностей, приводящих в нек-рых случаях к значит. усилению эффектов несохранения чётности в ядрах. Один из таких эффектов — относит. разность сечения поглощения H. с поляризацией по направлению распространения и против него, к-рая в случае ядра 139La равна 7% при 
Взаимодействия с нарушением барионного числа. Теоретич. модели великого объединения и суперобъединения предсказывают нестабильность барионов — их распад в лептоны и мезоны. Эти распады могут быть заметны только для легчайших барионов — p и п, входящих в состав атомных ядер. Для взаимодействия с изменением барионного числа на 1, DB = 1, можно было бы ожидать превращения H. типа: n 

Др. возможный тип взаимодействия с D В = 2 может привести к явлению взаимопревращения H. и антинейтронов в вакууме, т. е. к осцилляции 


Прямые эксперименты по наблюдению рождения и в пучках холодных H. от высокопоточного реактора дают ограничение t осц > 107 с. В готовящихся экспериментах можно ожидать увеличения чувствительности до уровня t осц ~ 109 с. Ограничивающими обстоятельствами являются макс. интенсивность пучков H. и имитация явлений аннигиляции антинейтронов в детекторе космич. лучами.
Др. метод наблюдения осцилляции 
Следует отметить, что поиски распада нуклона с DB = 1 и поиски 
Гравитационное взаимодействие H. Нейтрон — одна из немногих элементарных частиц, падение к-рой в гравитац. поле Земли можно наблюдать экспериментально. Прямое измерение ускорения свободного падения для H. выполнено с точностью 0,3% и не отличается от макроскопического. Актуальным остаётся вопрос о соблюдении эквивалентности принципа (равенства инертной и гравитац. масс) для H. и протонов.
Самые точные эксперименты выполнены методом Эт-веша для тел, имеющих разные ср. значения отношения A/Z, где А — ат. номер, Z — заряд ядер (в ед. элементарного заряда е). Из этих опытов следует одинаковость ускорения свободного падения для H. и протонов на уровне 2·10-9, а равенство гравитац. и инертной масс на уровне ~10-12.
Гравитац. ускорение и замедление широко используются в опытах с ультрахолодными H. Применение гравитац. рефрактометра для холодных и ультрахолодных H. позволяет с большой точностью измерить длины когерентного рассеяния H. на веществе.
H. в космологии и астрофизике
Согласно совр. представлениям, в модели Горячей Вселенной (см. Горячей Вселенной теория )образование барионов, в т. ч. протонов и H., происходит в первые минуты жизни Вселенной. В дальнейшем нек-рая часть H., не успевших распасться, захватывается протонами с образованием 4He. Соотношение водорода и 4He при этом составляет по массе 70% к 30%. При формировании звёзд и их эволюции происходит дальнейший нуклеосинтез, вплоть до ядер железа. Образование более тяжёлых ядер происходит в результате взрывов сверхновых с рождением нейтронных звёзд, создающих возможность последоват. захвата H. нуклидами. При этом комбинация т. н. s -процесса — медленного захвата H. с b-распадом между последовательными захватами и r -процесса — быстрого последоват. захвата при взрывах звёзд в осн. может объяснить наблюдаемую распространённость элементов в космич. объектах.
В первичной компоненте космич. лучей H. из-за своей нестабильности вероятно отсутствуют. H., образующиеся у поверхности Земли, диффундирующие в космич. пространство и распадающиеся там, по-видимому, вносят вклад в формирование электронной и протонной компоненты радиационных поясов Земли.
Лит.: Гуревич И. С., Тарасов Л. В., Физика нейтронов низких энергий, M., 1965; Александров Ю. А.,. Фундаментальные свойства нейтрона, 2 изд., M., 1982.
В. M. Лобашов.
Физическая энциклопедия. В 5-ти томах. — М.: Советская энциклопедия.Главный редактор А. М. Прохоров.1988.






