Исаак Ньютон — биография
Исаак Ньютон – математик, физик, астроном, механик. Сформулировал закон о всемирном тяготении, автор трех законов механики, вошедших в основу классической механики. Ему принадлежит разработка интегрального и дифференциального исчисления и теория цвета.
Исаака Ньютона считают величайшим светилом научного мира. Он прославился в физике и математике, открыл закон гравитации, движения и исчисления. И это кроме основной деятельности. Родившись в семье неграмотных крестьян, он собственным умом постиг тайны Вселенной, стал одним из создателей классической физики. Отличался скрытностью и замкнутым характером, некоторые свои открытия он так и не продемонстрировал своим современникам.
Детство
Родился Исаак Ньютон 4 января 1643 года (по юлианскому календарю) в деревне Вулсторп, расположенной в графстве Линкольншир в Великобритании. Мальчик родился недоношенным в самый канун Рождества, и потом считал это хорошей приметой. А пока он был хилым и слабым ребенком, у которого было мало шансов на выживание. Его долго не крестили, потому что не были уверены, что он вообще выживет. Однако мальчишка оказался на удивление живучим, он не только выкарабкался, но и сумел дожить до глубокой старости. Ньютон умер в 84, и это было скорее исключением, чем правилом в семнадцатом веке.
Своего отца мальчик не знал, Исаак Ньютон-старший умер за несколько месяцев до рождения сына. Новорожденного назвали в честь отца, достаточно состоятельного и успешного мелкого фермера. После того, как он умер, жена унаследовала поля и лесные угодия с плодородной землей. А еще ей досталась баснословная по тем временам сумма – пятьсот фунтов стерлингов.
Мама мальчика – Анна Эйскоу, вскоре устроила свою личную жизнь. Ее мужем стал богатый священник Варнава Смит, который не питал нежных чувств к своему трехлетнему пасынку. Мать с ее новым мужем переехали в другую деревню, а Исаак остался на попечении бабушки, а потом дяди Уильяма Эйскоу. Вскоре один за другим у Анны и Варнавы родилось трое детей.
Исаак рос разносторонне развитым ребенком. Ему нравилась поэзия, живопись, он трудился над изобретением ветряной мельницы и водяных часов, часами возился с бумажными змеями. Мальчик по-прежнему не отличался богатырским здоровьем и не любил общаться со сверстниками. Вместо веселых игр во дворе он проводил время в уединении, предпочитая заниматься тем, что представляло для него интерес.
В школе Исаак никак не мог подружиться со сверстниками, к тому же часто болел и пропускал занятия. Все это раздражало его одноклассников, и однажды они избили его до полусмерти. Это было большим унижением, и ответить кулаками своим обидчикам Ньютон не мог, потому что никогда не был силачом. Тогда он решил завоевать уважение своим умом.
До этого происшествия Исаак учился очень плохо, из-за чего его не любили учителя. После драки он всерьез взялся за учебу, постепенно приобрел себе славу лучшего ученика. Теперь его все больше интересовала математика, техника и необъяснимые явления в природе.
К шестнадцатилетию старшего сына мать снова овдовела, и ей самой было трудно управляться с хозяйством. Она привезла Исаака в родное поместье, в надежде на то, что он поможет ей вести домашние дела. Но, в то время Ньютон уже был серьезно увлечен конструированием разных механизмов, много читал и даже сочинял стихи.
Мать это очень раздражало, а тут еще друзья и родственники начали уговаривать ее дать согласие на то, чтобы парень продолжал учебу. Так, с помощью школьного учителя мистера Стокса, родного дяди Уильяма Эйскоу и знакомого Хэмфри Бабингтона, Исаак смог в 1661-м окончить школу и стать студентом Кембриджского университета.
Научная карьера
В вузе Исаак учился в статусе «sizar». Это человек, который учится бесплатно, но за это задействуется в разноплановых работах, в том числе и в помощи обеспеченным студентам. Ньютону не нравилось его положение, но он собрал все свое мужество и справился. Он был таким же нелюдимым, как и раньше, у него абсолютно не было друзей.
В те времена в Кембриджском университете учили естествознание и философию, опираясь на учения Аристотеля, несмотря на то, что уже было известно об открытиях Галлилея, Коперника и Кеплера. Ньютон много читал, он живо интересовался всеми новинками в мире астрономии, математики, фонетики и оптики. Молодой человек изучал даже теорию музыки, в общем, все, что было новым и попадалось ему под руку. Ему так нравилось это занятие, что он иногда не мог вспомнить, спал ли он, и что ел.
В 1664-м Исаак Ньютон начал самостоятельно трудиться. Он выделил основные проблемы человека и природы, которых насчитывалось сорок пять, и которые никто до него не пытался решить. Биография студента изменилась в том же году, после того, как в его жизни появился талантливый математик Исаак Барроу, преподаватель математической кафедры вуза. Спустя некоторое время Барроу стал учителем Ньютона и по совместительству одним из малочисленных друзей ученого.
Барроу сумел привить Ньютону любовь к математике, он стал серьезно заниматься этой наукой. Вскоре он уже мог похвастаться своим первым открытием в области математики – биноминальным разложением для производного рационального показателя. В это же время Ньютон стал бакалавром.
С 1665 по 1667 годы Исаак жил в родовом поместье в Вусторпе. Тогда Англия находилась во власти бубонной чумы, воевала с Голландией, и поэтому университет закрыли. Однако и дома он не прекращает своих научных изысканий. Основной интерес в те годы для Ньютона представляла оптика. Его интересовал вопрос преодоления хроматической аберрации в линзовых телескопах, и изучение этого явления привело его к открытию дисперсии. Он ставил эксперименты для познания физической природы света. Его опыты и сейчас проводят во многих вузах.
В итоге Исаак открыл корпускулярную модель света, он понял, что это поток частиц, вылетающий из источника света и прямолинейно двигающийся к ближайшему препятствию. Эта модель была очень далека от объективности, но стала основой в классической физике. Именно благодаря ей, потом сформировались современные понятия о физике явлений.
В то же время Ньютон открыл свой самый известный закон – о всемирном тяготении. Однако опубликован он был спустя несколько десятилетий, потому что Ньютона больше интересовал сам процесс, а не слава.
Любители любопытных фактов придерживаются мнения, что в открытии этого закона Ньютону помогло упавшее на голову яблоко. На самом деле ученый долго шел к этому открытию, проделывал опыты, записывал все в журнал.
Результатом долгого и кропотливого труда и стало это открытие. А вот легенда об упавшем на голову ученого яблоке принадлежит перу философа Вольтера.
Могут быть знакомы
Светило науки
После возвращения в конце 1660-х в Кембридж, Исаак Ньютон стал магистром. Теперь ему полагалась собственная комната и группа молодых студентов, которым он преподавал математику. Однако Исаак не очень любил преподавательскую деятельность, его больше интересовали научные разработки. Студенты это быстро «просекли» и стали прогуливать его лекции. Случалось такое, что аудитория была абсолютно пустой во время его урока. Зато Ньютон отметился изобретением телескопа-рефлектора, благодаря которому стал членом Лондонского королевского общества. Благодаря его изобретению, стали возможными большие открытия в астрономии.
В 1687-м в печать попала самая важная из всех работ ученого – книга, которую он назвал «Математические начала натуральной философии». Ньютон и до этого уже печатался, но именно этот труд имел очень большое значение – благодаря ему возникла рациональная механика и все математическое естествознание. Этот труд состоял из закона всемирного тяготения, трех уже знакомых законов механики, которые стали основой классической физики, ключевых понятий в физике.
Математический и физический уровень труда Ньютона превосходили все то, что до него открыли другие ученые в этой области. Работа не содержала недоказанную метафизику, в ней отсутствовали пространные рассуждения, необоснованные законы и расплывчатые формулировки, которых придерживались в своих трудах Декарт и Аристотель.
В 1699-м в Кембриджском университете студентов учили по системе мира Ньютона. В это время ученый занимал административные должности.
Личная жизнь и смерть
Выдающийся ученый был слишком занят своими изысканиями, он иногда забывал поесть и поспать, не говоря уже о женщинах. У него полностью отсутствовала личная жизнь, только бесконечное служение науке. Ученый не был женат, и наследников после себя не оставил.
Здоровье Ньютона резко пошатнулось в 1725 году. Он переехал в Кенсингтон рядом с Лондоном, где и умер 31 марта 1727 года. Ученый не оставил письменное завещание, но буквально перед смертью большую часть своего состояния отдал близким родственникам. Хоронили Ньютона с большими почестями. Местом его упокоения стало Вестминстерское аббатство, по соседству с королями и выдающимися общественными деятелями.
Основные труды
- «Новая теория света и цветов»
- «Движение тел по орбите»
- «Математические начала натуральной философии»
- «Оптика или трактат об отражениях, преломлениях, изгибаниях и цветах света»
- «О квадратуре кривых»
- «Перечисление линий третьего порядка»
- «Универсальная арифметика»
- «Анализ с помощью уравнений с бесконечным числом членов»
- «Метод разностей»
Ссылки
- Страница в Википедии
If you have found a spelling error, please, notify us by selecting that text and tap on selected text.
Биография Ньютона
4 Января 1643 – 31 Марта 1727 гг. (84 года)
4.3
Средняя оценка: 4.3
Всего получено оценок: 625.
Исаак Ньютон (1642–1727 гг.) – выдающийся английский ученый, один из создателей классической физики. Биография Ньютона богата во всех смыслах этого слова. Он сделал немало открытий в области физики, астрономии, механики и математики, в том числе открыл закон всемирного тяготения.
Детские и юные годы
Исаак Ньютон родился 25 декабря 1642 (или 4 января 1643 г. по грегорианскому календарю) в деревне Вулсторп, графство Линкольншир.
Юный Исаак, по свидетельству современников, отличался мрачным, замкнутым характером. Мальчишеским шалостям и проказам он предпочитал чтение книг и изготовление примитивных технических игрушек.
Когда Исааку исполнилось 12 лет, он поступил на обучение в Грэнтемскую школу. Незаурядные способности будущего ученого обнаружились именно там.
В 1659 г., по настоянию матери, Ньютон был вынужден вернуться домой, чтобы вести фермерское хозяйство. Но благодаря усилиям учителей, сумевших разглядеть будущий гений, он вернулся в школу. В 1661 г. Ньютон продолжил образование в Кембриджском университете.
Обучение в колледже
В апреле 1664 г. Ньютон успешно сдал экзамены и приобрел более высокую студенческую ступень. Во время обучения он активно интересовался работами Г. Галилея, Н. Коперника, а также атомистической теорией Гассенди.
Весной 1663 г. на новой, математической кафедре начались лекции И. Барроу. Известный математик и крупный ученый позже стал близким другом Ньютона. Именно благодаря ему у Исаака возрос интерес к математике.
Во время обучения в колледже Ньютон пришел к своему основному математическому методу – разложению функции в бесконечный ряд. В конце этого же года И. Ньютон получил бакалаврскую степень.
Известные открытия
Изучая краткую биографию Исаака Ньютона, следует знать, что именно ему принадлежит изложение закона всемирного тяготения. Еще одним важнейшим открытием ученого является теория движения небесных тел. Открытые Ньютоном 3 закона механики легли в основу классической механики.
Ньютон сделал немало открытий в области оптики и теории цвета. Им были разработаны многие физические и математические теории. Научные труды выдающегося ученого во многом определяли время и часто были непонятны современникам.
Его гипотезы относительно сплюснутости полюсов Земли, явления поляризации света и отклонения света в поле тяготения и сегодня вызывают удивление ученых.
В 1668 г. Ньютон получил степень магистра. Еще через год он стал доктором математических наук. После создания им рефлектора, предтечи телескопа, в астрономии были сделаны важнейшие открытия.
Общественная деятельность
В 1689 г., в результате переворота, был свергнут король Яков II, с которым у Ньютона был конфликт. После этого ученого избрали в парламент от Кембриджского университета, в котором он заседал около 12 мес.
В 1679 г. произошло знакомство Ньютона с Ч. Монтегю, будущим графом Галифаксом. По протекции Монтегю Ньютон был назначен хранителем Монетного двора.
Последние годы жизни
В 1725 г. здоровье великого ученого стало стремительно ухудшаться. Он ушел из жизни 20 (31) марта 1727 г., в Кенсингтоне. Смерть наступила во сне. Похоронен Исаак Ньютон был в Вестминстерском аббатстве.
Другие варианты биографии
Более сжатая для доклада или сообщения в классе
Вариант 2
Интересные факты
- В самом начале своего школьного обучения, Ньютон считался весьма посредственным, едва ли не худшим учеником. В лучшие его заставила выбиться моральная травма, когда он был избит своим рослым и намного более сильным одноклассником.
- В последние годы жизни великий ученый писал некую книгу, которая, по его мнению, должна была стать неким откровением. К сожалению, рукописи горят. По вине любимой собаки ученого, опрокинувшей лампу, книга исчезла в огне.
Тест по биографии
Доска почёта

Чтобы попасть сюда — пройдите тест.
-
Кристина Малышева
9/10
-
Наиль Галямов
10/10
-
Алексей Коваленко
7/10
-
Ами Магомедова
6/10
-
Михаил Калугин
10/10
-
Диана Сергеева
7/10
-
Александр Котков
9/10
Оценка по биографии
4.3
Средняя оценка: 4.3
Всего получено оценок: 625.
А какая ваша оценка за эту биографию?
This article is about the scientist and mathematician. For the American agriculturalist, see Isaac Newton (agriculturalist).
|
Sir Isaac Newton PRS |
|
|---|---|

Portrait of Newton at 46 by Godfrey Kneller, 1689 |
|
| Born | 4 January 1643 [O.S. 25 December 1642][a]
Woolsthorpe-by-Colsterworth, Lincolnshire, England |
| Died | 31 March 1727 (aged 84) [O.S. 20 March 1726][a]
Kensington, Middlesex, Great Britain |
| Resting place | Westminster Abbey |
| Education | Trinity College, Cambridge (M.A., 1668)[2] |
| Known for |
List
|
| Awards |
|
| Scientific career | |
| Fields |
|
| Institutions |
|
| Academic advisors |
|
| Notable students |
|
| Influences |
|
| Influenced |
List
|
| Member of Parliament for the University of Cambridge | |
| In office 1689–1690 |
|
| Preceded by | Robert Brady |
| Succeeded by | Edward Finch |
| In office 1701–1702 |
|
| Preceded by | Anthony Hammond |
| Succeeded by | Arthur Annesley, 5th Earl of Anglesey |
| 12th President of the Royal Society | |
| In office 1703–1727 |
|
| Preceded by | John Somers |
| Succeeded by | Hans Sloane |
| Master of the Mint | |
| In office 1699–1727 |
|
| 1696–1699 | Warden of the Mint |
| Preceded by | Thomas Neale |
| Succeeded by | John Conduitt |
| 2nd Lucasian Professor of Mathematics | |
| In office 1669–1702 |
|
| Preceded by | Isaac Barrow |
| Succeeded by | William Whiston |
| Personal details | |
| Political party | Whig |
| Signature | |
 |
Sir Isaac Newton PRS (25 December 1642 – 20 March 1726/27)[a] was an English mathematician, physicist, astronomer, alchemist, theologian, and author (described in his time as a «natural philosopher»), widely recognised as one of the greatest mathematicians and physicists and among the most influential scientists of all time. He was a key figure in the philosophical revolution known as the Enlightenment. His book Philosophiæ Naturalis Principia Mathematica (Mathematical Principles of Natural Philosophy), first published in 1687, established classical mechanics. Newton also made seminal contributions to optics, and shares credit with German mathematician Gottfried Wilhelm Leibniz for developing infinitesimal calculus.
In the Principia, Newton formulated the laws of motion and universal gravitation that formed the dominant scientific viewpoint for centuries until it was superseded by the theory of relativity. Newton used his mathematical description of gravity to derive Kepler’s laws of planetary motion, account for tides, the trajectories of comets, the precession of the equinoxes and other phenomena, eradicating doubt about the Solar System’s heliocentricity. He demonstrated that the motion of objects on Earth and celestial bodies could be accounted for by the same principles. Newton’s inference that the Earth is an oblate spheroid was later confirmed by the geodetic measurements of Maupertuis, La Condamine, and others, convincing most European scientists of the superiority of Newtonian mechanics over earlier systems.
Newton built the first practical reflecting telescope and developed a sophisticated theory of colour based on the observation that a prism separates white light into the colours of the visible spectrum. His work on light was collected in his highly influential book Opticks, published in 1704. He also formulated an empirical law of cooling, made the first theoretical calculation of the speed of sound, and introduced the notion of a Newtonian fluid. In addition to his work on calculus, as a mathematician Newton contributed to the study of power series, generalised the binomial theorem to non-integer exponents, developed a method for approximating the roots of a function, and classified most of the cubic plane curves.
Newton was a fellow of Trinity College and the second Lucasian Professor of Mathematics at the University of Cambridge. He was a devout but unorthodox Christian who privately rejected the doctrine of the Trinity. He refused to take holy orders in the Church of England, unlike most members of the Cambridge faculty of the day. Beyond his work on the mathematical sciences, Newton dedicated much of his time to the study of alchemy and biblical chronology, but most of his work in those areas remained unpublished until long after his death. Politically and personally tied to the Whig party, Newton served two brief terms as Member of Parliament for the University of Cambridge, in 1689–1690 and 1701–1702. He was knighted by Queen Anne in 1705 and spent the last three decades of his life in London, serving as Warden (1696–1699) and Master (1699–1727) of the Royal Mint, as well as president of the Royal Society (1703–1727).
Early life
Early life
Isaac Newton was born (according to the Julian calendar in use in England at the time) on Christmas Day, 25 December 1642 (NS 4 January 1643[a]), «an hour or two after midnight»,[17] at Woolsthorpe Manor in Woolsthorpe-by-Colsterworth, a hamlet in the county of Lincolnshire. His father, also named Isaac Newton, had died three months before. Born prematurely, Newton was a small child; his mother Hannah Ayscough reportedly said that he could have fit inside a quart mug.[18] When Newton was three, his mother remarried and went to live with her new husband, the Reverend Barnabas Smith, leaving her son in the care of his maternal grandmother, Margery Ayscough (née Blythe). Newton disliked his stepfather and maintained some enmity towards his mother for marrying him, as revealed by this entry in a list of sins committed up to the age of 19: «Threatening my father and mother Smith to burn them and the house over them.»[19] Newton’s mother had three children (Mary, Benjamin, and Hannah) from her second marriage.[20]
The King’s School
From the age of about twelve until he was seventeen, Newton was educated at The King’s School in Grantham, which taught Latin and Ancient Greek and probably imparted a significant foundation of mathematics.[21] He was removed from school and returned to Woolsthorpe-by-Colsterworth by October 1659. His mother, widowed for the second time, attempted to make him a farmer, an occupation he hated.[22] Henry Stokes, master at The King’s School, persuaded his mother to send him back to school. Motivated partly by a desire for revenge against a schoolyard bully, he became the top-ranked student,[23] distinguishing himself mainly by building sundials and models of windmills.[24]
University of Cambridge
In June 1661, Newton was admitted to Trinity College at the University of Cambridge. His uncle Reverend William Ayscough, who had studied at Cambridge, recommended him to the university. At Cambridge, Newton started as a subsizar, paying his way by performing valet duties until he was awarded a scholarship in 1664, which covered his university costs for four more years until the completion of his MA.[25] At the time, Cambridge’s teachings were based on those of Aristotle, whom Newton read along with then more modern philosophers, including Descartes and astronomers such as Galileo Galilei and Thomas Street. He set down in his notebook a series of «Quaestiones» about mechanical philosophy as he found it. In 1665, he discovered the generalised binomial theorem and began to develop a mathematical theory that later became calculus. Soon after Newton obtained his BA degree at Cambridge in August 1665, the university temporarily closed as a precaution against the Great Plague. Although he had been undistinguished as a Cambridge student,[26] Newton’s private studies at his home in Woolsthorpe over the next two years saw the development of his theories on calculus,[27] optics, and the law of gravitation.
In April 1667, Newton returned to the University of Cambridge, and in October he was elected as a fellow of Trinity.[28][29] Fellows were required to be ordained as priests, although this was not enforced in the restoration years and an assertion of conformity to the Church of England was sufficient. However, by 1675 the issue could not be avoided and by then his unconventional views stood in the way.[30] Nevertheless, Newton managed to avoid it by means of special permission from Charles II.
His academic work impressed the Lucasian professor Isaac Barrow, who was anxious to develop his own religious and administrative potential (he became master of Trinity College two years later); in 1669, Newton succeeded him, only one year after receiving his MA. Newton was elected a Fellow of the Royal Society (FRS) in 1672.[3]
Work
Calculus
Newton’s work has been said «to distinctly advance every branch of mathematics then studied».[31] His work on the subject, usually referred to as fluxions or calculus, seen in a manuscript of October 1666, is now published among Newton’s mathematical papers.[32] His work De analysi per aequationes numero terminorum infinitas, sent by Isaac Barrow to John Collins in June 1669, was identified by Barrow in a letter sent to Collins that August as the work «of an extraordinary genius and proficiency in these things».[33]
Newton later became involved in a dispute with Leibniz over priority in the development of calculus (the Leibniz–Newton calculus controversy). Most modern historians believe that Newton and Leibniz developed calculus independently, although with very different mathematical notations. Occasionally it has been suggested that Newton published almost nothing about it until 1693, and did not give a full account until 1704, while Leibniz began publishing a full account of his methods in 1684. Leibniz’s notation and «differential Method», nowadays recognised as much more convenient notations, were adopted by continental European mathematicians, and after 1820 or so, also by British mathematicians.[citation needed]
His work extensively uses calculus in geometric form based on limiting values of the ratios of vanishingly small quantities: in the Principia itself, Newton gave demonstration of this under the name of «the method of first and last ratios»[34] and explained why he put his expositions in this form,[35] remarking also that «hereby the same thing is performed as by the method of indivisibles.»[36]
Because of this, the Principia has been called «a book dense with the theory and application of the infinitesimal calculus» in modern times[37] and in Newton’s time «nearly all of it is of this calculus.»[38] His use of methods involving «one or more orders of the infinitesimally small» is present in his De motu corporum in gyrum of 1684[39] and in his papers on motion «during the two decades preceding 1684».[40]
Newton had been reluctant to publish his calculus because he feared controversy and criticism.[41] He was close to the Swiss mathematician Nicolas Fatio de Duillier. In 1691, Duillier started to write a new version of Newton’s Principia, and corresponded with Leibniz.[42] In 1693, the relationship between Duillier and Newton deteriorated and the book was never completed.[43]
Starting in 1699, other members[who?] of the Royal Society accused Leibniz of plagiarism.[44] The dispute then broke out in full force in 1711 when the Royal Society proclaimed in a study that it was Newton who was the true discoverer and labelled Leibniz a fraud; it was later found that Newton wrote the study’s concluding remarks on Leibniz. Thus began the bitter controversy which marred the lives of both Newton and Leibniz until the latter’s death in 1716.[45]
Newton is generally credited with the generalised binomial theorem, valid for any exponent. He discovered Newton’s identities, Newton’s method, classified cubic plane curves (polynomials of degree three in two variables), made substantial contributions to the theory of finite differences, and was the first to use fractional indices and to employ coordinate geometry to derive solutions to Diophantine equations. He approximated partial sums of the harmonic series by logarithms (a precursor to Euler’s summation formula) and was the first to use power series with confidence and to revert power series. Newton’s work on infinite series was inspired by Simon Stevin’s decimals.[46]
When Newton received his MA and became a Fellow of the «College of the Holy and Undivided Trinity» in 1667, he made the commitment that «I will either set Theology as the object of my studies and will take holy orders when the time prescribed by these statutes [7 years] arrives, or I will resign from the college.»[47] Up until this point he had not thought much about religion and had twice signed his agreement to the thirty-nine articles, the basis of Church of England doctrine.
He was appointed Lucasian Professor of Mathematics in 1669, on Barrow’s recommendation. During that time, any Fellow of a college at Cambridge or Oxford was required to take holy orders and become an ordained Anglican priest. However, the terms of the Lucasian professorship required that the holder not be active in the church – presumably,[weasel words] so as to have more time for science. Newton argued that this should exempt him from the ordination requirement, and Charles II, whose permission was needed, accepted this argument; thus, a conflict between Newton’s religious views and Anglican orthodoxy was averted.[48]
Optics
In 1666, Newton observed that the spectrum of colours exiting a prism in the position of minimum deviation is oblong, even when the light ray entering the prism is circular, which is to say, the prism refracts different colours by different angles.[50][51] This led him to conclude that colour is a property intrinsic to light – a point which had, until then, been a matter of debate.
From 1670 to 1672, Newton lectured on optics.[52] During this period he investigated the refraction of light, demonstrating that the multicoloured image produced by a prism, which he named a spectrum, could be recomposed into white light by a lens and a second prism.[53] Modern scholarship has revealed that Newton’s analysis and resynthesis of white light owes a debt to corpuscular alchemy.[54]
He showed that coloured light does not change its properties by separating out a coloured beam and shining it on various objects, and that regardless of whether reflected, scattered, or transmitted, the light remains the same colour. Thus, he observed that colour is the result of objects interacting with already-coloured light rather than objects generating the colour themselves. This is known as Newton’s theory of colour.[55]
Illustration of a dispersive prism separating white light into the colours of the spectrum, as discovered by Newton
From this work, he concluded that the lens of any refracting telescope would suffer from the dispersion of light into colours (chromatic aberration). As a proof of the concept, he constructed a telescope using reflective mirrors instead of lenses as the objective to bypass that problem.[56][57] Building the design, the first known functional reflecting telescope, today known as a Newtonian telescope,[57] involved solving the problem of a suitable mirror material and shaping technique. Newton ground his own mirrors out of a custom composition of highly reflective speculum metal, using Newton’s rings to judge the quality of the optics for his telescopes. In late 1668,[58] he was able to produce this first reflecting telescope. It was about eight inches long and it gave a clearer and larger image. In 1671, the Royal Society asked for a demonstration of his reflecting telescope.[59] Their interest encouraged him to publish his notes, Of Colours,[60] which he later expanded into the work Opticks. When Robert Hooke criticised some of Newton’s ideas, Newton was so offended that he withdrew from public debate. Newton and Hooke had brief exchanges in 1679–80, when Hooke, appointed to manage the Royal Society’s correspondence, opened up a correspondence intended to elicit contributions from Newton to Royal Society transactions,[61] which had the effect of stimulating Newton to work out a proof that the elliptical form of planetary orbits would result from a centripetal force inversely proportional to the square of the radius vector. But the two men remained generally on poor terms until Hooke’s death.[62]
Facsimile of a 1682 letter from Newton to William Briggs, commenting on Briggs’ A New Theory of Vision
Newton argued that light is composed of particles or corpuscles, which were refracted by accelerating into a denser medium. He verged on soundlike waves to explain the repeated pattern of reflection and transmission by thin films (Opticks Bk.II, Props. 12), but still retained his theory of ‘fits’ that disposed corpuscles to be reflected or transmitted (Props.13). However, later physicists favoured a purely wavelike explanation of light to account for the interference patterns and the general phenomenon of diffraction. Today’s quantum mechanics, photons, and the idea of wave–particle duality bear only a minor resemblance to Newton’s understanding of light.
In his Hypothesis of Light of 1675, Newton posited the existence of the ether to transmit forces between particles. The contact with the Cambridge Platonist philosopher Henry More revived his interest in alchemy.[63] He replaced the ether with occult forces based on Hermetic ideas of attraction and repulsion between particles. John Maynard Keynes, who acquired many of Newton’s writings on alchemy, stated that «Newton was not the first of the age of reason: He was the last of the magicians.»[64] Newton’s contributions to science cannot be isolated from his interest in alchemy.[63] This was at a time when there was no clear distinction between alchemy and science, and had he not relied on the occult idea of action at a distance, across a vacuum, he might not have developed his theory of gravity.
In 1704, Newton published Opticks, in which he expounded his corpuscular theory of light. He considered light to be made up of extremely subtle corpuscles, that ordinary matter was made of grosser corpuscles and speculated that through a kind of alchemical transmutation «Are not gross Bodies and Light convertible into one another, … and may not Bodies receive much of their Activity from the Particles of Light which enter their Composition?»[65] Newton also constructed a primitive form of a frictional electrostatic generator, using a glass globe.[66]
In his book Opticks, Newton was the first to show a diagram using a prism as a beam expander, and also the use of multiple-prism arrays.[67] Some 278 years after Newton’s discussion, multiple-prism beam expanders became central to the development of narrow-linewidth tunable lasers. Also, the use of these prismatic beam expanders led to the multiple-prism dispersion theory.[67]
Subsequent to Newton, much has been amended. Young and Fresnel discarded Newton’s particle theory in favour of Huygens’ wave theory to show that colour is the visible manifestation of light’s wavelength. Science also slowly came to realise the difference between perception of colour and mathematisable optics. The German poet and scientist, Goethe, could not shake the Newtonian foundation but «one hole Goethe did find in Newton’s armour, … Newton had committed himself to the doctrine that refraction without colour was impossible. He, therefore, thought that the object-glasses of telescopes must forever remain imperfect, achromatism and refraction being incompatible. This inference was proved by Dollond to be wrong.»[68]
Gravity
In 1679, Newton returned to his work on celestial mechanics by considering gravitation and its effect on the orbits of planets with reference to Kepler’s laws of planetary motion. This followed stimulation by a brief exchange of letters in 1679–80 with Hooke, who had been appointed to manage the Royal Society’s correspondence, and who opened a correspondence intended to elicit contributions from Newton to Royal Society transactions.[61] Newton’s reawakening interest in astronomical matters received further stimulus by the appearance of a comet in the winter of 1680–1681, on which he corresponded with John Flamsteed.[69] After the exchanges with Hooke, Newton worked out a proof that the elliptical form of planetary orbits would result from a centripetal force inversely proportional to the square of the radius vector. Newton communicated his results to Edmond Halley and to the Royal Society in De motu corporum in gyrum, a tract written on about nine sheets which was copied into the Royal Society’s Register Book in December 1684.[70] This tract contained the nucleus that Newton developed and expanded to form the Principia.
The Principia was published on 5 July 1687 with encouragement and financial help from Halley. In this work, Newton stated the three universal laws of motion. Together, these laws describe the relationship between any object, the forces acting upon it and the resulting motion, laying the foundation for classical mechanics. They contributed to many advances during the Industrial Revolution which soon followed and were not improved upon for more than 200 years. Many of these advances continue to be the underpinnings of non-relativistic technologies in the modern world. He used the Latin word gravitas (weight) for the effect that would become known as gravity, and defined the law of universal gravitation.[71]
In the same work, Newton presented a calculus-like method of geometrical analysis using ‘first and last ratios’, gave the first analytical determination (based on Boyle’s law) of the speed of sound in air, inferred the oblateness of Earth’s spheroidal figure, accounted for the precession of the equinoxes as a result of the Moon’s gravitational attraction on the Earth’s oblateness, initiated the gravitational study of the irregularities in the motion of the Moon, provided a theory for the determination of the orbits of comets, and much more.[71] Newton’s biographer David Brewster reported that the complexity of applying his theory of gravity to the motion of the moon was so great it affected Newton’s health: «[H]e was deprived of his appetite and sleep» during his work on the problem in 1692-3, and told the astronomer John Machin that «his head never ached but when he was studying the subject». According to Brewster Edmund Halley also told John Conduitt that when pressed to complete his analysis Newton «always replied that it made his head ache, and kept him awake so often, that he would think of it no more«. [Emphasis in original][72]
Newton made clear his heliocentric view of the Solar System—developed in a somewhat modern way because already in the mid-1680s he recognised the «deviation of the Sun» from the centre of gravity of the Solar System.[73] For Newton, it was not precisely the centre of the Sun or any other body that could be considered at rest, but rather «the common centre of gravity of the Earth, the Sun and all the Planets is to be esteem’d the Centre of the World», and this centre of gravity «either is at rest or moves uniformly forward in a right line» (Newton adopted the «at rest» alternative in view of common consent that the centre, wherever it was, was at rest).[74]
Newton’s postulate of an invisible force able to act over vast distances led to him being criticised for introducing «occult agencies» into science.[75] Later, in the second edition of the Principia (1713), Newton firmly rejected such criticisms in a concluding General Scholium, writing that it was enough that the phenomena implied a gravitational attraction, as they did; but they did not so far indicate its cause, and it was both unnecessary and improper to frame hypotheses of things that were not implied by the phenomena. (Here Newton used what became his famous expression «hypotheses non-fingo»[76]).
With the Principia, Newton became internationally recognised.[77] He acquired a circle of admirers, including the Swiss-born mathematician Nicolas Fatio de Duillier.[78]
In 1710, Newton found 72 of the 78 «species» of cubic curves and categorised them into four types.[79] In 1717, and probably with Newton’s help, James Stirling proved that every cubic was one of these four types. Newton also claimed that the four types could be obtained by plane projection from one of them, and this was proved in 1731, four years after his death.[80]
Later life
Royal Mint
In the 1690s, Newton wrote a number of religious tracts dealing with the literal and symbolic interpretation of the Bible. A manuscript Newton sent to John Locke in which he disputed the fidelity of 1 John 5:7—the Johannine Comma—and its fidelity to the original manuscripts of the New Testament, remained unpublished until 1785.[81]
Newton was also a member of the Parliament of England for Cambridge University in 1689 and 1701, but according to some accounts his only comments were to complain about a cold draught in the chamber and request that the window be closed.[82] He was, however, noted by Cambridge diarist Abraham de la Pryme to have rebuked students who were frightening locals by claiming that a house was haunted.[83]
Newton moved to London to take up the post of warden of the Royal Mint in 1696, a position that he had obtained through the patronage of Charles Montagu, 1st Earl of Halifax, then Chancellor of the Exchequer. He took charge of England’s great recoining, trod on the toes of Lord Lucas, Governor of the Tower, and secured the job of deputy comptroller of the temporary Chester branch for Edmond Halley. Newton became perhaps the best-known Master of the Mint upon the death of Thomas Neale in 1699, a position Newton held for the last 30 years of his life.[84][85] These appointments were intended as sinecures, but Newton took them seriously. He retired from his Cambridge duties in 1701, and exercised his authority to reform the currency and punish clippers and counterfeiters.
As Warden, and afterwards as Master, of the Royal Mint, Newton estimated that 20 percent of the coins taken in during the Great Recoinage of 1696 were counterfeit. Counterfeiting was high treason, punishable by the felon being hanged, drawn and quartered. Despite this, convicting even the most flagrant criminals could be extremely difficult, but Newton proved equal to the task.[86]
Disguised as a habitué of bars and taverns, he gathered much of that evidence himself.[87] For all the barriers placed to prosecution, and separating the branches of government, English law still had ancient and formidable customs of authority. Newton had himself made a justice of the peace in all the home counties. A draft letter regarding the matter is included in Newton’s personal first edition of Philosophiæ Naturalis Principia Mathematica, which he must have been amending at the time.[88] Then he conducted more than 100 cross-examinations of witnesses, informers, and suspects between June 1698 and Christmas 1699. Newton successfully prosecuted 28 coiners.[89]
Newton was made president of the Royal Society in 1703 and an associate of the French Académie des Sciences. In his position at the Royal Society, Newton made an enemy of John Flamsteed, the Astronomer Royal, by prematurely publishing Flamsteed’s Historia Coelestis Britannica, which Newton had used in his studies.[91]
Knighthood
In April 1705, Queen Anne knighted Newton during a royal visit to Trinity College, Cambridge. The knighthood is likely to have been motivated by political considerations connected with the parliamentary election in May 1705, rather than any recognition of Newton’s scientific work or services as Master of the Mint.[92] Newton was the second scientist to be knighted, after Francis Bacon.[93]
As a result of a report written by Newton on 21 September 1717 to the Lords Commissioners of His Majesty’s Treasury, the bimetallic relationship between gold coins and silver coins was changed by royal proclamation on 22 December 1717, forbidding the exchange of gold guineas for more than 21 silver shillings.[94] This inadvertently resulted in a silver shortage as silver coins were used to pay for imports, while exports were paid for in gold, effectively moving Britain from the silver standard to its first gold standard. It is a matter of debate as to whether he intended to do this or not.[95] It has been argued that Newton conceived of his work at the Mint as a continuation of his alchemical work.[96]
Newton was invested in the South Sea Company and lost some £20,000 (£4.4 million in 2020[97]) when it collapsed in around 1720.[98]
Toward the end of his life, Newton took up residence at Cranbury Park, near Winchester, with his niece and her husband, until his death.[99] His half-niece, Catherine Barton,[100] served as his hostess in social affairs at his house on Jermyn Street in London; he was her «very loving Uncle»,[101] according to his letter to her when she was recovering from smallpox.
Death
Newton died in his sleep in London on 20 March 1727 (OS 20 March 1726; NS 31 March 1727).[a] He was given a ceremonial funeral, attended by nobles, scientists, and philosophers, and was buried in Westminster Abbey among kings and queens. He is also the first scientist to be buried in the abbey.[102] Voltaire may have been present at his funeral.[103] A bachelor, he had divested much of his estate to relatives during his last years, and died intestate.[104] His papers went to John Conduitt and Catherine Barton.[105]
After his death, Newton’s hair was examined and found to contain mercury, probably resulting from his alchemical pursuits. Mercury poisoning could explain Newton’s eccentricity in late life.[104]
Personality
Although it was claimed that he was once engaged,[b] Newton never married. The French writer and philosopher Voltaire, who was in London at the time of Newton’s funeral, said that he «was never sensible to any passion, was not subject to the common frailties of mankind, nor had any commerce with women—a circumstance which was assured me by the physician and surgeon who attended him in his last moments».[107] There exists a widespread belief that Newton died a virgin, and writers as diverse as mathematician Charles Hutton,[108] economist John Maynard Keynes,[109] and physicist Carl Sagan each have commented on it.[110]
Newton had a close friendship with the Swiss mathematician Nicolas Fatio de Duillier, who he met in London around 1689[78]—some of their correspondence has survived.[111][112] Their relationship came to an abrupt and unexplained end in 1693, and at the same time Newton suffered a nervous breakdown,[113] which included sending wild accusatory letters to his friends Samuel Pepys and John Locke. His note to the latter included the charge that Locke «endeavoured to embroil me with woemen».[114]
Newton was relatively modest about his achievements, writing in a letter to Robert Hooke in February 1676, «If I have seen further it is by standing on the shoulders of giants.»[115] Two writers think that the sentence, written at a time when Newton and Hooke were in dispute over optical discoveries, was an oblique attack on Hooke (said to have been short and hunchbacked), rather than—or in addition to—a statement of modesty.[116][117] On the other hand, the widely known proverb about standing on the shoulders of giants, published among others by seventeenth-century poet George Herbert (a former orator of the University of Cambridge and fellow of Trinity College) in his Jacula Prudentum (1651), had as its main point that «a dwarf on a giant’s shoulders sees farther of the two», and so its effect as an analogy would place Newton himself rather than Hooke as the ‘dwarf’.
In a later memoir, Newton wrote, «I do not know what I may appear to the world, but to myself I seem to have been only like a boy playing on the sea-shore, and diverting myself in now and then finding a smoother pebble or a prettier shell than ordinary, whilst the great ocean of truth lay all undiscovered before me.»[118]
In 2015, Steven Weinberg, a Nobel laureate in physics, called Newton «a nasty antagonist» and «a bad man to have as an enemy»,[119] noting Newton’s attitude towards Robert Hooke and Gottfried Wilhelm Leibniz.
It has been suggested by some scientists and clinicians that, based on these and other traits along with his profound power of concentration, that Newton may have had an undiagnosed form of high-functioning autism, now properly known as ASD1 within autism spectrum; formerly known as Asperger syndrome.[120][121][122]
Theology
Religious views
Although born into an Anglican family, by his thirties Newton held a Christian faith that, had it been made public, would not have been considered orthodox by mainstream Christianity,[123] with one historian labelling him a heretic.[124]
By 1672, he had started to record his theological researches in notebooks which he showed to no one and which have only recently[when?] been examined. They demonstrate an extensive knowledge of early Church writings and show that in the conflict between Athanasius and Arius which defined the Creed, he took the side of Arius, the loser, who rejected the conventional view of the Trinity. Newton «recognized Christ as a divine mediator between God and man, who was subordinate to the Father who created him.»[125] He was especially interested in prophecy, but for him, «the great apostasy was trinitarianism.»[126]
Newton tried unsuccessfully to obtain one of the two fellowships that exempted the holder from the ordination requirement. At the last moment in 1675 he received a dispensation from the government that excused him and all future holders of the Lucasian chair.[127]
In Newton’s eyes, worshipping Christ as God was idolatry, to him the fundamental sin.[128] In 1999, historian Stephen D. Snobelen wrote, «Isaac Newton was a heretic. But … he never made a public declaration of his private faith—which the orthodox would have deemed extremely radical. He hid his faith so well that scholars are still unraveling his personal beliefs.»[124] Snobelen concludes that Newton was at least a Socinian sympathiser (he owned and had thoroughly read at least eight Socinian books), possibly an Arian and almost certainly an anti-trinitarian.[124]
The view that Newton was Semi-Arian has lost support now that scholars have investigated Newton’s theological papers, and now most scholars identify Newton as an Antitrinitarian monotheist.[124][129]
Although the laws of motion and universal gravitation became Newton’s best-known discoveries, he warned against using them to view the Universe as a mere machine, as if akin to a great clock. He said, «So then gravity may put the planets into motion, but without the Divine Power it could never put them into such a circulating motion, as they have about the sun».[131]
Along with his scientific fame, Newton’s studies of the Bible and of the early Church Fathers were also noteworthy. Newton wrote works on textual criticism, most notably An Historical Account of Two Notable Corruptions of Scripture and Observations upon the Prophecies of Daniel, and the Apocalypse of St. John.[132] He placed the crucifixion of Jesus Christ at 3 April, AD 33, which agrees with one traditionally accepted date.[133]
He believed in a rationally immanent world, but he rejected the hylozoism implicit in Leibniz and Baruch Spinoza. The ordered and dynamically informed Universe could be understood, and must be understood, by an active reason. In his correspondence, Newton claimed that in writing the Principia «I had an eye upon such Principles as might work with considering men for the belief of a Deity».[134] He saw evidence of design in the system of the world: «Such a wonderful uniformity in the planetary system must be allowed the effect of choice». But Newton insisted that divine intervention would eventually be required to reform the system, due to the slow growth of instabilities.[135] For this, Leibniz lampooned him: «God Almighty wants to wind up his watch from time to time: otherwise it would cease to move. He had not, it seems, sufficient foresight to make it a perpetual motion.»[136]
Newton’s position was vigorously defended by his follower Samuel Clarke in a famous correspondence. A century later, Pierre-Simon Laplace’s work Celestial Mechanics had a natural explanation for why the planet orbits do not require periodic divine intervention.[137] The contrast between Laplace’s mechanistic worldview and Newton’s one is the most strident considering the famous answer which the French scientist gave Napoleon, who had criticised him for the absence of the Creator in the Mécanique céleste: «Sire, j’ai pu me passer de cette hypothèse» («Sir, I didn’t need this hypothesis»).[138]
Scholars long debated whether Newton disputed the doctrine of the Trinity. His first biographer, David Brewster, who compiled his manuscripts, interpreted Newton as questioning the veracity of some passages used to support the Trinity, but never denying the doctrine of the Trinity as such.[139] In the twentieth century, encrypted manuscripts written by Newton and bought by John Maynard Keynes (among others) were deciphered[64] and it became known that Newton did indeed reject Trinitarianism.[124]
Religious thought
Newton and Robert Boyle’s approach to the mechanical philosophy was promoted by rationalist pamphleteers as a viable alternative to the pantheists and enthusiasts, and was accepted hesitantly by orthodox preachers as well as dissident preachers like the latitudinarians.[140] The clarity and simplicity of science was seen as a way to combat the emotional and metaphysical superlatives of both superstitious enthusiasm and the threat of atheism,[141] and at the same time, the second wave of English deists used Newton’s discoveries to demonstrate the possibility of a «Natural Religion».
The attacks made against pre-Enlightenment «magical thinking», and the mystical elements of Christianity, were given their foundation with Boyle’s mechanical conception of the universe. Newton gave Boyle’s ideas their completion through mathematical proofs and, perhaps more importantly, was very successful in popularising them.[142]
The occult
In a manuscript he wrote in 1704 (never intended to be published), he mentions the date of 2060, but it is not given as a date for the end of days. It has been falsely reported as a prediction.[143] The passage is clear when the date is read in context. He was against date setting for the end of days, concerned that this would put Christianity into disrepute.
So then the time times & half a time [sic] are 42 months or 1260 days or three years & an half, recconing twelve months to a year & 30 days to a month as was done in the Calender [sic] of the primitive year. And the days of short lived Beasts being put for the years of [long-]lived kingdoms the period of 1260 days, if dated from the complete conquest of the three kings A.C. 800, will end 2060. It may end later, but I see no reason for its ending sooner.[144]
This I mention not to assert when the time of the end shall be, but to put a stop to the rash conjectures of fanciful men who are frequently predicting the time of the end, and by doing so bring the sacred prophesies into discredit as often as their predictions fail. Christ comes as a thief in the night, and it is not for us to know the times and seasons which God hath put into his own breast.[145][143]
Alchemy
In the character of Morton Opperly in «Poor Superman» (1951), speculative fiction author Fritz Leiber says of Newton, «Everyone knows Newton as the great scientist. Few remember that he spent half his life muddling with alchemy, looking for the philosopher’s stone. That was the pebble by the seashore he really wanted to find.»[146]
Of an estimated ten million words of writing in Newton’s papers, about one million deal with alchemy. Many of Newton’s writings on alchemy are copies of other manuscripts, with his own annotations.[105] Alchemical texts mix artisanal knowledge with philosophical speculation, often hidden behind layers of wordplay, allegory, and imagery to protect craft secrets.[147] Some of the content contained in Newton’s papers could have been considered heretical by the church.[105]
In 1888, after spending sixteen years cataloguing Newton’s papers, Cambridge University kept a small number and returned the rest to the Earl of Portsmouth. In 1936, a descendant offered the papers for sale at Sotheby’s.[148] The collection was broken up and sold for a total of about £9,000.[149] John Maynard Keynes was one of about three dozen bidders who obtained part of the collection at auction. Keynes went on to reassemble an estimated half of Newton’s collection of papers on alchemy before donating his collection to Cambridge University in 1946.[105][148][150]
All of Newton’s known writings on alchemy are currently being put online in a project undertaken by Indiana University: «The Chymistry of Isaac Newton»[151] and summarised in a book.[152][153]
Newton’s fundamental contributions to science include the quantification of gravitational attraction, the discovery that white light is actually a mixture of immutable spectral colors, and the formulation of the calculus. Yet there is another, more mysterious side to Newton that is imperfectly known, a realm of activity that spanned some thirty years of his life, although he kept it largely hidden from his contemporaries and colleagues. We refer to Newton’s involvement in the discipline of alchemy, or as it was often called in seventeenth-century England, «chymistry.»[151]
Charles Coulston Gillispie disputes that Newton ever practised alchemy, saying that «his chemistry was in the spirit of Boyle’s corpuscular philosophy.»[154]
In June 2020, two unpublished pages of Newton’s notes on Jan Baptist van Helmont’s book on plague, De Peste,[155] were being auctioned online by Bonhams. Newton’s analysis of this book, which he made in Cambridge while protecting himself from London’s 1665–1666 infection, is the most substantial written statement he is known to have made about the plague, according to Bonhams. As far as the therapy is concerned, Newton writes that «the best is a toad suspended by the legs in a chimney for three days, which at last vomited up earth with various insects in it, on to a dish of yellow wax, and shortly after died. Combining powdered toad with the excretions and serum made into lozenges and worn about the affected area drove away the contagion and drew out the poison».[156]
Legacy
Fame
The mathematician Joseph-Louis Lagrange said that Newton was the greatest genius who ever lived, and once added that Newton was also «the most fortunate, for we cannot find more than once a system of the world to establish.»[157] English poet Alexander Pope wrote the famous epitaph:
Nature, and Nature’s laws lay hid in night.
God said, Let Newton be! and all was light.
But this was not allowed to be inscribed in the monument. The epitaph in the monument is as follows:[158]
H. S. E. ISAACUS NEWTON Eques Auratus, / Qui, animi vi prope divinâ, / Planetarum Motus, Figuras, / Cometarum semitas, Oceanique Aestus. Suâ Mathesi facem praeferente / Primus demonstravit: / Radiorum Lucis dissimilitudines, / Colorumque inde nascentium proprietates, / Quas nemo antea vel suspicatus erat, pervestigavit. / Naturae, Antiquitatis, S. Scripturae, / Sedulus, sagax, fidus Interpres / Dei O. M. Majestatem Philosophiâ asseruit, / Evangelij Simplicitatem Moribus expressit. / Sibi gratulentur Mortales, / Tale tantumque exstitisse / HUMANI GENERIS DECUS. / NAT. XXV DEC. A.D. MDCXLII. OBIIT. XX. MAR. MDCCXXVI,
which can be translated as follows:[158]
Here is buried Isaac Newton, Knight, who by a strength of mind almost divine, and mathematical principles peculiarly his own, explored the course and figures of the planets, the paths of comets, the tides of the sea, the dissimilarities in rays of light, and, what no other scholar has previously imagined, the properties of the colours thus produced. Diligent, sagacious and faithful, in his expositions of nature, antiquity and the holy Scriptures, he vindicated by his philosophy the majesty of God mighty and good, and expressed the simplicity of the Gospel in his manners. Mortals rejoice that there has existed such and so great an ornament of the human race! He was born on 25th December 1642, and died on 20th March 1726.
In a 2005 survey of members of Britain’s Royal Society (formerly headed by Newton) asking who had the greater effect on the history of science, Newton or Albert Einstein, the members deemed Newton to have made the greater overall contribution.[159] In 1999, an opinion poll of 100 of the day’s leading physicists voted Einstein the «greatest physicist ever,» with Newton the runner-up, while a parallel survey of rank-and-file physicists by the site PhysicsWeb gave the top spot to Newton.[160] Einstein kept a picture of Newton on his study wall alongside ones of Michael Faraday and James Clerk Maxwell.[161]
The SI derived unit of force is named the newton in his honour.
Woolsthorpe Manor is a Grade I listed building by Historic England through being his birthplace and «where he discovered gravity and developed his theories regarding the refraction of light».[162]
In 1816, a tooth said to have belonged to Newton was sold for £730[163] (US$3,633) in London to an aristocrat who had it set in a ring.[164] Guinness World Records 2002 classified it as the most valuable tooth, which would value approximately £25,000 (US$35,700) in late 2001.[164] Who bought it and who currently has it has not been disclosed.
Apple incident
Newton himself often told the story that he was inspired to formulate his theory of gravitation by watching the fall of an apple from a tree.[165][166] The story is believed to have passed into popular knowledge after being related by Catherine Barton, Newton’s niece, to Voltaire.[167] Voltaire then wrote in his Essay on Epic Poetry (1727), «Sir Isaac Newton walking in his gardens, had the first thought of his system of gravitation, upon seeing an apple falling from a tree.»[168][169]
Although it has been said that the apple story is a myth and that he did not arrive at his theory of gravity at any single moment,[170] acquaintances of Newton (such as William Stukeley, whose manuscript account of 1752 has been made available by the Royal Society) do in fact confirm the incident, though not the apocryphal version that the apple actually hit Newton’s head. Stukeley recorded in his Memoirs of Sir Isaac Newton’s Life a conversation with Newton in Kensington on 15 April 1726:[171][172][173]
we went into the garden, & drank thea under the shade of some appletrees, only he, & myself. amidst other discourse, he told me, he was just in the same situation, as when formerly, the notion of gravitation came into his mind. «why should that apple always descend perpendicularly to the ground,» thought he to him self: occasion’d by the fall of an apple, as he sat in a comtemplative mood: «why should it not go sideways, or upwards? but constantly to the earths centre? assuredly, the reason is, that the earth draws it. there must be a drawing power in matter. & the sum of the drawing power in the matter of the earth must be in the earths center, not in any side of the earth. therefore dos this apple fall perpendicularly, or toward the center. if matter thus draws matter; it must be in proportion of its quantity. therefore the apple draws the earth, as well as the earth draws the apple.»
John Conduitt, Newton’s assistant at the Royal Mint and husband of Newton’s niece, also described the event when he wrote about Newton’s life:[174]
In the year 1666 he retired again from Cambridge to his mother in Lincolnshire. Whilst he was pensively meandering in a garden it came into his thought that the power of gravity (which brought an apple from a tree to the ground) was not limited to a certain distance from earth, but that this power must extend much further than was usually thought. Why not as high as the Moon said he to himself & if so, that must influence her motion & perhaps retain her in her orbit, whereupon he fell a calculating what would be the effect of that supposition.
It is known from his notebooks that Newton was grappling in the late 1660s with the idea that terrestrial gravity extends, in an inverse-square proportion, to the Moon; however, it took him two decades to develop the full-fledged theory.[175] The question was not whether gravity existed, but whether it extended so far from Earth that it could also be the force holding the Moon to its orbit. Newton showed that if the force decreased as the inverse square of the distance, one could indeed calculate the Moon’s orbital period, and get good agreement. He guessed the same force was responsible for other orbital motions, and hence named it «universal gravitation».
Various trees are claimed to be «the» apple tree which Newton describes. The King’s School, Grantham claims that the tree was purchased by the school, uprooted and transported to the headmaster’s garden some years later. The staff of the (now) National Trust-owned Woolsthorpe Manor dispute this, and claim that a tree present in their gardens is the one described by Newton. A descendant of the original tree[176] can be seen growing outside the main gate of Trinity College, Cambridge, below the room Newton lived in when he studied there. The National Fruit Collection at Brogdale in Kent[177] can supply grafts from their tree, which appears identical to Flower of Kent, a coarse-fleshed cooking variety.[178]
Commemorations
Newton’s monument (1731) can be seen in Westminster Abbey, at the north of the entrance to the choir against the choir screen, near his tomb. It was executed by the sculptor Michael Rysbrack (1694–1770) in white and grey marble with design by the architect William Kent.[179] The monument features a figure of Newton reclining on top of a sarcophagus, his right elbow resting on several of his great books and his left hand pointing to a scroll with a mathematical design. Above him is a pyramid and a celestial globe showing the signs of the Zodiac and the path of the comet of 1680. A relief panel depicts putti using instruments such as a telescope and prism.[180] The Latin inscription on the base translates as:
Here is buried Isaac Newton, Knight, who by a strength of mind almost divine, and mathematical principles peculiarly his own, explored the course and figures of the planets, the paths of comets, the tides of the sea, the dissimilarities in rays of light, and, what no other scholar has previously imagined, the properties of the colours thus produced. Diligent, sagacious and faithful, in his expositions of nature, antiquity and the holy Scriptures, he vindicated by his philosophy the majesty of God mighty and good, and expressed the simplicity of the Gospel in his manners. Mortals rejoice that there has existed such and so great an ornament of the human race! He was born on 25 December 1642, and died on 20 March 1726/7.
- —Translation from G. L. Smyth, The Monuments and Genii of St. Paul’s Cathedral, and of Westminster Abbey (1826), ii, 703–704.[180]
From 1978 until 1988, an image of Newton designed by Harry Ecclestone appeared on Series D £1 banknotes issued by the Bank of England (the last £1 notes to be issued by the Bank of England). Newton was shown on the reverse of the notes holding a book and accompanied by a telescope, a prism and a map of the Solar System.[181]
A statue of Isaac Newton, looking at an apple at his feet, can be seen at the Oxford University Museum of Natural History. A large bronze statue, Newton, after William Blake, by Eduardo Paolozzi, dated 1995 and inspired by Blake’s etching, dominates the piazza of the British Library in London. A bronze statue of Newton was erected in 1858 in the centre of Grantham where he went to school, prominently standing in front of Grantham Guildhall.
The still-surviving farmhouse at Woolsthorpe By Colsterworth is a Grade I listed building by Historic England through being his birthplace and «where he discovered gravity and developed his theories regarding the refraction of light».[162]
The Enlightenment
Enlightenment philosophers chose a short history of scientific predecessors—Galileo, Boyle, and Newton principally—as the guides and guarantors of their applications of the singular concept of nature and natural law to every physical and social field of the day. In this respect, the lessons of history and the social structures built upon it could be discarded.[182]
It is held by European philosophers of the Enlightenment and by historians of the Enlightenment that Newton’s publication of the Principia was a turning point in the Scientific Revolution and started the Enlightenment. It was Newton’s conception of the universe based upon natural and rationally understandable laws that became one of the seeds for Enlightenment ideology.[183] Locke and Voltaire applied concepts of natural law to political systems advocating intrinsic rights; the physiocrats and Adam Smith applied natural conceptions of psychology and self-interest to economic systems; and sociologists criticised the current social order for trying to fit history into natural models of progress. Monboddo and Samuel Clarke resisted elements of Newton’s work, but eventually rationalised it to conform with their strong religious views of nature.
Works
Published in his lifetime
- De analysi per aequationes numero terminorum infinitas (1669, published 1711)[184]
- Of Natures Obvious Laws & Processes in Vegetation (unpublished, c. 1671–75)[185]
- De motu corporum in gyrum (1684)[186]
- Philosophiæ Naturalis Principia Mathematica (1687)[187]
- Scala graduum Caloris. Calorum Descriptiones & signa (1701)[188]
- Opticks (1704)[189]
- Reports as Master of the Mint (1701–1725)[190]
- Arithmetica Universalis (1707)[190]
Published posthumously
- De mundi systemate (The System of the World) (1728)[190]
- Optical Lectures (1728)[190]
- The Chronology of Ancient Kingdoms Amended (1728)[190]
- Observations on Daniel and The Apocalypse of St. John (1733)[190]
- Method of Fluxions (1671, published 1736)[191]
- An Historical Account of Two Notable Corruptions of Scripture (1754)[190]
See also
- Elements of the Philosophy of Newton, a book by Voltaire
- List of multiple discoveries: seventeenth century
- List of things named after Isaac Newton
References
Notes
- ^ a b c d e During Newton’s lifetime, two calendars were in use in Europe: the Julian («Old Style») calendar in Protestant and Orthodox regions, including Britain; and the Gregorian («New Style») calendar in Roman Catholic Europe. At Newton’s birth, Gregorian dates were ten days ahead of Julian dates; thus, his birth is recorded as taking place on 25 December 1642 Old Style, but it can be converted to a New Style (modern) date of 4 January 1643. By the time of his death, the difference between the calendars had increased to eleven days. Moreover, he died in the period after the start of the New Style year on 1 January but before that of the Old Style new year on 25 March. His death occurred on 20 March 1726, according to the Old Style calendar, but the year is usually adjusted to 1727. A full conversion to New Style gives the date 31 March 1727.[1]
- ^ This claim was made by William Stukeley in 1727, in a letter about Newton written to Richard Mead. Charles Hutton, who in the late eighteenth century collected oral traditions about earlier scientists, declared that there «do not appear to be any sufficient reason for his never marrying, if he had an inclination so to do. It is much more likely that he had a constitutional indifference to the state, and even to the sex in general.»[106]
Citations
- ^ Thony, Christie (2015). «Calendrical confusion or just when did Newton die?». The Renaissance Mathematicus. Archived from the original on 2 April 2015. Retrieved 20 March 2015.
- ^ Kevin C. Knox, Richard Noakes (eds.), From Newton to Hawking: A History of Cambridge University’s Lucasian Professors of Mathematics, Cambridge University Press, 2003, p. 61.
- ^ a b «Fellows of the Royal Society». London: Royal Society. Archived from the original on 16 March 2015.
- ^ Feingold, Mordechai. Barrow, Isaac (1630–1677) Archived 29 January 2013 at the Wayback Machine, Oxford Dictionary of National Biography, Oxford University Press, September 2004; online edn, May 2007. Retrieved 24 February 2009; explained further in Feingold, Mordechai (1993). «Newton, Leibniz, and Barrow Too: An Attempt at a Reinterpretation». Isis. 84 (2): 310–338. Bibcode:1993Isis…84..310F. doi:10.1086/356464. JSTOR 236236. S2CID 144019197.
- ^ «Dictionary of Scientific Biography». Notes, No. 4. Archived from the original on 25 February 2005.
- ^ Gjertsen 1986, p. [page needed]
- ^ Newton, Isaac (February 1678). Philosophical tract from Mr Isaac Newton. Cambridge University. Archived from the original on 8 October 2016. Retrieved 1 October 2021.
But because I am indebted to you & yesterday met with a friend Mr Maulyverer, who told me he was going to London & intended to give you the trouble of a visit, I could not forbear to take the opportunity of conveying this to you by him.
- ^ I. Bernard Cohen; George E. Smith (25 April 2002). The Cambridge Companion to Newton. Cambridge University Press. p. 69. ISBN 978-0-521-65696-2. Archived from the original on 16 September 2020. Retrieved 15 May 2013.
- ^ Niccolò Guicciardini (2009). Isaac Newton on mathematical certainty and method. MIT Press. p. 344. ISBN 978-0-262-01317-8. Archived from the original on 16 September 2020. Retrieved 15 May 2013.
- ^ a b Ducheyne, Steffen (2009). «The Flow of Influence from Newton to Locke… and Back». Rivista di Storia della Filosofia (1984–). 64 (2): 245–268. doi:10.3280/SF2009-002001. ISSN 0393-2516. JSTOR 44024132. Archived from the original on 23 May 2022. Retrieved 23 May 2022.
- ^ a b Rogers, G. A. J. (1978). «Locke’s Essay and Newton’s Principia». Journal of the History of Ideas. 39 (2): 217–232. doi:10.2307/2708776. ISSN 0022-5037. JSTOR 2708776. Archived from the original on 23 May 2022. Retrieved 23 May 2022.
- ^ «Isaac Newton: «Judaic monotheist of the school of Maimonides»«. Achgut.com. 19 June 2007. Archived from the original on 28 April 2015. Retrieved 13 March 2010.
- ^ See e.g. D. T. Whiteside, Before the Principia, in Journal for the History of Astronomy 1 (1970), 5–17, p. 7.
- ^ Jeremy Jennings. Revolution and the Republic: A History of Political Thought in France Since the Eighteenth Century. Oxford University Press, 2011. p. 347.
- ^ Bruni, Luigino; Porta, Pier Luigi (2003). «Economia Civile and Pubblica Felicità in the Italian Enlightenment». History of Political Economy. 35 (Suppl. 1): 361–385 (365). doi:10.1215/00182702-35-Suppl_1-361. S2CID 143538016.
- ^ Pearson, Roger (2005). Voltaire almighty : a life in pursuit of freedom. Internet Archive. New York : Bloomsbury : Distributed to the trade by Holtzbrinck. p. 138. ISBN 978-1-58234-630-4.
- ^ «Isaac Newton, horoscope for birth date 25 December 1642 Jul.Cal». Astro-Databank Wiki. Archived from the original on 5 January 2017. Retrieved 4 January 2017.
- ^ Storr, Anthony (December 1985). «Isaac Newton». British Medical Journal (Clinical Research Edition). 291 (6511): 1779–1784. doi:10.1136/bmj.291.6511.1779. JSTOR 29521701. PMC 1419183. PMID 3936583.
- ^ Keynes, Milo (20 September 2008). «Balancing Newton’s Mind: His Singular Behaviour and His Madness of 1692–93». Notes and Records of the Royal Society of London. 62 (3): 289–300. doi:10.1098/rsnr.2007.0025. JSTOR 20462679. PMID 19244857.
- ^ Westfall 1980, p. 55.
- ^ «Newton the Mathematician» Z. Bechler, ed., Contemporary Newtonian Research(Dordrecht 1982) pp. 110–111
- ^ Westfall 1994, pp. 16–19.
- ^ White 1997, p. 22.
- ^ Westfall 1980, pp. 60–62.
- ^ Westfall 1980, pp. 71, 103.
- ^ Hoskins, Michael, ed. (1997). Cambridge Illustrated History of Astronomy. Cambridge University Press. p. 159. ISBN 978-0-521-41158-5.
- ^ Newton, Isaac. «Waste Book». Cambridge University Digital Library. Archived from the original on 8 January 2012. Retrieved 10 January 2012.
- ^ «Newton, Isaac (NWTN661I)». A Cambridge Alumni Database. University of Cambridge.
- ^ Westfall 1980, p. 178.
- ^ Westfall 1980, pp. 330–331.
- ^ Ball 1908, p. 319.
- ^ Whiteside, D.T., ed. (1967). «Part 7: The October 1666 Tract on Fluxions». The Mathematical Papers of Isaac Newton. 1. Cambridge University Press. p. 400. Archived 12 May 2016 at the Wayback Machine.
- ^ Gjertsen 1986, p. 149.
- ^ Newton, Principia, 1729 English translation, p. 41 Archived 3 October 2015 at the Wayback Machine.
- ^ Newton, Principia, 1729 English translation, p. 54 Archived 3 May 2016 at the Wayback Machine.
- ^ Newton, Sir Isaac (1850). Newton’s Principia: The Mathematical Principles of Natural Philosophy. Geo. P. Putnam. Archived from the original on 26 June 2019. Retrieved 9 March 2019.
- ^ Clifford Truesdell, Essays in the History of Mechanics (1968), p. 99.
- ^ In the preface to the Marquis de L’Hospital’s Analyse des Infiniment Petits (Paris, 1696).
- ^ Starting with De motu corporum in gyrum, see also (Latin) Theorem 1 Archived 12 May 2016 at the Wayback Machine.
- ^ Whiteside, D.T., ed. (1970). «The Mathematical principles underlying Newton’s Principia Mathematica». Journal for the History of Astronomy. 1. Cambridge University Press. pp. 116–138.
- ^ Stewart 2009, p. 107.
- ^ Westfall 1980, pp. 538–539.
- ^ Stern, Keith (2009). Queers in history : the comprehensive encyclopedia of historical gays, lesbians, bisexuals, and transgenders. Dallas, Tex.: BenBella. ISBN 978-1-933771-87-8. OCLC 317453194.
- ^ Nowlan, Robert (2017). Masters of Mathematics: The Problems They Solved, Why These Are Important, and What You Should Know about Them. Rotterdam: Sense Publishers. p. 136. ISBN 978-94-6300-891-4.
- ^ Ball 1908, p. 356.
- ^ Błaszczyk, P.; et al. (March 2013). «Ten misconceptions from the history of analysis and their debunking». Foundations of Science. 18 (1): 43–74. arXiv:1202.4153. doi:10.1007/s10699-012-9285-8. S2CID 119134151.
- ^ Westfall 1980, p. 179.
- ^ White 1997, p. 151.
- ^ King, Henry C (2003). The History of the Telescope. p. 74. ISBN 978-0-486-43265-6.
- ^ Whittaker, E.T., A History of the Theories of Aether and Electricity, Dublin University Press, 1910.
- ^ Olivier Darrigol (2012). A History of Optics from Greek Antiquity to the Nineteenth Century. Oxford University Press. p. 81. ISBN 978-0-19-964437-7.
- ^ Newton, Isaac. «Hydrostatics, Optics, Sound and Heat». Cambridge University Digital Library. Archived from the original on 8 January 2012. Retrieved 10 January 2012.
- ^ Ball 1908, p. 324.
- ^ William R. Newman, «Newton’s Early Optical Theory and its Debt to Chymistry», in Danielle Jacquart and Michel Hochmann, eds., Lumière et vision dans les sciences et dans les arts (Geneva: Droz, 2010), pp. 283–307. A free access online version of this article can be found at the Chymistry of Isaac Newton project Archived 28 May 2016 at the Wayback Machine (PDF)
- ^ Ball 1908, p. 325.
- ^ «The Early Period (1608–1672)». James R. Graham’s Home Page. Retrieved 3 February 2009.[permanent dead link]
- ^ a b White 1997, p. 170
- ^ Hall, Alfred Rupert (1996). Isaac Newton: adventurer in thought. Cambridge University Press. p. 67. ISBN 978-0-521-56669-8. OCLC 606137087.
This is the one dated 23 February 1669, in which Newton described his first reflecting telescope, constructed (it seems) near the close of the previous year.
- ^ White 1997, p. 168.
- ^ Newton, Isaac. «Of Colours». The Newton Project. Archived from the original on 9 October 2014. Retrieved 6 October 2014.
- ^ a b See ‘Correspondence of Isaac Newton, vol. 2, 1676–1687’ ed. H.W. Turnbull, Cambridge University Press 1960; at p. 297, document No. 235, letter from Hooke to Newton dated 24 November 1679.
- ^ Iliffe, Robert (2007) Newton. A very short introduction, Oxford University Press 2007
- ^ a b Westfall, Richard S. (1983) [1980]. Never at Rest: A Biography of Isaac Newton. Cambridge: Cambridge University Press. pp. 530–531. ISBN 978-0-521-27435-7.
- ^ a b Keynes, John Maynard (1972). «Newton, The Man». The Collected Writings of John Maynard Keynes Volume X. MacMillan St. Martin’s Press. pp. 363–366.
- ^ Dobbs, J.T. (December 1982). «Newton’s Alchemy and His Theory of Matter». Isis. 73 (4): 523. doi:10.1086/353114. S2CID 170669199. quoting Opticks
- ^ Opticks, 2nd Ed 1706. Query 8.
- ^ a b Duarte, F.J. (2000). «Newton, prisms, and the ‘opticks’ of tunable lasers» (PDF). Optics and Photonics News. 11 (5): 24–25. Bibcode:2000OptPN..11…24D. doi:10.1364/OPN.11.5.000024. Archived (PDF) from the original on 17 February 2015. Retrieved 17 February 2015.
- ^ Tyndall, John. (1880). Popular Science Monthly Volume 17, July. s:Popular Science Monthly/Volume 17/July 1880/Goethe’s Farbenlehre: Theory of Colors II
- ^ Westfall 1980, pp. 391–392.
- ^ Whiteside, D.T., ed. (1974). Mathematical Papers of Isaac Newton, 1684–1691. 6. Cambridge University Press. p. 30.
- ^ a b Schmitz, Kenneth S. (2018). Physical Chemistry: Multidisciplinary Applications in Society. Amsterdam: Elsevier. p. 251. ISBN 978-0-12-800599-6. Archived from the original on 10 March 2020. Retrieved 1 March 2020.
- ^ Brewster, Sir David Memoirs of the Life, Writings, and Discoveries of Sir Isaac Newton, Volume 2. Edinburgh: 1860 p108
- ^ See Curtis Wilson, «The Newtonian achievement in astronomy», pp. 233–274 in R Taton & C Wilson (eds) (1989) The General History of Astronomy, Volume, 2A’, at p. 233 Archived 3 October 2015 at the Wayback Machine.
- ^ Text quotations are from 1729 translation of Newton’s Principia, Book 3 (1729 vol.2) at pp. 232–233.
- ^ Edelglass et al., Matter and Mind, ISBN 0-940262-45-2. p. 54
- ^ On the meaning and origins of this expression, see Kirsten Walsh, Does Newton feign an hypothesis? Archived 14 July 2014 at the Wayback Machine, Early Modern Experimental Philosophy Archived 21 July 2011 at the Wayback Machine, 18 October 2010.
- ^ Westfall 1980, Chapter 11.
- ^ a b Professor Robert A. Hatch, University of Florida. «Newton Timeline». Archived from the original on 2 August 2012. Retrieved 13 August 2012.
- ^ Weisstein, Eric W. «Cubic Curve». mathworld.wolfram.com. Archived from the original on 27 January 2021. Retrieved 20 January 2021.
- ^ Conics and Cubics, Robert Bix, Springer Undergraduate Texts in Mathematics, 2nd edition, 2006, Springer Verlag.
- ^ «John Locke Manuscripts – Chronological Listing: 1690». psu.edu. Archived from the original on 9 July 2017. Retrieved 20 January 2013.; and John C. Attig, John Locke Bibliography — Chapter 5, Religion, 1751–1900 Archived 12 November 2012 at the Wayback Machine
- ^ White 1997, p. 232.
- ^ Patrick Sawer (6 September 2016). «What students should avoid during fresher’s week (100 years ago and now)». The Daily Telegraph. Archived from the original on 10 January 2022. Retrieved 7 September 2016.
- ^ «Isaac Newton: Physicist And … Crime Fighter?». Science Friday. 5 June 2009. NPR. Archived from the original on 1 November 2014. Transcript. Retrieved 1 August 2014.
- ^ Levenson, Thomas (2009). Newton and the counterfeiter: the unknown detective career of the world’s greatest scientist. Houghton Mifflin Harcourt. ISBN 978-0-15-101278-7. OCLC 276340857.
- ^ White 1997, p. 259.
- ^ White 1997, p. 267.
- ^ Newton, Isaac. «Philosophiæ Naturalis Principia Mathematica». Cambridge University Digital Library. pp. 265–266. Archived from the original on 8 January 2012. Retrieved 10 January 2012.
- ^ Westfall 2007, p. 73.
- ^ Wagner, Anthony (1972). Historic Heraldry of Britain (2nd ed.). London and Chichester: Phillimore. p. 85. ISBN 978-0-85033-022-9.; and Genealogical Memoranda Relating to the Family of Newton. London: Taylor and Co. 1871.
- ^ White 1997, p. 317.
- ^ «The Queen’s ‘great Assistance’ to Newton’s election was his knighting, an honor bestowed not for his contributions to science, nor for his service at the Mint, but for the greater glory of party politics in the election of 1705.» Westfall 1994, p. 245
- ^ «This Day in History: Isaac Newton is Knighted». History Channel. A&E Television Networks. 20 June 2016. Archived from the original on 19 August 2014. Retrieved 18 August 2014; and Barnham, Kay (2014). Isaac Newton. Raintree. p. 26. ISBN 978-1-4109-6235-5.
- ^ On the Value of Gold and Silver in European Currencies and the Consequences on the Worldwide Gold- and Silver-Trade Archived 6 April 2017 at the Wayback Machine, Sir Isaac Newton, 21 September 1717; «By The King, A Proclamation Declaring the Rates at which Gold shall be current in Payments». Royal Numismatic Society. V. April 1842 – January 1843.
- ^ Fay, C.R. (1 January 1935). «Newton and the Gold Standard». Cambridge Historical Journal. 5 (1): 109–117. doi:10.1017/S1474691300001256. JSTOR 3020836.
- ^ «Sir Isaac Newton’s Unpublished Manuscripts Explain Connections He Made Between Alchemy and Economics». Georgia Tech Research News. 12 September 2006. Archived from the original on 17 February 2013. Retrieved 30 July 2014.
- ^ Eric W. Nye, Pounds Sterling to Dollars: Historical Conversion of Currency Archived 15 August 2021 at the Wayback Machine. Retrieved: 5 October 2020
- ^ Holodny, Elena (21 January 2016). «Isaac Newton was a genius, but even he lost millions in the stock market». Business Insider. Archived from the original on 25 March 2016. Retrieved 21 December 2019.
- ^ Yonge, Charlotte M. (1898). «Cranbury and Brambridge». John Keble’s Parishes – Chapter 6. online-literature.com. Archived from the original on 8 December 2008. Retrieved 23 September 2009.
- ^ Westfall 1980, p. 44.
- ^ Westfall 1980, p. 595.
- ^ «No. 6569». The London Gazette. 1 April 1727. p. 7.
- ^ Dobre and Nyden suggest that there is no clear evidence that Voltaire was present; see p. 89 of Mihnea Dobre, Tammy Nyden (2013). Cartesian Empiricism. Springer. ISBN 978-94-007-7690-6.
- ^ a b «Newton, Isaac (1642–1727)». Eric Weisstein’s World of Biography. Eric W. Weisstein. Archived from the original on 28 April 2006. Retrieved 30 August 2006.
- ^ a b c d Mann, Adam (14 May 2014). «The Strange, Secret History of Isaac Newton’s Papers». Science. Archived from the original on 11 September 2017. Retrieved 25 April 2016.
- ^ Hutton, Charles (1795/6). A Mathematical and Philosophical Dictionary. vol. 2. p. 100.
- ^ Voltaire (1894). «14». Letters on England. Cassell. p. 100.
- ^ Hutton, Charles (1815). A Philosophical and Mathematical Dictionary Containing … Memoirs of the Lives and Writings of the Most Eminent Authors, Volume 2. p. 100.
- ^ Keynes, John Maynard. «Newton: the Man». University of St Andrews School of Mathematics and Statistics. Archived from the original on 17 June 2019. Retrieved 11 September 2012.
- ^ Sagan, Carl (1980). Cosmos. New York: Random House. p. 68. ISBN 978-0-394-50294-6.
- ^ «Duillier, Nicholas Fatio de (1664–1753) mathematician and natural philosopher». Janus database. Archived from the original on 1 July 2013. Retrieved 22 March 2013.
- ^ «Collection Guide: Fatio de Duillier, Nicolas [Letters to Isaac Newton]». Online Archive of California. Archived from the original on 31 May 2013. Retrieved 22 March 2013.
- ^ Westfall 1980, pp. 493–497 on the friendship with Fatio, pp. 531–540 on Newton’s breakdown.
- ^ Manuel 1968, p. 219.
- ^ Letter from Isaac Newton to Robert Hooke, 5 February 1676, as transcribed in Maury, Jean-Pierre (1992) [1990]. Newton: Understanding the Cosmos. «New Horizons» series. Translated by Paris, I. Mark. London: Thames & Hudson. ISBN 978-0-500-30023-7. Archived from the original on 21 December 2020. Retrieved 18 October 2020.
- ^ John Gribbin (2002) Science: A History 1543–2001, p. 164.
- ^ White 1997, p. 187.
- ^ Memoirs of the Life, Writings, and Discoveries of Sir Isaac Newton (1855) by Sir David Brewster (Volume II. Ch. 27)
- ^ «Is Islam Hostile to Science?». National Geographic News. 27 February 2015. Archived from the original on 9 January 2021. Retrieved 5 January 2021.
- ^ James, Ioan (January 2003). «Singular scientists». Journal of the Royal Society of Medicine. 96 (1): 36–39. doi:10.1177/014107680309600112. PMC 539373. PMID 12519805.
- ^ «Einstein and Newton showed signs of autism». Archived from the original on 18 June 2017. Retrieved 29 September 2021.
- ^ «Understanding Level 1 of Autism Spectrum Disorder | Ascend Autism». ascendautism.com. 12 February 2021. Retrieved 8 January 2023.
- ^ Richard S. Westfall – Indiana University The Galileo Project. (Rice University). Archived from the original on 29 September 2020. Retrieved 5 July 2008.
- ^ a b c d e Snobelen, Stephen D. (December 1999). «Isaac Newton, heretic: the strategies of a Nicodemite». The British Journal for the History of Science. 32 (4): 381–419. doi:10.1017/S0007087499003751. JSTOR 4027945. S2CID 145208136.
- ^ Westfall 1980, p. 315.
- ^ Westfall 1980, p. 321.
- ^ Westfall 1980, pp. 331–334.
- ^ Westfall 1994, p. 124.
- ^ Avery Cardinal Dulles. The Deist Minimum Archived 6 October 2013 at the Wayback Machine (January 2005).
- ^ «Newton, object 1 (Butlin 306) «Newton»«. William Blake Archive. 25 September 2013. Archived from the original on 27 September 2013. Retrieved 25 September 2013.
- ^ Newton, Isaac (1782). Isaaci Newtoni Opera quae exstant omnia. London: Joannes Nichols. pp. 436–437. Archived from the original on 14 April 2021. Retrieved 18 October 2020.
- ^ Observations upon the Prophecies of Daniel, and the Apocalypse of St. John Archived 20 January 2017 at the Wayback Machine 1733
- ^ John P. Meier, A Marginal Jew, v. 1, pp. 382–402. after narrowing the years to 30 or 33, provisionally judges 30 most likely.
- ^ Newton to Richard Bentley 10 December 1692, in Turnbull et al. (1959–77), vol 3, p. 233.
- ^ Opticks, 2nd Ed 1706. Query 31.
- ^ H.G. Alexander (ed) The Leibniz-Clarke correspondence, Manchester University Press, 1998, p. 11.
- ^ Tyson, Neil Degrasse (1 November 2005). «The Perimeter of Ignorance». Natural History Magazine. Archived from the original on 6 September 2018. Retrieved 7 January 2016.
- ^ Dijksterhuis, E. J. The Mechanization of the World Picture, IV 329–330, Oxford University Press, 1961. The author’s final comment on this episode is:»The mechanization of the world picture led with irresistible coherence to the conception of God as a sort of ‘retired engineer’, and from here to God’s complete elimination it took just one more step».
- ^ Brewster states that Newton was never known as an Arian during his lifetime, it was William Whiston, an Arian, who first argued that «Sir Isaac Newton was so hearty for the Baptists, as well as for the Eusebians or Arians, that he sometimes suspected these two were the two witnesses in the Revelations,» while others like Hopton Haynes (a Mint employee and Humanitarian), «mentioned to Richard Baron, that Newton held the same doctrine as himself». David Brewster. Memoirs of the Life, Writings, and Discoveries of Sir Isaac Newton. p. 268.
- ^ Jacob, Margaret C. (1976). The Newtonians and the English Revolution: 1689–1720. Cornell University Press. pp. 37, 44. ISBN 978-0-85527-066-7.
- ^ Westfall, Richard S. (1958). Science and Religion in Seventeenth-Century England. New Haven: Yale University Press. p. 200. ISBN 978-0-208-00843-5.
- ^ Haakonssen, Knud (1996). «The Enlightenment, politics and providence: some Scottish and English comparisons». In Martin Fitzpatrick (ed.). Enlightenment and Religion: Rational Dissent in Eighteenth-century Britain. Cambridge: Cambridge University Press. p. 64. ISBN 978-0-521-56060-3.
- ^ a b Isaac Newton and Apocalypse Now: a response to Tom Harpur’s «Newton’s strange bedfellows» Archived 27 September 2017 at the Wayback Machine (PDF) Stephen D. Snobelen
- ^ Grayling, A.C. (2016). The Age of Genius: The Seventeenth Century and the Birth of the Modern Mind. ISBN 978-1-4088-4329-1. Archived from the original on 14 April 2021. Retrieved 18 October 2020.
- ^ «Papers Show Isaac Newton’s Religious Side, Predict Date of Apocalypse». Associated Press. 19 June 2007. Archived from the original on 13 August 2007. Retrieved 1 August 2007.
- ^ Leiber, Fritz (1981). «Poor Superman». In Heinlein, Robert A. (ed.). Tomorrow, the Stars (16th ed.). New York: Berkley Book / published by arrangement with Doubleday & Company, Inc. p. 208.
First published in Galaxy magazine, July 1951; Variously titled Appointment in Tomorrow; in some reprints of Leiber’s story the sentence ‘That was the pebble..’ is replaced by ‘Which Newton did the world need then?’
- ^ Meyer, Michal (2014). «Gold, secrecy and prestige». Chemical Heritage Magazine. 32 (1): 42–43. Archived from the original on 20 March 2018. Retrieved 20 March 2018.
- ^ a b Kean, Sam (2011). «Newton, The Last Magician». Humanities. 32 (1). Archived from the original on 13 April 2016. Retrieved 25 April 2016.
- ^ Greshko, Michael (4 April 2016). «Isaac Newton’s Lost Alchemy Recipe Rediscovered». National Geographic. Archived from the original on 26 April 2016. Retrieved 25 April 2016.
- ^ Dry, Sarah (2014). The Newton papers : the strange and true odyssey of Isaac Newton’s manuscripts. Oxford: Oxford University Press. ISBN 978-0-19-995104-8.
- ^ a b «The Chymistry of Isaac Newton». Indiana University, Bloomington. Archived from the original on 26 April 2016. Retrieved 25 April 2016.
- ^ Levitin, Dimitri (March 2019). «Going for Gold». Literary Review. Archived from the original on 7 March 2019. Retrieved 6 March 2019.
- ^ Newman, William R (2018). Newton the Alchemist Science, Enigma, and the Quest for Nature’s «Secret Fire». Princeton University Press. ISBN 978-0-691-17487-7. Archived from the original on 2 April 2019. Retrieved 6 March 2019.
- ^ Gillispie, Charles Coulston (1960). The Edge of Objectivity: An Essay in the History of Scientific Ideas. Princeton University Press. p. 122. ISBN 0-691-02350-6.
- ^ Van Helmont, Iohannis Baptistae, Opuscula Medica Inaudita: IV. De Peste, Editor Hieronymo Christian Paullo (Frankfurt am Main) Publisher Sumptibus Hieronimi Christiani Pauli, typis Matthiæ Andreæ, 1707.
- ^ Flood, Alison (2 June 2020). «Isaac Newton proposed curing plague with toad vomit, unseen papers show». The Guardian. Archived from the original on 6 June 2020. Retrieved 6 June 2020.
- ^ Fred L. Wilson, History of Science: Newton citing: Delambre, M. «Notice sur la vie et les ouvrages de M. le comte J.L. Lagrange», Oeuvres de Lagrange I. Paris, 1867, p. xx.
- ^ a b Westminster Abbey. «Sir Isaac Newton Scientist, Mathematician and Astronomer». westminster-abbey.org. Archived from the original on 9 August 2022. Retrieved 19 January 2022.
- ^ «Newton beats Einstein in polls of Royal Society scientists and the public». The Royal Society. Archived from the original on 13 July 2017. Retrieved 24 August 2010.
- ^ «Opinion poll. Einstein voted ‘greatest physicist ever’ by leading physicists; Newton runner-up». BBC News. 29 November 1999. Archived from the original on 12 August 2017. Retrieved 17 January 2012.
- ^ Gleeson-White, Jane (10 November 2003). «Einstein’s Heroes». The Sydney Morning Herald. Archived from the original on 28 November 2019. Retrieved 29 September 2021.
- ^ a b Historic England. «Woolsthorpe Manor House, Colsterworth (1062362)». National Heritage List for England. Retrieved 5 October 2021.
- ^ «Silly relic-worship». The New York Times. 16 January 1881. p. 10. Archived from the original on 20 January 2019. Retrieved 12 July 2009.
- ^ a b Cunningham, Antonia, ed. (2002). Guinness World Records 2002. ISBN 978-0-553-58378-6. Archived from the original on 9 August 2022. Retrieved 12 July 2009.
- ^ White 1997, p. 86.
- ^ Numbers 2015, pp. 48–56.
- ^ Malament, David B. (2002). Reading Natural Philosophy: Essays in the History and Philosophy of Science and Mathematics. Open Court Publishing. ISBN 978-0-8126-9507-6. Archived from the original on 14 April 2021. Retrieved 18 October 2020.
- ^ Voltaire (1727). An Essay upon the Civil Wars of France, extracted from curious Manuscripts and also upon the Epick Poetry of the European Nations, from Homer down to Milton. London, England: Samuel Jallasson. p. 104. Archived from the original on 14 June 2021. Retrieved 14 June 2021. From p. 104: ‘In the like Manner Pythagoras ow’d the Invention of Musik to the noise of the Hammer of a Blacksmith. And thus in our Days Sir Isaak Newton walking in his Garden had the first Thought of his System of Gravitation, upon seeing an apple falling from a Tree.’
- ^ Voltaire (1786) heard the story of Newton and the apple tree from Newton’s niece, Catherine Conduit (née Barton) (1679–1740): Voltaire (1786). Oeuvres completes de Voltaire [The complete works of Voltaire] (in French). Vol. 31. Basel, Switzerland: Jean-Jacques Tourneisen. p. 175. Archived from the original on 9 July 2021. Retrieved 15 June 2021. From p. 175: «Un jour en l’année 1666, Newton retiré à la campagne, et voyant tomber des fruits d’un arbre, à ce que m’a conté sa nièce, (Mme Conduit) se laissa aller à une méditation profonde sur la cause qui entraine ainsi tous les corps dans une ligne, qui, si elle était prolongée, passerait à peu près par le centre de la terre.» (One day in the year 1666 Newton withdrew to the country, and seeing the fruits of a tree fall, according to what his niece (Madame Conduit) told me, he entered into a deep meditation on the cause that draws all bodies in a [straight] line, which, if it were extended, would pass very near to the center of the Earth.)
- ^ Berkun, Scott (2010). The Myths of Innovation. O’Reilly Media, Inc. p. 4. ISBN 978-1-4493-8962-8.
- ^ «Newton’s apple: The real story». New Scientist. 18 January 2010. Archived from the original on 21 January 2010. Retrieved 10 May 2010.
- ^ Hamblyn, Richard (2011). «Newtonian Apples: William Stukeley». The Art of Science. Pan Macmillan. ISBN 978-1-4472-0415-2.
- ^ «Revised Memoir of Newton (Normalized Version)». The Newton Project. Archived from the original on 14 March 2017. Retrieved 13 March 2017.
- ^ Conduitt, John. «Keynes Ms. 130.4:Conduitt’s account of Newton’s life at Cambridge». Newtonproject. Imperial College London. Archived from the original on 7 November 2009. Retrieved 30 August 2006.
- ^ I. Bernard Cohen and George E. Smith, eds. The Cambridge Companion to Newton (2002) p. 6
- ^ Alberto A. Martinez Science Secrets: The Truth about Darwin’s Finches, Einstein’s Wife, and Other Myths, p. 69 (University of Pittsburgh Press, 2011); ISBN 978-0-8229-4407-2
- ^ «Brogdale – Home of the National Fruit Collection». Brogdale.org. Archived from the original on 1 December 2008. Retrieved 20 December 2008.
- ^ «From the National Fruit Collection: Isaac Newton’s Tree». Retrieved 10 January 2009.[permanent dead link] Alternate Page Archived 5 July 2022 at the Wayback Machine Retrieved 5 July 2022.
- ^ ‘The Abbey Scientists’ Hall, A.R. p13: London; Roger & Robert Nicholson; 1966
- ^ a b «Famous People & the Abbey: Sir Isaac Newton». Westminster Abbey. Archived from the original on 16 October 2009. Retrieved 13 November 2009.
- ^ «Withdrawn banknotes reference guide». Bank of England. Archived from the original on 5 May 2010. Retrieved 27 August 2009.
- ^ Cassels, Alan. Ideology and International Relations in the Modern World. p. 2.
- ^ «Although it was just one of the many factors in the Enlightenment, the success of Newtonian physics in providing a mathematical description of an ordered world clearly played a big part in the flowering of this movement in the eighteenth century» by John Gribbin, Science: A History 1543–2001 (2002), p. 241 ISBN 978-0-7139-9503-9
- ^ Anders Hald 2003 – A history of probability and statistics and their applications before 1750 – 586 pages Volume 501 of Wiley series in probability and statistics Wiley-IEEE, 2003 Archived 2 June 2022 at the Wayback Machine Retrieved 27 January 2012 ISBN 0-471-47129-1
- ^ «Natures obvious laws & processes in vegetation – Introduction». The Chymistry of Isaac Newton. Archived from the original on 17 January 2021. Retrieved 17 January 2021. Transcribed and online at Indiana University.
- ^ Whiteside, D.T., ed. (1974). Mathematical Papers of Isaac Newton, 1684–1691. 6. Cambridge University Press. pp. 30–91. Archived 10 June 2016 at the Wayback Machine
- ^ «Museum of London exhibit including facsimile of title page from John Flamsteed’s copy of 1687 edition of Newton’s Principia«. Museumoflondon.org.uk. Archived from the original on 31 March 2012. Retrieved 16 March 2012.
- ^ Published anonymously as «Scala graduum Caloris. Calorum Descriptiones & signa.» in Philosophical Transactions, 1701, 824 Archived 21 January 2020 at the Wayback Machine–829;
ed. Joannes Nichols, Isaaci Newtoni Opera quae exstant omnia, vol. 4 (1782), 403 Archived 17 June 2016 at the Wayback Machine–407.
Mark P. Silverman, A Universe of Atoms, An Atom in the Universe, Springer, 2002, p. 49. Archived 24 June 2016 at the Wayback Machine - ^ Newton, Isaac (1704). Opticks or, a Treatise of the reflexions, refractions, inflexions and colours of light. Also two treatises of the species and magnitude of curvilinear figures. Sam. Smith. and Benj. Walford. Archived from the original on 24 February 2021. Retrieved 17 March 2018.
- ^ a b c d e f g Pickover, Clifford (2008). Archimedes to Hawking: Laws of Science and the Great Minds Behind Them. Oxford University Press. pp. 117–118. ISBN 978-0-19-979268-9. Retrieved 17 March 2018.
- ^ Swetz, Frank J. «Mathematical Treasure: Newton’s Method of Fluxions». Convergence. Mathematical Association of America. Archived from the original on 28 June 2017. Retrieved 17 March 2018.
Bibliography
- Ball, W.W. Rouse (1908). A Short Account of the History of Mathematics. New York: Dover. ISBN 978-0-486-20630-1.
- Christianson, Gale (1984). In the Presence of the Creator: Isaac Newton & His Times. New York: Free Press. ISBN 978-0-02-905190-0. This well documented work provides, in particular, valuable information regarding Newton’s knowledge of Patristics
- Craig, John (1958). «Isaac Newton – Crime Investigator». Nature. 182 (4629): 149–152. Bibcode:1958Natur.182..149C. doi:10.1038/182149a0. S2CID 4200994.
- Craig, John (1963). «Isaac Newton and the Counterfeiters». Notes and Records of the Royal Society of London. 18 (2): 136–145. doi:10.1098/rsnr.1963.0017. S2CID 143981415.
- Gjertsen, Derek (1986). The Newton Handbook. London: Routledge & Kegan Paul. ISBN 0-7102-0279-2.
- Levenson, Thomas (2010). Newton and the Counterfeiter: The Unknown Detective Career of the World’s Greatest Scientist. Mariner Books. ISBN 978-0-547-33604-6.
- Manuel, Frank E (1968). A Portrait of Isaac Newton. Belknap Press of Harvard University, Cambridge, MA.
- Stewart, James (2009). Calculus: Concepts and Contexts. Cengage Learning. ISBN 978-0-495-55742-5.
- Westfall, Richard S. (1980). Never at Rest. Cambridge University Press. ISBN 978-0-521-27435-7.
- Westfall, Richard S. (2007). Isaac Newton. Cambridge University Press. ISBN 978-0-19-921355-9.
- Westfall, Richard S. (1994). The Life of Isaac Newton. Cambridge University Press. ISBN 978-0-521-47737-6.
- White, Michael (1997). Isaac Newton: The Last Sorcerer. Fourth Estate Limited. ISBN 978-1-85702-416-6.
Further reading
Primary
- Newton, Isaac. The Principia: Mathematical Principles of Natural Philosophy. University of California Press, (1999)
- Brackenridge, J. Bruce. The Key to Newton’s Dynamics: The Kepler Problem and the Principia: Containing an English Translation of Sections 1, 2, and 3 of Book One from the First (1687) Edition of Newton’s Mathematical Principles of Natural Philosophy, University of California Press (1996)
- Newton, Isaac. The Optical Papers of Isaac Newton. Vol. 1: The Optical Lectures, 1670–1672, Cambridge University Press (1984)
- Newton, Isaac. Opticks (4th ed. 1730) online edition
- Newton, I. (1952). Opticks, or A Treatise of the Reflections, Refractions, Inflections & Colours of Light. New York: Dover Publications.
- Newton, I. Sir Isaac Newton’s Mathematical Principles of Natural Philosophy and His System of the World, tr. A. Motte, rev. Florian Cajori. Berkeley: University of California Press (1934)
- Whiteside, D.T., ed. (1967–1982). The Mathematical Papers of Isaac Newton. Cambridge: Cambridge University Press. ISBN 978-0-521-07740-8. – 8 volumes.
- Newton, Isaac. The correspondence of Isaac Newton, ed. H.W. Turnbull and others, 7 vols (1959–77)
- Newton’s Philosophy of Nature: Selections from His Writings edited by H.S. Thayer (1953; online edition)
- Isaac Newton, Sir; J Edleston; Roger Cotes, Correspondence of Sir Isaac Newton and Professor Cotes, including letters of other eminent men, London, John W. Parker, West Strand; Cambridge, John Deighton (1850, Google Books)
- Maclaurin, C. (1748). An Account of Sir Isaac Newton’s Philosophical Discoveries, in Four Books. London: A. Millar and J. Nourse
- Newton, I. (1958). Isaac Newton’s Papers and Letters on Natural Philosophy and Related Documents, eds. I.B. Cohen and R.E. Schofield. Cambridge: Harvard University Press
- Newton, I. (1962). The Unpublished Scientific Papers of Isaac Newton: A Selection from the Portsmouth Collection in the University Library, Cambridge, ed. A.R. Hall and M.B. Hall. Cambridge: Cambridge University Press
- Newton, I. (1975). Isaac Newton’s ‘Theory of the Moon’s Motion’ (1702). London: Dawson
Alchemy
- Craig, John (1946). Newton at the Mint. Cambridge, England: Cambridge University Press.
- Craig, John (1953). «XII. Isaac Newton». The Mint: A History of the London Mint from A.D. 287 to 1948. Cambridge, England: Cambridge University Press. pp. 198–222. ASIN B0000CIHG7.
- de Villamil, Richard (1931). Newton, the Man. London: G.D. Knox. – Preface by Albert Einstein. Reprinted by Johnson Reprint Corporation, New York (1972)
- Dobbs, B.J.T. (1975). The Foundations of Newton’s Alchemy or «The Hunting of the Greene Lyon». Cambridge: Cambridge University Press.
- Keynes, John Maynard (1963). Essays in Biography. W.W. Norton & Co. ISBN 978-0-393-00189-1. Keynes took a close interest in Newton and owned many of Newton’s private papers.
- Stukeley, W. (1936). Memoirs of Sir Isaac Newton’s Life. London: Taylor and Francis. (edited by A.H. White; originally published in 1752)
- Trabue, J. «Ann and Arthur Storer of Calvert County, Maryland, Friends of Sir Isaac Newton,» The American Genealogist 79 (2004): 13–27.
Religion
- Dobbs, Betty Jo Tetter. The Janus Faces of Genius: The Role of Alchemy in Newton’s Thought. (1991), links the alchemy to Arianism
- Force, James E., and Richard H. Popkin, eds. Newton and Religion: Context, Nature, and Influence. (1999), pp. xvii, 325.; 13 papers by scholars using newly opened manuscripts
- Pfizenmaier, Thomas C (1997). «Was Isaac Newton an Arian?». Journal of the History of Ideas. 58 (1): 57–80. doi:10.1353/jhi.1997.0001. JSTOR 3653988. S2CID 170545277.
- Ramati, Ayval (2001). «The Hidden Truth of Creation: Newton’s Method of Fluxions». The British Journal for the History of Science. 34 (4): 417–438. doi:10.1017/S0007087401004484. JSTOR 4028372. S2CID 143045863.
- Snobelen, Stephen D. (2001). «‘God of Gods, and Lord of Lords’: The Theology of Isaac Newton’s General Scholium to the Principia». Osiris. 16: 169–208. Bibcode:2001Osir…16..169S. doi:10.1086/649344. JSTOR 301985. S2CID 170364912.
- Snobelen, Stephen D. (December 1999). «Isaac Newton, heretic: the strategies of a Nicodemite». The British Journal for the History of Science. 32 (4): 381–419. doi:10.1017/S0007087499003751. JSTOR 4027945. S2CID 145208136.
Science
- Bechler, Zev (2013). Contemporary Newtonian Research (Studies in the History of Modern Science)(Volume 9). Springer. ISBN 978-94-009-7717-4.
- Berlinski, David. Newton’s Gift: How Sir Isaac Newton Unlocked the System of the World. (2000); ISBN 0-684-84392-7
- Chandrasekhar, Subrahmanyan (1995). Newton’s Principia for the Common Reader. Oxford: Clarendon Press. ISBN 978-0-19-851744-3.
- Cohen, I. Bernard and Smith, George E., ed. The Cambridge Companion to Newton. (2002). Focuses on philosophical issues only; excerpt and text search; complete edition online «The Cambridge Companion to Newton». Archived from the original on 8 October 2008. Retrieved 13 October 2008.
{{cite web}}: CS1 maint: bot: original URL status unknown (link) - Cohen, I.B. (1980). The Newtonian Revolution. Cambridge: Cambridge University Press. ISBN 978-0-521-22964-7.
- Gleick, James (2003). Isaac Newton. Alfred A. Knopf. ISBN 978-0-375-42233-1.
- Halley, E. (1687). «Review of Newton’s Principia». Philosophical Transactions. 186: 291–297.
- Hawking, Stephen, ed. On the Shoulders of Giants. ISBN 0-7624-1348-4 Places selections from Newton’s Principia in the context of selected writings by Copernicus, Kepler, Galileo and Einstein
- Herivel, J.W. (1965). The Background to Newton’s Principia. A Study of Newton’s Dynamical Researches in the Years 1664–84. Oxford: Clarendon Press.
- Newton, Isaac. Papers and Letters in Natural Philosophy, edited by I. Bernard Cohen. Harvard University Press, 1958, 1978; ISBN 0-674-46853-8.
- Numbers, R.L. (2015). Newton’s Apple and Other Myths about Science. Harvard University Press. ISBN 978-0-674-91547-3.
- Pemberton, H. (1728). «A View of Sir Isaac Newton’s Philosophy». The Physics Teacher. 4 (1): 8–9. Bibcode:1966PhTea…4….8M. doi:10.1119/1.2350900.
- Shamos, Morris H. (1959). Great Experiments in Physics. New York: Henry Holt and Company, Inc. ISBN 978-0-486-25346-6.
External links
This audio file was created from a revision of this article dated 30 July 2008, and does not reflect subsequent edits.
Writings by Newton
- Newton’s works – full texts, at the Newton Project
- Newton’s papers in the Royal Society’s archives
- The Newton Manuscripts at the National Library of Israel – the collection of all his religious writings
- Works by Isaac Newton at Project Gutenberg
- Works by or about Isaac Newton at Internet Archive
- Works by Isaac Newton at LibriVox (public domain audiobooks)
- «Newton Papers» – Cambridge Digital Library
This article is about the scientist and mathematician. For the American agriculturalist, see Isaac Newton (agriculturalist).
|
Sir Isaac Newton PRS |
|
|---|---|

Portrait of Newton at 46 by Godfrey Kneller, 1689 |
|
| Born | 4 January 1643 [O.S. 25 December 1642][a]
Woolsthorpe-by-Colsterworth, Lincolnshire, England |
| Died | 31 March 1727 (aged 84) [O.S. 20 March 1726][a]
Kensington, Middlesex, Great Britain |
| Resting place | Westminster Abbey |
| Education | Trinity College, Cambridge (M.A., 1668)[2] |
| Known for |
List
|
| Awards |
|
| Scientific career | |
| Fields |
|
| Institutions |
|
| Academic advisors |
|
| Notable students |
|
| Influences |
|
| Influenced |
List
|
| Member of Parliament for the University of Cambridge | |
| In office 1689–1690 |
|
| Preceded by | Robert Brady |
| Succeeded by | Edward Finch |
| In office 1701–1702 |
|
| Preceded by | Anthony Hammond |
| Succeeded by | Arthur Annesley, 5th Earl of Anglesey |
| 12th President of the Royal Society | |
| In office 1703–1727 |
|
| Preceded by | John Somers |
| Succeeded by | Hans Sloane |
| Master of the Mint | |
| In office 1699–1727 |
|
| 1696–1699 | Warden of the Mint |
| Preceded by | Thomas Neale |
| Succeeded by | John Conduitt |
| 2nd Lucasian Professor of Mathematics | |
| In office 1669–1702 |
|
| Preceded by | Isaac Barrow |
| Succeeded by | William Whiston |
| Personal details | |
| Political party | Whig |
| Signature | |
 |
Sir Isaac Newton PRS (25 December 1642 – 20 March 1726/27)[a] was an English mathematician, physicist, astronomer, alchemist, theologian, and author (described in his time as a «natural philosopher»), widely recognised as one of the greatest mathematicians and physicists and among the most influential scientists of all time. He was a key figure in the philosophical revolution known as the Enlightenment. His book Philosophiæ Naturalis Principia Mathematica (Mathematical Principles of Natural Philosophy), first published in 1687, established classical mechanics. Newton also made seminal contributions to optics, and shares credit with German mathematician Gottfried Wilhelm Leibniz for developing infinitesimal calculus.
In the Principia, Newton formulated the laws of motion and universal gravitation that formed the dominant scientific viewpoint for centuries until it was superseded by the theory of relativity. Newton used his mathematical description of gravity to derive Kepler’s laws of planetary motion, account for tides, the trajectories of comets, the precession of the equinoxes and other phenomena, eradicating doubt about the Solar System’s heliocentricity. He demonstrated that the motion of objects on Earth and celestial bodies could be accounted for by the same principles. Newton’s inference that the Earth is an oblate spheroid was later confirmed by the geodetic measurements of Maupertuis, La Condamine, and others, convincing most European scientists of the superiority of Newtonian mechanics over earlier systems.
Newton built the first practical reflecting telescope and developed a sophisticated theory of colour based on the observation that a prism separates white light into the colours of the visible spectrum. His work on light was collected in his highly influential book Opticks, published in 1704. He also formulated an empirical law of cooling, made the first theoretical calculation of the speed of sound, and introduced the notion of a Newtonian fluid. In addition to his work on calculus, as a mathematician Newton contributed to the study of power series, generalised the binomial theorem to non-integer exponents, developed a method for approximating the roots of a function, and classified most of the cubic plane curves.
Newton was a fellow of Trinity College and the second Lucasian Professor of Mathematics at the University of Cambridge. He was a devout but unorthodox Christian who privately rejected the doctrine of the Trinity. He refused to take holy orders in the Church of England, unlike most members of the Cambridge faculty of the day. Beyond his work on the mathematical sciences, Newton dedicated much of his time to the study of alchemy and biblical chronology, but most of his work in those areas remained unpublished until long after his death. Politically and personally tied to the Whig party, Newton served two brief terms as Member of Parliament for the University of Cambridge, in 1689–1690 and 1701–1702. He was knighted by Queen Anne in 1705 and spent the last three decades of his life in London, serving as Warden (1696–1699) and Master (1699–1727) of the Royal Mint, as well as president of the Royal Society (1703–1727).
Early life
Early life
Isaac Newton was born (according to the Julian calendar in use in England at the time) on Christmas Day, 25 December 1642 (NS 4 January 1643[a]), «an hour or two after midnight»,[17] at Woolsthorpe Manor in Woolsthorpe-by-Colsterworth, a hamlet in the county of Lincolnshire. His father, also named Isaac Newton, had died three months before. Born prematurely, Newton was a small child; his mother Hannah Ayscough reportedly said that he could have fit inside a quart mug.[18] When Newton was three, his mother remarried and went to live with her new husband, the Reverend Barnabas Smith, leaving her son in the care of his maternal grandmother, Margery Ayscough (née Blythe). Newton disliked his stepfather and maintained some enmity towards his mother for marrying him, as revealed by this entry in a list of sins committed up to the age of 19: «Threatening my father and mother Smith to burn them and the house over them.»[19] Newton’s mother had three children (Mary, Benjamin, and Hannah) from her second marriage.[20]
The King’s School
From the age of about twelve until he was seventeen, Newton was educated at The King’s School in Grantham, which taught Latin and Ancient Greek and probably imparted a significant foundation of mathematics.[21] He was removed from school and returned to Woolsthorpe-by-Colsterworth by October 1659. His mother, widowed for the second time, attempted to make him a farmer, an occupation he hated.[22] Henry Stokes, master at The King’s School, persuaded his mother to send him back to school. Motivated partly by a desire for revenge against a schoolyard bully, he became the top-ranked student,[23] distinguishing himself mainly by building sundials and models of windmills.[24]
University of Cambridge
In June 1661, Newton was admitted to Trinity College at the University of Cambridge. His uncle Reverend William Ayscough, who had studied at Cambridge, recommended him to the university. At Cambridge, Newton started as a subsizar, paying his way by performing valet duties until he was awarded a scholarship in 1664, which covered his university costs for four more years until the completion of his MA.[25] At the time, Cambridge’s teachings were based on those of Aristotle, whom Newton read along with then more modern philosophers, including Descartes and astronomers such as Galileo Galilei and Thomas Street. He set down in his notebook a series of «Quaestiones» about mechanical philosophy as he found it. In 1665, he discovered the generalised binomial theorem and began to develop a mathematical theory that later became calculus. Soon after Newton obtained his BA degree at Cambridge in August 1665, the university temporarily closed as a precaution against the Great Plague. Although he had been undistinguished as a Cambridge student,[26] Newton’s private studies at his home in Woolsthorpe over the next two years saw the development of his theories on calculus,[27] optics, and the law of gravitation.
In April 1667, Newton returned to the University of Cambridge, and in October he was elected as a fellow of Trinity.[28][29] Fellows were required to be ordained as priests, although this was not enforced in the restoration years and an assertion of conformity to the Church of England was sufficient. However, by 1675 the issue could not be avoided and by then his unconventional views stood in the way.[30] Nevertheless, Newton managed to avoid it by means of special permission from Charles II.
His academic work impressed the Lucasian professor Isaac Barrow, who was anxious to develop his own religious and administrative potential (he became master of Trinity College two years later); in 1669, Newton succeeded him, only one year after receiving his MA. Newton was elected a Fellow of the Royal Society (FRS) in 1672.[3]
Work
Calculus
Newton’s work has been said «to distinctly advance every branch of mathematics then studied».[31] His work on the subject, usually referred to as fluxions or calculus, seen in a manuscript of October 1666, is now published among Newton’s mathematical papers.[32] His work De analysi per aequationes numero terminorum infinitas, sent by Isaac Barrow to John Collins in June 1669, was identified by Barrow in a letter sent to Collins that August as the work «of an extraordinary genius and proficiency in these things».[33]
Newton later became involved in a dispute with Leibniz over priority in the development of calculus (the Leibniz–Newton calculus controversy). Most modern historians believe that Newton and Leibniz developed calculus independently, although with very different mathematical notations. Occasionally it has been suggested that Newton published almost nothing about it until 1693, and did not give a full account until 1704, while Leibniz began publishing a full account of his methods in 1684. Leibniz’s notation and «differential Method», nowadays recognised as much more convenient notations, were adopted by continental European mathematicians, and after 1820 or so, also by British mathematicians.[citation needed]
His work extensively uses calculus in geometric form based on limiting values of the ratios of vanishingly small quantities: in the Principia itself, Newton gave demonstration of this under the name of «the method of first and last ratios»[34] and explained why he put his expositions in this form,[35] remarking also that «hereby the same thing is performed as by the method of indivisibles.»[36]
Because of this, the Principia has been called «a book dense with the theory and application of the infinitesimal calculus» in modern times[37] and in Newton’s time «nearly all of it is of this calculus.»[38] His use of methods involving «one or more orders of the infinitesimally small» is present in his De motu corporum in gyrum of 1684[39] and in his papers on motion «during the two decades preceding 1684».[40]
Newton had been reluctant to publish his calculus because he feared controversy and criticism.[41] He was close to the Swiss mathematician Nicolas Fatio de Duillier. In 1691, Duillier started to write a new version of Newton’s Principia, and corresponded with Leibniz.[42] In 1693, the relationship between Duillier and Newton deteriorated and the book was never completed.[43]
Starting in 1699, other members[who?] of the Royal Society accused Leibniz of plagiarism.[44] The dispute then broke out in full force in 1711 when the Royal Society proclaimed in a study that it was Newton who was the true discoverer and labelled Leibniz a fraud; it was later found that Newton wrote the study’s concluding remarks on Leibniz. Thus began the bitter controversy which marred the lives of both Newton and Leibniz until the latter’s death in 1716.[45]
Newton is generally credited with the generalised binomial theorem, valid for any exponent. He discovered Newton’s identities, Newton’s method, classified cubic plane curves (polynomials of degree three in two variables), made substantial contributions to the theory of finite differences, and was the first to use fractional indices and to employ coordinate geometry to derive solutions to Diophantine equations. He approximated partial sums of the harmonic series by logarithms (a precursor to Euler’s summation formula) and was the first to use power series with confidence and to revert power series. Newton’s work on infinite series was inspired by Simon Stevin’s decimals.[46]
When Newton received his MA and became a Fellow of the «College of the Holy and Undivided Trinity» in 1667, he made the commitment that «I will either set Theology as the object of my studies and will take holy orders when the time prescribed by these statutes [7 years] arrives, or I will resign from the college.»[47] Up until this point he had not thought much about religion and had twice signed his agreement to the thirty-nine articles, the basis of Church of England doctrine.
He was appointed Lucasian Professor of Mathematics in 1669, on Barrow’s recommendation. During that time, any Fellow of a college at Cambridge or Oxford was required to take holy orders and become an ordained Anglican priest. However, the terms of the Lucasian professorship required that the holder not be active in the church – presumably,[weasel words] so as to have more time for science. Newton argued that this should exempt him from the ordination requirement, and Charles II, whose permission was needed, accepted this argument; thus, a conflict between Newton’s religious views and Anglican orthodoxy was averted.[48]
Optics
In 1666, Newton observed that the spectrum of colours exiting a prism in the position of minimum deviation is oblong, even when the light ray entering the prism is circular, which is to say, the prism refracts different colours by different angles.[50][51] This led him to conclude that colour is a property intrinsic to light – a point which had, until then, been a matter of debate.
From 1670 to 1672, Newton lectured on optics.[52] During this period he investigated the refraction of light, demonstrating that the multicoloured image produced by a prism, which he named a spectrum, could be recomposed into white light by a lens and a second prism.[53] Modern scholarship has revealed that Newton’s analysis and resynthesis of white light owes a debt to corpuscular alchemy.[54]
He showed that coloured light does not change its properties by separating out a coloured beam and shining it on various objects, and that regardless of whether reflected, scattered, or transmitted, the light remains the same colour. Thus, he observed that colour is the result of objects interacting with already-coloured light rather than objects generating the colour themselves. This is known as Newton’s theory of colour.[55]
Illustration of a dispersive prism separating white light into the colours of the spectrum, as discovered by Newton
From this work, he concluded that the lens of any refracting telescope would suffer from the dispersion of light into colours (chromatic aberration). As a proof of the concept, he constructed a telescope using reflective mirrors instead of lenses as the objective to bypass that problem.[56][57] Building the design, the first known functional reflecting telescope, today known as a Newtonian telescope,[57] involved solving the problem of a suitable mirror material and shaping technique. Newton ground his own mirrors out of a custom composition of highly reflective speculum metal, using Newton’s rings to judge the quality of the optics for his telescopes. In late 1668,[58] he was able to produce this first reflecting telescope. It was about eight inches long and it gave a clearer and larger image. In 1671, the Royal Society asked for a demonstration of his reflecting telescope.[59] Their interest encouraged him to publish his notes, Of Colours,[60] which he later expanded into the work Opticks. When Robert Hooke criticised some of Newton’s ideas, Newton was so offended that he withdrew from public debate. Newton and Hooke had brief exchanges in 1679–80, when Hooke, appointed to manage the Royal Society’s correspondence, opened up a correspondence intended to elicit contributions from Newton to Royal Society transactions,[61] which had the effect of stimulating Newton to work out a proof that the elliptical form of planetary orbits would result from a centripetal force inversely proportional to the square of the radius vector. But the two men remained generally on poor terms until Hooke’s death.[62]
Facsimile of a 1682 letter from Newton to William Briggs, commenting on Briggs’ A New Theory of Vision
Newton argued that light is composed of particles or corpuscles, which were refracted by accelerating into a denser medium. He verged on soundlike waves to explain the repeated pattern of reflection and transmission by thin films (Opticks Bk.II, Props. 12), but still retained his theory of ‘fits’ that disposed corpuscles to be reflected or transmitted (Props.13). However, later physicists favoured a purely wavelike explanation of light to account for the interference patterns and the general phenomenon of diffraction. Today’s quantum mechanics, photons, and the idea of wave–particle duality bear only a minor resemblance to Newton’s understanding of light.
In his Hypothesis of Light of 1675, Newton posited the existence of the ether to transmit forces between particles. The contact with the Cambridge Platonist philosopher Henry More revived his interest in alchemy.[63] He replaced the ether with occult forces based on Hermetic ideas of attraction and repulsion between particles. John Maynard Keynes, who acquired many of Newton’s writings on alchemy, stated that «Newton was not the first of the age of reason: He was the last of the magicians.»[64] Newton’s contributions to science cannot be isolated from his interest in alchemy.[63] This was at a time when there was no clear distinction between alchemy and science, and had he not relied on the occult idea of action at a distance, across a vacuum, he might not have developed his theory of gravity.
In 1704, Newton published Opticks, in which he expounded his corpuscular theory of light. He considered light to be made up of extremely subtle corpuscles, that ordinary matter was made of grosser corpuscles and speculated that through a kind of alchemical transmutation «Are not gross Bodies and Light convertible into one another, … and may not Bodies receive much of their Activity from the Particles of Light which enter their Composition?»[65] Newton also constructed a primitive form of a frictional electrostatic generator, using a glass globe.[66]
In his book Opticks, Newton was the first to show a diagram using a prism as a beam expander, and also the use of multiple-prism arrays.[67] Some 278 years after Newton’s discussion, multiple-prism beam expanders became central to the development of narrow-linewidth tunable lasers. Also, the use of these prismatic beam expanders led to the multiple-prism dispersion theory.[67]
Subsequent to Newton, much has been amended. Young and Fresnel discarded Newton’s particle theory in favour of Huygens’ wave theory to show that colour is the visible manifestation of light’s wavelength. Science also slowly came to realise the difference between perception of colour and mathematisable optics. The German poet and scientist, Goethe, could not shake the Newtonian foundation but «one hole Goethe did find in Newton’s armour, … Newton had committed himself to the doctrine that refraction without colour was impossible. He, therefore, thought that the object-glasses of telescopes must forever remain imperfect, achromatism and refraction being incompatible. This inference was proved by Dollond to be wrong.»[68]
Gravity
In 1679, Newton returned to his work on celestial mechanics by considering gravitation and its effect on the orbits of planets with reference to Kepler’s laws of planetary motion. This followed stimulation by a brief exchange of letters in 1679–80 with Hooke, who had been appointed to manage the Royal Society’s correspondence, and who opened a correspondence intended to elicit contributions from Newton to Royal Society transactions.[61] Newton’s reawakening interest in astronomical matters received further stimulus by the appearance of a comet in the winter of 1680–1681, on which he corresponded with John Flamsteed.[69] After the exchanges with Hooke, Newton worked out a proof that the elliptical form of planetary orbits would result from a centripetal force inversely proportional to the square of the radius vector. Newton communicated his results to Edmond Halley and to the Royal Society in De motu corporum in gyrum, a tract written on about nine sheets which was copied into the Royal Society’s Register Book in December 1684.[70] This tract contained the nucleus that Newton developed and expanded to form the Principia.
The Principia was published on 5 July 1687 with encouragement and financial help from Halley. In this work, Newton stated the three universal laws of motion. Together, these laws describe the relationship between any object, the forces acting upon it and the resulting motion, laying the foundation for classical mechanics. They contributed to many advances during the Industrial Revolution which soon followed and were not improved upon for more than 200 years. Many of these advances continue to be the underpinnings of non-relativistic technologies in the modern world. He used the Latin word gravitas (weight) for the effect that would become known as gravity, and defined the law of universal gravitation.[71]
In the same work, Newton presented a calculus-like method of geometrical analysis using ‘first and last ratios’, gave the first analytical determination (based on Boyle’s law) of the speed of sound in air, inferred the oblateness of Earth’s spheroidal figure, accounted for the precession of the equinoxes as a result of the Moon’s gravitational attraction on the Earth’s oblateness, initiated the gravitational study of the irregularities in the motion of the Moon, provided a theory for the determination of the orbits of comets, and much more.[71] Newton’s biographer David Brewster reported that the complexity of applying his theory of gravity to the motion of the moon was so great it affected Newton’s health: «[H]e was deprived of his appetite and sleep» during his work on the problem in 1692-3, and told the astronomer John Machin that «his head never ached but when he was studying the subject». According to Brewster Edmund Halley also told John Conduitt that when pressed to complete his analysis Newton «always replied that it made his head ache, and kept him awake so often, that he would think of it no more«. [Emphasis in original][72]
Newton made clear his heliocentric view of the Solar System—developed in a somewhat modern way because already in the mid-1680s he recognised the «deviation of the Sun» from the centre of gravity of the Solar System.[73] For Newton, it was not precisely the centre of the Sun or any other body that could be considered at rest, but rather «the common centre of gravity of the Earth, the Sun and all the Planets is to be esteem’d the Centre of the World», and this centre of gravity «either is at rest or moves uniformly forward in a right line» (Newton adopted the «at rest» alternative in view of common consent that the centre, wherever it was, was at rest).[74]
Newton’s postulate of an invisible force able to act over vast distances led to him being criticised for introducing «occult agencies» into science.[75] Later, in the second edition of the Principia (1713), Newton firmly rejected such criticisms in a concluding General Scholium, writing that it was enough that the phenomena implied a gravitational attraction, as they did; but they did not so far indicate its cause, and it was both unnecessary and improper to frame hypotheses of things that were not implied by the phenomena. (Here Newton used what became his famous expression «hypotheses non-fingo»[76]).
With the Principia, Newton became internationally recognised.[77] He acquired a circle of admirers, including the Swiss-born mathematician Nicolas Fatio de Duillier.[78]
In 1710, Newton found 72 of the 78 «species» of cubic curves and categorised them into four types.[79] In 1717, and probably with Newton’s help, James Stirling proved that every cubic was one of these four types. Newton also claimed that the four types could be obtained by plane projection from one of them, and this was proved in 1731, four years after his death.[80]
Later life
Royal Mint
In the 1690s, Newton wrote a number of religious tracts dealing with the literal and symbolic interpretation of the Bible. A manuscript Newton sent to John Locke in which he disputed the fidelity of 1 John 5:7—the Johannine Comma—and its fidelity to the original manuscripts of the New Testament, remained unpublished until 1785.[81]
Newton was also a member of the Parliament of England for Cambridge University in 1689 and 1701, but according to some accounts his only comments were to complain about a cold draught in the chamber and request that the window be closed.[82] He was, however, noted by Cambridge diarist Abraham de la Pryme to have rebuked students who were frightening locals by claiming that a house was haunted.[83]
Newton moved to London to take up the post of warden of the Royal Mint in 1696, a position that he had obtained through the patronage of Charles Montagu, 1st Earl of Halifax, then Chancellor of the Exchequer. He took charge of England’s great recoining, trod on the toes of Lord Lucas, Governor of the Tower, and secured the job of deputy comptroller of the temporary Chester branch for Edmond Halley. Newton became perhaps the best-known Master of the Mint upon the death of Thomas Neale in 1699, a position Newton held for the last 30 years of his life.[84][85] These appointments were intended as sinecures, but Newton took them seriously. He retired from his Cambridge duties in 1701, and exercised his authority to reform the currency and punish clippers and counterfeiters.
As Warden, and afterwards as Master, of the Royal Mint, Newton estimated that 20 percent of the coins taken in during the Great Recoinage of 1696 were counterfeit. Counterfeiting was high treason, punishable by the felon being hanged, drawn and quartered. Despite this, convicting even the most flagrant criminals could be extremely difficult, but Newton proved equal to the task.[86]
Disguised as a habitué of bars and taverns, he gathered much of that evidence himself.[87] For all the barriers placed to prosecution, and separating the branches of government, English law still had ancient and formidable customs of authority. Newton had himself made a justice of the peace in all the home counties. A draft letter regarding the matter is included in Newton’s personal first edition of Philosophiæ Naturalis Principia Mathematica, which he must have been amending at the time.[88] Then he conducted more than 100 cross-examinations of witnesses, informers, and suspects between June 1698 and Christmas 1699. Newton successfully prosecuted 28 coiners.[89]
Newton was made president of the Royal Society in 1703 and an associate of the French Académie des Sciences. In his position at the Royal Society, Newton made an enemy of John Flamsteed, the Astronomer Royal, by prematurely publishing Flamsteed’s Historia Coelestis Britannica, which Newton had used in his studies.[91]
Knighthood
In April 1705, Queen Anne knighted Newton during a royal visit to Trinity College, Cambridge. The knighthood is likely to have been motivated by political considerations connected with the parliamentary election in May 1705, rather than any recognition of Newton’s scientific work or services as Master of the Mint.[92] Newton was the second scientist to be knighted, after Francis Bacon.[93]
As a result of a report written by Newton on 21 September 1717 to the Lords Commissioners of His Majesty’s Treasury, the bimetallic relationship between gold coins and silver coins was changed by royal proclamation on 22 December 1717, forbidding the exchange of gold guineas for more than 21 silver shillings.[94] This inadvertently resulted in a silver shortage as silver coins were used to pay for imports, while exports were paid for in gold, effectively moving Britain from the silver standard to its first gold standard. It is a matter of debate as to whether he intended to do this or not.[95] It has been argued that Newton conceived of his work at the Mint as a continuation of his alchemical work.[96]
Newton was invested in the South Sea Company and lost some £20,000 (£4.4 million in 2020[97]) when it collapsed in around 1720.[98]
Toward the end of his life, Newton took up residence at Cranbury Park, near Winchester, with his niece and her husband, until his death.[99] His half-niece, Catherine Barton,[100] served as his hostess in social affairs at his house on Jermyn Street in London; he was her «very loving Uncle»,[101] according to his letter to her when she was recovering from smallpox.
Death
Newton died in his sleep in London on 20 March 1727 (OS 20 March 1726; NS 31 March 1727).[a] He was given a ceremonial funeral, attended by nobles, scientists, and philosophers, and was buried in Westminster Abbey among kings and queens. He is also the first scientist to be buried in the abbey.[102] Voltaire may have been present at his funeral.[103] A bachelor, he had divested much of his estate to relatives during his last years, and died intestate.[104] His papers went to John Conduitt and Catherine Barton.[105]
After his death, Newton’s hair was examined and found to contain mercury, probably resulting from his alchemical pursuits. Mercury poisoning could explain Newton’s eccentricity in late life.[104]
Personality
Although it was claimed that he was once engaged,[b] Newton never married. The French writer and philosopher Voltaire, who was in London at the time of Newton’s funeral, said that he «was never sensible to any passion, was not subject to the common frailties of mankind, nor had any commerce with women—a circumstance which was assured me by the physician and surgeon who attended him in his last moments».[107] There exists a widespread belief that Newton died a virgin, and writers as diverse as mathematician Charles Hutton,[108] economist John Maynard Keynes,[109] and physicist Carl Sagan each have commented on it.[110]
Newton had a close friendship with the Swiss mathematician Nicolas Fatio de Duillier, who he met in London around 1689[78]—some of their correspondence has survived.[111][112] Their relationship came to an abrupt and unexplained end in 1693, and at the same time Newton suffered a nervous breakdown,[113] which included sending wild accusatory letters to his friends Samuel Pepys and John Locke. His note to the latter included the charge that Locke «endeavoured to embroil me with woemen».[114]
Newton was relatively modest about his achievements, writing in a letter to Robert Hooke in February 1676, «If I have seen further it is by standing on the shoulders of giants.»[115] Two writers think that the sentence, written at a time when Newton and Hooke were in dispute over optical discoveries, was an oblique attack on Hooke (said to have been short and hunchbacked), rather than—or in addition to—a statement of modesty.[116][117] On the other hand, the widely known proverb about standing on the shoulders of giants, published among others by seventeenth-century poet George Herbert (a former orator of the University of Cambridge and fellow of Trinity College) in his Jacula Prudentum (1651), had as its main point that «a dwarf on a giant’s shoulders sees farther of the two», and so its effect as an analogy would place Newton himself rather than Hooke as the ‘dwarf’.
In a later memoir, Newton wrote, «I do not know what I may appear to the world, but to myself I seem to have been only like a boy playing on the sea-shore, and diverting myself in now and then finding a smoother pebble or a prettier shell than ordinary, whilst the great ocean of truth lay all undiscovered before me.»[118]
In 2015, Steven Weinberg, a Nobel laureate in physics, called Newton «a nasty antagonist» and «a bad man to have as an enemy»,[119] noting Newton’s attitude towards Robert Hooke and Gottfried Wilhelm Leibniz.
It has been suggested by some scientists and clinicians that, based on these and other traits along with his profound power of concentration, that Newton may have had an undiagnosed form of high-functioning autism, now properly known as ASD1 within autism spectrum; formerly known as Asperger syndrome.[120][121][122]
Theology
Religious views
Although born into an Anglican family, by his thirties Newton held a Christian faith that, had it been made public, would not have been considered orthodox by mainstream Christianity,[123] with one historian labelling him a heretic.[124]
By 1672, he had started to record his theological researches in notebooks which he showed to no one and which have only recently[when?] been examined. They demonstrate an extensive knowledge of early Church writings and show that in the conflict between Athanasius and Arius which defined the Creed, he took the side of Arius, the loser, who rejected the conventional view of the Trinity. Newton «recognized Christ as a divine mediator between God and man, who was subordinate to the Father who created him.»[125] He was especially interested in prophecy, but for him, «the great apostasy was trinitarianism.»[126]
Newton tried unsuccessfully to obtain one of the two fellowships that exempted the holder from the ordination requirement. At the last moment in 1675 he received a dispensation from the government that excused him and all future holders of the Lucasian chair.[127]
In Newton’s eyes, worshipping Christ as God was idolatry, to him the fundamental sin.[128] In 1999, historian Stephen D. Snobelen wrote, «Isaac Newton was a heretic. But … he never made a public declaration of his private faith—which the orthodox would have deemed extremely radical. He hid his faith so well that scholars are still unraveling his personal beliefs.»[124] Snobelen concludes that Newton was at least a Socinian sympathiser (he owned and had thoroughly read at least eight Socinian books), possibly an Arian and almost certainly an anti-trinitarian.[124]
The view that Newton was Semi-Arian has lost support now that scholars have investigated Newton’s theological papers, and now most scholars identify Newton as an Antitrinitarian monotheist.[124][129]
Although the laws of motion and universal gravitation became Newton’s best-known discoveries, he warned against using them to view the Universe as a mere machine, as if akin to a great clock. He said, «So then gravity may put the planets into motion, but without the Divine Power it could never put them into such a circulating motion, as they have about the sun».[131]
Along with his scientific fame, Newton’s studies of the Bible and of the early Church Fathers were also noteworthy. Newton wrote works on textual criticism, most notably An Historical Account of Two Notable Corruptions of Scripture and Observations upon the Prophecies of Daniel, and the Apocalypse of St. John.[132] He placed the crucifixion of Jesus Christ at 3 April, AD 33, which agrees with one traditionally accepted date.[133]
He believed in a rationally immanent world, but he rejected the hylozoism implicit in Leibniz and Baruch Spinoza. The ordered and dynamically informed Universe could be understood, and must be understood, by an active reason. In his correspondence, Newton claimed that in writing the Principia «I had an eye upon such Principles as might work with considering men for the belief of a Deity».[134] He saw evidence of design in the system of the world: «Such a wonderful uniformity in the planetary system must be allowed the effect of choice». But Newton insisted that divine intervention would eventually be required to reform the system, due to the slow growth of instabilities.[135] For this, Leibniz lampooned him: «God Almighty wants to wind up his watch from time to time: otherwise it would cease to move. He had not, it seems, sufficient foresight to make it a perpetual motion.»[136]
Newton’s position was vigorously defended by his follower Samuel Clarke in a famous correspondence. A century later, Pierre-Simon Laplace’s work Celestial Mechanics had a natural explanation for why the planet orbits do not require periodic divine intervention.[137] The contrast between Laplace’s mechanistic worldview and Newton’s one is the most strident considering the famous answer which the French scientist gave Napoleon, who had criticised him for the absence of the Creator in the Mécanique céleste: «Sire, j’ai pu me passer de cette hypothèse» («Sir, I didn’t need this hypothesis»).[138]
Scholars long debated whether Newton disputed the doctrine of the Trinity. His first biographer, David Brewster, who compiled his manuscripts, interpreted Newton as questioning the veracity of some passages used to support the Trinity, but never denying the doctrine of the Trinity as such.[139] In the twentieth century, encrypted manuscripts written by Newton and bought by John Maynard Keynes (among others) were deciphered[64] and it became known that Newton did indeed reject Trinitarianism.[124]
Religious thought
Newton and Robert Boyle’s approach to the mechanical philosophy was promoted by rationalist pamphleteers as a viable alternative to the pantheists and enthusiasts, and was accepted hesitantly by orthodox preachers as well as dissident preachers like the latitudinarians.[140] The clarity and simplicity of science was seen as a way to combat the emotional and metaphysical superlatives of both superstitious enthusiasm and the threat of atheism,[141] and at the same time, the second wave of English deists used Newton’s discoveries to demonstrate the possibility of a «Natural Religion».
The attacks made against pre-Enlightenment «magical thinking», and the mystical elements of Christianity, were given their foundation with Boyle’s mechanical conception of the universe. Newton gave Boyle’s ideas their completion through mathematical proofs and, perhaps more importantly, was very successful in popularising them.[142]
The occult
In a manuscript he wrote in 1704 (never intended to be published), he mentions the date of 2060, but it is not given as a date for the end of days. It has been falsely reported as a prediction.[143] The passage is clear when the date is read in context. He was against date setting for the end of days, concerned that this would put Christianity into disrepute.
So then the time times & half a time [sic] are 42 months or 1260 days or three years & an half, recconing twelve months to a year & 30 days to a month as was done in the Calender [sic] of the primitive year. And the days of short lived Beasts being put for the years of [long-]lived kingdoms the period of 1260 days, if dated from the complete conquest of the three kings A.C. 800, will end 2060. It may end later, but I see no reason for its ending sooner.[144]
This I mention not to assert when the time of the end shall be, but to put a stop to the rash conjectures of fanciful men who are frequently predicting the time of the end, and by doing so bring the sacred prophesies into discredit as often as their predictions fail. Christ comes as a thief in the night, and it is not for us to know the times and seasons which God hath put into his own breast.[145][143]
Alchemy
In the character of Morton Opperly in «Poor Superman» (1951), speculative fiction author Fritz Leiber says of Newton, «Everyone knows Newton as the great scientist. Few remember that he spent half his life muddling with alchemy, looking for the philosopher’s stone. That was the pebble by the seashore he really wanted to find.»[146]
Of an estimated ten million words of writing in Newton’s papers, about one million deal with alchemy. Many of Newton’s writings on alchemy are copies of other manuscripts, with his own annotations.[105] Alchemical texts mix artisanal knowledge with philosophical speculation, often hidden behind layers of wordplay, allegory, and imagery to protect craft secrets.[147] Some of the content contained in Newton’s papers could have been considered heretical by the church.[105]
In 1888, after spending sixteen years cataloguing Newton’s papers, Cambridge University kept a small number and returned the rest to the Earl of Portsmouth. In 1936, a descendant offered the papers for sale at Sotheby’s.[148] The collection was broken up and sold for a total of about £9,000.[149] John Maynard Keynes was one of about three dozen bidders who obtained part of the collection at auction. Keynes went on to reassemble an estimated half of Newton’s collection of papers on alchemy before donating his collection to Cambridge University in 1946.[105][148][150]
All of Newton’s known writings on alchemy are currently being put online in a project undertaken by Indiana University: «The Chymistry of Isaac Newton»[151] and summarised in a book.[152][153]
Newton’s fundamental contributions to science include the quantification of gravitational attraction, the discovery that white light is actually a mixture of immutable spectral colors, and the formulation of the calculus. Yet there is another, more mysterious side to Newton that is imperfectly known, a realm of activity that spanned some thirty years of his life, although he kept it largely hidden from his contemporaries and colleagues. We refer to Newton’s involvement in the discipline of alchemy, or as it was often called in seventeenth-century England, «chymistry.»[151]
Charles Coulston Gillispie disputes that Newton ever practised alchemy, saying that «his chemistry was in the spirit of Boyle’s corpuscular philosophy.»[154]
In June 2020, two unpublished pages of Newton’s notes on Jan Baptist van Helmont’s book on plague, De Peste,[155] were being auctioned online by Bonhams. Newton’s analysis of this book, which he made in Cambridge while protecting himself from London’s 1665–1666 infection, is the most substantial written statement he is known to have made about the plague, according to Bonhams. As far as the therapy is concerned, Newton writes that «the best is a toad suspended by the legs in a chimney for three days, which at last vomited up earth with various insects in it, on to a dish of yellow wax, and shortly after died. Combining powdered toad with the excretions and serum made into lozenges and worn about the affected area drove away the contagion and drew out the poison».[156]
Legacy
Fame
The mathematician Joseph-Louis Lagrange said that Newton was the greatest genius who ever lived, and once added that Newton was also «the most fortunate, for we cannot find more than once a system of the world to establish.»[157] English poet Alexander Pope wrote the famous epitaph:
Nature, and Nature’s laws lay hid in night.
God said, Let Newton be! and all was light.
But this was not allowed to be inscribed in the monument. The epitaph in the monument is as follows:[158]
H. S. E. ISAACUS NEWTON Eques Auratus, / Qui, animi vi prope divinâ, / Planetarum Motus, Figuras, / Cometarum semitas, Oceanique Aestus. Suâ Mathesi facem praeferente / Primus demonstravit: / Radiorum Lucis dissimilitudines, / Colorumque inde nascentium proprietates, / Quas nemo antea vel suspicatus erat, pervestigavit. / Naturae, Antiquitatis, S. Scripturae, / Sedulus, sagax, fidus Interpres / Dei O. M. Majestatem Philosophiâ asseruit, / Evangelij Simplicitatem Moribus expressit. / Sibi gratulentur Mortales, / Tale tantumque exstitisse / HUMANI GENERIS DECUS. / NAT. XXV DEC. A.D. MDCXLII. OBIIT. XX. MAR. MDCCXXVI,
which can be translated as follows:[158]
Here is buried Isaac Newton, Knight, who by a strength of mind almost divine, and mathematical principles peculiarly his own, explored the course and figures of the planets, the paths of comets, the tides of the sea, the dissimilarities in rays of light, and, what no other scholar has previously imagined, the properties of the colours thus produced. Diligent, sagacious and faithful, in his expositions of nature, antiquity and the holy Scriptures, he vindicated by his philosophy the majesty of God mighty and good, and expressed the simplicity of the Gospel in his manners. Mortals rejoice that there has existed such and so great an ornament of the human race! He was born on 25th December 1642, and died on 20th March 1726.
In a 2005 survey of members of Britain’s Royal Society (formerly headed by Newton) asking who had the greater effect on the history of science, Newton or Albert Einstein, the members deemed Newton to have made the greater overall contribution.[159] In 1999, an opinion poll of 100 of the day’s leading physicists voted Einstein the «greatest physicist ever,» with Newton the runner-up, while a parallel survey of rank-and-file physicists by the site PhysicsWeb gave the top spot to Newton.[160] Einstein kept a picture of Newton on his study wall alongside ones of Michael Faraday and James Clerk Maxwell.[161]
The SI derived unit of force is named the newton in his honour.
Woolsthorpe Manor is a Grade I listed building by Historic England through being his birthplace and «where he discovered gravity and developed his theories regarding the refraction of light».[162]
In 1816, a tooth said to have belonged to Newton was sold for £730[163] (US$3,633) in London to an aristocrat who had it set in a ring.[164] Guinness World Records 2002 classified it as the most valuable tooth, which would value approximately £25,000 (US$35,700) in late 2001.[164] Who bought it and who currently has it has not been disclosed.
Apple incident
Newton himself often told the story that he was inspired to formulate his theory of gravitation by watching the fall of an apple from a tree.[165][166] The story is believed to have passed into popular knowledge after being related by Catherine Barton, Newton’s niece, to Voltaire.[167] Voltaire then wrote in his Essay on Epic Poetry (1727), «Sir Isaac Newton walking in his gardens, had the first thought of his system of gravitation, upon seeing an apple falling from a tree.»[168][169]
Although it has been said that the apple story is a myth and that he did not arrive at his theory of gravity at any single moment,[170] acquaintances of Newton (such as William Stukeley, whose manuscript account of 1752 has been made available by the Royal Society) do in fact confirm the incident, though not the apocryphal version that the apple actually hit Newton’s head. Stukeley recorded in his Memoirs of Sir Isaac Newton’s Life a conversation with Newton in Kensington on 15 April 1726:[171][172][173]
we went into the garden, & drank thea under the shade of some appletrees, only he, & myself. amidst other discourse, he told me, he was just in the same situation, as when formerly, the notion of gravitation came into his mind. «why should that apple always descend perpendicularly to the ground,» thought he to him self: occasion’d by the fall of an apple, as he sat in a comtemplative mood: «why should it not go sideways, or upwards? but constantly to the earths centre? assuredly, the reason is, that the earth draws it. there must be a drawing power in matter. & the sum of the drawing power in the matter of the earth must be in the earths center, not in any side of the earth. therefore dos this apple fall perpendicularly, or toward the center. if matter thus draws matter; it must be in proportion of its quantity. therefore the apple draws the earth, as well as the earth draws the apple.»
John Conduitt, Newton’s assistant at the Royal Mint and husband of Newton’s niece, also described the event when he wrote about Newton’s life:[174]
In the year 1666 he retired again from Cambridge to his mother in Lincolnshire. Whilst he was pensively meandering in a garden it came into his thought that the power of gravity (which brought an apple from a tree to the ground) was not limited to a certain distance from earth, but that this power must extend much further than was usually thought. Why not as high as the Moon said he to himself & if so, that must influence her motion & perhaps retain her in her orbit, whereupon he fell a calculating what would be the effect of that supposition.
It is known from his notebooks that Newton was grappling in the late 1660s with the idea that terrestrial gravity extends, in an inverse-square proportion, to the Moon; however, it took him two decades to develop the full-fledged theory.[175] The question was not whether gravity existed, but whether it extended so far from Earth that it could also be the force holding the Moon to its orbit. Newton showed that if the force decreased as the inverse square of the distance, one could indeed calculate the Moon’s orbital period, and get good agreement. He guessed the same force was responsible for other orbital motions, and hence named it «universal gravitation».
Various trees are claimed to be «the» apple tree which Newton describes. The King’s School, Grantham claims that the tree was purchased by the school, uprooted and transported to the headmaster’s garden some years later. The staff of the (now) National Trust-owned Woolsthorpe Manor dispute this, and claim that a tree present in their gardens is the one described by Newton. A descendant of the original tree[176] can be seen growing outside the main gate of Trinity College, Cambridge, below the room Newton lived in when he studied there. The National Fruit Collection at Brogdale in Kent[177] can supply grafts from their tree, which appears identical to Flower of Kent, a coarse-fleshed cooking variety.[178]
Commemorations
Newton’s monument (1731) can be seen in Westminster Abbey, at the north of the entrance to the choir against the choir screen, near his tomb. It was executed by the sculptor Michael Rysbrack (1694–1770) in white and grey marble with design by the architect William Kent.[179] The monument features a figure of Newton reclining on top of a sarcophagus, his right elbow resting on several of his great books and his left hand pointing to a scroll with a mathematical design. Above him is a pyramid and a celestial globe showing the signs of the Zodiac and the path of the comet of 1680. A relief panel depicts putti using instruments such as a telescope and prism.[180] The Latin inscription on the base translates as:
Here is buried Isaac Newton, Knight, who by a strength of mind almost divine, and mathematical principles peculiarly his own, explored the course and figures of the planets, the paths of comets, the tides of the sea, the dissimilarities in rays of light, and, what no other scholar has previously imagined, the properties of the colours thus produced. Diligent, sagacious and faithful, in his expositions of nature, antiquity and the holy Scriptures, he vindicated by his philosophy the majesty of God mighty and good, and expressed the simplicity of the Gospel in his manners. Mortals rejoice that there has existed such and so great an ornament of the human race! He was born on 25 December 1642, and died on 20 March 1726/7.
- —Translation from G. L. Smyth, The Monuments and Genii of St. Paul’s Cathedral, and of Westminster Abbey (1826), ii, 703–704.[180]
From 1978 until 1988, an image of Newton designed by Harry Ecclestone appeared on Series D £1 banknotes issued by the Bank of England (the last £1 notes to be issued by the Bank of England). Newton was shown on the reverse of the notes holding a book and accompanied by a telescope, a prism and a map of the Solar System.[181]
A statue of Isaac Newton, looking at an apple at his feet, can be seen at the Oxford University Museum of Natural History. A large bronze statue, Newton, after William Blake, by Eduardo Paolozzi, dated 1995 and inspired by Blake’s etching, dominates the piazza of the British Library in London. A bronze statue of Newton was erected in 1858 in the centre of Grantham where he went to school, prominently standing in front of Grantham Guildhall.
The still-surviving farmhouse at Woolsthorpe By Colsterworth is a Grade I listed building by Historic England through being his birthplace and «where he discovered gravity and developed his theories regarding the refraction of light».[162]
The Enlightenment
Enlightenment philosophers chose a short history of scientific predecessors—Galileo, Boyle, and Newton principally—as the guides and guarantors of their applications of the singular concept of nature and natural law to every physical and social field of the day. In this respect, the lessons of history and the social structures built upon it could be discarded.[182]
It is held by European philosophers of the Enlightenment and by historians of the Enlightenment that Newton’s publication of the Principia was a turning point in the Scientific Revolution and started the Enlightenment. It was Newton’s conception of the universe based upon natural and rationally understandable laws that became one of the seeds for Enlightenment ideology.[183] Locke and Voltaire applied concepts of natural law to political systems advocating intrinsic rights; the physiocrats and Adam Smith applied natural conceptions of psychology and self-interest to economic systems; and sociologists criticised the current social order for trying to fit history into natural models of progress. Monboddo and Samuel Clarke resisted elements of Newton’s work, but eventually rationalised it to conform with their strong religious views of nature.
Works
Published in his lifetime
- De analysi per aequationes numero terminorum infinitas (1669, published 1711)[184]
- Of Natures Obvious Laws & Processes in Vegetation (unpublished, c. 1671–75)[185]
- De motu corporum in gyrum (1684)[186]
- Philosophiæ Naturalis Principia Mathematica (1687)[187]
- Scala graduum Caloris. Calorum Descriptiones & signa (1701)[188]
- Opticks (1704)[189]
- Reports as Master of the Mint (1701–1725)[190]
- Arithmetica Universalis (1707)[190]
Published posthumously
- De mundi systemate (The System of the World) (1728)[190]
- Optical Lectures (1728)[190]
- The Chronology of Ancient Kingdoms Amended (1728)[190]
- Observations on Daniel and The Apocalypse of St. John (1733)[190]
- Method of Fluxions (1671, published 1736)[191]
- An Historical Account of Two Notable Corruptions of Scripture (1754)[190]
See also
- Elements of the Philosophy of Newton, a book by Voltaire
- List of multiple discoveries: seventeenth century
- List of things named after Isaac Newton
References
Notes
- ^ a b c d e During Newton’s lifetime, two calendars were in use in Europe: the Julian («Old Style») calendar in Protestant and Orthodox regions, including Britain; and the Gregorian («New Style») calendar in Roman Catholic Europe. At Newton’s birth, Gregorian dates were ten days ahead of Julian dates; thus, his birth is recorded as taking place on 25 December 1642 Old Style, but it can be converted to a New Style (modern) date of 4 January 1643. By the time of his death, the difference between the calendars had increased to eleven days. Moreover, he died in the period after the start of the New Style year on 1 January but before that of the Old Style new year on 25 March. His death occurred on 20 March 1726, according to the Old Style calendar, but the year is usually adjusted to 1727. A full conversion to New Style gives the date 31 March 1727.[1]
- ^ This claim was made by William Stukeley in 1727, in a letter about Newton written to Richard Mead. Charles Hutton, who in the late eighteenth century collected oral traditions about earlier scientists, declared that there «do not appear to be any sufficient reason for his never marrying, if he had an inclination so to do. It is much more likely that he had a constitutional indifference to the state, and even to the sex in general.»[106]
Citations
- ^ Thony, Christie (2015). «Calendrical confusion or just when did Newton die?». The Renaissance Mathematicus. Archived from the original on 2 April 2015. Retrieved 20 March 2015.
- ^ Kevin C. Knox, Richard Noakes (eds.), From Newton to Hawking: A History of Cambridge University’s Lucasian Professors of Mathematics, Cambridge University Press, 2003, p. 61.
- ^ a b «Fellows of the Royal Society». London: Royal Society. Archived from the original on 16 March 2015.
- ^ Feingold, Mordechai. Barrow, Isaac (1630–1677) Archived 29 January 2013 at the Wayback Machine, Oxford Dictionary of National Biography, Oxford University Press, September 2004; online edn, May 2007. Retrieved 24 February 2009; explained further in Feingold, Mordechai (1993). «Newton, Leibniz, and Barrow Too: An Attempt at a Reinterpretation». Isis. 84 (2): 310–338. Bibcode:1993Isis…84..310F. doi:10.1086/356464. JSTOR 236236. S2CID 144019197.
- ^ «Dictionary of Scientific Biography». Notes, No. 4. Archived from the original on 25 February 2005.
- ^ Gjertsen 1986, p. [page needed]
- ^ Newton, Isaac (February 1678). Philosophical tract from Mr Isaac Newton. Cambridge University. Archived from the original on 8 October 2016. Retrieved 1 October 2021.
But because I am indebted to you & yesterday met with a friend Mr Maulyverer, who told me he was going to London & intended to give you the trouble of a visit, I could not forbear to take the opportunity of conveying this to you by him.
- ^ I. Bernard Cohen; George E. Smith (25 April 2002). The Cambridge Companion to Newton. Cambridge University Press. p. 69. ISBN 978-0-521-65696-2. Archived from the original on 16 September 2020. Retrieved 15 May 2013.
- ^ Niccolò Guicciardini (2009). Isaac Newton on mathematical certainty and method. MIT Press. p. 344. ISBN 978-0-262-01317-8. Archived from the original on 16 September 2020. Retrieved 15 May 2013.
- ^ a b Ducheyne, Steffen (2009). «The Flow of Influence from Newton to Locke… and Back». Rivista di Storia della Filosofia (1984–). 64 (2): 245–268. doi:10.3280/SF2009-002001. ISSN 0393-2516. JSTOR 44024132. Archived from the original on 23 May 2022. Retrieved 23 May 2022.
- ^ a b Rogers, G. A. J. (1978). «Locke’s Essay and Newton’s Principia». Journal of the History of Ideas. 39 (2): 217–232. doi:10.2307/2708776. ISSN 0022-5037. JSTOR 2708776. Archived from the original on 23 May 2022. Retrieved 23 May 2022.
- ^ «Isaac Newton: «Judaic monotheist of the school of Maimonides»«. Achgut.com. 19 June 2007. Archived from the original on 28 April 2015. Retrieved 13 March 2010.
- ^ See e.g. D. T. Whiteside, Before the Principia, in Journal for the History of Astronomy 1 (1970), 5–17, p. 7.
- ^ Jeremy Jennings. Revolution and the Republic: A History of Political Thought in France Since the Eighteenth Century. Oxford University Press, 2011. p. 347.
- ^ Bruni, Luigino; Porta, Pier Luigi (2003). «Economia Civile and Pubblica Felicità in the Italian Enlightenment». History of Political Economy. 35 (Suppl. 1): 361–385 (365). doi:10.1215/00182702-35-Suppl_1-361. S2CID 143538016.
- ^ Pearson, Roger (2005). Voltaire almighty : a life in pursuit of freedom. Internet Archive. New York : Bloomsbury : Distributed to the trade by Holtzbrinck. p. 138. ISBN 978-1-58234-630-4.
- ^ «Isaac Newton, horoscope for birth date 25 December 1642 Jul.Cal». Astro-Databank Wiki. Archived from the original on 5 January 2017. Retrieved 4 January 2017.
- ^ Storr, Anthony (December 1985). «Isaac Newton». British Medical Journal (Clinical Research Edition). 291 (6511): 1779–1784. doi:10.1136/bmj.291.6511.1779. JSTOR 29521701. PMC 1419183. PMID 3936583.
- ^ Keynes, Milo (20 September 2008). «Balancing Newton’s Mind: His Singular Behaviour and His Madness of 1692–93». Notes and Records of the Royal Society of London. 62 (3): 289–300. doi:10.1098/rsnr.2007.0025. JSTOR 20462679. PMID 19244857.
- ^ Westfall 1980, p. 55.
- ^ «Newton the Mathematician» Z. Bechler, ed., Contemporary Newtonian Research(Dordrecht 1982) pp. 110–111
- ^ Westfall 1994, pp. 16–19.
- ^ White 1997, p. 22.
- ^ Westfall 1980, pp. 60–62.
- ^ Westfall 1980, pp. 71, 103.
- ^ Hoskins, Michael, ed. (1997). Cambridge Illustrated History of Astronomy. Cambridge University Press. p. 159. ISBN 978-0-521-41158-5.
- ^ Newton, Isaac. «Waste Book». Cambridge University Digital Library. Archived from the original on 8 January 2012. Retrieved 10 January 2012.
- ^ «Newton, Isaac (NWTN661I)». A Cambridge Alumni Database. University of Cambridge.
- ^ Westfall 1980, p. 178.
- ^ Westfall 1980, pp. 330–331.
- ^ Ball 1908, p. 319.
- ^ Whiteside, D.T., ed. (1967). «Part 7: The October 1666 Tract on Fluxions». The Mathematical Papers of Isaac Newton. 1. Cambridge University Press. p. 400. Archived 12 May 2016 at the Wayback Machine.
- ^ Gjertsen 1986, p. 149.
- ^ Newton, Principia, 1729 English translation, p. 41 Archived 3 October 2015 at the Wayback Machine.
- ^ Newton, Principia, 1729 English translation, p. 54 Archived 3 May 2016 at the Wayback Machine.
- ^ Newton, Sir Isaac (1850). Newton’s Principia: The Mathematical Principles of Natural Philosophy. Geo. P. Putnam. Archived from the original on 26 June 2019. Retrieved 9 March 2019.
- ^ Clifford Truesdell, Essays in the History of Mechanics (1968), p. 99.
- ^ In the preface to the Marquis de L’Hospital’s Analyse des Infiniment Petits (Paris, 1696).
- ^ Starting with De motu corporum in gyrum, see also (Latin) Theorem 1 Archived 12 May 2016 at the Wayback Machine.
- ^ Whiteside, D.T., ed. (1970). «The Mathematical principles underlying Newton’s Principia Mathematica». Journal for the History of Astronomy. 1. Cambridge University Press. pp. 116–138.
- ^ Stewart 2009, p. 107.
- ^ Westfall 1980, pp. 538–539.
- ^ Stern, Keith (2009). Queers in history : the comprehensive encyclopedia of historical gays, lesbians, bisexuals, and transgenders. Dallas, Tex.: BenBella. ISBN 978-1-933771-87-8. OCLC 317453194.
- ^ Nowlan, Robert (2017). Masters of Mathematics: The Problems They Solved, Why These Are Important, and What You Should Know about Them. Rotterdam: Sense Publishers. p. 136. ISBN 978-94-6300-891-4.
- ^ Ball 1908, p. 356.
- ^ Błaszczyk, P.; et al. (March 2013). «Ten misconceptions from the history of analysis and their debunking». Foundations of Science. 18 (1): 43–74. arXiv:1202.4153. doi:10.1007/s10699-012-9285-8. S2CID 119134151.
- ^ Westfall 1980, p. 179.
- ^ White 1997, p. 151.
- ^ King, Henry C (2003). The History of the Telescope. p. 74. ISBN 978-0-486-43265-6.
- ^ Whittaker, E.T., A History of the Theories of Aether and Electricity, Dublin University Press, 1910.
- ^ Olivier Darrigol (2012). A History of Optics from Greek Antiquity to the Nineteenth Century. Oxford University Press. p. 81. ISBN 978-0-19-964437-7.
- ^ Newton, Isaac. «Hydrostatics, Optics, Sound and Heat». Cambridge University Digital Library. Archived from the original on 8 January 2012. Retrieved 10 January 2012.
- ^ Ball 1908, p. 324.
- ^ William R. Newman, «Newton’s Early Optical Theory and its Debt to Chymistry», in Danielle Jacquart and Michel Hochmann, eds., Lumière et vision dans les sciences et dans les arts (Geneva: Droz, 2010), pp. 283–307. A free access online version of this article can be found at the Chymistry of Isaac Newton project Archived 28 May 2016 at the Wayback Machine (PDF)
- ^ Ball 1908, p. 325.
- ^ «The Early Period (1608–1672)». James R. Graham’s Home Page. Retrieved 3 February 2009.[permanent dead link]
- ^ a b White 1997, p. 170
- ^ Hall, Alfred Rupert (1996). Isaac Newton: adventurer in thought. Cambridge University Press. p. 67. ISBN 978-0-521-56669-8. OCLC 606137087.
This is the one dated 23 February 1669, in which Newton described his first reflecting telescope, constructed (it seems) near the close of the previous year.
- ^ White 1997, p. 168.
- ^ Newton, Isaac. «Of Colours». The Newton Project. Archived from the original on 9 October 2014. Retrieved 6 October 2014.
- ^ a b See ‘Correspondence of Isaac Newton, vol. 2, 1676–1687’ ed. H.W. Turnbull, Cambridge University Press 1960; at p. 297, document No. 235, letter from Hooke to Newton dated 24 November 1679.
- ^ Iliffe, Robert (2007) Newton. A very short introduction, Oxford University Press 2007
- ^ a b Westfall, Richard S. (1983) [1980]. Never at Rest: A Biography of Isaac Newton. Cambridge: Cambridge University Press. pp. 530–531. ISBN 978-0-521-27435-7.
- ^ a b Keynes, John Maynard (1972). «Newton, The Man». The Collected Writings of John Maynard Keynes Volume X. MacMillan St. Martin’s Press. pp. 363–366.
- ^ Dobbs, J.T. (December 1982). «Newton’s Alchemy and His Theory of Matter». Isis. 73 (4): 523. doi:10.1086/353114. S2CID 170669199. quoting Opticks
- ^ Opticks, 2nd Ed 1706. Query 8.
- ^ a b Duarte, F.J. (2000). «Newton, prisms, and the ‘opticks’ of tunable lasers» (PDF). Optics and Photonics News. 11 (5): 24–25. Bibcode:2000OptPN..11…24D. doi:10.1364/OPN.11.5.000024. Archived (PDF) from the original on 17 February 2015. Retrieved 17 February 2015.
- ^ Tyndall, John. (1880). Popular Science Monthly Volume 17, July. s:Popular Science Monthly/Volume 17/July 1880/Goethe’s Farbenlehre: Theory of Colors II
- ^ Westfall 1980, pp. 391–392.
- ^ Whiteside, D.T., ed. (1974). Mathematical Papers of Isaac Newton, 1684–1691. 6. Cambridge University Press. p. 30.
- ^ a b Schmitz, Kenneth S. (2018). Physical Chemistry: Multidisciplinary Applications in Society. Amsterdam: Elsevier. p. 251. ISBN 978-0-12-800599-6. Archived from the original on 10 March 2020. Retrieved 1 March 2020.
- ^ Brewster, Sir David Memoirs of the Life, Writings, and Discoveries of Sir Isaac Newton, Volume 2. Edinburgh: 1860 p108
- ^ See Curtis Wilson, «The Newtonian achievement in astronomy», pp. 233–274 in R Taton & C Wilson (eds) (1989) The General History of Astronomy, Volume, 2A’, at p. 233 Archived 3 October 2015 at the Wayback Machine.
- ^ Text quotations are from 1729 translation of Newton’s Principia, Book 3 (1729 vol.2) at pp. 232–233.
- ^ Edelglass et al., Matter and Mind, ISBN 0-940262-45-2. p. 54
- ^ On the meaning and origins of this expression, see Kirsten Walsh, Does Newton feign an hypothesis? Archived 14 July 2014 at the Wayback Machine, Early Modern Experimental Philosophy Archived 21 July 2011 at the Wayback Machine, 18 October 2010.
- ^ Westfall 1980, Chapter 11.
- ^ a b Professor Robert A. Hatch, University of Florida. «Newton Timeline». Archived from the original on 2 August 2012. Retrieved 13 August 2012.
- ^ Weisstein, Eric W. «Cubic Curve». mathworld.wolfram.com. Archived from the original on 27 January 2021. Retrieved 20 January 2021.
- ^ Conics and Cubics, Robert Bix, Springer Undergraduate Texts in Mathematics, 2nd edition, 2006, Springer Verlag.
- ^ «John Locke Manuscripts – Chronological Listing: 1690». psu.edu. Archived from the original on 9 July 2017. Retrieved 20 January 2013.; and John C. Attig, John Locke Bibliography — Chapter 5, Religion, 1751–1900 Archived 12 November 2012 at the Wayback Machine
- ^ White 1997, p. 232.
- ^ Patrick Sawer (6 September 2016). «What students should avoid during fresher’s week (100 years ago and now)». The Daily Telegraph. Archived from the original on 10 January 2022. Retrieved 7 September 2016.
- ^ «Isaac Newton: Physicist And … Crime Fighter?». Science Friday. 5 June 2009. NPR. Archived from the original on 1 November 2014. Transcript. Retrieved 1 August 2014.
- ^ Levenson, Thomas (2009). Newton and the counterfeiter: the unknown detective career of the world’s greatest scientist. Houghton Mifflin Harcourt. ISBN 978-0-15-101278-7. OCLC 276340857.
- ^ White 1997, p. 259.
- ^ White 1997, p. 267.
- ^ Newton, Isaac. «Philosophiæ Naturalis Principia Mathematica». Cambridge University Digital Library. pp. 265–266. Archived from the original on 8 January 2012. Retrieved 10 January 2012.
- ^ Westfall 2007, p. 73.
- ^ Wagner, Anthony (1972). Historic Heraldry of Britain (2nd ed.). London and Chichester: Phillimore. p. 85. ISBN 978-0-85033-022-9.; and Genealogical Memoranda Relating to the Family of Newton. London: Taylor and Co. 1871.
- ^ White 1997, p. 317.
- ^ «The Queen’s ‘great Assistance’ to Newton’s election was his knighting, an honor bestowed not for his contributions to science, nor for his service at the Mint, but for the greater glory of party politics in the election of 1705.» Westfall 1994, p. 245
- ^ «This Day in History: Isaac Newton is Knighted». History Channel. A&E Television Networks. 20 June 2016. Archived from the original on 19 August 2014. Retrieved 18 August 2014; and Barnham, Kay (2014). Isaac Newton. Raintree. p. 26. ISBN 978-1-4109-6235-5.
- ^ On the Value of Gold and Silver in European Currencies and the Consequences on the Worldwide Gold- and Silver-Trade Archived 6 April 2017 at the Wayback Machine, Sir Isaac Newton, 21 September 1717; «By The King, A Proclamation Declaring the Rates at which Gold shall be current in Payments». Royal Numismatic Society. V. April 1842 – January 1843.
- ^ Fay, C.R. (1 January 1935). «Newton and the Gold Standard». Cambridge Historical Journal. 5 (1): 109–117. doi:10.1017/S1474691300001256. JSTOR 3020836.
- ^ «Sir Isaac Newton’s Unpublished Manuscripts Explain Connections He Made Between Alchemy and Economics». Georgia Tech Research News. 12 September 2006. Archived from the original on 17 February 2013. Retrieved 30 July 2014.
- ^ Eric W. Nye, Pounds Sterling to Dollars: Historical Conversion of Currency Archived 15 August 2021 at the Wayback Machine. Retrieved: 5 October 2020
- ^ Holodny, Elena (21 January 2016). «Isaac Newton was a genius, but even he lost millions in the stock market». Business Insider. Archived from the original on 25 March 2016. Retrieved 21 December 2019.
- ^ Yonge, Charlotte M. (1898). «Cranbury and Brambridge». John Keble’s Parishes – Chapter 6. online-literature.com. Archived from the original on 8 December 2008. Retrieved 23 September 2009.
- ^ Westfall 1980, p. 44.
- ^ Westfall 1980, p. 595.
- ^ «No. 6569». The London Gazette. 1 April 1727. p. 7.
- ^ Dobre and Nyden suggest that there is no clear evidence that Voltaire was present; see p. 89 of Mihnea Dobre, Tammy Nyden (2013). Cartesian Empiricism. Springer. ISBN 978-94-007-7690-6.
- ^ a b «Newton, Isaac (1642–1727)». Eric Weisstein’s World of Biography. Eric W. Weisstein. Archived from the original on 28 April 2006. Retrieved 30 August 2006.
- ^ a b c d Mann, Adam (14 May 2014). «The Strange, Secret History of Isaac Newton’s Papers». Science. Archived from the original on 11 September 2017. Retrieved 25 April 2016.
- ^ Hutton, Charles (1795/6). A Mathematical and Philosophical Dictionary. vol. 2. p. 100.
- ^ Voltaire (1894). «14». Letters on England. Cassell. p. 100.
- ^ Hutton, Charles (1815). A Philosophical and Mathematical Dictionary Containing … Memoirs of the Lives and Writings of the Most Eminent Authors, Volume 2. p. 100.
- ^ Keynes, John Maynard. «Newton: the Man». University of St Andrews School of Mathematics and Statistics. Archived from the original on 17 June 2019. Retrieved 11 September 2012.
- ^ Sagan, Carl (1980). Cosmos. New York: Random House. p. 68. ISBN 978-0-394-50294-6.
- ^ «Duillier, Nicholas Fatio de (1664–1753) mathematician and natural philosopher». Janus database. Archived from the original on 1 July 2013. Retrieved 22 March 2013.
- ^ «Collection Guide: Fatio de Duillier, Nicolas [Letters to Isaac Newton]». Online Archive of California. Archived from the original on 31 May 2013. Retrieved 22 March 2013.
- ^ Westfall 1980, pp. 493–497 on the friendship with Fatio, pp. 531–540 on Newton’s breakdown.
- ^ Manuel 1968, p. 219.
- ^ Letter from Isaac Newton to Robert Hooke, 5 February 1676, as transcribed in Maury, Jean-Pierre (1992) [1990]. Newton: Understanding the Cosmos. «New Horizons» series. Translated by Paris, I. Mark. London: Thames & Hudson. ISBN 978-0-500-30023-7. Archived from the original on 21 December 2020. Retrieved 18 October 2020.
- ^ John Gribbin (2002) Science: A History 1543–2001, p. 164.
- ^ White 1997, p. 187.
- ^ Memoirs of the Life, Writings, and Discoveries of Sir Isaac Newton (1855) by Sir David Brewster (Volume II. Ch. 27)
- ^ «Is Islam Hostile to Science?». National Geographic News. 27 February 2015. Archived from the original on 9 January 2021. Retrieved 5 January 2021.
- ^ James, Ioan (January 2003). «Singular scientists». Journal of the Royal Society of Medicine. 96 (1): 36–39. doi:10.1177/014107680309600112. PMC 539373. PMID 12519805.
- ^ «Einstein and Newton showed signs of autism». Archived from the original on 18 June 2017. Retrieved 29 September 2021.
- ^ «Understanding Level 1 of Autism Spectrum Disorder | Ascend Autism». ascendautism.com. 12 February 2021. Retrieved 8 January 2023.
- ^ Richard S. Westfall – Indiana University The Galileo Project. (Rice University). Archived from the original on 29 September 2020. Retrieved 5 July 2008.
- ^ a b c d e Snobelen, Stephen D. (December 1999). «Isaac Newton, heretic: the strategies of a Nicodemite». The British Journal for the History of Science. 32 (4): 381–419. doi:10.1017/S0007087499003751. JSTOR 4027945. S2CID 145208136.
- ^ Westfall 1980, p. 315.
- ^ Westfall 1980, p. 321.
- ^ Westfall 1980, pp. 331–334.
- ^ Westfall 1994, p. 124.
- ^ Avery Cardinal Dulles. The Deist Minimum Archived 6 October 2013 at the Wayback Machine (January 2005).
- ^ «Newton, object 1 (Butlin 306) «Newton»«. William Blake Archive. 25 September 2013. Archived from the original on 27 September 2013. Retrieved 25 September 2013.
- ^ Newton, Isaac (1782). Isaaci Newtoni Opera quae exstant omnia. London: Joannes Nichols. pp. 436–437. Archived from the original on 14 April 2021. Retrieved 18 October 2020.
- ^ Observations upon the Prophecies of Daniel, and the Apocalypse of St. John Archived 20 January 2017 at the Wayback Machine 1733
- ^ John P. Meier, A Marginal Jew, v. 1, pp. 382–402. after narrowing the years to 30 or 33, provisionally judges 30 most likely.
- ^ Newton to Richard Bentley 10 December 1692, in Turnbull et al. (1959–77), vol 3, p. 233.
- ^ Opticks, 2nd Ed 1706. Query 31.
- ^ H.G. Alexander (ed) The Leibniz-Clarke correspondence, Manchester University Press, 1998, p. 11.
- ^ Tyson, Neil Degrasse (1 November 2005). «The Perimeter of Ignorance». Natural History Magazine. Archived from the original on 6 September 2018. Retrieved 7 January 2016.
- ^ Dijksterhuis, E. J. The Mechanization of the World Picture, IV 329–330, Oxford University Press, 1961. The author’s final comment on this episode is:»The mechanization of the world picture led with irresistible coherence to the conception of God as a sort of ‘retired engineer’, and from here to God’s complete elimination it took just one more step».
- ^ Brewster states that Newton was never known as an Arian during his lifetime, it was William Whiston, an Arian, who first argued that «Sir Isaac Newton was so hearty for the Baptists, as well as for the Eusebians or Arians, that he sometimes suspected these two were the two witnesses in the Revelations,» while others like Hopton Haynes (a Mint employee and Humanitarian), «mentioned to Richard Baron, that Newton held the same doctrine as himself». David Brewster. Memoirs of the Life, Writings, and Discoveries of Sir Isaac Newton. p. 268.
- ^ Jacob, Margaret C. (1976). The Newtonians and the English Revolution: 1689–1720. Cornell University Press. pp. 37, 44. ISBN 978-0-85527-066-7.
- ^ Westfall, Richard S. (1958). Science and Religion in Seventeenth-Century England. New Haven: Yale University Press. p. 200. ISBN 978-0-208-00843-5.
- ^ Haakonssen, Knud (1996). «The Enlightenment, politics and providence: some Scottish and English comparisons». In Martin Fitzpatrick (ed.). Enlightenment and Religion: Rational Dissent in Eighteenth-century Britain. Cambridge: Cambridge University Press. p. 64. ISBN 978-0-521-56060-3.
- ^ a b Isaac Newton and Apocalypse Now: a response to Tom Harpur’s «Newton’s strange bedfellows» Archived 27 September 2017 at the Wayback Machine (PDF) Stephen D. Snobelen
- ^ Grayling, A.C. (2016). The Age of Genius: The Seventeenth Century and the Birth of the Modern Mind. ISBN 978-1-4088-4329-1. Archived from the original on 14 April 2021. Retrieved 18 October 2020.
- ^ «Papers Show Isaac Newton’s Religious Side, Predict Date of Apocalypse». Associated Press. 19 June 2007. Archived from the original on 13 August 2007. Retrieved 1 August 2007.
- ^ Leiber, Fritz (1981). «Poor Superman». In Heinlein, Robert A. (ed.). Tomorrow, the Stars (16th ed.). New York: Berkley Book / published by arrangement with Doubleday & Company, Inc. p. 208.
First published in Galaxy magazine, July 1951; Variously titled Appointment in Tomorrow; in some reprints of Leiber’s story the sentence ‘That was the pebble..’ is replaced by ‘Which Newton did the world need then?’
- ^ Meyer, Michal (2014). «Gold, secrecy and prestige». Chemical Heritage Magazine. 32 (1): 42–43. Archived from the original on 20 March 2018. Retrieved 20 March 2018.
- ^ a b Kean, Sam (2011). «Newton, The Last Magician». Humanities. 32 (1). Archived from the original on 13 April 2016. Retrieved 25 April 2016.
- ^ Greshko, Michael (4 April 2016). «Isaac Newton’s Lost Alchemy Recipe Rediscovered». National Geographic. Archived from the original on 26 April 2016. Retrieved 25 April 2016.
- ^ Dry, Sarah (2014). The Newton papers : the strange and true odyssey of Isaac Newton’s manuscripts. Oxford: Oxford University Press. ISBN 978-0-19-995104-8.
- ^ a b «The Chymistry of Isaac Newton». Indiana University, Bloomington. Archived from the original on 26 April 2016. Retrieved 25 April 2016.
- ^ Levitin, Dimitri (March 2019). «Going for Gold». Literary Review. Archived from the original on 7 March 2019. Retrieved 6 March 2019.
- ^ Newman, William R (2018). Newton the Alchemist Science, Enigma, and the Quest for Nature’s «Secret Fire». Princeton University Press. ISBN 978-0-691-17487-7. Archived from the original on 2 April 2019. Retrieved 6 March 2019.
- ^ Gillispie, Charles Coulston (1960). The Edge of Objectivity: An Essay in the History of Scientific Ideas. Princeton University Press. p. 122. ISBN 0-691-02350-6.
- ^ Van Helmont, Iohannis Baptistae, Opuscula Medica Inaudita: IV. De Peste, Editor Hieronymo Christian Paullo (Frankfurt am Main) Publisher Sumptibus Hieronimi Christiani Pauli, typis Matthiæ Andreæ, 1707.
- ^ Flood, Alison (2 June 2020). «Isaac Newton proposed curing plague with toad vomit, unseen papers show». The Guardian. Archived from the original on 6 June 2020. Retrieved 6 June 2020.
- ^ Fred L. Wilson, History of Science: Newton citing: Delambre, M. «Notice sur la vie et les ouvrages de M. le comte J.L. Lagrange», Oeuvres de Lagrange I. Paris, 1867, p. xx.
- ^ a b Westminster Abbey. «Sir Isaac Newton Scientist, Mathematician and Astronomer». westminster-abbey.org. Archived from the original on 9 August 2022. Retrieved 19 January 2022.
- ^ «Newton beats Einstein in polls of Royal Society scientists and the public». The Royal Society. Archived from the original on 13 July 2017. Retrieved 24 August 2010.
- ^ «Opinion poll. Einstein voted ‘greatest physicist ever’ by leading physicists; Newton runner-up». BBC News. 29 November 1999. Archived from the original on 12 August 2017. Retrieved 17 January 2012.
- ^ Gleeson-White, Jane (10 November 2003). «Einstein’s Heroes». The Sydney Morning Herald. Archived from the original on 28 November 2019. Retrieved 29 September 2021.
- ^ a b Historic England. «Woolsthorpe Manor House, Colsterworth (1062362)». National Heritage List for England. Retrieved 5 October 2021.
- ^ «Silly relic-worship». The New York Times. 16 January 1881. p. 10. Archived from the original on 20 January 2019. Retrieved 12 July 2009.
- ^ a b Cunningham, Antonia, ed. (2002). Guinness World Records 2002. ISBN 978-0-553-58378-6. Archived from the original on 9 August 2022. Retrieved 12 July 2009.
- ^ White 1997, p. 86.
- ^ Numbers 2015, pp. 48–56.
- ^ Malament, David B. (2002). Reading Natural Philosophy: Essays in the History and Philosophy of Science and Mathematics. Open Court Publishing. ISBN 978-0-8126-9507-6. Archived from the original on 14 April 2021. Retrieved 18 October 2020.
- ^ Voltaire (1727). An Essay upon the Civil Wars of France, extracted from curious Manuscripts and also upon the Epick Poetry of the European Nations, from Homer down to Milton. London, England: Samuel Jallasson. p. 104. Archived from the original on 14 June 2021. Retrieved 14 June 2021. From p. 104: ‘In the like Manner Pythagoras ow’d the Invention of Musik to the noise of the Hammer of a Blacksmith. And thus in our Days Sir Isaak Newton walking in his Garden had the first Thought of his System of Gravitation, upon seeing an apple falling from a Tree.’
- ^ Voltaire (1786) heard the story of Newton and the apple tree from Newton’s niece, Catherine Conduit (née Barton) (1679–1740): Voltaire (1786). Oeuvres completes de Voltaire [The complete works of Voltaire] (in French). Vol. 31. Basel, Switzerland: Jean-Jacques Tourneisen. p. 175. Archived from the original on 9 July 2021. Retrieved 15 June 2021. From p. 175: «Un jour en l’année 1666, Newton retiré à la campagne, et voyant tomber des fruits d’un arbre, à ce que m’a conté sa nièce, (Mme Conduit) se laissa aller à une méditation profonde sur la cause qui entraine ainsi tous les corps dans une ligne, qui, si elle était prolongée, passerait à peu près par le centre de la terre.» (One day in the year 1666 Newton withdrew to the country, and seeing the fruits of a tree fall, according to what his niece (Madame Conduit) told me, he entered into a deep meditation on the cause that draws all bodies in a [straight] line, which, if it were extended, would pass very near to the center of the Earth.)
- ^ Berkun, Scott (2010). The Myths of Innovation. O’Reilly Media, Inc. p. 4. ISBN 978-1-4493-8962-8.
- ^ «Newton’s apple: The real story». New Scientist. 18 January 2010. Archived from the original on 21 January 2010. Retrieved 10 May 2010.
- ^ Hamblyn, Richard (2011). «Newtonian Apples: William Stukeley». The Art of Science. Pan Macmillan. ISBN 978-1-4472-0415-2.
- ^ «Revised Memoir of Newton (Normalized Version)». The Newton Project. Archived from the original on 14 March 2017. Retrieved 13 March 2017.
- ^ Conduitt, John. «Keynes Ms. 130.4:Conduitt’s account of Newton’s life at Cambridge». Newtonproject. Imperial College London. Archived from the original on 7 November 2009. Retrieved 30 August 2006.
- ^ I. Bernard Cohen and George E. Smith, eds. The Cambridge Companion to Newton (2002) p. 6
- ^ Alberto A. Martinez Science Secrets: The Truth about Darwin’s Finches, Einstein’s Wife, and Other Myths, p. 69 (University of Pittsburgh Press, 2011); ISBN 978-0-8229-4407-2
- ^ «Brogdale – Home of the National Fruit Collection». Brogdale.org. Archived from the original on 1 December 2008. Retrieved 20 December 2008.
- ^ «From the National Fruit Collection: Isaac Newton’s Tree». Retrieved 10 January 2009.[permanent dead link] Alternate Page Archived 5 July 2022 at the Wayback Machine Retrieved 5 July 2022.
- ^ ‘The Abbey Scientists’ Hall, A.R. p13: London; Roger & Robert Nicholson; 1966
- ^ a b «Famous People & the Abbey: Sir Isaac Newton». Westminster Abbey. Archived from the original on 16 October 2009. Retrieved 13 November 2009.
- ^ «Withdrawn banknotes reference guide». Bank of England. Archived from the original on 5 May 2010. Retrieved 27 August 2009.
- ^ Cassels, Alan. Ideology and International Relations in the Modern World. p. 2.
- ^ «Although it was just one of the many factors in the Enlightenment, the success of Newtonian physics in providing a mathematical description of an ordered world clearly played a big part in the flowering of this movement in the eighteenth century» by John Gribbin, Science: A History 1543–2001 (2002), p. 241 ISBN 978-0-7139-9503-9
- ^ Anders Hald 2003 – A history of probability and statistics and their applications before 1750 – 586 pages Volume 501 of Wiley series in probability and statistics Wiley-IEEE, 2003 Archived 2 June 2022 at the Wayback Machine Retrieved 27 January 2012 ISBN 0-471-47129-1
- ^ «Natures obvious laws & processes in vegetation – Introduction». The Chymistry of Isaac Newton. Archived from the original on 17 January 2021. Retrieved 17 January 2021. Transcribed and online at Indiana University.
- ^ Whiteside, D.T., ed. (1974). Mathematical Papers of Isaac Newton, 1684–1691. 6. Cambridge University Press. pp. 30–91. Archived 10 June 2016 at the Wayback Machine
- ^ «Museum of London exhibit including facsimile of title page from John Flamsteed’s copy of 1687 edition of Newton’s Principia«. Museumoflondon.org.uk. Archived from the original on 31 March 2012. Retrieved 16 March 2012.
- ^ Published anonymously as «Scala graduum Caloris. Calorum Descriptiones & signa.» in Philosophical Transactions, 1701, 824 Archived 21 January 2020 at the Wayback Machine–829;
ed. Joannes Nichols, Isaaci Newtoni Opera quae exstant omnia, vol. 4 (1782), 403 Archived 17 June 2016 at the Wayback Machine–407.
Mark P. Silverman, A Universe of Atoms, An Atom in the Universe, Springer, 2002, p. 49. Archived 24 June 2016 at the Wayback Machine - ^ Newton, Isaac (1704). Opticks or, a Treatise of the reflexions, refractions, inflexions and colours of light. Also two treatises of the species and magnitude of curvilinear figures. Sam. Smith. and Benj. Walford. Archived from the original on 24 February 2021. Retrieved 17 March 2018.
- ^ a b c d e f g Pickover, Clifford (2008). Archimedes to Hawking: Laws of Science and the Great Minds Behind Them. Oxford University Press. pp. 117–118. ISBN 978-0-19-979268-9. Retrieved 17 March 2018.
- ^ Swetz, Frank J. «Mathematical Treasure: Newton’s Method of Fluxions». Convergence. Mathematical Association of America. Archived from the original on 28 June 2017. Retrieved 17 March 2018.
Bibliography
- Ball, W.W. Rouse (1908). A Short Account of the History of Mathematics. New York: Dover. ISBN 978-0-486-20630-1.
- Christianson, Gale (1984). In the Presence of the Creator: Isaac Newton & His Times. New York: Free Press. ISBN 978-0-02-905190-0. This well documented work provides, in particular, valuable information regarding Newton’s knowledge of Patristics
- Craig, John (1958). «Isaac Newton – Crime Investigator». Nature. 182 (4629): 149–152. Bibcode:1958Natur.182..149C. doi:10.1038/182149a0. S2CID 4200994.
- Craig, John (1963). «Isaac Newton and the Counterfeiters». Notes and Records of the Royal Society of London. 18 (2): 136–145. doi:10.1098/rsnr.1963.0017. S2CID 143981415.
- Gjertsen, Derek (1986). The Newton Handbook. London: Routledge & Kegan Paul. ISBN 0-7102-0279-2.
- Levenson, Thomas (2010). Newton and the Counterfeiter: The Unknown Detective Career of the World’s Greatest Scientist. Mariner Books. ISBN 978-0-547-33604-6.
- Manuel, Frank E (1968). A Portrait of Isaac Newton. Belknap Press of Harvard University, Cambridge, MA.
- Stewart, James (2009). Calculus: Concepts and Contexts. Cengage Learning. ISBN 978-0-495-55742-5.
- Westfall, Richard S. (1980). Never at Rest. Cambridge University Press. ISBN 978-0-521-27435-7.
- Westfall, Richard S. (2007). Isaac Newton. Cambridge University Press. ISBN 978-0-19-921355-9.
- Westfall, Richard S. (1994). The Life of Isaac Newton. Cambridge University Press. ISBN 978-0-521-47737-6.
- White, Michael (1997). Isaac Newton: The Last Sorcerer. Fourth Estate Limited. ISBN 978-1-85702-416-6.
Further reading
Primary
- Newton, Isaac. The Principia: Mathematical Principles of Natural Philosophy. University of California Press, (1999)
- Brackenridge, J. Bruce. The Key to Newton’s Dynamics: The Kepler Problem and the Principia: Containing an English Translation of Sections 1, 2, and 3 of Book One from the First (1687) Edition of Newton’s Mathematical Principles of Natural Philosophy, University of California Press (1996)
- Newton, Isaac. The Optical Papers of Isaac Newton. Vol. 1: The Optical Lectures, 1670–1672, Cambridge University Press (1984)
- Newton, Isaac. Opticks (4th ed. 1730) online edition
- Newton, I. (1952). Opticks, or A Treatise of the Reflections, Refractions, Inflections & Colours of Light. New York: Dover Publications.
- Newton, I. Sir Isaac Newton’s Mathematical Principles of Natural Philosophy and His System of the World, tr. A. Motte, rev. Florian Cajori. Berkeley: University of California Press (1934)
- Whiteside, D.T., ed. (1967–1982). The Mathematical Papers of Isaac Newton. Cambridge: Cambridge University Press. ISBN 978-0-521-07740-8. – 8 volumes.
- Newton, Isaac. The correspondence of Isaac Newton, ed. H.W. Turnbull and others, 7 vols (1959–77)
- Newton’s Philosophy of Nature: Selections from His Writings edited by H.S. Thayer (1953; online edition)
- Isaac Newton, Sir; J Edleston; Roger Cotes, Correspondence of Sir Isaac Newton and Professor Cotes, including letters of other eminent men, London, John W. Parker, West Strand; Cambridge, John Deighton (1850, Google Books)
- Maclaurin, C. (1748). An Account of Sir Isaac Newton’s Philosophical Discoveries, in Four Books. London: A. Millar and J. Nourse
- Newton, I. (1958). Isaac Newton’s Papers and Letters on Natural Philosophy and Related Documents, eds. I.B. Cohen and R.E. Schofield. Cambridge: Harvard University Press
- Newton, I. (1962). The Unpublished Scientific Papers of Isaac Newton: A Selection from the Portsmouth Collection in the University Library, Cambridge, ed. A.R. Hall and M.B. Hall. Cambridge: Cambridge University Press
- Newton, I. (1975). Isaac Newton’s ‘Theory of the Moon’s Motion’ (1702). London: Dawson
Alchemy
- Craig, John (1946). Newton at the Mint. Cambridge, England: Cambridge University Press.
- Craig, John (1953). «XII. Isaac Newton». The Mint: A History of the London Mint from A.D. 287 to 1948. Cambridge, England: Cambridge University Press. pp. 198–222. ASIN B0000CIHG7.
- de Villamil, Richard (1931). Newton, the Man. London: G.D. Knox. – Preface by Albert Einstein. Reprinted by Johnson Reprint Corporation, New York (1972)
- Dobbs, B.J.T. (1975). The Foundations of Newton’s Alchemy or «The Hunting of the Greene Lyon». Cambridge: Cambridge University Press.
- Keynes, John Maynard (1963). Essays in Biography. W.W. Norton & Co. ISBN 978-0-393-00189-1. Keynes took a close interest in Newton and owned many of Newton’s private papers.
- Stukeley, W. (1936). Memoirs of Sir Isaac Newton’s Life. London: Taylor and Francis. (edited by A.H. White; originally published in 1752)
- Trabue, J. «Ann and Arthur Storer of Calvert County, Maryland, Friends of Sir Isaac Newton,» The American Genealogist 79 (2004): 13–27.
Religion
- Dobbs, Betty Jo Tetter. The Janus Faces of Genius: The Role of Alchemy in Newton’s Thought. (1991), links the alchemy to Arianism
- Force, James E., and Richard H. Popkin, eds. Newton and Religion: Context, Nature, and Influence. (1999), pp. xvii, 325.; 13 papers by scholars using newly opened manuscripts
- Pfizenmaier, Thomas C (1997). «Was Isaac Newton an Arian?». Journal of the History of Ideas. 58 (1): 57–80. doi:10.1353/jhi.1997.0001. JSTOR 3653988. S2CID 170545277.
- Ramati, Ayval (2001). «The Hidden Truth of Creation: Newton’s Method of Fluxions». The British Journal for the History of Science. 34 (4): 417–438. doi:10.1017/S0007087401004484. JSTOR 4028372. S2CID 143045863.
- Snobelen, Stephen D. (2001). «‘God of Gods, and Lord of Lords’: The Theology of Isaac Newton’s General Scholium to the Principia». Osiris. 16: 169–208. Bibcode:2001Osir…16..169S. doi:10.1086/649344. JSTOR 301985. S2CID 170364912.
- Snobelen, Stephen D. (December 1999). «Isaac Newton, heretic: the strategies of a Nicodemite». The British Journal for the History of Science. 32 (4): 381–419. doi:10.1017/S0007087499003751. JSTOR 4027945. S2CID 145208136.
Science
- Bechler, Zev (2013). Contemporary Newtonian Research (Studies in the History of Modern Science)(Volume 9). Springer. ISBN 978-94-009-7717-4.
- Berlinski, David. Newton’s Gift: How Sir Isaac Newton Unlocked the System of the World. (2000); ISBN 0-684-84392-7
- Chandrasekhar, Subrahmanyan (1995). Newton’s Principia for the Common Reader. Oxford: Clarendon Press. ISBN 978-0-19-851744-3.
- Cohen, I. Bernard and Smith, George E., ed. The Cambridge Companion to Newton. (2002). Focuses on philosophical issues only; excerpt and text search; complete edition online «The Cambridge Companion to Newton». Archived from the original on 8 October 2008. Retrieved 13 October 2008.
{{cite web}}: CS1 maint: bot: original URL status unknown (link) - Cohen, I.B. (1980). The Newtonian Revolution. Cambridge: Cambridge University Press. ISBN 978-0-521-22964-7.
- Gleick, James (2003). Isaac Newton. Alfred A. Knopf. ISBN 978-0-375-42233-1.
- Halley, E. (1687). «Review of Newton’s Principia». Philosophical Transactions. 186: 291–297.
- Hawking, Stephen, ed. On the Shoulders of Giants. ISBN 0-7624-1348-4 Places selections from Newton’s Principia in the context of selected writings by Copernicus, Kepler, Galileo and Einstein
- Herivel, J.W. (1965). The Background to Newton’s Principia. A Study of Newton’s Dynamical Researches in the Years 1664–84. Oxford: Clarendon Press.
- Newton, Isaac. Papers and Letters in Natural Philosophy, edited by I. Bernard Cohen. Harvard University Press, 1958, 1978; ISBN 0-674-46853-8.
- Numbers, R.L. (2015). Newton’s Apple and Other Myths about Science. Harvard University Press. ISBN 978-0-674-91547-3.
- Pemberton, H. (1728). «A View of Sir Isaac Newton’s Philosophy». The Physics Teacher. 4 (1): 8–9. Bibcode:1966PhTea…4….8M. doi:10.1119/1.2350900.
- Shamos, Morris H. (1959). Great Experiments in Physics. New York: Henry Holt and Company, Inc. ISBN 978-0-486-25346-6.
External links
This audio file was created from a revision of this article dated 30 July 2008, and does not reflect subsequent edits.
Writings by Newton
- Newton’s works – full texts, at the Newton Project
- Newton’s papers in the Royal Society’s archives
- The Newton Manuscripts at the National Library of Israel – the collection of all his religious writings
- Works by Isaac Newton at Project Gutenberg
- Works by or about Isaac Newton at Internet Archive
- Works by Isaac Newton at LibriVox (public domain audiobooks)
- «Newton Papers» – Cambridge Digital Library
Знаменитый английский ученый Исаак Ньютон не случайно признан одним из выдающихся умов мировой науки. Этот человек сделал бесценный вклад в развитие физики и математики, разработал множество уникальных теорий, большинство которых было подтверждено более поздними научными исследованиями. Его гениальность проявилась во многих научных сферах, включая астрономию и философию. Ньютона по праву считают основоположником классической физики.
Происхождение и ранние годы
Родина Исаака Ньютона – усадьба Вулсторп, где он родился 4 января 1643 года немного раньше положенного срока. Его отец, Исаак Ньютон, довольно зажиточный фермер, умер, не дождавшись появления сына на свет. Новорожденный Исаак был настолько слабеньким, что доктора не давали никаких гарантий, но, вопреки их прогнозам, мальчику удалось выжить.
Дом, где родился Ньютон. Вулсторп
Мать Ньютона звали Анна Эйскоу. После смерти мужа она получила в наследство 500 фунтов стерлингов и несколько сотен акров земли, что по тем временам считалось неплохим состоянием. Вскоре женщина снова вышла замуж, и через некоторое время в семье появилось еще трое детей. Из-за ухода за малышами у матери совсем не оставалось времени на воспитание Исаака, поэтому эту заботу взяли на себя его бабушка и дядя, Уильям Эйскоу. Ньютон часто болел, рос молчаливым и замкнутым, предпочитал в полном уединении читать или мастерить какие-нибудь игрушки. Так, еще в детстве он сконструировал водяные часы и ветряную мельницу. Когда Исааку исполнилось десять лет, его мать овдовела во второй раз. В двенадцать лет он поступил в школу, которая находилась недалеко от Грэнтема.
Образование
Ньютон оказался хорошим учеником и с легкостью осваивал новые дисциплины. Также у него проснулся талант к сочинительству стихов, а чтение продолжало оставаться любимым занятием подростка. Когда Исаак достиг шестнадцатилетия, мать решила забрать его в поместье, потому что ей понадобился помощник по хозяйству. Только благодаря уговорам дяди и знакомого семьи, Хэмфри Бабингтона, она согласилась оставить Ньютона в школе. В 1661 году юноша успешно окончил учебное заведение и поступил в Кембриджский университет.
Г. Кнеллер. Портрет Ньютона. 1689
В университете Ньютон получил статус «sizar», так как не оплачивал свое обучение. Взамен этого он должен был помогать в уборке и выполнять другие работы в университете. Всё это ущемляло самолюбие Исаака, но он преодолел это испытание, так как тяга к науке не позволяла ему показывать свое раздражение. Молодой человек все так же предпочитал одиночество и не пытался завести друзей.
В основе университетских программ по философии и естествознанию лежали труды Аристотеля, хотя весь научный мир того времени уже знал об открытиях Галилея и других ученых. По этой причине Ньютон самостоятельно, после основных занятий штудировал труды Коперника, Кеплера, уже упомянутого Галилея, чтобы получить более обширные знания в области физики, астрономии, математики.
Научная деятельность
Ньютону повезло попасть под покровительство английского математика Исаака Барроу, который смог рассмотреть в юноше огромный потенциал. Именно Барроу одобрительно принял первое открытие молодого ученого, которое легло в основу математического метода, и за которое Ньютон получил степень бакалавра.
После этого Исаак приступил к разработке новой теории, которая впоследствии получила название Закона всемирного тяготения. Это была очень кропотливая работа, так как Ньютону пришлось обобщить знания таких ученых, как Галилией, Декарт и Кеплер. Исследования прервала начавшаяся в 1665 году эпидемия чумы, и Ньютон был вынужден вернуться в Вулсторп, прихватив с собой учебники и инструменты.
В своем поместье, в полном уединении Ньютон вновь занялся научными исследованиями. В 1666 году
Потомок дерева, вдохновившего Ньютона на Закон о гравитации
молодой ученый сделал важное открытие, что белый солнечный свет, пропущенный через призму – это комбинация всех видимых цветов спектра. Если же сложить все цвета и пропустить их через установленные определенным образом призмы, то в итоге можно снова получить белый свет. Окончание работы над Законом всемирного тяготения также пришлось на этот период. Ньютон был напрочь лишен тщеславия, поэтому о его открытиях стало известно лишь через двадцать лет. В 1667 году Ньютон вернулся в Кембридж и получил степень магистра Тринити-колледжа. Ему была предоставлена отдельная комната, назначено небольшое содержание и дана возможность читать лекции. Студенты без особого желания посещали его занятия, так как у Ньютона так и не развился талант педагога, а его лекции оставляли желать лучшего. В этот период Ньютон заинтересовался алхимией, но основной акцент его исследований был направлен на математику и оптику.
В 1672 году Ньютон стал членом Королевского общества. Его знаменитый телескоп, над которым он трудился, находясь в своем имении во время эпидемии, был продемонстрирован самому королю Карлу II. Изобретение Ньютона произвело фурор в научных кругах и стало предметом национальной гордости Англии.
В конце семидесятых годов в жизни Ньютона произошла череда печальных событий. Умер его наставник, ученый Исаак Барроу, Люди, сменившие старое руководство Королевского общества, относились к Ньютону весьма недоброжелательно. Через некоторое время в доме ученого произошел пожар, в результате которого сгорело много ценных рукописей. Наконец, тяжело заболела и вскоре умерла мать Исаака. Но, несмотря ни на что, ученый продолжал работать.
Рефлектор Ньютона
Ньютону удалось закончить свою фундаментальную работу «Математические начала натуральной философии», которую он начал в 1682 году. В ней был сформулирован закон всемирного тяготения и законы движения планет с описанием их орбит, даны основные определения механики, введены новые физические величины. Сначала Ньютон отказался от публикации этого труда, несмотря на многочисленные просьбы ученых, но затем, вопреки своим принципам, все же согласился. Средства для этого предоставил Эдмонд Галлей, так как у Королевского общества на тот момент не было денег. В 1687 году в свет вышло трехтомное издание общим, огромным для того времени тиражом три тысячи экземпляров, которое было моментально раскуплено. Труд Ньютона имел такой успех, что еще при его жизни переиздавался несколько раз.
В 1696 году Ньютона назначили хранителем Тауэрского монетного двора. Вникнув в технологию отливки монет, он разработал способ, позволяющий отличать фальшивые деньги от настоящих, а также стал участником проводимой в то время денежной реформы. Через некоторое время на Ньютона начали поступать доносы. Как оказалось, их писали фальшивомонетчики, которые очень боялись быть разоблаченными при помощи методов Ньютона. В 1703 году Ньютон был избран президентом Королевского Общества, в 1705 году он подошел к завершению своего научного труда «Оптика», который на целых два столетия определил направление развития этой науки. Идеи ученого приобретали все больше последователей, а его теорию движения небесных тел ввели в учебную программу университетов.
Тринити-колледж
В том же 1705 году Ньютону было присвоено звание рыцаря, которое давало сэру Исааку Ньютону право принимать участие в правительственных организациях. В этот период у ученого проснулся интерес к истории, и на этой волне им была написана «Хронология древних царств». В это же время готовилась к выходу в свет третья книга его «Начал» с правками и дополнениями, а также рассчитанной орбитой кометы Галлея.
Научные достижения Ньютона
Исааком Ньютоном сделано много важных открытий, послуживших дальнейшему развитию науки. Многие из его физических законов не потеряли своей актуальности и на сегодняшний день.
Три закона движения
Этот труд по праву считается одним из важнейших открытий Ньютона. Работу, которая появились в 1687 году под названием «Математические начала натуральной философии», можно назвать основой классической механики и огромным вкладом в развитие физики. Три ньютоновских закона были разработаны на основе теории движения планет Иоганна Кеплера и сформулированы следующим образом:
- Тело будет оставаться в покое до тех пор, пока на него не начнет воздействовать сила, лишенная баланса. Движущийся объект будет иметь те же изначальные скорость и направление, если не встретит на своем пути несбалансированную силу. Этот закон Ньютона также называют Законом инерции.
- Второй закон Ньютона гласит о взаимозависимости массы тела, ускорения и силы. Ускорение начинает проявляться во время действия силы на массу. Ускорение тела прямо пропорционально приложенной к нему силе и обратно пропорционально массе.
- В формулировке самого Ньютона его третий закон выглядит так: «Действию всегда есть равное и противоположное противодействие, иначе, взаимодействия двух тел друг на друга между собою равны и направлены в противоположные стороны. Для каждого действия существует равное противодействие».
Универсальная гравитация
Закон всемирного тяготения был открыт Ньютоном в 1667 году. В своем труде он обобщил результаты, полученные Галилеем, и впоследствии Кеплером. В итоге он пришел к выводу, что все тела во Вселенной притягиваются друг к другу, при этом можно рассчитать силу, с которой это притяжение происходит. Это и есть Закон всемирного тяготения, а сила притяжения называется гравитацией. Согласно легенде, во время прогулки в саду в своей усадьбе на голову Ньютона упало яблоко, и в этот момент к нему пришло озарение: если луна остается неподвижной, а яблоко падает, это означает, что какая-то сила воздействует и на Луну и на яблоко. Многие историки считают этот случай не иначе, как выдумкой. Однако, не суть важно, действительно ли падало яблоко на голову ученого, важно то, что он первый понял, что гравитация только одна и ее действие можно описать универсальным физическим законом.
Форма Земли
Раньше было принято считать, что Земля имеет идеально круглую форму шара. Это доказывали великие ученые древности: Пифагор, Аристотель и др. В 1687 году Ньютон, опираясь на открытый им закон всемирного тяготения, выдвинул теорию о том, что Земля сплюснута у полюсов. Впоследствии эта гипотеза получила безусловное подтверждение.
Оптика
Ньютон пропускает свет через призму
В начале 18 века Ньютон написал фундаментальный научный труд под названием «Оптика». На ближайшее столетие эта книга стала базовой в развитии этого раздела физики. Ньютоном был создан первый зеркальный телескоп (рефлектор Ньютона) с особым расположением зеркал, что позволяло получить изображение в хорошем качестве. В 1672 году ученым была открыта дисперсия света. Установив, что при прохождении через призму белый свет раскладывается в радужный спектр, Ньютон стал одним из основателей теории цвета.
Много работ Ньютона посвящено термодинамике, звуку, изучению солнечной энергии, им был сформулирован закон Ньютона-Рихмана, который касался вопросов тепловой передачи, а также выведена математическая формула, известная как Бином Ньютона.
Последние годы
Могила Ньютона в Вестминстерском аббатстве
К середине двадцатых годов здоровье Ньютона стало ухудшаться, и он перебрался в пригород Лондона Кенсингтон. В 1725 году ученый уже не мог ходить на службу и 31 марта 1727 года тихо скончался во сне в своей постели. В день похорон в Лондоне был объявлен общенациональный траур, а проводить рыцаря английской науки пришел весь город. Похоронили Ньютона в Вестминстерском аббатстве, где покоятся короли и другие великие люди Англии. На могиле сэра Исаака Ньютона установлен необычный памятник, на котором изображены главные открытия ученого.
Личная жизнь
Главным в жизни Ньютона была наука, и всего себя он посвящал только ей. Возможно, по этой причине ученый так и не создал семью и не оставил после себя наследников.
Хронологическая таблица
| Год (годы) | Событие |
| 25.12.1642 | Дата рождения Исаака Ньютона |
| 1648 | Поступление в школу |
| 1655 | Поступление в Королевскую школу в Грэнтэме |
| 1664 | Становится стипендиатом Тринити-колледжа |
| 1665 | Присвоение звания бакалавра искусств. Отъезд в Вулсторп |
| 1667 | Возвращение в Кембридж |
| 1669 | Назначение лукасианским профессором математики |
| 1671 | Демонстрация телескопа в Королевском обществе |
| 1672 | Избрание в члены Королевского общества. Наброски книги «Оптика» |
| 1677 | Смерть И. Барроу. Пожар в квартире Ньютона |
| 1679 | Смерть матери |
| 1685 | Формулировка закона всемирного тяготения |
| 1687 | Выход первого издания книги «Математические начала натуральной философии» |
| 1689 | Трактат «О природе кислот» |
| 1696 | Назначение смотрителем Монетного двора. Переезд в Лондон |
| 1698 | Избрание членом Парижской академии наук |
| 1703 | Избрание президентом Королевского общества |
| 1704 | Выход первого издания «Оптики» |
| 1705 | Возведение в рыцарское звание |
| 1713 | Второе издание «Начал» |
| 1717 | Второе издание «Оптики» |
| 31.03.1727 | Дата смерти Исаака Ньютона |
Интересные факты
- Исаак Ньютон назван в честь своего отца, умершего до его рождения;
- всю жизнь ученый считал своими предками шотландских дворян, но его биографы позже установили, что он имеет крестьянское происхождение;
- в юном возрасте будущий ученый увлекался стихосложением;
- уже в возрасте 23 лет Ньютон совершил ряд важных открытий в области математики;
- Ньютона редко можно было увидеть смеющимся, он был очень сдержан в эмоциях;
- ученый был близорук, но никогда не пользовался очками;
- Ньютон был равнодушен к любым видам искусства, не проявлял он интереса и к путешествиям;
- очень не любил публиковать свои труды, поэтому многие из них мир увидел только через два-три десятка лет;
- несколько лет последнего периода жизни Ньютон посвятил написанию богословской книги, которая сгорела при пожаре;
- Ньютону принадлежит идея делать край монеты ребристым, так как мошенники часто срезали с них кусочки металла;
- несмотря на то, что врачи пророчили Ньютону смерть еще в детстве, ученый прожил долгую жизнь и умер в 84 года.
Память
Памятник Ньютону в Оксфорде
Существует международная награда в области физики в виде Медали Исаака Ньютона. К ней прилагается денежная премия в размере 1000 фунтов стерлингов и сертификат.
В честь Ньютона назван так называемый элемент «х» – мировой эфир. По мнению Д.И. Менделеева, этот гипотетический элемент должен был располагаться в самом начале всей Таблицы (нулевая группа нулевого ряда).
Имя Ньютона носит горная вершина на острове Западный Шпицберген, высшая точка одноименного архипелага.
В Эльдорадо штата Арканзас работает Дом-музей Исаака Ньютона.
Скульптура у здания Британской библиотеки
В честь великого ученого воздвигнуты памятники. В частности, статуя Ньютона расположена над входом в Тринити-колледж Кембриджского университета. В Музее национальной истории Оксфордского университета установлен памятник Ньютону. Необычная скульптура установлена во дворе Британской библиотеки в Лондоне. Она представляет собой Ньютона с циркулем в руках, склонившегося к земле, словно измеряющего бесконечность Вселенной.
Цитаты
«То, что мы знаем, это капля, а то, что мы не знаем, это океан».
«Природа проста и не роскошествует излишними причинами».
«При изучении наук примеры полезнее правил».
«Гений есть терпение мысли, сосредоточенной в известном направлении».
«Опыт – это не то, что происходит с вами; это то, что вы делаете с тем, что происходит с вами».
«Действию всегда есть равное и противоположное противодействие».
«Природа неистощима в своих выдумках».
Литература
- Вавилов С. И. Исаак Ньютон. — 2-е доп. изд. — М.—Л.: Изд. АН СССР, 1945.
- Кобзарев И. Ю. Ньютон и его время. — М.: Знание, 1978.
- Акройд П. Исаак Ньютон. Биография. — М.: КоЛибри, Азбука-Аттикус, 2011.
- Храмов Ю. А. Ньютон Исаак (Newton Isaac) // Физики : Биографический справочник / Под ред. А. И. Ахиезера. — Изд. 2-е, испр. и доп. — М. : Наука, 1983.
- Кузнецов Б. Г. Ньютон. — М.: Мысль, 1982.
Ирина Зарицкая | Просмотров: 2.3k
В современном мире люди крайне редко задумываются над устройством нашего мира, ведь, казалось бы, все уже давно известно и открыто. Но в далекие времена Нового времени было понятно далеко не все. Люди все еще сомневались в гелиоцентрическом устройстве нашей Солнечной системы, а многие законы физики и вовсе были неизвестны. К счастью, было множество ученых, стремящихся познать тайны нашей Вселенной, несмотря на церковные запреты. Одним из таких и был известный всем физик, математик, изобретатель Исаак Ньютон. Очень многие люди слышали миф, что закон гравитации якобы был создан знаменитым ученым Исааком Ньютоном, когда на голову тому упало яблоко. Впервые об этом было упомянуто в его биографии, но даже там яблоко упало не на самого физика, а рядом с ним. На самом же деле и это не больше, чем просто красивая легенда, распространению которой поспособствовал писатель Вольтер. Закон всемирного тяготения формировался постепенно, как видно по записям самого Ньютона. Конечно, ученый разработал не только один этот закон, на его счету также довольно много изобретений и открытий в разных научных сферах. Именно он стал основоположником механики, сформировав основные ее законы. Преуспел он и в математике, создав ряд вычислений, а также один из методов нахождения корней функции. Сверх всего этого и прочего ряда немалых заслуг, он улучшил монетную систему Англии. Кстати, таким же достижением отметился в истории Коперник, исследования которого стали фундаментом для работы Ньютона.
Происхождение детство
Предки великого физика и математика были обычными крестьянами, хоть он и думал, что его корни восходят к древнему шотландскому роду. Исаак Ньютон родился в маленьком поместье Вулсторп в Англии. Отец его был преуспевающим фермером. Он умер еще до рождения сына, а потому будущего ученого назвали в его честь, также Исааком.
Изначально Ньютон выглядел очень болезненным, так как родился недоношенным, а потому его долгое время не крестили, ведь думали, что он не выживет. Когда ему исполнилось четыре, мать Ньютона снова вышла замуж, и в новом браке у нее родилось еще трое детей, а потому Исааку она внимания почти не уделяла. Это повлияло на то, что он был молчаливым и почти ни с кем не общался, а все свое свободное время будущий изобретатель читал и изготавливал технические игрушки. В семье он был белой вороной, как и в родном селе. Его часто просили о помощи по хозяйству, но Исаак был трудным ребенком и не желал ничего делать, кроме того, что было ему интересно. Мать считала сына никудышным фермером и корила за лень. Никто не понимал в деревне такого хобби, как чтение.
Ньютон в детстве
В 1655 году Ньютона отправили в школу в городе Грэнтеме, где он показывал выдающиеся способности, однако его мать забрала его назад спустя четыре года, так как требовалась помощь по хозяйству. Но школьный преподаватель Ньютона, а также несколько других людей науки, заинтересованные в дальнейшей судьбе одаренного парня, не без труда уговорили Анну вернуть его обратно в школу, чтобы в дальнейшем он смог поступить в Кембриджский университет, что и случилось в 1661 году. В такие перспективы тогда было трудно поверить, скепсис Анны можно понять. Сын фермера в Кембридже — такая остроумная шутка могла бы иметь успех на базарной площади. Но учитель вовсе не шутил.
Юность и образование
Исаак Ньютон попал в Тринити-колледж Кембриджского университета в 18 лет. Учебный процесс поначалу шел очень плохо из-за прошедшей недавно гражданской войны, вследствие которой выплаты постоянно задерживались, а преподавательский состав был очень мал.
Ньютон состоял в ряде студентов, которые могли не платить за свое обучение, выполняя вместо этого различную работу в самом университете. Таково было понимание бюджетного отделения в те времена. Друзей у ученого все еще не было. Другие ученики презирали его за бедность и неродовитость.
статуя и яблоня Ньютона в Кембридже
Спустя три года Ньютон справился с экзаменами и начал получать стипендию, переведясь на более старший курс. Он проводил все свое время за учебой и получением новых знаний, иногда забывая даже о базовых потребностях вроде сна или приема пищи. Тогда же время в университете появился новый преподаватель – Исаак Барроу, пробудивший в Ньютоне интерес к математике с новой силой. Уже тогда молодой ученый открыл метод разложения функции в бесконечный ряд.
В конце 1664 года Ньютон получил степень бакалавра. Но в через год у Исаака не было возможности больше оставаться в Тринити-колледже в связи с эпидемией чумы, свирепствующей тогда в Англии.
Научная карьера
Как раз таки годы чумы стали для Исаака Ньютона самыми продуктивными, не исключая того, что для остальных англичан это время было очень бедственным. Самым, наверное, важным открытием в этот период стал закон Всемирного тяготения, к которому ученый шел продолжительное время. Сначала в законе были допущены некоторые погрешности, так как ученый брал сведения из писаний Галилея, однако после использования данных, предоставленных Пикаром, неточности были устранены. Но все открытия Ньютона, сделанные им в этот период времени, были опубликованы гораздо позже, примерно через 20-30 лет. Ньютон не гнался за славой и считал, что известность несет с собой новые знакомства, которых ученый старался избегать. Он ценил уединение и утверждал, что только в таком состоянии к нему приходят гениальные мысли. В этом он был похож на Николу Теслу, который так никогда и не женился.
В 1667 году, после окончания вспышек чумы, Ньютон возвратился в Тринити-колледж, где стал магистром. Сначала он взялся за преподавание, однако успешным это дело не стало, а на его лекции почти никто не приходил. Слишком сложно и занудно. Теории Ньютона опережали время.
портреты Ньютона
Через два года бывший наставник Ньютона, Исаак Барроу, убедил молодого ученого написать небольшую работу на тему разложения в бесконечные ряды, чем он и занялся. Трактат был переслан в Лондон, и в итоге получил небольшую известность в Англии и за границей. Вскоре Ньютон стал профессором математики и оптики в Тринити-колледже. Ньютон всерьез занялся и алхимией, делая различные опыты в лаборатории, оставленной ему Барроу. В те времена эта почти колдовская наука была праматерью химии, хоть и преследовала метафизические цели вроде создания философского камня. Сам Ньютон верил в возможность его открытия и даже посвящал поискам некоторые работы.
В 1668 году Исаак занялся созданием телескопа-рефлектора, и хотя первая попытка провалилась, следующая уже отличалась хорошим качеством. Изобретение посчитали очень полезным и достойным, оно получило положительный отзыв даже у короля, и поэтому Ньютон стал членом Королевского общества в 1672 году. Однако постоянные научные споры отрицательно сказывались на здоровье и настроении Исаака. Ему не нравилась критика его трудов и постоянные нападки со стороны остальных членов общества, а потому уже через год он вышел из общества. По его мнению, ученые больше любили болтать, чем делать. У большинства завсегдатаев общества вообще не было достижений. А вот биография физика Ньютона насчитывает их немало.
Открытия Ньютона
изобретения Ньютона
Что изобрел Ньютон? Что открыл ученый? Вот краткая выжимка:
- Три закона движения. В этих законах раскрываются основы механики. Первый закон – объект будет оставаться в состоянии покоя, пока на него не повлияет сила, лишенная баланса. Второй – ускорение появляется, пока сила влияет на массу. Третий – для каждого действия существует равное противодействие.
- Закон Всемирного тяготения гласит, что каждая точка массы притягивает другую. Этот же закон доказал научному миру, что Земля не является центром Вселенной, и что строение нашей Солнечной системы гелиоцентрическое. То, за что держали в тюрьме Галилея, оказалось научной истиной, а не ересью.
- Ньютон сделал открытие и в области оптики, доказав, что цвет формируется из-за взаимодействия изначально окрашенных объектов.
- Также немаловажным является вышеупомянутое изобретение Ньютона – телескоп-рефлектор, который использовался астрономами еще очень долгое время и имел большую практическую важность в те времена.
- Ньютон изобрел прототип дрезины — транспортного средства, которое до сих пор используется в шахтах и на ЖД.
Интересные факты о Ньютоне
Жизнью Ньютона богата интересными фактами:
- В детстве Ньютон смастерил очень точные водяные часы, которыми пользовалась вся его семья.
- До поступления в Кембриджский университет Ньютон часто писал стихотворения.
- Ньютона очень вдохновляли гениальные ученые прошлого – Кеплер, Декарт и Галилей.
- Исаак первым доказал, что белый является смешением всех остальных цветов.
- Книга, написанная Ньютоном, стала основой оптики на ближайший век.
- Ньютон был очень молчаливым, и даже на собрании палаты лордов, которые он часто посещал, он ничего не говорил.
- Исаак Ньютон немного заикался.
- Ньютон не спешил показывать свои достижения. Он годами копил результаты опытов, шлифовал формулировки, добивался полной ясности, чтобы никто не мог оспорить его доводы. Его работы выходили в свет лишь спустя 20-30 лет трудов.
- Ньютон определил семья цветов основного спектра не сразу, сначала он выбрал пять.
- 10.В последние годы Ньютон работал над книгой, посвященной философии и богословию. Но она сгорела при пожаре и не сохранилась. А ведь ее ученый считал главным достижением в жизни.
- Именно Ньютон занял место на первом логотипе компании Apple. Но потом того решили сделать более лаконичным и оставили только яблоко.
- Ученый верил в Бога, но не был частым гостем церкви. Зато он пытался расшифровать Библию. По его мнению, там зашифрована реальная истина, которую люди просто не могут понять. Он хотел разгадать замысел Бога и считал, что храм царя Соломона на земле обетованной хранит ключи к шифру.
- Ньютон стремился изобрести элексир бессмертия, но не преуспел. Также он искал Атлантиду и думал, что многие ответы можно получить там.
- Чтобы помешать мошенникам срезать слои с золотых и серебряных монет, Ньютон придумал ребристую поверхность по краям. Также он увеличил темпы изготовления денег в 8 раз, переведя монетный двор на круглосуточный режим работы.
- Изучая различные религиозные трактаты и применяя расчеты, Ньютон прогнозировал конец света в 2060 году. Что ж, посмотрим…
- Сегодня многие исследователи говорят о том, что в конце жизни Ньютон немного сошел с ума. Он не выходил из дома, писал очень странные вещи и забросил даже науку. Вот такая интересная биография Ньютона.
Личная жизнь
Биография и открытия Ньютона тесно связаны. Всю свою жизнь Исаак Ньютон оставался очень молчаливым и нелюдимым. Он не искал новых знакомств и друзей, а все свое свободное время посвящал науке. Немногие из его знакомых отмечали то, что он был очень спокойным и рассудительным, но проявлял некоторую рассеянность из-за того, что всегда был сосредоточен на своей работе и ставил ее превыше всего.
Но он не был бессердечным, своих родственников он любил и очень уважал. К примеру, детей своей умершей сводной сестры он взял на попечение, и в течение жизни помогал всем остальным родственником. Но за все время он так и не обзавелся собственной семьей и детьми, потому что не видел в этом смысла, да и времени на любовные романы у него не было. Впрочем, он не один такой. Например, тот же Эйнштейн отправил первую дочку в аналог детского дома — на воспитание другой семье. Ребенок мешал его работе.
Последние годы
В 1705 году Ньютон стал рыцарем. Это стало первым случаем, когда кого-либо делали рыцарем за научные заслуги. В следующий раз это произошло только через сто лет.
Спустя три года Исаак начал открытый спор с Готфридом Лейбницем, математиком и философом. Этот спор не прекратился и после смерти Готфрида. В 1714 году Исаак Ньютон предложил внести корректировку в стоимость золота и серебра, и значительная часть этих предложений была принята, что хорошо сказалось на экономике Англии.
Статуи Лейбница и Ньютона
За 7 лет до смерти Ньютон попал в крупную финансовую аферу, которую провела «Компания Южных морей», в результате которой он и еще многие вкладчики потеряли тысячи долларов. Одной из последних работ ученого, над которой он трудился весьма продолжительное время, была «Хронология древних царств».
В 1725 году здоровье великого физика стало ухудшаться, а потому он переехал в Кенсингтон, ныне один из районов Лондона. Там же он и умер на 84 году жизни. Похороны его были очень пышными, на них присутствовали как многие знатные люди Англии того времени, так и толпы обычных людей.
Теперь Вы знаете самое главное из биографии Ньютона!
Автор: Ксения Бирюкова
Тут будут похожие посты. Загружаются.
Содержание
- Семья и детство
- Университет, чума и открытия
- Рождение физики как науки
- Признание и успех
- Личная жизнь
- Последние годы и смерть
- Основные достижения Ньютона
- Интересные факты
Сэр Исаак Ньютон — английский физик, математик, механик и астроном, один из создателей классической физики. Автор фундаментального труда «Математические начала натуральной философии», в котором он изложил закон всемирного тяготения и три закона механики, ставшие основой классической механики. Разработал дифференциальное и интегральное исчисления, теорию цвета, заложил основы современной физической оптики, создал многие другие математические и физические теории.
Семья и детство
Исаак Ньютон появился на свет 4 января 1643 года в небольшой британской деревушке Вулсторп, располагавшейся на территории графства Линкольншир. Его отец был из небогатых крестьян, которые волею случая нажили землю и благодаря этому преуспели. Но до рождения Исаака его отец не дожил — и умер за несколько недель до этого. Мальчика назвали в его честь.
Исаак Ньютон в детстве
Когда Ньютону было три года, его мать снова вышла замуж — за почти втрое старшего за себя богатого фермера. После рождение ещё троих детей в новом браке, Исааком начал заниматься брат его матери — Уильям Эйскоу. Но дать хоть какое-либо образование дядя Ньютону не мог, поэтому мальчик был предоставлен сам себе — играл собственноручно сделанными механическими игрушками, кроме того он был немного замкнутым.
Новый муж матери Исаака прожил с ней всего семь лет и умер. Половина наследства досталась вдове, и та сразу переписала всё на Исаака. Несмотря на то, что мать вернулась домой, внимания мальчику она почти не уделяла, поскольку младшие дети требовали его ещё больше, а помощниц у неё не было.
Двенадцатилетним Ньютон пошёл учиться в школу в соседнем городке Грэнтем. Чтобы каждый день не возвращаться несколько миль домой, его поселили в доме у местного аптекаря мистера Кларка. В школе мальчик «расцвёл»: он жадно хватался за новые знания, учителя были в восторге от его ума и способностей. Но уже через четыре года матери потребовался помощник и она решила, что 16-летний сын вполне сможет справиться с фермой.
Но даже вернувшись домой, Исаак не спешит решать хозяйственные проблемы, а читает книги, пишет стихи и продолжает заниматься придумыванием различных механизмов. Поэтому знакомые обратились к его матери, чтобы та вернула парня в школу. Был среди них и преподаватель Тринити-колледжа, знакомый того самого аптекаря, у которого Исаак жил во время учёбы. Общими усилиями Ньютон поехал поступать в Кембридж.
Университет, чума и открытия
В 1661 году парень успешно прошёл экзамен с латыни, и его зачислили в колледж Святой Троицы при Кембриджском университете как студента, который вместо оплаты за учёбу выполняет разные поручение и работы на благо альма матер.
Поскольку жизнь в Англии в те годы была весьма тяжёлой, то не лучшим делом обстояли дела и в Кембридже. Биографы сходятся на мысли, что именно годы в колледже закалили характер учёного и его желание доходить до сути предмета собственными усилиями. Через три года он уже добился стипендии.
В 1664 году одним из преподавателей Ньютона стал Исаак Барроу, который привил ему любовь к математике. В те годы Ньютон делает своё первое открытие в математике, известное сейчас как Бином Ньютона.
Через несколько месяцев учёбу в Кембридже прекратили из-за эпидемии чумы, которая разрасталась в Англии. Ньютон вернулся домой, где продолжал свои научные труды. Именно в те годы он начал разрабатывать закон, который со времен получил имя Ньютона-Лейбница; в родном доме он открыл, что белый цвет — не что иное, как смесь всех цветов, и назвал явление «спектром». Тогда же он открыл свой известный закон всемирного тяготения.
То, что было чертой Ньютоновского характера, и было не слишком полезно для науки — это его излишняя скромность. Некоторые свои исследования он публиковал лишь через 20-30 лет после их открытий. Некоторые нашлись спустя три столетия после его смерти.
В 1667 Ньютон вернулся в колледж, а через год стал магистром, его пригласили поработать преподавателем. Но читать лекции Исааку было не слишком по душе, да и особенной популярностью среди учеников он не пользовался.
В 1669 году разные математики начали публиковать свои варианты разложений в бесконечные ряды. Несмотря на то, что Ньютон разработал свою теорию на эту тему уже много лет назад, он её нигде не публиковал. Опять-таки из-за скромности. Но его бывший преподаватель, а теперь уже и друг Барроу уговорил Исаака. И тот написал «Анализ с помощью уравнений с бесконечным числом членов», где изложил коротко и по сути свои открытия. И хотя Ньютон просил не называть своего имени, Барроу не удержался. Так о Ньютоне впервые узнали ученые всего мира.
В этом же году он переходит на место Барроу и становится профессором математики и оптики в колледже Святой Троицы. А поскольку Барроу оставил ему свою лабораторию, Исаак увлекается алхимией и проводит много опытов на эту тему. Но не оставил он и исследование со светом. Так, он разработал свой первый телескоп-рефлектор, который давал увеличение в 40 раз. Новой разработкой заинтересовались при дворе короля, и после презентации перед учёными, механизм оценили как революционный и очень необходимый, особенно для мореплавателей. А Ньютона в 1672 году приняли в Королевское научное общество. Но уже после первой полемики о спектре, Исаак решил покинуть организацию — его утомляли споры и дискуссии, он привык работать в одиночку и без лишней суеты. Его едва удалось уговорить остаться в Королевском обществе, но контакты с ними у учёного стали минимальными.
Рождение физики как науки
В 1684-1686 годах Ньютон писал свой первый великий печатный труд — «Математические начала натуральной философии». Опубликовать её его уговорил ещё один учёный — Эдмонд Галлей, который сперва предложил разработать формулу эллиптического движение по орбите планет, используя формулу закона тяготения. И тут оказалось, что Ньютон уже всё давно решил. Галлей не отступил, пока не выбил из Исаака обещание опубликовать работу, и тот согласился.
Писал её два года, финансировать публикацию согласился сам Галлей, и в 1686 году она наконец увидела мир.
В этой книге учёный впервые использовал понятия «внешняя сила», «масса» и «количество движения». Ньютон давал три базовые закона механики, делал выводы из законов Кеплера.
Первый тираж в 300 экземпляров раскупили за четыре года, что по тогдашним меркам было триумфом. Всего книгу переиздавали трижды ещё при жизни учёного.
Признание и успех
В 1689 Ньютона избирают членом парламента университета Кембриджа. Ещё через год его перебирают вторично.
В 1696, благодаря содействию своего бывшего ученика, а сейчас президента Королевского общества и канцлера Казначейства Монтегю, Ньютон становится хранителем Монетного двора, для чего переезжает в Лондон. Вместе они приводят в порядок дела Монетного двора и проводят денежную реформу с перечеканкой монет.
В 1699 году в его родном Кембридже начали преподавать Ньютоновскую систему мира, ещё через пять лет такой же курс лекций появился и в Оксфорде.
Его также приняли в Парижский научный клуб, сделав Ньютона почётным иностранным членом общества.
Личная жизнь
Ньютон не оставил после себя потомков, так как никогда не был женат: всё своё свободное время он посвящал науке, а его заурядная, серая внешность делала его неприметным для женщин. Биографы упоминают лишь одну симпатию, промелькнувшую в юности Ньютона: учась в Грэнтэме, он был влюблён в мисс Сторей, свою сверстницу, с которой поддерживал тёплые, дружеские отношения до конца своих дней.
Последние годы и смерть
В 1704 Ньютон издал свой труд «Об оптике», через год королева Анна возвела его в рыцари.
Последние годы жизни Ньютона ушли на допечатку «Начал» и подготовку обновлений для следующих изданий. Кроме того он писал «Хронологию древних царств».
В 1725 году его здоровье серьёзно ухудшилось и он переехал из шумного Лондона в Кенсингтон. Умер там же, во сне. Его тело похоронили в Вестминстерском аббатстве.
Основные достижения Ньютона
- Ньютон — основатель механики, важного раздела физики.
- Ему принадлежат три закона, названные его же именем.
- Открыл закон всемирного тяготения.
- Разложил солнечный свет на спектр и обратно.
- Стал автором популярной корпускулярной теории света.
- Открыл «кольца Ньютона», изучая интерференцию света.
- В математике Ньютон стал основателем интегрального счисления.
- Автор бинома, который также носит его имя.
- Построил зеркальный телескоп.
- Объяснил с научной точки зрения движение Луны вокруг Земли и планет вокруг Солнца.
Интересные факты
- Возведение Ньютона в рыцари было первым в английской истории, когда звание рыцаря было присвоено за научные заслуги. Ньютон обзавёлся собственным гербом и не очень достоверной родословной.
- К концу жизни Ньютон рассорился с Лейбницем, что пагубно сказалось на науке британской и европейской в частности — не было сделано много открытий из-за этих ссор.
- В честь Ньютона назвали единицу силы в Международной системе единиц (СИ).
Легенда о яблоке Ньютона широко распространилась благодаря Вольтеру.
Видео
Источники
- https://ru.wikipedia.org/wiki/%D0%9D%D1%8C%D1%8E%D1%82%D0%BE%D0%BD,_%D0%98%D1%81%D0%B0%D0%B0%D0%BA
https://24smi.org/celebrity/3876-isaak-niuton.html
https://theperson.pro/isaak-nyuton/
https://stuki-druki.com/authors/Newton.php
http://biografix.ru/isaak-nyuton
История исследования космоса > Биография астрономов > Исаак Ньютон
 Биография Исаака Ньютона (1642-1727 гг.)
Биография Исаака Ньютона (1642-1727 гг.)
Краткая биография:
Имя: Исаак Ньютон
Дата рождения: 25 декабря 1642 г.
Дата смерти:20 марта 1726 г.
Образование: Кембриджский университет
Место рождения: Вулсторп, Линкольншир, Королевство Англия
Место смерти: Кенсингтон, Мидлсекс, Англия, Королевство Великобритания
Исаак Ньютон – английский астроном, физик, математик: биография с фото, идеи и классическая физика Ньютона, закон всемирного тяготения, три закона движения.
Сэр Исаак Ньютон был английским физиком и математиком из бедной фермерской семьи. Его краткая биография началась 25 декабря в 1642 году в Вулсторпе вблизи Грэнтэма в Линкольншире. Ньютон был бедным фермером и в конечном итоге его отослали в Тринити-колледж в Кембриджский университет для обучения в качестве проповедника. Обучаясь в Кембридже, Ньютон преследовал свои личные интересы и изучал философию и математику. Он получил степень бакалавра в 1665 году и позже был вынужден покинуть Кембридж, поскольку его закрыли из-за чумы. Он вернулся в 1667 году и был принят в братство. Исаак Ньютон получил степень магистра в 1668 году.
Ньютон считается одним из величайших ученых в истории. По ходу своей краткой биографии он сделал весомые вложения во многие отрасли современных наук. К сожалению, известная история Ньютона и яблока во многом основана на вымысле, нежели на реальных событиях. Его открытия и теории заложили фундамент для дальнейшего прогресса в науке с тех времен. Ньютон был одним из создателей математического раздела, который именовали как исчисление. Он также раскрыл загадку света и оптики, сформулировал три закона движения и с их помощью создал закон всемирного тяготения. Законы движения Ньютона являются одними из самых основополагающих природных законов в классической механике. В 1686 году Ньютон описал собственные открытия в его книге Principia Mathematica. Три закона движения Ньютона, в объединении, лежат в основе всех взаимодействий силы, материи и движения, помимо тех, которые включают релятивность и квантовые эффекты.
Первый закон движения Ньютона – это Закон Инерции. Если сказать кратко, он заключается в том, что объект в состоянии покоя имеет тенденцию оставаться в таком состоянии, пока на него не влияет внешняя сила.
Второй закон движения Ньютона гласит о том, что существует связь между несбалансированными силами, воздействующими на определенный объект. В результате, объект ускоряется. (Иными словами, сила равняется массе, умноженной на ускорение либо F = ma).
Третий закон движения Ньютона, также именуемый как принцип действия и реакции, описывает то, что абсолютно для каждого действия существует равноценная ему ответная реакция. После тяжелого нервного срыва в 1693 году, Ньютон отошел от собственных изучений для поиска поста губернатора в Лондоне. В 1696 году он стал ректором Королевского монетного двора. В 1708 году Ньютон был избран Королевой Анной. Он является первым ученым, настолько почтенным за свою работу. С этого момента он был известен как сэр Исаак Ньютон. Ученый посвятил большую часть своего времени теологии. Он написал большое количество пророчеств и предсказаний насчет предметов, которые были ему интересны. В 1703 году он был выбран на пост президента Королевского сообщества и был переизбран каждый год вплоть до своей смерти 20 марта 1727 года.
На чтение 8 мин Просмотров 11.7к. Опубликовано 25.01.2022
Исаак Ньютон – английский физик, механик, математик и астроном. Именно его считают одним из создателей классической физики. При этом далеко не каждому человеку известно, что конкретно изобрел Исаак Ньютон. Этот ученый является автором важного труда «Математические начала натуральной философии». Там он заложил базу законов всемирного тяготения. Там же опубликованы законы механики, которые стали базой современной науки.
Биография
Великий ученый имеет достаточно интересную биографию. Будущий математик получил хорошее образование, которое позволило ему сделать много важных открытий.
Происхождение и детство
Исаак Ньютон родился в 1642 году. Будущий ученый появился на свет в деревне Вулсторп, расположенной в графстве Линкольншир. Мальчик с детства был достаточно хилым и слабеньким. Тем не менее, он выжил и умер в почтенном для тех времен возрасте – в 84 года.
Отец мальчика скончался незадолго до его рождения. При жизни он был успешным фермером. Потому после смерти оставил много земли и приличную сумму денег. Мать Исаака в скором времени опять вышла замуж и родила еще троих детей. Женщина посвящала больше времени малышам, потому воспитанием будущего гения вначале занималась бабушка, а затем дядя.
В детские годы Ньютон занимался живописью и поэзией. Он увлекался созданием бумажных змеев и делал первые изобретения. При этом мальчик был весьма болезненным и необщительным. Все свободное время Исаак посвящал своим увлечениям.
Школа
Когда мальчику исполнилось 12 лет, он начал учиться в Грэнтемской школе. Именно там и проявились выдающиеся умственные способности мальчика. В 1659 году мать настояла, чтобы парень вернулся домой и занялся фермерством. Однако благодаря усилиям учителей, которые смогли разглядеть гениальность мальчика, он вернулся в школу.
Колледж
В 1661 году юноша приступил к обучению в Кембридже. В 1664 году Исаак успешно сдал экзамены и получил более высокую студенческую степень. В период обучения он посвящал много времени изучению трудов Коперник и Галилея. Также парня интересовала атомистическая теория Гассенди.
В 1663 году известный ученый Барроу начал читать лекции на математической кафедре. Впоследствии знаменитый математик стал ближайшим другом Исаака. Именно благодаря его работам Ньютон всерьез заинтересовался математикой.
В период обучения в колледже будущий ученый придумал свой главный математический метод, а именно – разложение функции в бесконечный ряд. По результатам обучения Ньютон получил степень бакалавра.
Дорога к науке
Во времена Ньютона система образования в Кембридже была средневековой. В тот период студенты занимались изучением астрономии Аристотеля, однако работы Коперника или Галилея не упоминались. Тем не менее, будущий ученый уже тогда интересовался новыми разработками, хотя астрономия была не единственной сферой его интересов.
В тот же период он стал увлекаться математикой, оптикой, фонетикой. Также Исаак занимался изучением теории музыки. Важным документом того периода считается список научных проблем, которые, по мнению будущего ученого, требовалось решать.
Исаак кратко обозначил то, чем исследователи впоследствии занимались 2 столетия. В список входило больше 40 пунктов. Покровителем Ньютона был известный ученый Барроу, который преимущественно занимался математикой и сразу смог оценить способности юноши. Также исследователь одобрил первое открытие Ньютона, которое стало основой нового математического метода. При этом молодой ученый получил степень бакалавра.
Затем Исаак начал разработку своей основной теории, а именно – закона всемирного тяготения. При этом молодой ученый обобщил информацию своих предшественников. Среди них стоит выделить Декарта, Галилея и Кеплера. При этом в 1665 году ученый вынужден был остановить свои изыскания, поскольку в Англии разгорелась эпидемия чумы. Именно тогда Исаак решил вернуться в Линкольншир. Чтобы продолжать работу, Ньютон взять с собой книги и инструменты.
В тот период он направил свою деятельность на открытие секретов оптики. Исследователь пытался определить, как устранить хроматическую аберрацию линзовых телескопов. Благодаря этому ученый пришел к изучению дисперсии. Суть опытов Исаака заключалась в познании физической природы света. При этом многие из экспериментов ученого по сей день проводят в образовательных учреждениях.
В результате своих работ Ньютону удалось создать корпускулярную модель света. Конечно, она не могла претендовать на полную объективность, но стала основой классической физики. Без этой теории не смогли бы появиться и современные знания о физических явлениях.
Приблизительно тогда же Исаак стал автором своего наиболее известного открытия – закона всемирного тяготения. Но исследования были опубликованы на десятки лет позже, поскольку ученый не стремился прославиться.
В конце 1660 годов Ньютон возвратился в Кембридж, в котором получил статус магистра, комнату и даже группу студентов. При этом преподавание не было сильной стороной ученого, потому его лекции посещали плохо.
В тот же период исследователь придумал телескоп-рефлектор, который позволил ему стать членом королевского общества. Созданное Ньютоном приспособление позволяло делать много астрономических открытий.
Что открыл Исаак Ньютон?
Исаак Ньютон создал много важных законов, которые внесли существенный вклад в развитие современной науки.
Три закона движения Ньютона
Самая важная работа ученого была написана в 1687 году. Она называлась «Математические начала натуральной философии». В этом труде описывалась база классической механики, которая внесла большой вклад в развитие физики.
Исаак Ньютон придумал 3 закона движения. Они были сформулированы на основе законов планетарного движения, которые разработал Иоганн Кеплер. Законы Ньютона звучали так:
- Объект в спокойном состоянии будет оставаться в покое до того момента, как на него повлияет сила, которая не имеет баланса. Объект в движении будет перемещаться с той же скоростью и в таком же направлении, если не столкнется с несбалансированной силой.
- Ускорение проявляется в момент влияния силы на массу. Чем больше масса, тем большее количество силы понадобится.
- Для каждого действия существует такое же противодействие.
Универсальная гравитация
Важным достижением Ньютона считается закон всемирного тяготения. Он установил, что каждая точка массы притягивает другую силу, которая направлена вдоль линии, пересекающей две точки. Законы гравитации позволяют измерять траектории комет, равноденствий, приливов и других явлений.
Доказательства Ньютона развенчали последние сомнения в отношении гелиоцентрической модели. При этом научное сообщество удостоверилось в том, что Земля не является центром Вселенной.
Всем известна легенда, что Ньютон придумал законы гравитации благодаря яблоку, которое упало ему на голову. Многие люди считают, что эта история является шуточной, а ученый придумал формулу другим способом. Однако в пользу этого рассказа говорят и записи в дневнике Ньютона. Также это подтверждают пересказы современников ученого.
Форма Земли
Ученый считал, что планета Земля образовалась в форме сплющенного сфероида. Впоследствии догадка не нашла подтверждения. Но во времена Ньютона эта информация имела особое значение. Она помогла перевести основную часть научного сообщества с системы Декарта на механику Ньютона.
Оптика
В 1666 году Ньютон все больше внимания уделял оптике. При этом вначале он занимался изучением характеристик света, которые измерял через призму. С 1670 по 1672 год ученый посвятил себя исследованию рефракции света. При этом Исаак демонстрировал, как цветной спектр перестраивается в одиночный белый свет посредством линзы или еще одной призмы.
В результате своих научных изысканий Ньютону удалось понять, что формирование цвета происходит вследствие взаимодействия окрашенных объектов. К тому же он понял, что объектив каждого инструмента страдает вследствие светового рассеивания. Ученому удалось решить проблемы, используя телескоп, оснащенный зеркалом. Его разработку называют первой версией отражающего телескопа. Она положительно повлияла на последующее развитие науки.
Абсолютная температура
Этот показатель также называют термодинамической температурой. Это связано с тем, что речь идет о законе термодинамики. Именно он дает определение температуры. Согласно этой теории, температура тела служит для изменения кинетической энергии частиц веществ, из которых оно состоит. Важно учитывать, что капли материи имеют значительно больше энергии по сравнению с обыкновенной кинетической энергией атомов в блоке.
Реликтовое излучение
Этим термином называют фоновое свечение Большого Взрыва. Его изучение имеет большое значение, поскольку это подтверждает тот факт, что событие действительно имело место.
Звук
Ньютон первым придумал вывод о скорости звука в газе. При этом его заключение основывалось на законе Бойля-Мариотта.
Солнечная энергия
В своей фундаментальной работе Ньютон высказал и доказал правильное предположение, что комета обладает твердым ядром. Его испарение под воздействием солнечной энергии формирует обширный хвост. При этом он всегда направляется в сторону, которая противоположна Солнцу.
Тепловая передача
Ньютон посвятил много внимания вопросам тепловой передачи. В результате ему удалось сформулировать важный закон, который получил название Ньютона-Рихмана.
Теорема Бинома
Бином Ньютона представляет собой формулу, которая используется для разложения целой неотрицательной степени суммы двух переменных на отдельные слагаемые. Причем этой формулой пользовались еще индийские и исламские математики.
Однако Ньютон вывел формулу для более общего случая, когда показатели степени представляют собой производное действительное или даже комплексное число. В таком случае бином – это бесконечный ряд.
Ньютон по праву считается величайшим ученым, который сделал много важных открытий. Самыми главными достижениями исследователя считаются сформулированные им физические законы, которые стали основой для последующего развития науки.
И. Ньютон. Портрет работы Г. Неллера. 1689.
НЬЮ́ТОН (Newton) Исаак (25.12.1642, Вулсторп – 20.3.1727, Кенсингтон, ныне район Лондона), сэр, англ. математик, механик, оптик, философ, гос. деятель; чл. (1672) и президент (1703) Лондонского королевского об-ва (ЛКО), чл. Парижской АН (1699), пэр Англии (1705). Один из создателей математич. анализа, открывшего новую эпоху в количественном описании природных явлений. Разработал основы классич. механики, физич. оптики.
Жизнь и деятельность
Н. рос в зажиточной фермерской семье. Его отец умер до рождения сына, Н. получил имя отца – Исаак. Мать вторично вышла замуж за свящ. Б. Смита, к которому переехала, оставив Н. на воспитание своим родителям – М. и Дж. Аскью. В 1661, после окончания средней школы и при поддержке её директора, Н. был направлен в Тринити-колледж Кембриджского ун-та, где в 1665 получил степень бакалавра, в 1668 – степень магистра, в 1669 стал проф. кафедры математики.
С авг. 1665 по март 1667 ун-т был закрыт из-за эпидемии чумы. Вынужденные каникулы Н. проводил в имении матери, размышляя об основах математич. анализа, разрабатывая теорию удара и проводя исследования в области оптики. В 1668 он создал первый телескоп-рефлектор, конструкцию которого смог существенно улучшить к 1671. Это изобретение принесло ему междунар. славу и стало основанием для избрания в ЛКО, членство в котором позволило опубликовать результаты своих исследований в 1672 в ст. «Новая теория света и цветов» («New theory about light and colours») в ж. «Philosophical Transactions», издаваемом ЛКО.
И. Ньютон. Портрет работы Дж. Торнхилла. 1712.
С сер. 1670-х гг. Н. полностью прекратил занятия естеств. науками, отказался от всякой науч. переписки и контактов с коллегами по ЛКО, полностью посвятив себя алхимии, теологии и библейской истории. Будучи официально членом Англиканской церкви, Н., однако, в результате систематич. изучения Библии, трудов ранних отцов Церкви и истории арианских споров (см. Арианство) подверг критике догмат Троицы, считая, что лат. переводы Священного Писания были искажены в пользу тринитарного толкования по сравнению с греч. оригиналами.
Поводом для возвращения к науч. занятиям послужило письмо, полученное в 1679 от Р. Гука, который предложил Н. принять участие в обсуждении задач, занимавших ЛКО. К таким задачам, в частности, относилась задача о движении свободно падающего тяжёлого тела.
В 1684 в Кембридж приехал Э. Галлей, чтобы обсудить с Н. возможность выведения Кеплера законов из общих принципов механики. Н. заявил, что эта задача была решена им ещё 4 года назад, и чуть позднее прислал Галлею 9-страничный трактат «О движении тел по орбите» («De Motu Corporum in Gyrum»). Поняв, что имеет дело с гениальным сочинением, Галлей пытался склонить Н. к изданию работы. Однако Н. не соглашался на скорое издание, продолжая упорную работу над проблемой. За 3 года 9-страничный трактат преобразился в фундам. труд «Математические начала натуральной философии» («Philosophiae naturalis principia mathematica», опубл. в 1687), в котором законы природы были сформулированы языком математики. 1-е издание «Начал…» вышло в 1687, открыв новый период в истории науки. Б. ч. хлопот по подготовке издания взял на себя Галлей.
Надгробный памятник И. Ньютону в Вестминстерском аббатстве в Лондоне.
В 1689 Н. был в первый раз избран в парламент (от Кембриджского ун-та) и заседал там немногим более года. 1690-е гг. в жизни Н. были отмечены творческим и общим спадом; он много болел и полностью отошёл от исследовательской работы. Однако на рубеже 17–18 вв. Н. нашёл себя в новом деле: в 1696 он перебрался в Лондон и стал смотрителем монетного двора, а в 1699 его директором. Столь неожиданное назначение было связано с тем, что у Н. появились высокопоставленные покровители (среди них – будущий премьер-министр Ч. Монтегю граф Галифакс и Дж. Локк). В этой должности Н. добился приведения в порядок расстроенной финансовой системы страны и преодоления последствий глобального кризиса. Оставшиеся годы он провёл, занимаясь делами ЛКО и публикуя свои рукописи. В 1704 был издан большой трактат «Оптика или трактат об отражениях, преломлениях, изгибаниях и цветах света» («Opticks, or A treatise of the reflexions, refractions, inflexions and colours of light», опубл. на англ. языке, в отличие от предыдущих трудов, написанных на латыни), в 1713 подготовлено 2-е издание «Начал…» (3-е издание, последнее при жизни Н., увидело свет в 1726). В 1701–02 Н. вновь заседал в парламенте. В 1703 Н. стал президентом ЛКО, в 1705 получил титул лорда. Похоронен в Вестминстерском аббатстве.
Работы в области математики
Математика для Н. была гл. инструментом в физич. изысканиях; он считал, что понятия математики возникают как абстракции явлений и процессов реального мира. Разработка Н. дифференциального и интегрального исчислений явилась важнейшим этапом развития математики. Осн. идеи флюксий исчисления сложились у Н. в 1665–66 под влиянием его предшественников и современников.
Титульный лист первого издания труда «Математические начала натуральной философии» (1687).
В исходных понятиях и терминологии метода флюксий отразилось влияние идей, развитых рядом учёных 17 в. – Б. Кавальери, П. Ферма, Дж. Валлисом; в этих понятиях отчётливо проявилась связь между математич. и механич. исследованиями. Понятие непрерывной математич. величины Н. ввёл как абстракцию от разл. видов непрерывного механич. движения. Линии можно получать движением точек, поверхности – движением линий, тела – движением поверхностей, углы – вращением сторон, и т. д. Непрерывные переменные величины Н. назвал флюентами (текущими величинами, от лат. fluo – течь). Общим аргументом разл. текущих величин – флюент – у Н. является «время», понимаемое формально как некая отвлечённая равномерно текущая величина, к которой отнесены прочие зависимые переменные. Флюента – изменяющаяся со временем величина, изменение которой можно изобразить линией в декартовых координатах. Скорости изменения флюент Н. назвал флюксиями, а необходимые для вычисления флюксий бесконечно малые изменения флюент – моментами (у Г. В. Лейбница, который достиг в дифференциальном и интегральном исчислениях примерно тех же результатов, что и Н., почти одновременно и независимо от него, они называются дифференциалами). Н. вычислил (1669, опубл. в 1711) производную и интеграл любой степенной функции. Разл. рациональные, в т. ч. дробно-рациональные функции, функции, содержащие радикалы, и некоторые трансцендентные функции (логарифмическую, показательную, синус, косинус, арксинус) Н. выражал с помощью бесконечных степенных рядов. Метод вычисления и изучения функций с помощью рядов приобрёл огромное значение для всего математич. анализа и его приложений.
Телескоп-рефлектор И. Ньютона, хранящийся в Лондонском королевском обществе.
В кон. 1660-х гг. Н. сформулировал две осн. взаимно обратные задачи математич. анализа: 1) определение скорости движения в данный момент времени по известному пройденному пути (задача дифференцирования), или определение соотношения между флюксиями по данному соотношению между флюентами; 2) определение пройденного за данное время пути по известной скорости движения (задача интегрирования дифференциального уравнения, в частности отыскания первообразной), или определение соотношения между флюентами по данному соотношению между флюксиями. Метод флюксий применялся Н. к большому числу геометрич. вопросов (задачи на касательные, кривизны, экстремумы, квадратуры, спрямления). Н. наметил, по существу, программу построения метода флюксий на основе понятий о «последних отношениях исчезающих величин» или «первых отношениях зарождающихся величин», не давая их формального определения и рассматривая их как интуитивно очевидные. Они нашли своё строгое обоснование в понятии предела, развитом математиками 2-й пол. 18 и 19 вв. (Ж. Д’Аламбер, Л. Эйлер, О. Коши и др.).
В кон. 1660-х гг. были написаны и др. сочинения Н. по математич. анализу, изданные значительно позднее. Был разработан метод вычисления корней уравнения (Ньютона метод) и один из безусловной минимизации методов. Некоторые математич. открытия Н. получили известность в 1670-х гг. по его рукописям и переписке. Большое значение имели также его работы по алгебре, геометрии и интерполяции. При решении мн. математич. задач используется Ньютона бином.
Работы в области механики
Сформулировав 3 аксиомы динамики (Ньютона законы механики) и дополнив их всемирного тяготения законом, Н. заложил основания теоретич. механики и предопределил пути её развития на последующие 200 лет. Он ввёл осн. понятия механики: масса, сила, количество движения и др. Механика, опирающаяся на положения, выдвинутые Н., называется классической или ньютоновской. Пользуясь преим. геометрич. методами, Н. показал, что траектория материальной точки в сферически-симметричном центральном поле будет представлять собой плоскую кривую, причём за равные промежутки времени радиус-вектор будет заметать равные углы (т. е. будет выполняться 2-й закон Кеплера).
Н. рассмотрел также движение материальной точки в сопротивляющейся среде, проводя различие между сухим трением, при котором сила трения не зависит от скорости движения, и вязким, при котором сила трения пропорциональна скорости или её квадрату. Переходя от этих задач к движению среды как таковой, Н. дал одну из первых оценок скорости звука в упругой среде, фактически положив начало физич. акустике. При этом он воспользовался аналогией между движениями упругой среды и физич. маятника. Н. дал новое решение задачи об изохронности колебаний маятника, показав, что для того, чтобы период маятника не зависел от амплитуды, конец маятника должен двигаться по циклоиде.
Н. проводил исследования по теории удара, которая в 17 в. считалась одной из ключевых проблем механики. Достигнутые результаты, в частности, позволили Н. вычислить центростремительное ускорение и центробежную силу (решая эту задачу, Н. заменил движение по окружности движением по правильному многоугольнику с упругими столкновениями в каждой вершине). Найденное решение позволило Н. утверждать, что 3-й закон Кеплера будет выполняться в том и только в том случае, когда центробежная сила убывает обратно пропорционально квадрату расстояния от центра. Решения этих и мн. др. задач механики были опубликованы Н. в его гл. сочинении – «Математические начала натуральной философии».
Особое место в этом труде заняло обсуждение случаев, когда законы Кеплера нарушаются: рассмотрение лунных вариаций, прецессии земной орбиты, несферичности формы Земли и др. Вывод Н. о том, что из-за суточного вращения Земля должна быть сплющена с полюсов, вызвал длинную и бурную дискуссию. Окончательно этот вывод был подтверждён после проведения в 1736–37 меридиональных измерений (экспедиция под рук. П. Л. Мопертюи) и публикации в 1743 труда А. К. Клеро «Теория фигуры Земли».
Работы в области оптики
К осн. достижениям Н. в области оптики относятся: эксперим. доказательство составного характера белого цвета и дальнейшей неразложимости осн. цветов спектра, построение первого телескопа-рефлектора, обнаружение новых явлений, связанных с волновой природой света (в частности, Ньютона колец), и разработка дуалистической теории света.
Интерес Н. к оптич. явлениям был вызван некоторыми новыми эффектами, обнаруженными в 17 в. Так, благодаря развитию типографских методов цветной печати, опытным путём было установлено, что тремя красками можно воспроизвести практически любой оттенок цвета. Дать объяснение этому явлению не удавалось, так же как и эффекту окрашивания изображения в зрительной трубе (известному сейчас как хроматич. аберрация).
Свои первые оптич. опыты Н. проводил с треугольной призмой, получая спектральное разложение солнечного света на вертикальной стене комнаты. Из этих опытов Н. сделал ключевой вывод о том, что призма не окрашивает солнечный свет, а разлагает его на составляющие. Н. полагал, что солнечный свет представляет собой смесь лучей разных цветов, причём «лучи, различающиеся по цвету, различаются и по степени преломления», а каждому цвету отвечает поток корпускул определённой скорости.
Из заключения об однозначной зависимости скорости корпускул и степени преломления следовала, в частности, невозможность избавиться от хроматич. аберрации в телескопах-рефракторах, что подтолкнуло Н. к созданию принципиально новой конструкции телескопа. В результате в 1668 Н. создал телескоп-рефлектор, в котором эффект увеличения удалённых объектов достигался за счёт их отражения в вогнутом сферич. зеркале.
Учение Н. о свете систематизировало знания той эпохи и послужило быстрому прогрессу оптики. В то же время оно содержало некоторые ошибочные положения и стало предметом ожесточённой критики современников. Так, напр., Н. полагал дифракцию разновидностью рефракции и поэтому отрицал возможность попадания света в область тени, считал, что изменение угла преломления для лучей разных цветов не зависит от свойств стекла. Наиболее последовательная и аргументированная критика учения Н. исходила от Р. Гука, который точно воспроизвёл все описанные Н. эксперименты, но предложил им иную интерпретацию. Часто расхождение теоретич. позиций Гука и Н. представлялось как оппозиция волновой и корпускулярной теорий света.
Гл. сложность позиции Н. заключалась в дуалистичности его теории. Свет, по его словам, был подобен одновременно и камню, брошенному в воду, и волнам, вызванным падением камня и расходящимся по поверхности воды. Однако принять волновую теорию своих оппонентов Н. не мог, т. к. не видел возможности объяснить в её рамках прямолинейность световых лучей (это удалось значительно позднее О. Френелю). Противоречия между волновой и корпускулярной теориями света были сняты только в 20 в. при создании квантовой электродинамики.
Философские взгляды
Придерживаясь установок брит. эмпиризма, Н. противопоставил «самоочевидным истинам разума» Р. Декарта и всей рационалистич. традиции свою науч. программу «экспериментальной философии», опирающуюся в исследовании природы прежде всего на реальный (не только мысленный) эксперимент и метод индукции. Сформулированный в «Оптике» метод Н. заключался в сочетании анализа (понимаемого как «производство опытов и наблюдений, извлечение общих заключений из них посредством индукции и недопущение иных возражений против заключений, кроме полученных из опыта или других достоверных истин») и синтеза [переход «от соединений к ингредиентам, от движений к силам, их производящим, и вообще от действий к их причинам, от частных причин к более общим, пока аргумент не закончится наиболее общей причиной» (Оптика или трактат об отражениях, преломлениях, изгибаниях и цветах света. М., 1927. С. 306)]. При этом в качестве такой общей причины, позволяющей не только математически описать движение как земных, так и небесных тел, но и объяснить все физич. явления в рамках единой картины мира, выступает у Н. введённое им понятие силы тяготения, которая, однако, выходит за рамки механики: «…причину… свойств силы тяготения я до сих пор не мог вывести из явлений, гипотез же я не измышляю» (Математические начала натуральной философии. М., 1989. С. 662).
Первоначально природу тяготения Н. объяснял с помощью гипотезы эфира как «тончайшей», всепроникающей среды, в которой возможна передача разл. сил как в неживой, так и живой природе – гравитационное притяжение, химич. процессы, световые, электростатич. явления, теплота, звук, отправления живого организма. Понятие эфира, восходящее к пневме стоиков и мировой душе неоплатоников, было воспринято Н. в русле эзотерич. учений 16–17 вв., получивших распространение в т. ч. в алхимии («жизненный дух», spiritus mundi и т. п.), которой Н. занимался ок. 30 лет, исследуя возможности трансмутации металлов (сохранилось огромное количество текстов Н., содержащих конспекты алхимич. сочинений и его комментарии к ним, а также описания его собств. опытов). При этом эфир, «мировое дыхание», Н. мыслил как бестелесное бесконечное пространство, отвергая вслед за Г. Мором, оказавшим влияние на молодого Н., отождествление материи и протяжённости (пространства) у Р. Декарта. В полемике с Декартом, атомистами (П. Гассенди) и Г. В. Лейбницем Н. ввёл понятие единого, неделимого, абсолютного пространства – нематериального «вместилища» всего, что существует в физич. мире, а также всегда одинакового абсолютного времени и абсолютного движения, отличая их от воспринимаемых нашими чувствами относительных пространства, времени и движения. Абсолютное пространство рассматривается Н. как «чувствилище Бога» (sensorium Dei), который «управляет всем не как душа мира, а как властитель вселенной», Пантократор.
Математич. естествознание Н. быстро завоевало признание в Великобритании и начало распространяться в Европе, где ему противостояла науч. программа Г. В. Лейбница – Х. фон Вольфа. Однако у ньютонианцев в 18 в. закрепилось и абсолютизировалось представление о ньютоновской науч. программе как исключительно эмпирической, из неё, в сущности, полностью элиминировалось её филос. ядро (так, напр., Э. Б. де Кондильяк и др. считали, что принцип тяготения был получен Н. из опыта). Огромную роль в распространении физики Н. на континенте сыграли Вольтер и др. просветители, и, наряду с философией Дж. Локка, науч. программа Н. стала знаменем Просвещения как в самой Великобритании, так и на континенте, прежде всего во Франции.
Деятельность во главе монетного двора
В кон. 17 в. англ. финансовая система была практически разрушена. Номинальная цена англ. денег оказалась значительно ниже стоимости металла, из которого изготовлялись монеты. Контрабандисты большими партиями вывозили на материк англ. серебряные монеты машинной чеканки (введённые в оборот после реформы 1663), чтобы продавать их там переплавленными в слитки. Остающиеся в обороте старые монеты ручной чеканки, не имевшие насечек на ребре, при использовании теряли в весе (как за счёт стирания края, так и за счёт воровства металла). Доверие к англ. валюте дополнительно подрывалось заметным вбросом фальшивых денег. Торговля в 1690-х гг. стала практически невозможной из-за отсутствия денег, при помощи которых её можно было бы вести.
Для выхода из сложившегося положения необходимо было провести новую крупномасштабную денежную реформу, в частности перечеканить всю серебряную монету, изъяв ту, что имела хождение в стране до реформы. Именно эта задача и была возложена на Н., которому удалось успешно с ней справиться. Т. к. при имевшихся мощностях монетного двора перечеканка монеты должна была растянуться на 9 лет, Н. настоял на закупке нового оборудования, переходе к круглосуточному режиму работы и создании дополнит. монетных дворов. Т. о., скорость изготовления монет выросла в 8 раз. Недостающее для чеканки серебро закупалось в счёт гос. долга. Кроме того, Н. предложил несколько довольно эффективных мер против фальшивомонетчиков.
Распространение идей Ньютона в России
Для библиотеки Петра I был куплен экземпляр 1-го издания осн. труда Н. «Математические начала натуральной философии». После смерти императора этот экземпляр хранился в библиотеке АН, а в 1787 был подарен библиотеке Моск. ун-та.
Долгое время работы Н. не переводились и оставались знакомы только людям, умевшим читать по латыни. В 19 в., по мере того как латынь переставала быть языком междунар. общения учёных, возникла необходимость в переводах и пропаганде наследия Н. в России. Первый перевод «Начал…» на рус. язык был выполнен в 1916 А. Н. Крыловым.
«Оптика» была переведена на рус. язык С. И. Вавиловым и издана в 1927 под заголовком «Оптика или трактат об отражениях, преломлениях, изгибаниях и цветах света», а в 1946 появились в том же переводе и «Лекции по оптике». Вавилов написал также первую на рус. языке обстоятельную биографию Н. (1943). По инициативе Вавилова и при его непосредственном участии в Казани в 1943 прошли заседания, посвящённые 300-летию Н. Большое значение для отеч. ньютоноведения имела и междунар. конференция, посвящённая 300-летию «Начал…», проведённая в 1987 в Москве.


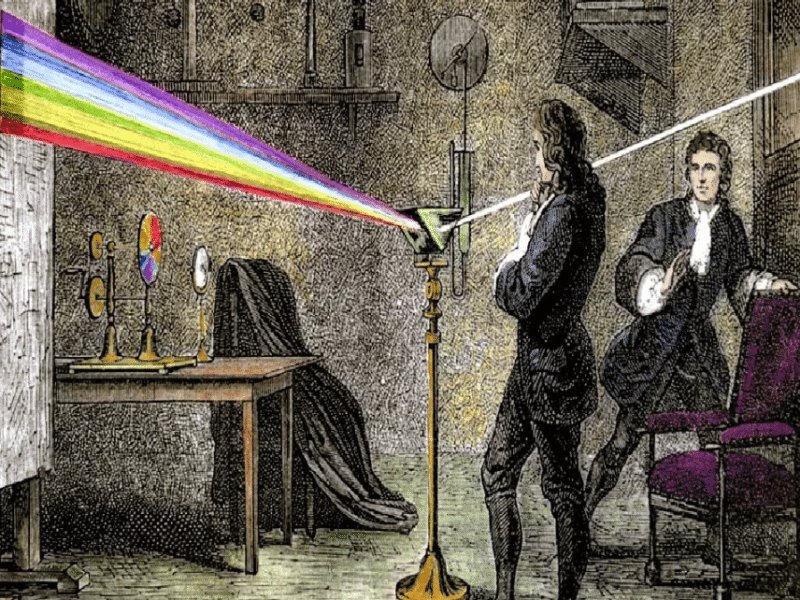







































 Биография Исаака Ньютона (1642-1727 гг.)
Биография Исаака Ньютона (1642-1727 гг.)


















